Gigi earned $65 for 5 hours of gardening. She earned $90 for 9 hours of office work. Which statement correctly compares Gigi’s earning per hour for gardening and office work?
a. She earned $3 more per hour for office work than for gardening.
b. She earned $4 more per hour for office work than for gardening.
c. She earned $3 more per hour for gardening than for office work.
d. She earned $4 more per hour for gardening than for office work.
Answers
C. She earned $3 per hour for gardening than for office work. You have to find the unit rate of how much she made per hour then subtract it.
90/9=10
65/5=13
13-10=3
Answer:
C
Step-by-step explanation:
Related Questions
79/40-162.5% enter the answer as an exact decimal or simplified fraction. please fast
Answers
This fraction can be simplified by dividing both the numerator and denominator by their greatest common divisor, the exact decimal or simplified fraction solution is \(\frac{7}{20}\).
To solve the expression \(\frac{79}{40}\) - 162.5%, we first need to convert the percentage to a decimal.
To convert a percentage to a decimal, we divide it by 100.
So, 162.5% becomes \(\frac{162.5}{100}\) = 1.625.
Now, we can rewrite the expression as \(\frac{79}{40}\) - 1.625.
To subtract fractions, we need a common denominator.
In this case, the least common multiple (LCM) of 40 and 1 is 40.
So, we need to rewrite both fractions with the denominator of 40.
For the first fraction, \(\frac{79}{40}\), we can multiply both the numerator and denominator by 1 to keep it the same.
For the second fraction, 1.625, we can multiply both the numerator and denominator by 40 to get \(\frac{65}{40}\)
Now we can subtract the fractions:
\(\frac{79}{40} - \frac{65}{40} = \frac{79-65}{40}\)
= \(\frac{14}{40}\)
To know more about decimal visit:
https://brainly.com/question/30958821
#SPJ11
\(\frac{79}{40} - 162.5\%\) is equal to \(\frac{7}{20}\) or \(0.35\) as a decimal. To solve the expression \(\frac{79}{40}-162.5\%\), we have to follow some step.
Steps to solve the expression:
1. Convert the percentage to a decimal: \(162.5\% = \frac{162.5}{100} = 1.625\)
2. Now, we have \(\frac{79}{40}-1.625\).
3. In order to subtract fractions, we need a common denominator. The least common denominator (LCD) for 40 and 1 is 40.
4. Rewrite the fractions with the common denominator:
\(\frac{79}{40}-1.625 =\frac{79}{40}- (1.625 * \frac{40}{40})\)
\(= \frac{79}{40} - \frac{65}{40}\)
5. Subtract the fractions:
\(\frac{79}{40} - \frac{65}{40} = \frac{79-65}{40}\)
\(= \frac{14}{40} \)
6. Simplify the fraction by dividing both the numerator and denominator by their greatest common divisor (GCD), which is 2 in this case:
\( \frac{14}{40} = \frac{(\frac{14}{2})}{(\frac{40}{2})}\)
\(= \frac{7}{20}\)
Therefore, the simplified answer to \(\frac{79}{40}-162.5\%\) is \(\frac{7}{20}\) or \(0.35\) as a decimal.
In conclusion, \(\frac{79}{40}-162.5\%\) is equal to \(\frac{7}{20}\) or \(0.35\) as a decimal.
Learn more about fractions from the given link:
https://brainly.com/question/10354322
#SPJ11
Select the correct answer.
If you roll a fair die twice, what is the probability of getting an even number at least once?
A.
0.75
B.
0.36
C.
0.5
D.
0.25
Answers
Answer:
A
Step-by-step explanation:
Got this right on edmentum
in the same distribution (mean is 70 and the standard deviation is 8. at least what fraction are between the following pairs
Answers
At least 68% of the data falls between 62 and 78, and at least 95% of the data falls between 54 and 86.
How to find the minimum fraction of the data?To answer this question, we can use the empirical rule, also known as the 68-95-99.7 rule, which tells us that for a normal distribution:
About 68% of the data falls within one standard deviation of the meanAbout 95% of the data falls within two standard deviations of the meanAbout 99.7% of the data falls within three standard deviations of the meanUsing this rule, we can estimate the fraction of the data that falls between the following pairs:
Between 54 and 86:
To find the range of values that is within two standard deviations of the mean, we can subtract and add two standard deviations from the mean:
Lower bound: 70 - 2*8 = 54
Upper bound: 70 + 2*8 = 86
So, about 95% of the data falls between 54 and 86.
Between 62 and 78:
To find the range of values that is within one standard deviation of the mean, we can subtract and add one standard deviation from the mean:
Lower bound: 70 - 8 = 62
Upper bound: 70 + 8 = 78
So, about 68% of the data falls between 62 and 78.
Therefore, we can conclude that at least 68% of the data falls between 62 and 78, and at least 95% of the data falls between 54 and 86.
Learn more about empirical rule
brainly.com/question/30700783
#SPJ11
PLZ HELP ASAPPPP PLZZZ HELPPP PLZZZ I GIVE BRAINLEST

Answers
Jon and Lexi are playing a marble game. According to their rules, 4 points are awarded for each red marble collected and 3 points are awarded for each blue marble collected. Which expression represents Jon's total score if he has r red marbles and b blue marbles?
4r − 3b
2r − 3b
4r + 3b
7rb
Answers
Answer:
i believe the answer is 4r - 3b
Answer:
4r+3b
Step-by-step explanation:
4 red awarded = 4r
3 blue awarded = 3b
Score = 4r + 3b
a:b = 9:4 and 10b = 7c
work out a:c in its simplest form
Answers
10(4) = 7 C
40 = 7 C Divide by 7
C = 40/7
A:C is = 9 : 40/7
marks] b. Given the P3(x) as the interpolating polynomial for the data points (0,0), (0.5,y),(1,3) and (2,2). Determine y value if the coefficient of x3 in P3(x) is 6. [5 Marks)
Answers
The value of y.\($$P_3(0.5)=6(0.5)^3+\frac{5-8y}{2}(0.5)^2+\frac{23-8y}{2}(0.5)=\frac{3}{4}y+\frac{17}{4}$$$$\Rightarrow \frac{3}{4}y+\frac{17}{4}=0.75b+1.5c+3$$$$\Rightarrow \frac{3}{4}y+\frac{17}{4}=0.75(\frac{5-8y}{2})+1.5(\frac{23-8y}{2})+3$$$$\Rightarrow y=-\frac{3}{4}$$\)Hence, the value of y is -3/4.
Given that P3(x) is the interpolating polynomial for the data points (0,0), (0.5,y), (1,3) and (2,2).
We need to find the y value if the coefficient of \(x3 in P3(x)\)is 6.Interpolation is the process of constructing a function from given discrete data points. We use the interpolation technique when we have a set of data points, and we want to establish a relationship between them.To solve the given problem, we need to find the value of the polynomial P3(x) for the given data points. The general expression for a polynomial of degree 3 can be written as:
\($$P_3(x)=ax^3+bx^2+cx+d$$\)
To find P3(x), we can use the method of Lagrange Interpolation, which is given by:
\($$P_3(x)=\sum_{i=0}^3y_iL_i(x)$$\)
where
\($L_i(x)$\)is the Lagrange polynomial. We have three data points, so we get three Lagrange polynomials\(:$$\begin{aligned} L_0(x)&=\frac{(x-0.5)(x-1)(x-2)}{(0-0.5)(0-1)(0-2)} \\ L_1(x)&=\frac{(x-0)(x-1)(x-2)}{(0.5-0)(0.5-1)(0.5-2)} \\ L_2(x)&=\frac{(x-0)(x-0.5)(x-2)}{(1-0)(1-0.5)(1-2)} \\ L_3(x)&=\frac{(x-0)(x-0.5)(x-1)}{(2-0)(2-0.5)(2-1)} \\ \end{aligned}$$\)Now, we can substitute these values in the equation of $P_3(x)$:\($$P_3(x)=y_0L_0(x)+y_1L_1(x)+y_2L_2(x)+y_3L_3(x)$$We know that the coefficient of x3 in P3(x\)) is 6. Therefore, the equation of P3(x) becomes:\($$P_3(x)=6x^3+bx^2+cx+d$$\)
Now we substitute the given values in the equation of $P_3(x)$ to get the value of y. The given data points are (0, 0), (0.5, y), (1, 3), and (2, 2).When we substitute (0, 0) in $P_3(x)$, we get:\($$P_3(0)=6(0)^3+b(0)^2+c(0)+d=0$$\)Hence, d=0.When we substitute (0.5, y) in $P_3(x)$, we get:\($$P_3(0.5)=6(0.5)^3+b(0.5)^2+c(0.5)=0.75b+1.5c+3=y$$$$\Rightarrow 0.75b+1.5c=-3+y$$\)When we substitute (1, 3) in $P_3(x)$, we get:\($$P_3(1)=6(1)^3+b(1)^2+c(1)=6+b+c=3$$$$\Rightarrow b+c=-3$$\)When we substitute (2, 2) in $P_3(x)$, we get:\($$P_3(2)=6(2)^3+b(2)^2+c(2)=48+4b+2c=2$$$$\\)Rightarrow 4b+2c=-23$$We can solve the above three equations simultaneously to get the values of b and c.$$b+c=-3\ldots(1)\($$$$0.75b+1.5c=-3+y\ldots(2)$$$$4b+2c=-23\ldots(3)$$\)Multiplying equation (1) by 0.5, we get:$$0.5b+0.5c=-1.5\ldots(4)$$Subtracting equation (4) from equation (2), we get:$$0.25b+0.5c=y-1.5\($$$$\Rightarrow 2b+4c=4y-12\ldots(5)$$\)Substituting equation (1) in equation (5), we get:\($$2b-6=4y-12\Rightarrow 2b=4y-6$$\)Substituting this value in equation (3), we get:$$8y-24+2c=-23\Rightarrow c=\frac{23-8y}{2}$$Substituting this value of c in equation (1), we get:$$b+\frac{23-8y}{2}=-3\($$$$\Rightarrow b=\frac{5-8y}{2}$$\)Now, we substitute the values of b and c in $P_3(x)$:\($$P_3(x)=6x^3+\frac{5-8y}{2}x^2+\frac{23-8y}{2}x$$\)The coefficient of x3 in P3(x) is 6.
Hence,\($$6=\frac{6}{2}\Rightarrow a=1$$$$\Rightarrow P_3(x)=6x^3+\frac{5-8y}{2}x^2+\frac{23-8y}{2}x$$\)We can now substitute x=0.5 in $P_3(x)$ and get the value of y.\($$P_3(0.5)=6(0.5)^3+\frac{5-8y}{2}(0.5)^2+\frac{23-8y}{2}(0.5)=\frac{3}{4}y+\frac{17}{4}$$$$\Rightarrow \frac{3}{4}y+\frac{17}{4}=0.75b+1.5c+3$$$$\Rightarrow \frac{3}{4}y+\frac{17}{4}=0.75(\frac{5-8y}{2})+1.5(\frac{23-8y}{2})+3$$$$\Rightarrow y=-\frac{3}{4}$$\)Hence, the value of y is -3/4. Answer: The value of y is -3/4.
To know more about polynomials visit:-
https://brainly.com/question/28813567
#SPJ11
Velocity of a Car A car moves along a straight road in such a way that its velocity (in feet per second) at any time t (in seconds) is given by v(t) = 3t√ 81-t² (0 ≤ t ≤ 9). Find the distance traveled by the car in the 9 sec from t = 0 to t = 9. ___ ft
Answers
To find the distance traveled by the car in the time interval from t = 0 to t = 9 seconds, we need to integrate the velocity function over that interval.
The velocity function is given by \(\(v(t) = 3t \sqrt{81 - t^2}\).\)
To find the distance traveled, we integrate the absolute value of the velocity function over the interval [0, 9]:
\(\[\text{{Distance}} = \int_{{0}}^{{9}} |v(t)| \, dt\]\)
Since the velocity function \(\(v(t)\)\) is non-negative over the interval [0, 9], we can simply integrate the velocity function from 0 to 9:
\(\[\text{{Distance}} = \int_{{0}}^{{9}} v(t) \, dt\]\)
Let's calculate the distance traveled:
\(\[\text{{Distance}} = \int_{{0}}^{{9}} 3t \sqrt{81 - t^2} \, dt\]\)
We can simplify this integral using a substitution. Let's substitute \(\(u = 81 - t^2\) and \(du = -2t \, dt\). When \(t = 0\), \(u = 81\), and when \(t = 9\), \(u = 0\)\). The integral becomes:
\(\[\text{{Distance}} = \int_{{81}}^{{0}} -\frac{3}{2} \sqrt{u} \, du\]\)
Now we can evaluate the integral:
\(\[\text{{Distance}} = -\frac{3}{2} \int_{{81}}^{{0}} \sqrt{u} \, du\]\)
We can reverse the limits of integration and change the sign:
\(\[\text{{Distance}} = \frac{3}{2} \int_{{0}}^{{81}} \sqrt{u} \, du\]\)
Integrating \(\(\sqrt{u}\) with respect to \(u\)\) gives us:
\(\[\text{{Distance}} = \frac{3}{2} \left[ \frac{2}{3} u^{3/2} \right]_{{0}}^{{81}}\]\)
Evaluating the integral at the limits:
\(\[\text{{Distance}} = \frac{3}{2} \left[ \frac{2}{3} (81)^{3/2} - \frac{2}{3} (0)^{3/2} \right]\]\)
Simplifying:
\(\[\text{{Distance}} = \frac{3}{2} \left( \frac{2}{3} \cdot 81^{3/2} - 0 \right)\]\)
\(\[\text{{Distance}} = 3 \cdot 81^{3/2}\]\)
Evaluating the expression:
\(\[\text{{Distance}} = 3 \cdot 9^3 = 3 \cdot 729 = 2187 \text{{ ft}}\]\)
Therefore, the distance traveled by the car in the 9 seconds from t = 0 to t = 9 is 2187 ft.
To know more about convergence visit-
brainly.com/question/31968534
#SPJ11
A company has a multi-tier investment. They have $40 million in operating expenses each year and a cost of capital of 12%. They can invest $12 million in new technology for their plant. There is a 40% chance that the new equipment reduces expenses by 15%, a 30% chance it reduces expenses by 10%, and a 30% chance it reduces expenses by 5%. Shareholders will view the results after 3 years and vote on continuing or abandoning the project. Abandoning costs the company $5 million. If expenses drop 15%, there is a 95% chance the vote is yes, to continue for another 3 years, If they drop 10%, the vote has a 75% chance to be yes. If expenses only drop 5%, there is a 40% change the shareholders vote to continue the project. Draw out the timeline, decision tree, and calculate the probabilities for all six possible scenarios.
Answers
The probabilities for all six possible scenarios based on the given information. The likelihood of each scenario and its respective outcome have been determined by multiplying the probabilities of the relevant events.
To analyze the given scenario and calculate the probabilities for all six possible scenarios, let's create a decision tree and timeline to visualize the information provided.
Timeline:
Year 1: Initial investment of $12 million in new technology.
Year 2: Results evaluated by shareholders.
Year 3: Final decision made by shareholders.
Decision Tree:
``` ┌─── Continue (95%)
│
15% ─────┼─── Continue (75%)
│
$12 million ─────┼─── Continue (40%)
investment │
│
10% ─────┼─── Abandon ($5 million cost)
│
│
5% ─────┼─── Abandon ($5 million cost)
│
└─── Abandon ($5 million cost)
```
Now, let's calculate the probabilities for each scenario:
1. New equipment reduces expenses by 15% (40% probability):
Probability = 40% * 95% = 0.4 * 0.95 = 0.38 (38%)
Outcome: The shareholders vote to continue for another 3 years.
2. New equipment reduces expenses by 10% (30% probability):
Probability = 30% * 75% = 0.3 * 0.75 = 0.225 (22.5%)
Outcome: The shareholders vote to continue for another 3 years.
3. New equipment reduces expenses by 5% (30% probability):
Probability = 30% * 40% = 0.3 * 0.4 = 0.12 (12%)
Outcome: The shareholders vote to continue for another 3 years.
4. New equipment does not reduce expenses (10% probability):
Probability = 10%
Outcome: The shareholders vote to abandon the project, incurring a $5 million cost.
5. New equipment reduces expenses by 15%, but shareholders vote to abandon (5% probability):
Probability = 15% * (1 - 95%) = 0.15 * 0.05 = 0.0075 (0.75%)
Outcome: The shareholders vote to abandon the project, incurring a $5 million cost.
6. New equipment reduces expenses by 10%, but shareholders vote to abandon (5% probability):
Probability = 10% * (1 - 75%) = 0.1 * 0.25 = 0.025 (2.5%)
Outcome: The shareholders vote to abandon the project, incurring a $5 million cost.
To summarize, we have calculated the probabilities for all six possible scenarios based on the given information. The likelihood of each scenario and its respective outcome have been determined by multiplying the probabilities of the relevant events.
Learn more about probabilities here
https://brainly.com/question/25839839
#SPJ11
The graphs below have the same shape. What is the equation of the blue
graph?
G(x) =
A. G(x) = (x + 3)2-5
B. G(x) = (x+3)2 +5
C. G(x) = (x - 3)2 + 5
D. G(x) = (x - 3)2-5

Answers
The equation of the translated function is:
g(x) = (x + 3)^2 - 2
How to get the equation for the blue graph?We can see that the blue graph is a translation of the red one, where the equation for the red graph is:
f(x) = x^2
Remember that the translations are:
Horizontal translation:
For a general function f(x), a horizontal translation of N units is written as:
g(x) = f(x + N).
If N is positive, the shift is to the left.
If N is negative, the shift is to the right.
Vertical translation:
For a general function f(x), a vertical translation of N units is written as:
g(x) = f(x) + N.
If N is positive, the shift is upwards.
If N is negative, the shift is downwards.
By analyzing the vertices, we can see that the vertex of the blue graph is at (-3, -2) so we have a translation of 3 units to the left and 2 units down, meaning that we have:
g(x) = f(x + 3) - 2 = (x + 3)^2 - 2
So the correct option is A.
If you want to learn more about translations, you can read:
brainly.com/question/11468584
#SPJ1
A certain surgery has a 70hance of success. the surgery is performed on three patients. find the probability of the surgery being successful on exactly two patients.
Answers
According to the given statement the probability of the surgery being successful on exactly two patients is 0.441 & 44.1%.
To find the probability of the surgery being successful on exactly two patients, we can use the binomial probability formula.
The formula for the probability of exactly k successes in n trials, when the probability of success in a single trial is p, is given by:
P(X = k) = nCk × \(p^{k}\) × \((1-p)^{n-k}\)
In this case,
n (number of trials) is 3 (since the surgery is performed on three patients),
p (probability of success) is 0.7 (given that the surgery has a 70% chance of success),
and
k (number of successes) is 2 (since we want exactly two successful surgeries).
Plugging the values into the formula, we have:
P(X = 2) = 3C2 × 0.7² × (1-0.7)³⁻²
Simplifying the calculation:
P(X = 2) = 3 × 0.7² × 0.3¹
Calculating the result:
P(X = 2) = 0.441
Therefore, the probability of the surgery being successful on exactly two patients is 0.441, or 44.1%.
To know more about probability visit :
https://brainly.com/question/31828911
#SPJ11
The probability of the surgery being successful on exactly two out of three patients is 0.441 or 44.1%.
The probability of the surgery being successful on exactly two patients can be found using the binomial probability formula.
The formula for the probability of exactly x successes in n trials, when the probability of success in each trial is p, is given by:
\(P(x) = (n_C_x) * p^x * (1-p)^{n-x}\)
In this case, the probability of success (the surgery being successful) is 0.7, as stated in the question.
Since the surgery is performed on three patients (n = 3), we want to find the probability of exactly two successful surgeries (x = 2).
Plugging these values into the formula, we get:
\(P(2) = (3_C_2) * 0.7^2 * (1-0.7)^{(3-2)}\)
Calculating the values, we find:
\(P(2) = 3 * 0.7^2 * 0.3^1\)
\(P(2) = 3 * 0.49 * 0.3\)
\(P(2) = 0.441\)
Therefore, the probability of the surgery being successful on exactly two patients is 0.441 or 44.1%.
In conclusion, the probability of the surgery being successful on exactly two out of three patients is 0.441 or 44.1%.
Learn more about probability from the given link:
https://brainly.com/question/32117953
#SPJ11
Which value could be substituted for the variable to make the equation TRUE? 24 = 4y
Answers
Explanation = you have to do the inverse operation. The equation is showing:
4 times y=24. To do the inverse you do 24 divided by 4, giving the answer 6. Hope that helps :)
suppose that a certain population obeys the logistic equation dy/dt=ry[1−(y/K)].(a) If y0=K/3,find the timeτat which the initial population has doubled. Find the value of τ corresponding tor=0.025 per year.(b) If y0/K=α, find the time T at which y(T)/K=β, where 0<α,β<1. Observe that T→[infinity] as α→0 or as β→1. Find the value of T for r=0.025 per year,α=0.1, andβ=0.9.
Answers
The initial population will double in about 27.73 years.
The population will reach β*K after about 25.44 years.
What is logistic regression?
The logistic model in statistics is a statistical model that depicts the likelihood that an event will occur by making the event's log-odds a linear combination of one or more independent variables. In regression analysis, logistic regression is used to estimate a logistic model's parameters.
The logistic equation is given by:
dy/dt = ry(1 - y/K)
(a) If y0=K/3, we want to find the time t at which the initial population has doubled.
Let Y denote the population size, so we want to find the value of t such that Y(t) = 2*K.
To solve for t, we need to first solve the differential equation using separation of variables:
dy/[y(1 - y/K)] = r*dt
Integrating both sides, we get:
ln|y/K - 1| - ln|y| = r*t + C
where C is a constant of integration. Solving for y, we get:
y(t) =\(K / [1 + Ae^{(-rt)}]\)
where A is a constant determined by the initial condition y(0) = K/3. Substituting this initial condition, we get:
K/3 = K / [1 + A]
Solving for A, we get:
A = 2
Substituting this value of A, we get:
y(t) = \(K / [1 + 2e^'(-rt)}]\)
Now, we need to find the time t such that Y(τ) = 2*K. Substituting Y(t) = y(t)*K, we get:
Y(t) =\(K^2 / [1 + 2e^{(-rt)}]\)
So we need to solve the equation:
2K = \(K^2 / [1 + 2e^{(-rt)}]\)
Solving for t, we get:
t = (1/r) * ln(2)
Substituting r = 0.025 per year, we get:
t = (1/0.025) * ln(2) ≈ 27.73 years
Therefore, the initial population will double in about 27.73 years.
(b) If y0/K=α, we want to find the time T at which y(T)/K=β. Letting Y denote the population size, we want to find the value of T such that Y(T) = β*K.
Following a similar procedure as in part (a), we can solve the logistic equation and obtain:
y(t) = \(K * [α/(α + (1 - α)*e^{(-rt)})]\)
Substituting Y(t) = y(t)*K, we get:
Y(t) = K *\([α/(α + (1 - α)e^{(-rt)})] = \beta K\)
Solving for t, we get:
t = (1/r) * ln[(β/α) - 1] - (1/r) * ln[(1 - α)/α]
Substituting r = 0.025 per year, α = 0.1, and β = 0.9, we get:
t = (1/0.025) * ln[8] - (1/0.025) * ln[9] ≈ 25.44 years
Therefore, the population will reach β*K after about 25.44 years.
The initial population will double in about 27.73 years.
The population will reach β*K after about 25.44 years.
We are given a sample of Logistic regression. Please refer the following link
https://brainly.com/question/28391630
#SPJ1
Answer this math question for 10 points

Answers
Measure of angle:
∠A = 36.86°
∠B = 90°
∠C = 53.13 °
Measure of side ,
AB = 28
BC = 21
CA = 35
Given triangle ABC.
Right angled at B.
Now, using trigonometric ratios to find angle A , B , C .
Right angled at B : ∠B = 90°
Angle A,
SinA = 21/35
∠A = 36.86
Angle C,
SinC = 28/35
∠C = 53.13
Now measures of side.
To find the length of side use sine rule .
Sine rule:
a/sinA = b/sinB = c /sinC
a = opposite side of angle A .
b = opposite site of angle B .
c = opposite side of angle C.
AB = 28
BC = 21
CA = 35
Hence the sides and angles of the triangles are measured .
Know more about sine rule,
https://brainly.com/question/30339239
#SPJ1
Mary has 36 flower pots. She plants daisies in 2/3 of her flower pots. In how many flower pots does Mary plant daisies?
Answers
Answer:
24
Step-by-step explanation:
36 Divided by three times 2 = 24 since fractions are division problems
Answer:
24 pots have daisies in them.
Step-by-step explanation:
36÷3= 12 and 12×2=24
How do i do this? Explain the answer and how it is done.

Answers
Answer:
4/9ths of a cup of sugar is needed for one cup of flour.
Step-by-step explanation:
To answer this you need to scale the number of cups of flour up from 3/4 to 1 that ratio would be 4/3 (multiplying a fraction by its own reciprocal will give you 1).
We can then simply take that ratio, and scale the sugar by the same amount:
1/3 * 4/3 = 4/9
Cara earn a bae pay of $1,800 per month at a car dealerhip plu a commiion of 6% of her ale. What are Cara' total earning in a month in which he ale $40,000 worth of merchandie?
Answers
Using the concept of percentages the total earning of Cara can be found to be $4200.
What are percentages?Percentage is a number expressed as a fraction of 100. The % sign means to divide the number by 100.
How to solve percentages?Cara's earning = commission + basic salary
basic salary = $1800 (this is constant)
commission = 6% of $40000
= (6/100)*40000
= $2400
Cara's earning = 1800 + 2400
Hence, her earning is $4200
To learn more about percentages visit the link below:
brainly.com/question/29306119
#SBJ4
researchers analyzed data from more than 5000 adults and found that the more diet sodas a person drank, the greater the person's weight gain. does this mean that drinking diet soda causes weight gain? choose a more plausible explanation for this association. actually, due to the very large sample size, this is good evidence to conclude that diet soda causes weight gain. the association in the sample must be due to random chance, since in the general population, diet products are associated with weight loss, not weight gain. people who gain more weight may be more likely to go on a diet and so choose to drink diet soda. younger adults are more likely to drink soda and are also more likely to be putting on large amounts of muscle mass through natural growth and/or exercise.
Answers
The more plausible explanation for the association between diet soda consumption and weight gain is that people who drink diet soda may have other factors or behaviors that contribute to weight gain.
For example, individuals who drink more diet soda may have a higher intake of calorie-dense foods or may engage in less physical activity.
Additionally, people who are already overweight or at risk of gaining weight.
May be more likely to choose diet soda as a way to manage their weight.
While the large sample size may provide strong statistical evidence of the association between diet soda consumption and weight gain.
It does not necessarily prove a causal relationship.
Further research is needed to establish a cause-and-effect relationship between diet soda consumption and weight gain.
Such as randomized controlled trials or longitudinal studies.
Learn more about diet soda here
brainly.com/question/30698193
#SPJ4
nd the area of a circle with a circumference of
12.56
Answers
Answer:
12.56 sq units
Step-by-step explanation:
C= 2 times pi times radius
12.56=2(3.14)R
12.56=6.28
r=2
area=A = πr²
A = 3.14(2²) = 3.14(4) = 12.56
the sum of n and 7 *
Answers
Answer:
n+7
Step-by-step explanation:
Sum means add, so it's just n added to 7:
n+7 :)
Answer:
n+7 is the answer or u can put it in parenthesis (n+7)
Step-by-step explanation:
What is the formula for a ratio? Is the x-axis horizontal or vertical? Which points are on the y-axis (0, 6)?
Answers
Answer:
formula for ratio: you can write a ratio with :, or, /
x axis is horizontal
0= x axis 6= y axis
Step-by-step explanation:
Find the Product: (x+9i)^2
Answers
Answer:
x² + 18xi - 81
Step-by-step explanation:
FOIL - First, Outside, Inside, Last
Step 1: Write out expression
(x + 9i)²
Step 2: Expand
(x + 9i)(x + 9i)
Step 3: FOIL
F - x²
O - 9xi
I - 9xi
L - 81i²
Step 4: Combine
x² + 18xi + 81i²
Step 5: Simplify
i² = -1
x² + 18xi + 81(-1)
x² + 18xi - 81
The probability P(Z>1.28) is closest to: (a) −0.10
(b) 0.10
(c) 0.20
(d) 0.90
Answers
Answer:
Step-by-step explanation:
The probability P(Z>1.28) represents the area under the standard normal distribution curve to the right of the z-score 1.28.
Using a standard normal distribution table or a calculator, we find that the area to the right of 1.28 is approximately 0.1003.
Therefore, the answer is closest to option (b) 0.10. there is a 10% chance of obtaining a value above 1.28 in a standard normal distribution.
know more about normal distribution: brainly.com/question/15103234
#SPJ11
the null hypothesis for the single factor anova states that all means are equal.
T/F
Answers
The null hypothesis for the single factor ANOVA states that all means are equally true.
The null hypothesis for a single-factor ANOVA (analysis of variance) states that all means are equal.
The alternative hypothesis, on the other hand, suggests that at least one of the means is different from the others.
The purpose of the ANOVA test is to determine whether there is sufficient evidence to reject the null hypothesis and conclude that there are significant differences between the means. A statistical formula used to compare variances across the means (or average) of different groups.
Hence, the statement is true .
To know more about null hypothesis click here :
https://brainly.com/question/30821298
#SPJ4
Need help it’s URGENT!

Answers
Answer:
either b or c
Step-by-step explanation:
i think
Translate the following argument into symbolic form, and use Truth Tables to determine whether the argument is valid or invalid.
If the boss snaps at you and you make a mistake, then he’s irritable. He didn’t snap at you. So he’s not irritable.
Answers
The last column evaluates to "T" in all rows. Therefore, the argument is valid since the conclusion always follows from the premises.
Let's assign symbols to represent the statements in the argument:
P: The boss snaps at you.
Q: You make a mistake.
R: The boss is irritable.
The argument can be symbolically represented as follows:
[(P ∧ Q) → R] ∧ ¬P → ¬R
To determine the validity of the argument, we can construct a truth table:
P | Q | R | (P ∧ Q) → R | ¬P | ¬R | [(P ∧ Q) → R] ∧ ¬P → ¬R
---------------------------------------------------------
T | T | T | T | F | F | T |
T | T | F | F | F | T | T |
T | F | T | T | F | F | T |
T | F | F | F | F | T | T |
F | T | T | T | T | F | F |
F | T | F | T | T | T | T |
F | F | T | T | T | F | F |
F | F | F | T | T | T | T |
The last column represents the evaluation of the entire argument. If it is always true (T), the argument is valid; otherwise, it is invalid.
Looking at the truth table, we can see that the last column evaluates to "T" in all rows. Therefore, the argument is valid since the conclusion always follows from the premises.
Learn more about argument here:
https://brainly.com/question/16052800
#SPJ11
c. A share of stock XYZ went from $40 a to $42 & What was the dollar gain for the
stock XYZ?
Answers
Answer:
$2 was the dollar gain for the stock XYZ
Please helppppppp ❤️
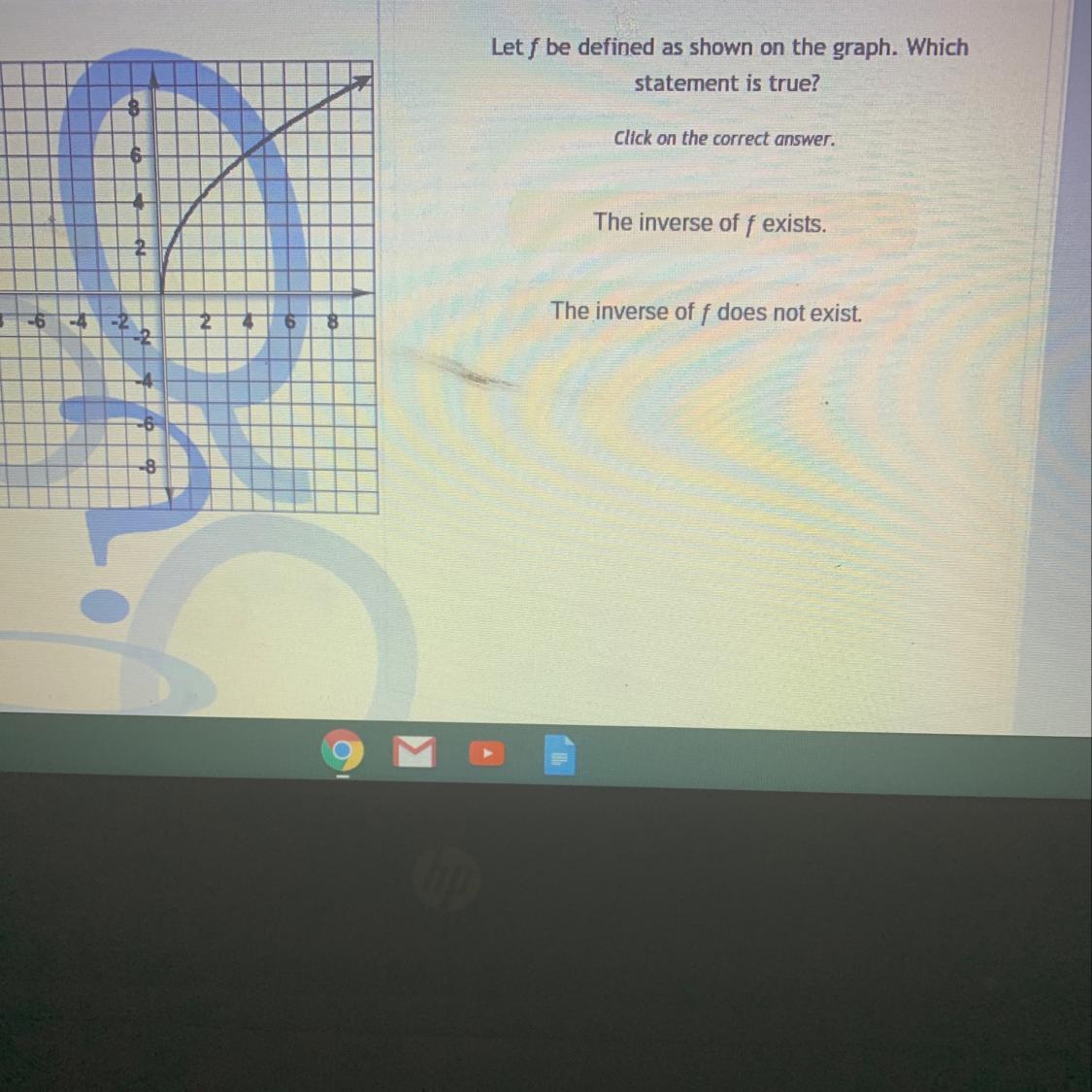
Answers
Answer:
Inverse of f exists.
Step-by-step explanation:
From the graph attached,
If we do the horizontal line test for the function graphed,
We find the function as one to one function.
In other words for every input value (x-value) there is a different output value.
Since, for one-to-one functions, inverse of the functions exist.
Therefore, the answer will be,
The inverse of 'f' exists.
Use the volume formula to find the volume of the prism.
|
NI
2
N
O A. 2, cubic units
B. 5 cubic units
C. 9 cubic units
D. 4 cubic units

Answers
Answer:
\(D.\ 4\frac{1}{2}\)
Step-by-step explanation:
Remember : 1 1/2 = (2+1)/2 = 3/2
Formula :
\(\large \text Volume\ V \ of \ a \ rectangular \ prism \ =base\ \times\ height\)
Then
\(V=\left( 2\times 1\frac{1}{2} \right) \times 1\frac{1}{2}\)
\(=\left( 2\times \frac{3}{2} \right) \times \frac{3}{2}\)
\(=3 \times \frac{3}{2}\)
\(=\frac{9}{2}\)
\(=\frac{8+1}{2} = \frac{8}{2} +\frac{1}{2} =4+\frac{1}{2} =4\frac{1}{2}\)
the number of cookies in a shipment of bags are normally distributed, with a mean of 64 and a standard deviation of 4. what percent of bags of cookies will contain between 64 and 68 cookies?
Answers
The percentage of bags of cookies that will contain between 64 and 68 cookies is approximately 18.23%.The number of cookies in a shipment of bags is normally distributed, with a mean of 64 and a standard deviation of 4.
What percentage of bags of cookies will contain between 64 and 68 cookies
When X is normally distributed with mean µ and standard deviation σ, the z-score formula can be used to find the probability that X is between two values.
Z = (X - µ) / σ
First, we convert both 64 and 68 to z-scores:
Z for 64 cookies = (64 - 64) / 4 = 0Z for 68 cookies = (68 - 64) / 4 = 1
Next, we find the probability that X is between these two z-scores using a standard normal distribution table or calculator:
Prob (0 < Z < 1) = 0.3413 - 0.5(0) - 0.159
= 0.1823
So, the percentage of bags of cookies that will contain between 64 and 68 cookies is approximately 18.23%.
To know more about standard deviation visit:
https://brainly.com/question/29115611
#SPJ11