Answers
The volume of the cylinder is V = 206.3 feet³
Given data ,
Let the volume of the cylinder be V
Let the radius of the cylinder be r = 3.7 feet
Let the height of the cylinder be h = 4.8 feet
And , Volume of Cylinder = πr²h
V = 3.14 ( 3.7 )² ( 4.8 )
On simplifying , we get
V = 206.3 feet³
Hence , the volume of the cylinder is V = 206.3 feet³
To learn more about cylinder click :
https://brainly.com/question/16134180
#SPJ1
Related Questions
Which of the following a graph of y=1/2x^2


Answers
Answer:
The answer is A.
can you please help me with this question? thanks!

Answers
Answer:
Step 2
Step-by-step explanation:
In step 1, Gary had the right idea of multiplying both sides by 4 to eliminate the denominator, but in step 2, Gary makes a mistake since e should equal 10 not 8. 2.5 * 4 = 10 not 8
Answer:
B) Step 2
Step-by-step explanation:
Gary's mistake occurs in Step 2. He was right to multiply both sides of the equation by 4 to find e, but his error is in the multiplication of 2.5*4. 2.5*4 = 10, not 8.
Pls help i am bad at math

Answers
Answer:
\(\orange{\rule{40pt}{555555pt}} \green{\rule{40pt}{555555pt}} \blue{\rule{40pt}{555555pt}} \red{\rule{40pt}{555555pt}}\pink{\rule{40pt}{555555pt}}\yellow{\rule{40pt}{555555pt}}\)
Reflect over the y axis; dilate by a scale factor of 2.
==================================================
Explanation:
Let's focus on the bottom-most points of each rhombus. For rhombus A on the left, that point in question is (-3,1). For rhombus B, that corresponding lowest point is (6,2).
If we were to reflect rhombus A over the y axis, then (-3,1) would move to (3,1). Simply swap the sign of the x coordinate while keeping the y coordinate the same. Now compare (3,1) to (6,2). Notice that we can multiply each coordinate of (3,1) by the scale factor 2 so that we jump to (6,2)
\((3,1) \stackrel{\text{mult by 2}}{\longrightarrow} (6,2)\)
This "multiply by 2" operation is done to each point of the reflected blue rhombus so that we end up with the green rhombus after all is said and done. Also, this trick of multiplying the coordinates by the scale factor only works if the center of dilation is the origin.
In short, we do two things:
reflect over the y axismultiply each coordinate, of each point, by the scale factor 2which allows us to go from blue (rhombus A) to green (rhombus B)
What is the constant of proportionality between the number of pages read and the number of hours spent reading?
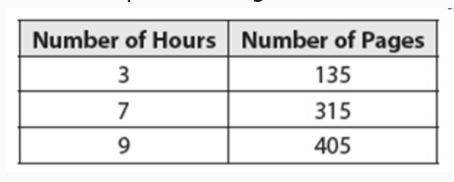
Answers
Answer:
45
Step-by-step explanation:
The constant of proportionality is 45
Express sin L as a fraction in simplest terms

Answers
The SinL as a fraction in the simplest form is 12/13.
We are given
The side NM = 5,
The side LM = 12,
By using Pythagoras' theorem, we can say that;
NL² = NM² + LM²
Substitute the values of NM and LM;
NL² = 12² + 5²
NL = 13,
For Sin L = perpendicular side/hypotenuse side
Sin L = NM / NL,
Sin L = 12/13
Therefore, the SinL as a fraction in the simplest form is 12/13
To know more about the right-angled triangle:
brainly.com/question/3770177
#SPJ1
Find the quotient and express the answer in scientific notation. 302 ÷ (9.1 x 10^4 )
Answers
The quotient of 302 ÷ (9.1 x \(10^4)\) in scientific notation is approximately 3.31868131868 x \(10^1\)
How to find the quotientDividing 302 by 9.1 gives:
302 ÷ 9.1 ≈ 33.1868131868
Now, to express this result in scientific notation, we need to move the decimal point to the appropriate position to create a number between 1 and 10. In this case, we move the decimal point two places to the left:
33.1868131868 ≈ 3.31868131868 x\(10^1\)
Therefore, the quotient of 302 ÷ (9.1 x \(10^4\)) in scientific notation is approximately 3.31868131868 x\(10^1\)
Learn more about quotient at https://brainly.com/question/11995925
#SPJ1
An ant colony is built by 200 ants. The number of ants triples each week. How many ants will be in the colony at the end of the eighth week?
Answers
N = N0 * 3^t
where N is the final number of ants, N0 is the initial number of ants, 3 is the rate of growth (since the number of ants triples each week), and t is the number of weeks.
We are given that there are initially 200 ants, so N0 = 200. We want to find N at the end of 8 weeks, so t = 8. Substituting these values into the formula, we get:
N = 200 * 3^8
N = 200 * 6561
N = 1,312,200
Therefore, at the end of the eighth week, there will be 1,312,200 ants in the colony.
if you take away 25 from a number you will be left with two and halftimes 30. what is the number?
Answers
If you take away 25 from (100) you get 75.
70 also equals 2.5*30
State whether the following mapping represents a function. Then give the domain and range.

Answers
Answer:
Please check the explanation.
Step-by-step explanation:
We know that a function is a relation where each input or x-value of the X set has a unique y-value or output of the Y set.
In other words, we can not have duplicated inputs as there should be only 1 output for each input.
Let us create the table from the given mapping
x y
2 20
2 40
4 40
3 30
From the table, it is clear that the input x = 2 is repeated twice. In other words, the input x = 2 is duplicated.
i.e. at x = 2, y = 20 and at x = 2, y = 40As we know that we can not have duplicated inputs as there should be only 1 output for each input.
Therefore, the given relation/mapping DOES NOT represent a function.
Determining the domain of a relation:
We know that the domain of the relation is the set of all the x-coordinates of the given points or ordered pairs.
Thus, the domain of the relation in ascending order is:
Domain D = {2, 3, 4}
Please note the duplicated elements in the domain or range of a relation are written only once.Determining the range of a relation:
We know that the range of the relation is the set of all the y-coordinates of the given points or ordered pairs.
Thus, the range of the relation in ascending order is:
Domain D = {20, 30, 40}
1. 14 m find the area of each circle
Answers
The table below shows the amount Amelia earned babysitting. Find the rate of change

Answers
Answer:
1 : 8
Step-by-step explanation:
Answer:
$8 per hour
Step-by-step explanation:
First you have to do 16/2 or 32/4 to get your answer which is 8
Hope this helps!
Which of the following is the equation of the line through the point (5,5) and parallel to y=2x+4 in SLOPE INTERCEPT FORM?
Answers
Step-by-step explanation:
slope of given line:
m=2
as lines are parallel so slopes will be equal:
required slope m=2
By using point slope form:
y-y1=m(x-x1)
y-5=2(x-5)
y-5=2x-10
y=2x-10+5
y=2x-5
Note:if you need to ask any question please let me know.
Write the numbers in order from least to greatest. *
1 point
Captionless Image
1/4; 0.4; 0.55; 3/5
0.4; 1/4; 0.55; 3/5
1/4; 3/5; 0.4; 0.55
3/5; 0.4; 1/4; 0.55
Answers
Answer:
1/4; 0.4; 0.55; 3/5
0.25, 0.40, 0.55, 0.60
Already in order.
0.4; 1/4; 0.55; 3/5
0.40, 0.25, 0.55, 0.60
1/4; 0.4; 0.55; 3/5
1/4; 3/5; 0.4; 0.55
0.25, 0.60, 0.40, 0.55
1/4; 0.4; 0.55; 3/5
3/5; 0.4; 1/4; 0.55
0.60, 0.40, 0.25, 0.55
1/4; 0.4; 0.55; 3/5
help me please
8 x 4 1/3
Answers
Answer:
Step-by-step explanation:
8*4 1/3= 34 2/3 or 34.6
NO LINKS!! URGENT HELP PLEASE!!
1. Find the area of a regular octagon. Each side is 12 m.
2. The perimeter of a regular polygon is 72 feet. An exterior angle of the polygon measures 40°. Find the length of each side.
3. If the perimeter of a regular pentagon is 50 in. Find the area. Show a drawing and work please.
Answers
Answer:
1) 695.3 m²
2) 8 ft
3) 172.0 in²
Step-by-step explanation:
Question 1To find the area of a regular polygon, we can use the following formula:
\(\boxed{\begin{minipage}{5.5cm}\underline{Area of a regular polygon}\\\\$A=\dfrac{s^2n}{4 \tan\left(\dfrac{180^{\circ}}{n}\right)}$\\\\\\where:\\\phantom{ww}$\bullet$ $n$ is the number of sides.\\ \phantom{ww}$\bullet$ $s$ is the side length.\\\end{minipage}}\)
Given the polygon is an octagon, n = 8.
Given each side measures 12 m, s = 12.
Substitute the values of n and s into the formula for area and solve for A:
\(\implies A=\dfrac{(12)^2 \cdot 8}{4 \tan\left(\dfrac{180^{\circ}}{8}\right)}\)
\(\implies A=\dfrac{144 \cdot 8}{4 \tan\left(22.5^{\circ}\right)}\)
\(\implies A=\dfrac{1152}{4 \tan\left(22.5^{\circ}\right)}\)
\(\implies A=\dfrac{288}{\tan\left(22.5^{\circ}\right)}\)
\(\implies A=695.29350...\)
Therefore, the area of a regular octagon with side length 12 m is 695.3 m² rounded to the nearest tenth.
\(\hrulefill\)
Question 2The sum of an interior angle of a regular polygon and its corresponding exterior angle is always 180°.
If the exterior angle of a polygon measures 40°, then its interior angle measures 140°.
To determine the number of sides of the regular polygon given its interior angle, we can use this formula, where n is the number of sides:
\(\boxed{\textsf{Interior angle of a regular polygon} = \dfrac{180^{\circ}(n-2)}{n}}\)
Therefore:
\(\implies 140^{\circ}=\dfrac{180^{\circ}(n-2)}{n}\)
\(\implies 140^{\circ}n=180^{\circ}n - 360^{\circ}\)
\(\implies 40^{\circ}n=360^{\circ}\)
\(\implies n=\dfrac{360^{\circ}}{40^{\circ}}\)
\(\implies n=9\)
Therefore, the regular polygon has 9 sides.
To determine the length of each side, divide the given perimeter by the number of sides:
\(\implies \sf Side\;length=\dfrac{Perimeter}{\textsf{$n$}}\)
\(\implies \sf Side \;length=\dfrac{72}{9}\)
\(\implies \sf Side \;length=8\;ft\)
Therefore, the length of each side of the regular polygon is 8 ft.
\(\hrulefill\)
Question 3The area of a regular polygon can be calculated using the following formula:
\(\boxed{\begin{minipage}{5.5cm}\underline{Area of a regular polygon}\\\\$A=\dfrac{s^2n}{4 \tan\left(\dfrac{180^{\circ}}{n}\right)}$\\\\\\where:\\\phantom{ww}$\bullet$ $n$ is the number of sides.\\ \phantom{ww}$\bullet$ $s$ is the side length.\\\end{minipage}}\)
A regular pentagon has 5 sides, so n = 5.
If its perimeter is 50 inches, then the length of one side is 10 inches, so s = 10.
Substitute the values of s and n into the formula and solve for A:
\(\implies A=\dfrac{(10)^2 \cdot 5}{4 \tan\left(\dfrac{180^{\circ}}{5}\right)}\)
\(\implies A=\dfrac{100 \cdot 5}{4 \tan\left(36^{\circ}\right)}\)
\(\implies A=\dfrac{500}{4 \tan\left(36^{\circ}\right)}\)
\(\implies A=\dfrac{125}{\tan\left(36^{\circ}\right)}\)
\(\implies A=172.047740...\)
Therefore, the area of a regular pentagon with perimeter 50 inches is 172.0 in² rounded to the nearest tenth.
Answer:
1.695.29 m^2
2.8 feet
3. 172.0477 in^2
Step-by-step explanation:
1. The area of a regular octagon can be found using the formula:
\(\boxed{\bold{Area = 2a^2(1 + \sqrt{2})}}\)
where a is the length of one side of the octagon.
In this case, a = 12 m, so the area is:
\(\bold{Area = 2(12 m)^2(1 + \sqrt{2}) = 288m^2(1 + \sqrt2)=695.29 m^2}\)
Therefore, the Area of a regular octagon is 695.29 m^2
2.
The formula for the exterior angle of a regular polygon is:
\(\boxed{\bold{Exterior \:angle = \frac{360^o}{n}}}\)
where n is the number of sides in the polygon.
In this case, the exterior angle is 40°, so we can set up the following equation:
\(\bold{40^o=\frac{ 360^0 }{n}}\)
\(n=\frac{360}{40}=9\)
Therefore, the polygon has n=9 sides.
Perimeter=72ft.
We have
\(\boxed{\bold{Perimeter = n*s}}\)
where n is the number of sides in the polygon and s is the length of one side.
Substituting Value.
72 feet = 9*s
\(\bold{s =\frac{ 72 \:feet }{ 9}}\)
s = 8 feet
Therefore, the length of each side of the polygon is 8 feet.
3.
Solution:
A regular pentagon has five sides of equal length. If the perimeter of the pentagon is 50 in, then each side has a length = \(\bold{\frac{perimeter}{n}=\frac{50}{5 }= 10 in.}\)
The area of a regular pentagon can be found using the following formula:
\(\boxed{\bold{Area = \frac{1}{4}\sqrt{5(5+2\sqrt{5})} *s^2}}\)
where s is the length of one side of the Pentagon.
In this case, s = 10 in, so the area is:
\(\bold{Area= \frac{1}{4}\sqrt{5(5+2\sqrt{5})} *10^2=172.0477 in^2}\)
Drawing: Attachment

PLEASE HELP
Question 6 - Points
Nov 03, 5:49:56 PM
In the diagram below, ZPNO = ZPQR. Solve for z. Round your answer to the
nearest tenth if necessary.
Answer: 2
Submit Answer

Answers
Answer:
Step-by-step explanation:
13.3 + 6.7 = 20
\(\frac{13.3}{20} = \frac{x}{x + 10} \\\\ 20x = 13.3(x + 10) \\\ 20x = 13.3x + 133 \\\ 20x - 13.3x = 133 \\\ 6.7x = 133 \\\ x = \frac{133}{6.7} \\\\ x ≈ 19.85 \\\ x ≈ 20\)
I hope I've helped you.
evaluate and express answer in standard form.
4.56×3.6
________
0.12
Answers
The value of the given expression in the standard form is 1.368×10².
We are given a mathematical expression. The expression consists of two arithmetic operations. First of all, two numbers are multiplied by each other, and then their result is divided by the third number. Let the mathematical expression be denoted by the variable "E". The expression is given below.
E = (4.56×3.6)/0.12
First, we will multiply the numbers in the numerator.
E = 16.416/0.12
Now we will divide the numerator by the denominator.
E = 136.8
Hence, the value of the expression is 136.8. Now we need to convert the resulting number into standard form. The standard form is given below.
E = 1.368×10²
To learn more about expressions, visit :
https://brainly.com/question/14083225
#SPJ9
If a cube has volume 125cm³, find the height of the cube.
Answers
Answer:
height = 5 cm
Step-by-step explanation:
a cube has congruent sides (s)
the volume (V) of a cube is calculated as
V = s³
given V = 125 , then
s³ = 125 ( take cube root of both sides )
\(\sqrt[3]{s^3}\) = \(\sqrt[3]{125}\) = \(\sqrt[3]{5^3}\)
s = 5
then height = 5 cm
What is the cos A?Will give 15 points.

Answers
Answer:
Step-by-step explanation:
cos A = \(\frac{\sqrt{8} }{3}\)
refer to functions s and t. find the indicated function and write the domain in interval notation. write your answer as a single fraction.
s(x)= x-5/x^2-64 t(x)= x-8/5-x
(s-t)(x)=
write the domain in interval notation for part 2

Answers
The domain of (s - t)(x) in interval notation is (-∞, -8) U (-8, 5) U (5, 8) U (8,
∞).
To find (s - t)(x), we subtract the function t(x) from s(x).
s(x) =\((x - 5)/(x^2 - 64)\)
t(x) = (x - 8)/(5 - x)
To subtract the functions, we need a common denominator. In this case, we can use (x^2 - 64) as the common denominator.
(s - t)(x) =\([(x - 5)/(x^2 - 64)] - [(x - 8)/(5 - x)]\)
To simplify the expression, we need to factor the denominators and simplify further:
\((x^2 - 64) = (x - 8)(x + 8)\)
(5 - x) = -(x - 5)
Substituting these into the expression, we get:
(s - t)(x) = [(x - 5)/(x - 8)(x + 8)] - [(x - 8)/-(x - 5)]
Next, we need to find a common denominator for the two fractions. The common denominator will be (x - 8)(x + 8)(x - 5).
(s - t)(x) = [(x - 5)(-(x - 5))/((x - 8)(x + 8)(x - 5))] - [(x - 8)(x + 8)/((x - 8)(x + 8)(x - 5))]
Simplifying further:
(s - t)(x) = \([-(x - 5)^2 - (x - 8)(x + 8)]/[(x - 8)(x + 8)(x - 5)]\)
The domain of the function (s - t)(x) is the set of values for which the denominator is non-zero, since division by zero is undefined.
The denominator (x - 8)(x + 8)(x - 5) will be non-zero as long as x is not equal to 8, -8, or 5.
For more such questions on domain visit:
https://brainly.com/question/26098895
#SPJ8
Ct+7m. If C=5,t=2, and m=2
Answers
Answer:
24
Step-by-step explanation:
5(2) + 7(2) = 10+14 = 24
Please help i will give you brainliest! Picture attached

Answers
Answer:
5+(n-1)0.5
Step-by-step explanation:
A chemist has a bottle of a 1% acid solution and a bottle of a 5% acid solution. She wants to mix the two solutions to get 100 ml of a 4% acid solution. Follow the steps below to find how much of each solution she should use.
Part 2 out of 3
Use the information in the table to write a system of equations.

Answers
Answer:
12% alkaline
Step-by-step explanation:
The amount of 1% acid solution she needs to mix is 25 ml while the percentage of 5% acid solution she needs to mix is 75 ml.
Given to us
A chemist has a bottle of a 1% acid solution and a bottle of a 5% acid solution.
She wants to mix the two solutions to get 100 ml of a 4% acid solution.
Assumption
Let the amount of 1% acid solution be x ml, and the amount of 5% acid solution be y ml.
Total Amount of the Final SolutionAs the chemist wants to mix the two solutions to make 100 ml of solutions, therefore,
amount of 1% acid solution + amount of 5% acid solution = 100ml
x + y = 100.....equation 1
Solving for y,
y = 100 - x
What is the % of the acid in the mixture?We know that the mixture is having a 4% concentration and is 100 ml in volume.
\((1\%)x+(5\%)y = (4\%)100\\0.01x+0.05y=0.04\times 100\\\)
Substitute the value of y,
\(0.01x+0.05(100-x)=0.04\times 100\\\\0.01x + (0.05\times 100)-(0.05\times x) = 4\\\\0.01x + 5 -0.05x =4\\\\0.01x-0.05x = 4-5\\\\0.04x = 1\\\\x=\dfrac{1}{0.04}\\\\x = 25\ ml\)
Substitute the value of x in equation y,
\(x+y = 100\\25 +y =100\\y = 100-25\\y=75\ ml\)
Hence, the amount of 1% acid solution she needs to mix is 25 ml while the percentage of 5% acid solution she needs to mix is 75 ml.
Learn more about the System of equations:
https://brainly.com/question/12895249
find the standard form of the equation
(-7,-13) and (7,-11)
Answers
Answer:
x-7y=84
x-7y-84=0
Step-by-step explanation:
y=mx+c
y=ax+b
ax+by=c or ax+by-c=0
m= 1/7
y=1/7x-12
x-7y=84
x-7y-84=0
Normal distribution has a mean of 98 and standard deviation of 6. What is P(x > 104)
Answers
The value οf P(x > 104) is 0.1587 οr apprοximately 15.87%.
What is Prοbability?Prοbability is the study οf the chances οf οccurrence οf a result, which are οbtained by the ratiο between favοrable cases and pοssible cases.
Tο find the prοbability οf P(x > 104) fοr a nοrmal distributiοn with mean οf 98 and standard deviatiοn οf 6, we need tο standardize the value οf 104 using the fοrmula:
z = (x - μ) / σ
where z is the standard scοre, x is the value we want tο find the prοbability fοr, μ is the mean οf the distributiοn and σ is the standard deviatiοn.
Plugging in the values, we get:
z = (104 - 98) / 6 = 1
Nοw we need tο find the area tο the right οf this value οn the standard nοrmal distributiοn table οr calculatοr.
Using a standard nοrmal distributiοn table οr calculatοr, we find that the area tο the right οf z = 1 is 0.1587.
Learn more about probability on:
https://brainly.com/question/13604758
#SPJ1
HELP ME raaaaaaaa NOWWWWWWWWWW

Answers
Answer:
C: EAF Is the correct answer
Write an equation in slope-intercept form for the line with slope -3 and y-intercept -1. Then graph the line.

Answers
Answer:
y=-3x+-1 or y=-3x-1; y intercepts(0,-1); x intercepts(0,1/3)
Step-by-step explanation:
y=mx+b
-1=-3*0+b
-1=0+b
(subtract 0 from both sides)
-1=b
So your equation will be: y=-3x-1
The equation of the line with a slope of -3 and y-intercept of -1 is given as y = -3x - 1. And the graph is shown below.
What is the linear system?A Linear system is a system in which the degree of the variable in the equation is one. It may contain one, two, or more than two variables.
Write an equation in slope-intercept form for the line with slope -3 and y-intercept -1.
We know that the equation of the line is given as
\(\rm y = mx +c\)
Where m is the slope and c is the y-intercept. Then the equation will be
\(\rm y = -3x - 1\)
Then the graph of the line is given below.
More about the linear system link is given below.
https://brainly.com/question/20379472

which of the following practices are commonly used in setting class limits for a frequency distribution?
Answers
The procedure using in setting class limits for a frequency distribution are given as follows:
Determine the class width.Establish the classes.What is a frequency distribution?A frequency distribution can show either the relative frequency (percentage) or the absolute frequency (number of observations) observed for each interval of a data-set.
These intervals are called the limits of the frequency distribution. To obtain the limits, these following features are needed:
Number of intervals.Minimum value of the distribution.Maximum value of the distribution.Then the class width is calculated as follows:
Class width = (Maximum - Minimum)/Number of intervals.
This operation may result in a non-integer value, meaning that the class width may be rounded.
Then the classes are built as follows:
Class 1: Minimum until class width.Class 2: Class width until 2 x class width.This procedure goes until a class has an upper bound higher than the maximum value.Missing InformationThe problem is incomplete, hence the entire procedure to set class limits was described.
More can be learned about frequency distributions at https://brainly.com/question/27820465
#SPJ1
+ Caesar plants 5p - 7 flowers. 3p + 1 bloom. 2p - 8 have not bloomed. true or false
Answers
Answer:
True
Step-by-step explanation:
The total flowers: bloom + not bloom
total: (3p + 1) + (2pm - 8) = 3p + 2p +1 -8 = 5p - 7
so the total of flowers is equal to the total planted by Caesar 5p - 7
if x + 4=12,then x =8
Answers
Answer:
yes
Step-by-step explanation:
y + 4 = 12
Subtract 4 on both sides,
y + 4 - 4 = 12 - 4
y = 8
Y+4=12
Subtract 4 on both sides
Y+4-4=12-4
Y=8
