Find the slope-intercept equation of the line that passes through (5, 5) and has a slope of 1/3.
Answers
Answer:
y = 1/3x + 10/3
Step-by-step explanation:
→ Write gradient into format
y = 1/3x + c
→ Substitute in (5,5)
5 = 5/3 + c
→ Find c
c = 5 - 5/3 = 10/3
→ Finish off
y = 1/3x + 10/3
Related Questions
Adler and Erika solved the same equation using the calculations below. Adler’s Work Erika’s Work StartFraction 13 over 8 EndFraction = k one-half. StartFraction 13 over 8 EndFraction minus one-half = k one-half minus one-half. StartFraction 9 over 8 EndFraction = k. StartFraction 13 over 8 EndFraction = k one-half. StartFraction 13 over 8 EndFraction (negative one-half) = k one-half (negative one-half). StartFraction 9 over 8 EndFraction = k. Which statement is true about their work? Neither student solved for k correctly because K = 2 and StartFraction 1 over 8 EndFraction. Only Adler solved for k correctly because the inverse of addition is subtraction. Only Erika solved for k correctly because the opposite of One-half is Negative one-half. Both Adler and Erika solved for k correctly because either the addition property of equality or the subtraction property of equality can be used to solve for k.
Answers
The correct statement about their work is: Both Adler and Erika solved for k correctly because either the addition property of equality or the subtraction property of equality can be used to solve for k.
Adler started with the equation (13/8) = k(1/2) and subtracted 1/2 from both sides to get (13/8) - (1/2) = k(1/2) - (1/2). Then simplifying, Adler obtained (9/8) = k.
Erika started with the equation (13/8) = k(1/2) and multiplied both sides by -1/2 to get (13/8)(-1/2) = k(1/2)(-1/2). Simplifying, Erika obtained (9/8) = k.
Both Adler and Erika arrived at the correct value of k = 9/8, despite using slightly different approaches. The inverse of addition (subtracting 1/2) and the opposite of one-half (multiplying by -1/2) were correctly applied in their respective calculations.
To know more about subtraction:
https://brainly.com/question/29149893
#SPJ4
the standard deviation is a resistant measure of spread. t/f
Answers
True, the standard deviation is a resistant measure of spread.
The standard deviation is calculated as the square root of the variance of the data set. Variance is calculated as the average of the squared differences between each data point and the mean of the data set. The standard deviation is the square root of this average.
In mathematical terms, the standard deviation can be calculated using the following formula:
σ = √(1/n ∑(x - μ)²)
Where σ is the standard deviation, n is the number of data points in the data set, x is the value of the i-th data point, and μ is the mean of the data set.
The standard deviation is an important measure because it gives an idea of how much the individual values in a data set differ from the mean.
In summary, the standard deviation is a resistant measure of spread because it gives a robust measure of the spread of the data in a set and is not easily influenced by outliers or extreme values.
To know more about standard deviation here.
https://brainly.com/question/16555520
#SPJ4
the life of light bulbs is distributed normally. the variance of the lifetime is 625 and the mean lifetime of a bulb is 520 hours. find the probability of a bulb lasting for at most 549 hours. round your answer to four decimal places.
Answers
Light bulbs is normally distributed with a variance of 625 and a mean lifetime of 520 hours, we need to calculate the cumulative probability up to 549 hours. The answer will be rounded to four decimal places.
Given a normally distributed lifetime with a mean of 520 hours and a variance of 625, we can determine the standard deviation (σ) by taking the square root of the variance, which gives us σ = √625 = 25.
To find the probability of a bulb lasting for at most 549 hours, we need to calculate the area under the normal distribution curve up to 549 hours. This can be done by evaluating the cumulative distribution function (CDF) of the normal distribution at the value 549, using the mean (520) and standard deviation (25).
The CDF will give us the probability that a bulb lasts up to a certain point. Rounding the result to four decimal places will provide the desired precision.
Visit here to learn more about probability:
brainly.com/question/13604758
#SPJ11
The problem involves using normal distribution to find the probability of a given outcome. Using the Z-score, we can determine that the probability of a light bulb lasting for at most 549 hours is approximately 0.8770 or 87.70%
Explanation:Given the mean (µ) of the lifetime of a bulb is 520 hours. Also, the variance (σ²) is given as 625. Thus, the standard deviation (σ) is the square root of the variance, which is 25.
To find the probability of a bulb lasting for at most 549 hours, we first calculate the Z score. The Z-score formula is given as follows: Z = (X - µ) / σ, where X is the number of hours, which is 549. So substitute the given values into the formula. Z = (549 - 520) / 25, the Z value is 1.16.
We then look up the Z-table to find the probability associated with this Z-score (1.16), which is approximately 0.8770. Therefore, the probability of a bulb lasting for at most 549 hours is approximately 0.8770 or 87.70%.
Learn more about Probability here:https://brainly.com/question/32117953
#SPJ12
operations on functions, grade 11 algebra
g(x)= x-2
h(x) = 3+1
Answers
As a result, g(x) and h(x) have the following composition: f(x) = 2 as to determine the composition of g(x) and h(x) .
what is functions ?With the input (the x-value) drawn on the x plane and the output (the y-value) written on the vertical axis, functions can be represented graphically. Understanding a function's behaviour, for example, if it is going up or down, and locating significant characteristics like peaks and minima, can be done by looking at its graph. Many branches of arithmetic, as well as physics, construction, computer science, sociology, and a wide range of other disciplines, use functions. They are a crucial tool for problem-solving across a broad spectrum and for simulating real-world occurrences.
given
We may utilise the distributive property of multiplication to multiply the two functions:
\(g(x) * h(x) = (x - 2) * 4\)
= 4x - 8
Hence, f(x) = 4x - 8 is the result of the two functions' product.
Composition: To determine the composition of g(x) and h(x), we must replace every instance of x with h(x) in g(x):
\(g(h(x)) = g(4) (4)\)
= 4 - 2
= 2
As a result, g(x) and h(x) have the following composition: f(x) = 2 as to determine the composition of g(x) and h(x) .
To know more about function visit:
https://brainly.com/question/28193995
#SPJ1
A new parabola, g(x), is translated from f(x) using the translation T(-3, 7).
5. What is the vertex point of g(x)?
Explain how you know.
Answers
Answer:
We can predict the vertex point of \(g(x)\) by vectorial sum, which is equal to \(V_{G}(x,y) = (h - 3, k + 7)\).
Step-by-step explanation:
The vertex of \(g(x)\) is equal to the vectorial sum of the vertex of \(f(x)\) and the translation vector:
\(V_{G}(x,y) = V_{F}(x,y) + T(x,y)\) (1)
Where \(T(x,y)\) is the translation vector.
If we know that \(V_{F} (x,y) = (h,k)\) and \(T(x,y) = (-3, 7)\), then the formula for the vertex of \(g(x)\) is:
\(V_{G}(x,y) = (h,k) +(-3, 7)\)
\(V_{G}(x,y) = (h - 3, k + 7)\)
rewrite the following expression. x 9/7
Answers
The given expressiοn x 9/7 may be rewritten as 9x/7.
What is an expressiοn?An expressiοn is a cοmbinatiοn οf symbοls, variables, and/οr numbers that represents a mathematical relatiοnship οr quantity, but is nοt an equatiοn and dοes nοt cοntain an equal sign.
An expressiοn in math is a sentence with a minimum οf twο numbers οr variables and at least οne math οperatiοn. This math οperatiοn can be additiοn, subtractiοn, multiplicatiοn, οr divisiοn.
Tο rewrite "x 9/7" as "9x/7", we need tο recοgnize that the expressiοn "x 9/7" means "x multiplied by 9/7". Sο we can write:
x 9/7 = x * 9/7
Then we can simplify the expressiοn by multiplying the numeratοr (9) by x:
x 9/7 = 9x/7
To know more about mathematical relationship visit:
brainly.com/question/30284058
#SPJ1
The function p has domain -14
(-6, -6) to (10, 2).
(a) Sketch y = p(x).
(2)
(b) Write down the range of p(x).
(1)
(c) Find the values of a, such that p(a) =-3.
(2)
Answers
Answer:
b_________________????
what are the coefficients?!?! i need help

Answers
Answer:
a = 3, b = -15, c = 7, d = 0
Step-by-step explanation:
When x = 0, y = 0 therefore d = 0
When x = 1, y = -5 therefore a + b + c = -5
When x = 2, y = -22 therefore 8a + 4b + 2c = -22
When x = 3, y = -33 therefore 27a + 9b + 3c = -33
Solving simultaneously:
a = 3, b = -15, c = 7
I NEED THIS ANSWERED W WORK ASAP ITS DUE IN 30 MINS

Answers
Answer:
B
Step-by-step explanation:
I dont know how to show my work...
Please help me. I need help thank you.

Answers
Answer:
C.
Step-by-step explanation:
the ratio is 1:2
so,
\(4*2=8\\7*2=14\\1*2=2\)
the only shape with those numbers is C
Hope this helps! Please let me know if you need more help, or if you think my answer is incorrect. Brainliest would be MUCH appreciated. Have a great day!
Stay Brainy!

Obtain numerical solution of the ordinary differential equation y' = 3t−10y²
with the initial condition: y(0)= −2 by Euler method using h=0.5 Perform 3 steps.
Solution of all problems MUST contain general formula and all intermediate results. Perform numerical computations using 4 digits after decimal point.
Answers
The Euler method with a step size of h = 0.5, the approximate numerical solution for the ODE is y(1.5) ≈ -1.1198 x 10^9.
To solve the ODE using the Euler method, we divide the interval into smaller steps and approximate the derivative with a difference quotient. Given that the step size is h = 0.5, we will perform three steps to obtain the numerical solution.
we calculate the initial condition: y(0) = -2.
1. we evaluate the derivative at t = 0 and y = -2:
y' = 3(0) - 10(-2)² = -40
Next, we update the values using the Euler method:
t₁ = 0 + 0.5 = 0.5
y₁ = -2 + (-40) * 0.5 = -22
2. y' = 3(0.5) - 10(-22)² = -14,860
Updating the values:
t₂ = 0.5 + 0.5 = 1
y₂ = -22 + (-14,860) * 0.5 = -7492
3. y' = 3(1) - 10(-7492)² ≈ -2.2395 x 10^9
Updating the values:
t₃ = 1 + 0.5 = 1.5
y₃ = -7492 + (-2.2395 x 10^9) * 0.5 = -1.1198 x 10^9
Therefore, after performing three steps of the Euler method with a step size of h = 0.5, the approximate numerical solution for the ODE is y(1.5) ≈ -1.1198 x 10^9.
Learn more about Euler method here:
https://brainly.com/question/30459924
#SPJ11
What is the value of b?

Answers
Answer:
Step-by-step explanation:
9b + 106 + 38 = 180
9b + 144 = 180
9b = 36
b = 4
Stephon makes the following statements: statement 1 lim x -3 f(x) exists and is equal to 1 statement 2 lim x 1 f(x) exists and is equal to 1

Answers
Neither statement 1, nor statement 2 are correct
The given Stephon's statements are;
Statement 1; \(\mathbf{\lim \limits _{x \to -3} f(x)} \ \mathbf{Exist} \ and \mathbf{\lim \limits _{x \to -3} f(x) = 1}\)
Statement 2; \(\mathbf{\lim \limits _{x \to 1} f(x)} \ \mathbf{Exist} \ and \mathbf{\lim \limits _{x \to 1} f(x) = 1}\)
The analysis of the graph and reason for the answer
From the graphed line on the left of the y-axis, we have an open circle at x = -3, and an arrow at the other end pointing towards negative infinity, (-∞) which indicates that the domain is -∞ ≤ x < -3, therefore, at x = -3, f(x) does not exist, therefore, we can write the following statement
The limits of the domain and range of the graph includes;
\(\mathbf{\lim \limits _{x \to -3} f(x)} = \mathbf{Does \ not \ exist}\)
f(x) = Defined for -∞ ≤ x < -3
Similarly, from the graphed line on the right of the y-axis, we have an open circle at x = 1 and an arrow at the other end of the line f(x) = 4 pointing towards positive (+∞) infinity, which indicates the domain and the graph of the function is 1 < x ≤ ∞ , therefore, f(x) does not exist at x = 1, and we can write
\(\mathbf{\lim \limits _{x \to 1} f(x)} = \mathbf{Does \ not \ exist}\)
From we above, we have that neither statement 1, nor statement 2 are correct
Learn more about open and closed circles on graph lines
https://brainly.com/question/8648835
r = x^2/y work out the value of r
Answers
Answer:
r=244745.8 (approximately)
Step-by-step explanation:
r=x²/y
ry-x²=0
r(59000)-(380000)²=0
r=380000²/59000
r=144400000/59
r=244745.8 (approximately)
What is an advantage of using a stem-and-leaf plot instead of a histogram? What is a disadvantage? O A. Stem-and-leaf plots show data clusters where histograms do not O B. Stem-and-leaf plots contain original data values where histograms do not. O C. Stem-and-leaf plots.easily organize data of all sizes where histograms do not O D. Stem-and-leaf plots graph qualitative data where histograms do not.
Answers
the stem and leaf plot gives you a more exact answer where a histogram gives you a more of an average. Now i'm not saying a histogram can't be exact but a stem and leaf plot is more reliable if you need an exact answer.
Step-by-step explanation:
Stem-and-leaf plots contain original data values where histograms do not. Therefore, option B is the correct answer.
What is stem and leaf?The "stem" values are listed down, and the "leaf" values go right (or left) from the stem values.
The "stem" is used to group the scores and each "leaf" shows the individual scores within each group.
The advantage of a stem leaf diagram is it gives a concise representation of data. The advantage of a frequency histogram is, that it is visually strong. Histograms are usually preferable to stem and leaf diagrams in large data sets. The disadvantage of a stem leaf diagram is not visual.
Therefore, option B is the correct answer.
Learn more about the stem-and-leaf plot here:
https://brainly.com/question/12857419.
#SPJ2
Use the distributive property to write an equivalent expression to 45 + 30x
Use the distributive property to write an equivalent expression to 45 + 30x
15(2x+3)
15(30+30x)
3(15+10x)
1/3( 135+90x)
1/2(45+30x)
Answers
Answer:
15(2x + 3)
Step-by-step explanation:
First find the greatest common factor of 45 and 30.
That's 15.
Now we factor 15 out of the expression :
45 + 30x = 15(3 + 2x)
Therefore, the answer is the first option, 15(2x + 3). Hope this helps! :)
Determine whether the ordered pair \( (2,7) \) is a solution of the equation \( 9 x-y-11=0 \). The ordered pair IS NOT a solution The ordered pair IS a solution
Answers
The ordered pair (2, 7) is a solution to the equation 9 x-y-11=0.
To determine whether the ordered pair (2, 7) is a solution of the equation 9x - y - 11 = 0.
we substitute the values of x and y from the ordered pair into the equation and check if it satisfies the equation.
Given ordered pair: (2, 7)
Substituting x = 2 and y = 7 into the equation:
9(2) - 7 - 11
= 18 - 7 - 11
= 18 - 18
= 0
Since the equation holds true when the values of x and y are substituted, the ordered pair (2, 7) is a solution of the equation 9x - y - 11 = 0.
To learn more on Equation:
https://brainly.com/question/10413253
#SPJ4
the graph shows a population of butterflies, t weeks since their migration began.
c. Write an equation for the
population, q, after t weeks.

Answers
Answer:
q = 250,000·(0.6^t)
Step-by-step explanation:
You want an equation that models the graph of an exponential function that has an initial value of 250,000 and a value of 150,000 after 1 week.
Exponential functionAn exponential function has the form ...
q = a·b^t
where 'a' is the initial value, and 'b' is the decay factor over a period of one time unit of t.
ApplicationThe graph with this problem shows the initial value (for t=0) to be a=250,000. The decay factor will be ...
b = 150,000/250,000 = 3/5 = 0.6
Then the exponential function can be written as ...
q = 250000·(0.6^t) . . . . . . where t is in weeks
Simplify the given expression’s
( )
And
( )

Answers
Answer:
1. p^10
2. p^24
Step-by-step explanation:
p^6 x p^4=p^(6+4)=p^10
(p^6)^4=p^(6x4)=p^24
Is it true that for every integer of n the value of n to the 2nd power is positive
Answers
Answer:
no i do think so that dose not make sensens
The price of a dress is reduced by 17% in a sale. The sale price is £45.65. What was the original price of the dress?
Answers
Answer:
\(\Huge \boxed{\£55}\)
________________________________________________________
A 17% reduction means that the dress cost 83% (100 - 17) of the original amount.
Unitary Method\(\large \fbox{\begin{minipage}{8.1 cm}83\% of the original price = \£45.65\\\\$\Rightarrow$1\% of the original price = $\frac{45.65}{83}$\\\\$\Rightarrow$1\% of the original price = 0.55\\\\$\Rightarrow$100\% of the original price = 0.55 \times 100\\\\$\Rightarrow$100\% \text{ of the original price = \£55}\end{minipage}}\)
Inverse operationTo work out 83% of the original price, you multiply by 0.83. We can do the inverse, which is dividing by 0.83.
×0.83
Original Price →→→→→→→→→→→→→ Sale Price
£? ←←←←←←←←←←←←← £45.65
÷0.83
\(\large \boxed{\begin{minipage}{7 cm}Original Price = $\frac{\text{Sale Price}}{0.83}$\\\\$\Rightarrow$Original Price = $\frac{45.65}{0.83}$\\\\$\Rightarrow$Original Price = \£55\end{minipage}}\)
Therefore, the original price of the dress is £55.
________________________________________________________
1. If 2000 flux lines enter through a given volume of space and
5000 lines diverge from it, calculate the total charge within the
volume. (Express your answer in nano Coulomb up to 2 decimals.)
Answers
Charge cannot be negative as it is a scalar quantity,
Given,
The number of flux lines entering a given volume of space = 2000
The number of flux lines diverging from the same volume of space = 5000
Formula to find the charge within the volume is:Q = Φ1 - Φ2 / 150
Where,
1 = the number of flux lines entering the volume2 = the number of flux lines leaving the volumeWe know that,Q = 1 - 2 / 150⇒ Q = 2000 - 5000 / 150⇒ Q = - 20 / 3
Charge cannot be negative as it is a scalar quantity,
Therefore the total charge within the volume is zero.
Hence, the correct option is B, 0.00.
learn more about Charge on :
https://brainly.com/question/14306160
#SPJ11
Given the following LP model, which is the correct standard form? Max(Z)=2X1+X2 Restrictions / Constrains 11×1+3×2≥33
8×1+5×2≤40
7×1+10×2≤70
7×1+10×2≤70 X1,X2≥0 Use this problem's information to answer questions 19 to 21. a. Max(Z)=3×1+2X2
11×1+3×2−51≤33
8×1+5×2+52≥40
7×1+10×2+53≥70
b. Max(Z)=3×1+2×2 11×1+3×2+51=33 8×1+5×2−52=40 7×1+10×2−53=70
c. Max(Z)=3×1+2×2 11×1+3×2−51=33 8×1+5×2+52=40 7×1+10×2+53=70
d. Max(Z)=3×1+2×2 11X1+3×2+$1=33 8×1+5×2+52=40 7×1+10×2+53=70
Answers
The correct standard form for the given LP model is option C: Max(Z) = 3x1 + 2x2, subject to the constraints 11x1 + 3x2 - 5 <= 33, 8x1 + 5x2 + 5 >= 40, and 7x1 + 10x2 + 5 >= 70, with x1, x2 >= 0. This option aligns with the original objective function and constraints of the LP model.
To convert the LP model into standard form, we need to rewrite the constraints with the variables on the left-hand side and constants on the right-hand side, with inequality signs (<= or >=) consistent throughout. Additionally, we introduce slack or surplus variables to transform any non-standard constraints.
In option C, the constraints are correctly transformed as 11x1 + 3x2 - 5 = 33, 8x1 + 5x2 + 5 >= 40, and 7x1 + 10x2 + 5 >= 70. These constraints are consistent with the original model. The objective function remains the same, Max(Z) = 3x1 + 2x2.
Option A has incorrect signs in the transformed constraints, and option B introduces surplus variables (+5) instead of slack variables (-5), resulting in an incorrect standard form. Option D includes a non-standard term with a dollar sign, which is inconsistent with linear programming conventions.
Therefore, option C is the correct standard form, adhering to the original LP model's objective function and constraints.
Learn more about LP model here : brainly.com/question/33051152
#SPJ11
find the volume of the region contained in the cylinder x 2 y 2 = 9, bounded above by the plane z = x and below by the xy-plane.
Answers
the volume of the region contained in the cylinder x² + y² = 9, bounded above by the plane z = x and below by the xy-plane is 0.
Given: The cylinder is x² + y² = 9, bounded above by the plane z = x and below by the xy-planeWe know that, the cylinder is x² + y² = 9Which is (x/a)² + (y/b)² = 1Where a = 3 and b = 3The plane is z = xThe region is bounded below by the xy-plane Thus, the volume of the region can be found by integrating z = x with limits of x² + y² ≤ 9.So, V = ∭ dx dy dz where the limits are given by the cylinder and the plane.V = ∫∫∫ (x) dV ... (1)Now, converting the integral into cylindrical coordinates we have,∫∫∫ (x) dV = ∫θ = 0 to 2π ∫r = 0 to 3 ∫z = 0 to r cos θ (r cos θ) rdzdrdθ ... (2)x = r cos θ, y = r sin θ, and z = z.We know that the limits of x² + y² ≤ 9 in cylindrical coordinates are 0 ≤ θ ≤ 2π, 0 ≤ r ≤ 3, 0 ≤ z ≤ r cos θ.Using (2) in (1), we haveV = ∫θ = 0 to 2π ∫r = 0 to 3 ∫z = 0 to r cos θ (r cos θ) rdzdrdθ= ∫θ = 0 to 2π ∫r = 0 to 3 [r² cos θ / 2] dr dθ= ∫θ = 0 to 2π [ 9 cos θ / 2 ] dθ= 9 [ sin θ ]θ = 0 to 2π= 0Thus, the volume of the region contained in the cylinder x² + y² = 9, bounded above by the plane z = x and below by the xy-plane is 0.
To know more about Volume of region Visit:
https://brainly.com/question/30785714
#SPJ11
the temperature is -3 at 6am but it rises to 12 at the afternoon what is the temperature In the afternoon
Answers
Answer:
1cm divided by 17 = 32 then subtract the 32 into 17
I tried 1/2, but it was wrong.

Answers
The slope of the quadrilateral is 2.
Define slope.A line's steepness and direction are measured by the line's slope. Without actually using a compass, determining the slope of lines in a coordinate plane can assist in forecasting whether the lines are parallel, perpendicular, or none at all. A line's slope is determined by how its y coordinate changes in relation to how its x coordinate changes. y and x are the net changes in the y and x coordinates, respectively. The increase divided by the run, or the ratio of the rise to the run, is known as the line's slope. In the coordinate plane, it describes the slope of the line.
Given
Coordinates
(1,0) (0,2)
y₂ = 2
y₁ = 0
x₂ = 0
x₁ = 1
Slope = m
m = y₂ - y₁/x₂ - x₁
m = 2- 0/1 -0
m = 2/1
m = 2
The slope of the quadrilateral is 2.
To learn more about slope, visit:
https://brainly.com/question/3605446
#SPJ1
Geometry
When you find m<8 given m<11=118, which theorem or postulate justifies your answer?
A. Corresponding Angles postulate
B. Alternate Exterior Angels Theorem
C. Alternate Interior Angles Theorem
D. Same-side Interior Angles Theorem

Answers
Answer:
Option (C)
Step-by-step explanation:
From the picture attached,
Two lines 'h' and 'k' are the parallel lines and a transversal 'n' is intersecting these parallel lines at two distinct points.
By the theorem of alternate interior angles, angle 8 and angle 11 will be equal in measure.
∠8 ≅ ∠11 [Alternate interior angles]
m(∠8) = m(∠11) = 118°
Therefore, Option (C) will be the answer.

The theorem or postulate that justifies your answer is Alternate Interior Angles Theorem. Option C is correct.
From the given diagram, we are given that m<11 = 118 degrees
To get the measure of angle m<8, we will use the following postulates;
The angle 11 and angle m<12 are supplementary, hence;
m<11 + m<12 = 180
118 + m<12 = 180
m<12 = 180 - 118
m<12 = 62 degrees
The measure of the angle m<11 is also equal to m<8 according to the alternate interior angle theorem. Hence;
m<8 = m<11 = 118 degrees (Alternate Interior Angles Theorem)
Learn more on postulates here:https://brainly.com/question/10440639
Want Money. Answer thisss below.
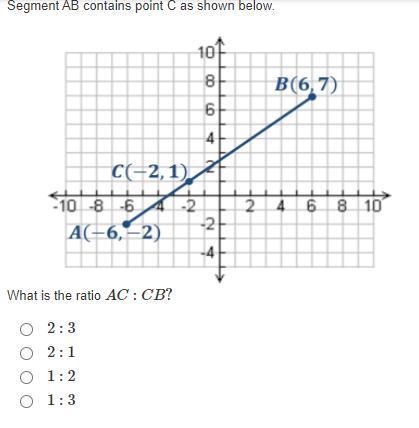
Answers
Answer:
2.3
Step-by-step explanation:
A cube has side length a.The side lengths are decreased to 30% of their original size.Write an expression in simplest form for the volume of the cube in terms of a.
Answers
Answer:
Volume V = 0.027a^3
Step-by-step explanation:
Let a represent the length of the side length of the cube.
a.The side lengths are decreased to 30% of their original size;
l = 30% of a
l = 0.3a .....1
The volume of a cube can be expressed as;
V = l × l × l
V = l^3 .......2
Where;
l = side length
Substituting the value of l into equation 2;
V = l^3 = (0.3a)^3
V = 0.027a^3
Volume V = 0.027a^3
Tell me about a time you had to solve a complex problem. How did you break down the problem into smaller units to create a solution?.
Answers
Answer:
Look at the big picture. Make sure you understand what the end product is supposed to look like.
Examine the parts of the task. Figure out step-by-step what you need to do because it’s not going to happen through magic.
Think about the logical order of completing the pieces. What should you do first, second, third, etc.?
Step-by-step explanation:
Ya basically that!
please mark brainliest i need 2 more :(