Find an equation of the tangent plane to the surface at the given point. 3x2 + 2y2 + 4z2 = 18, P = (2, 1, 1) = = (Express numbers in exact form. Use symbolic notation and fractions where needed. Let f(x, y, z) and give the equation in terms of x, y, and z.)
Answers
Then, we can write the equation of the tangent plane in terms of x, y, and z:z = 6/7 x - 3/7 y + 15/14.
The equation of the tangent plane is the following one:
z = 6/7 x - 3/7 y + 15/14
In order to find the equation of the tangent plane to the surface at the given point, we have to use partial derivatives of the function f(x, y, z)
= 3x² + 2y² + 4z² - 18.
Then we have:
fx(x, y, z) = 6x f(x, y, z)fy(x, y, z)
= 4y f(x, y, z)fz(x, y, z)
= 8z f(x, y, z)
We need the partial derivatives evaluated at the point P = (2, 1, 1),
then we have:
fx(2, 1, 1) = 6*2
= 12fy(2, 1, 1)
= 4*1
= 4fz(2, 1, 1)
= 8*1
= 8
The equation of the tangent plane is given by the formula:
z - z₀ = fx(x₀, y₀, z₀)(x - x₀) + fy(x₀, y₀, z₀)(y - y₀) + fz(x₀, y₀, z₀)(z - z₀)
where (x₀, y₀, z₀) = (2, 1, 1),
then we have:
z - 1 = 12(x - 2) + 4(y - 1) + 8(z - 1)
Now, we simplify the equation:
7z = 6x - 3y + 15
To know more about tangent plane visit:
https://brainly.com/question/30565764
#SPJ11
Related Questions
I'm lost at this point, if you can help me I'll give you 10 points..
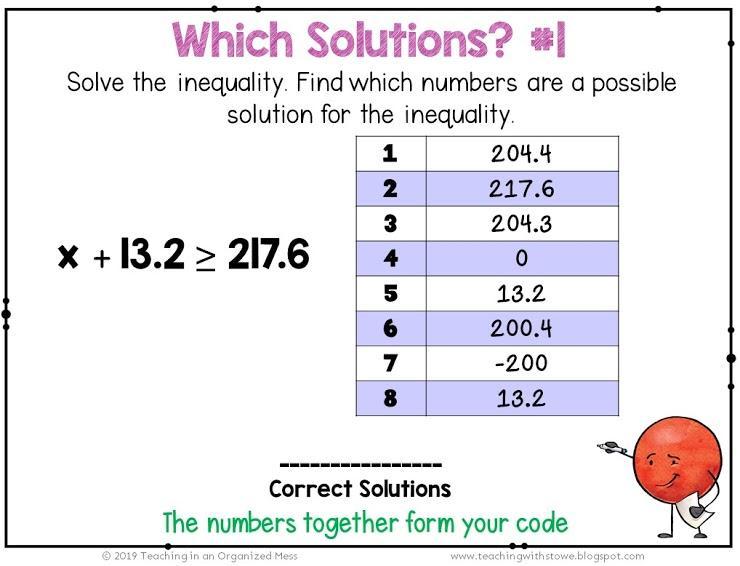
Answers
Answer:
So all the possible solutions are:
\(\bold{204.4~and~217.6.}\)Solution Steps:
First you need to solve the real inequality to understand how to find the rest of the possible equations.
Subtract 13.2 from both sides:
\(13.2-13.2=\) Cancels Out\(217.6-13.2=204.4\)So now we know the real answer, but now we must find all the possible solutions.
(Means anything greater than or equal to 217.6 when you plug it into x + 13.2 ≥ 217.6.)
Numbers that are greater than 378:
1.) \(204.4+13.2=217.6\) ≥ \(217.6\) (True)
2.) \(217.6+13.2=230.8\) ≥ \(217.6\) (True)
3.) \(204.3+13.2=217.5\) ≥ \(217.6\) (False)
4.) \(0+13.2=13.2\) ≥ \(217.6\) (False)
5.) \(13.2+13.2=26.4\) ≥ \(217.6\) (False)
6.) \(200.4+13.2=213.6\) ≥ \(217.6\) (False)
7.) \(-200+13.2=-186.8\) ≥ \(217.6\) (False)
8.) \(13.2+13.2=26.4\) ≥ \(217.6\) (False)
Now put all the numbers together to form the code.
(Could either be the numbers on the side of the table or the accumulation of all the numbers in possible solutions.)
Code:
\(2044,2176\) or \(1,2\)______________________________
\(\bold{Hope~this~helps!}\\\bold{If~you~need~help~with~anything~else,~feel~free~to~ask!}\\\\\bold{~~~~~-TotallyNotTrillex}\)
PLEASE help its easy I'm just dum!! Find the scale factor, for more info look at picture below.

Answers
The required scale factor is 2/3 for the given figures.
What is a scale image?Scale image is defined as a ratio that represents the relationship between the shape and size of a figure and the corresponding dimensions of the actual figure or object.
As we know scale factor is the ratio of sides in the original image and the image after transformation.
The side length of CD = 15 units
The side length of GH = 10 units
The scale factor = length of GH / length of CD
The scale factor = 10/15
The scale factor = 2/3
Therefore, the required scale factor is 2/3 for the given figures.
Learn more about the Scale images here:
brainly.com/question/13194929
#SPJ1
1. Sandy has 1.45 L of soft drink in a bottle. She pours the soft drink into glasses with capacity of 0.32 L each. At least how many glasses must she use in order to pour all soft drink out of the bottle?
2. A baker has 5.6 kg of flour. Later he buys 2.5 kg of flour. If he needs 0.48 kg of flour to make a dough, then how many doughs can he make with the flour? How much flour remains (in kg)?
Answers
Step-by-step explanation:
1.
how many glasses of 0.32 liter can she fill ?
that means, how often does 0.32 fit into 1.45 ?
that is solved by division :
1.45 / 0.32 = 4.53125
so, she will need at least 5 glasses to completely empty the bottle. the 5th glass will be filled only partly, but that does not matter in that regard.
2.
he has 5.6 kg and adds 2.5 kg. that is 5.6 + 2.5 = 8.1 kg.
1 dough (that means 1 portion of dough as described by his recipe) uses 0.48 kg flour.
how many doughs can he make
so, again, how often does 0.48 fit into 8.1 ?
8.1 / 0.48 = 16.875
he can therefore make 16 full portions of dough. he does not have enough flour for a full 17th.
so, he uses
16×0.48 = 7.68 kg of flour for the 16 doughs.
and he has
8.1 - 7.68 = 0.42 kg of flour left.
Suppose that f′(x)=2xf′(x)=2x for all xx.
a) Find f(1)f(1) if f(0)=0f(0)=0.
b) Find f(1)f(1) if f(3)=6f(3)=6.
c) Find f(1)f(1) if f(−2)=6f(−2)=6.
when f(0)=0f(1)f(0)=0f(1)
when f(3)=6f(1)f(3)=6f(1)
when f(−2)=6f(1)
Answers
We have found that f(1) = 1 when f(0) = 0, f(1) = -2 when f(3) = 6, and f(1) = 3 when f(-2) = 6. This can be answered by the concept of slope.
The problem asks us to find the value of f(1) given some information about the derivative of the function f(x). We are given that f'(x) = 2x for all x, and we need to use this information to find specific values of f(1) given different conditions on f(x).
To solve this problem, we need to use the fact that the derivative of a function gives us information about the rate of change of the function at any given point. Specifically, the derivative tells us the slope of the tangent line to the function at that point.
a) We are given that f'(x) = 2x for all x, and we know that f(0) = 0. This tells us that the function f(x) must be of the form f(x) = x² + C, where C is a constant that we can find by using the initial condition f(0) = 0. Plugging in x = 0, we get f(0) = 0² + C = 0, which implies that C = 0. Therefore, f(x) = x², and f(1) = 1² = 1.
b) We are given that f'(x) = 2x for all x, and we know that f(3) = 6. Using the same reasoning as before, we can write f(x) = x² + C, where C is a constant that we can find using the initial condition f(3) = 6.
Plugging in x = 3, we get f(3) = 3² + C = 9 + C = 6, which implies that C = -3.
Therefore, f(x) = x² - 3, and f(1) = 1² - 3 = -2.
c) We are given that f'(x) = 2x for all x, and we know that f(-2) = 6. Using the same reasoning as before, we can write f(x) = x² + C, where C is a constant that we can find using the initial condition f(-2) = 6.
Plugging in x = -2, we get f(-2) = (-2)² + C = 4 + C = 6, which implies that C = 2.
Therefore, f(x) = x² + 2, and f(1) = 1² + 2 = 3.
Therefore, we have found that f(1) = 1 when f(0) = 0, f(1) = -2 when f(3) = 6, and f(1) = 3 when f(-2) = 6.
To learn more about slope here:
brainly.com/question/19131126#
#SPJ11
PLEASE HELPPP WILL GIVE BRAINLIEST
Look at the screenshot attached
Step 1: Select Value
commutative property of addition
associative property of addition
distributive property
Step 2: Select Value
commutative property of addition
associative property of addition
distributive property
Step 3: Select Value
commutative property of addition
associative property of addition
distributive property

Answers
Answer:
step 1 is commutative property of addition
step 2 is commutative property of multiplication
step 3 is distributive property
Answer:
Step 1- commutative property of addition
Step 2- distributive property
Step 3- associative property of addition
:)
Use the Integrating Factor Method to solve the following differential equations: x⁴ dy/dx + 2x⁴y = x⁴e⁻ˣ
a) Rewrite the equation in Standard Form. b) Identify P(x). c) Identify Q(x). d) Evaluate Integrating Factor. e) Solve for the general solution.
Answers
The equation in Standard Form. b) Identify P(x). c) Identify Q(x). d) Evaluate Integrating Factor. e) Solve for the general solution are given below:
a) Rewrite the equation in Standard Form:
To rewrite the equation in standard form, divide the entire equation by x⁴:
dy/dx + 2y = e^(-x)
b) Identify P(x):
In standard form, the coefficient of the y term is 2, which is the function P(x). So, P(x) = 2.
c) Identify Q(x):
In standard form, the right-hand side of the equation is e^(-x), which is the function Q(x). So, Q(x) = e^(-x).
d) Evaluate the Integrating Factor:
The integrating factor (IF) is given by the exponential of the integral of P(x) with respect to x. In this case, the integrating factor is:
IF = e^(∫P(x)dx) = e^(∫2dx) = e^(2x)
e) Solve for the general solution:
Multiply the entire equation by the integrating factor (IF = e^(2x)):
e^(2x) * (dy/dx + 2y) = e^(2x) * e^(-x)
Simplify the left side by applying the product rule of exponents:
(e^(2x) * dy/dx) + 2y * e^(2x) = e^(x)
Notice that the left side is now in the form (f(x)g(x))' = f'(x)g(x) + f(x)g'(x), where f(x) = y and g(x) = e^(2x). Apply the product rule and simplify further:
(d/dx)(y * e^(2x)) = e^(x)
Integrate both sides with respect to x:
∫(d/dx)(y * e^(2x)) dx = ∫e^(x) dx
Integrating the left side gives:
y * e^(2x) = ∫e^(x) dx = e^(x) + C₁, where C₁ is the constant of integration.
Finally, solve for y by dividing both sides by e^(2x):
y = (e^(x) + C₁) / e^(2x)
This is the general solution to the given differential equation using the Integrating Factor Method.
Learn more about coefficient here:
https://brainly.com/question/13431100
#SPJ11
An arc of length 8 in. Is intersected by a central angle in a circle with a radius of 3 in. What is the measure of the angle? Round your answer to the nearest tenth. 0. 4 radian 1. 0 radian 2. 7 radians 5. 0 radians.
Answers
To solve the problem we must know about the formula of the length of the Arc.
Length of Arc\(\rm{ Length\ of\ Arc = \dfrac{2\pi r \theta}{360^o}\)
The angle made by the arc in the center is 2.7 radians.
ExplanationGiven to us
Length of the arc = 8 in.radius of the circle = 3 in.AssumptionLet the angle be θ.
Measure of the AngleSubstituting the values in the formula of Length of Arc,
\(\rm{ Length\ of\ Arc = \dfrac{2\pi r \theta}{360^o}\\\\ \)
\(8\ in. = \dfrac{2\pi \times 3 \times \theta}{360^o}\)
\(\theta = \dfrac{360^o\times 8\ in.}{2\pi \times 3 }\\\\ \theta = 152.788^o \approx 152.79^o\)
Degree to Radian\(\theta = 152.79^o\\\\ \)
\(=\dfrac{\theta \times \pi}{180^o}\)
\(\rm{=2.6666667 \approx 2.7\ radians\)
Hence, the angle made by the arc in the center is 2.7 radians.
Learn more about Length of Arc:
https://brainly.com/question/1577784
one-sample z test of the assumed 95% lower n mean se mean bound z p 8 105.20 1.77 ? ? ? standard deviation 5 mu 100 vs 7 100. ) fill in the missing values in the output. can the null hypothesis be rejected at the 0.05 level of significance? explain your answer. (b) suppose that the alternative hypothesis had been what is the p-value in this situation? can the null hypothesis be rejected at the 0.05 level of significance? (c) suppose that you were asked to find a 95% two-sided ci on the mean. would the lower confidence bound in the two-sided ci be greater than the one-sided lower confidence bound that you computed in part (a)?
Answers
The null hypothesis can be rejected at the 0.05 or 5 % level of significance according to Decision Rule.
The null hypothesis is a type of hypothesis that explains the population parameter and is used to examine if the provided experimental results are reliable. Depending on whether the population or sample under consideration is viable, this hypothesis is either rejected or not. Or to put it another way, the null hypothesis is a hypothesis that assumes that the sample observations are the product of chance. It is claimed to be a claim made by surveyors who wish to look at the data. The symbol for it is H0.
Given : n = 8
\(\large \bar{X}=105.20 \\\\ \larg\frac{\sigma}{\sqrt{n}}=1.77 \\ \\ \large \alpha=0.05 \large \mu_0=100\)
a ) We want to find the 95% confidence interval for mean
Therefore ,
\(\large (105.20-Z_{0.05}*1.77,105.20+Z_{0.05}*1.77)\\\\\large (105.20-1.64*1.77,105.20+1.64*1.77)\\\\\large (105.20-2.9028,105.20+2.9028)\)
(102.2972,108.1028)
b ) Hypothesis :
\(\large H_0:\mu=100 \\ \\ \large H_1:\mu\neq 100\)
The test statistic under H is given by ,
\(\large Z\rightarrow N(0,1)\\\\ \large Z =\frac{105.20-100}{1.77}\\\\ \large =\frac{5.20}{1.77}\\\\\large =2.9379\)
\(P value \large =P(Z > |Z_{cal}|)\)
=P(Z>2.9379)
=0.001652
Decision Rule : If P value \(< \large \alpha\) then reject at \(\large \alpha\) % level of significance accept otherwise
Here , P value = 0.001652 < \large \alpha = 0.05
Therefore , reject H at 5% level of significance.
Learn more about Null Hypothesis:
https://brainly.com/question/15980493
#SPJ4
A friend of yours, a senior, took the Graduate Record Exam in September and scored in the 99th percentile. In February, your friend took the same exam over again. This time your friend scored in the 90th percentile. As a research methods student, you told your friend that his/her lowered score was most likely due to:
Answers
His/her lowered score was most likely due to statistical regression.
How to determine the reason?The missing options in the question are:
A. compensation rivalry B. Demoralization C. Differential selection
D. Testing E. Statistical regression
From the question, we have:
September = 99th percentileFebruary = 90th percentileA change (whether higher or lower) in the score is caused by statistical regression.
This is so because several variables could attribute to the change in the score.
The relationship between these variables is referred to as statistical regression
Read more about statistical regression at:
https://brainly.com/question/25987747
#SPJ12
Find the volume of
a cone with a
radius of 3 meters
and a height of 2
meters. Give an exact
answer.
Answers
The formula for the volume of a cone is V = (1/3)πr²h, where r is the radius and h is the height.
Substituting the given values, we get:
V = (1/3)π(3²)(2)
V = (1/3)π(9)(2)
V = (1/3)π(18)
V = (6/3)π
V = 2π
Therefore, the exact volume of the cone is 2π cubic meters.
150 students living in Dunedin hostels became sick with the flu over a 3 month period. What measure of occurrence does this statement describe
Answers
The statement describes the incidence measure of occurrence, which refers to the number of new cases of a disease or condition that occur in a defined population over a specific period of time.
This statement describes the incidence rate of flu among students living in Dunedin hostels.
The incidence rate is a measure of occurrence that calculates the number of new cases (in this case, students getting sick with the flu) in a specific population (150 students in Dunedin hostels) over a specific time period (3 months). This rate helps us understand the frequency at which the flu is affecting this particular group of students
For similar question on population.
https://brainly.com/question/28683624
#SPJ11
PLEASE HELP ASAP 15 POINTS

Answers
values for different values of x.which expression represents h(x)?(f ∙ g)(x)(g f)(x)(f – g)(x)(g – f)(x)
Answers
The expression that represents h(x) is (f - g)(x).
In the given options, we need to determine the expression that represents h(x). The expression (f - g)(x) represents the subtraction of g(x) from f(x). By subtracting the value of g(x) from f(x) at a given x, we obtain the value of h(x). This expression captures the concept of finding the difference between the two functions at a specific input value.
To understand the concept further, let's break down the expression (f - g)(x) into its components. The subtraction operation (f - g) indicates that we are subtracting the values of g(x) from f(x). This means that for each value of x, we evaluate f(x) and g(x), and then subtract the latter from the former. By doing so, we obtain the value of h(x), which represents the difference between the two functions.
The expression (f - g)(x) is a concise and clear way to denote the function h(x). It helps us understand the relationship between f(x) and g(x) by focusing on the difference between their values at each x. By utilizing this expression, we can analyze the behavior and properties of h(x) in various mathematical contexts.
Learn more about: Specific input value
brainly.com/question/30397084
#SPJ11
Which of the following statements is true regarding the influence of a small alpha level in the context of hypothesis testing? O a. A small alpha level reduces the effect size. O b. A small alpha level increases statistical power. c. A small alpha level reduces the likelihood of a Type I error. O d. A small alpha level increases the likelihood of detecting statistical significance
Answers
The influence of a small alpha level in the context of hypothesis testing is:
c. A small alpha level reduces the likelihood of a Type I error.
What is null hypothesis?A hypothesis known as the null hypothesis states that sample observations are the result of chance.
The correct statement regarding the influence of a small alpha level in the context of hypothesis testing is:
c. A small alpha level reduces the likelihood of a Type I error.
A small alpha level (typically denoted as α) is the significance level used in hypothesis testing to determine the threshold for rejecting the null hypothesis. By setting a small alpha level, such as 0.01 or 0.05, we are reducing the probability of making a Type I error, which is the incorrect rejection of a true null hypothesis.
In other words, a small alpha level provides a stricter criterion for rejecting the null hypothesis, making it less likely to reject the null hypothesis when it is true. This reduces the likelihood of claiming a significant result when there is no true effect or relationship in the population.
Learn more about null hypothesis on:
https://brainly.com/question/28042334
#SPJ4
how many solutions does this equation have 2m=8+3m
Answers
Answer:
2
Step-by-step explanation:
because there are 2 x'S
Answer:
hiiii lol. so ganfNFnnq6mq6n1j
Solve for x in the triangle. Round your answer to the nearest tenth.
6
X
69°
X

Answers
hello i am just need points so sorry you didnt get answer
its a 50 50 question both A and B are wrong please help

Answers
Answer: d/ 30x +200 <= 1400
Step-by-step explanation:
It can't be the first two answers because the truck can only hold 1400
So it must be < or = 1400
It says that she loaded computers for 200 pounds and x number of books, 30 pounds each.
So the equation is 30x + 200 <=1400
Longitud de circunferencia si diámetro es 32cm
Answers
Por lo tanto, la longitud de la circunferencia con un diámetro de 32 cm sería aproximadamente 100.53 cm.
How to solve for the circumferenceLa fórmula para calcular la longitud de una circunferencia es:
Longitud = π * Diámetro
En este caso, si el diámetro es de 32 cm, podemos calcular la longitud de la siguiente manera:
Longitud = π * 32 cm
El valor de π (pi) es una constante que representa la relación entre la circunferencia de un círculo y su diámetro. Usualmente, se aproxima a 3.14159.
Por lo tanto, la longitud de la circunferencia sería:
Longitud ≈ 3.14159 * 32 cm
Calculando el resultado:
Longitud ≈ 100.53096 cm
Por lo tanto, la longitud de la circunferencia con un diámetro de 32 cm sería aproximadamente 100.53 cm.
Read moe on circumference here https://brainly.com/question/27447563
#SPJ1
what is sin x or sin y?
Answers
The sine x or sine theta can be defined as the ratio of the opposite side of a right triangle to its hypotenuse.
The sine function relates a real number t to the y-coordinate of the point where the corresponding angle intercepts the unit circle.
What is meant by trigonometric function?Trigonometric functions are also known as Circular Functions can be simply defined as the functions of an angle of a triangle. It means that the relationship between the angles and sides of a triangle are given by these trig functions.
Trigonometric functions are functions that relate an angle in a right angled triangle to the ratio of two of its sides. Sin , cos and tan are trigonometric functions.
Learn more about Trigonometric functions at:
https://brainly.com/question/13019801
#SPJ4
evaluate each expression; 6t + 9 - 22; t = 60
Answers
We have the next expression
\(6t+9-22\)if t=60
Then we evaluate the expression with the value of t given
\(6(60)+9-22=347\)the result is 347
A drone is flying at the altitude of 16 1/4 feet above sea level a groundhog is directly below the drone at an altitude of 7 3/8 feet below sea level what is the distance between the drone and the groundhog
Answers
one is above sea level the other below sea level, so their distance is just their sum from sea level, so hmmm let's convert the mixed fractions to improper fractions and then sum them up.
\(\stackrel{mixed}{16\frac{1}{4}}\implies \cfrac{16\cdot 4+1}{4}\implies \stackrel{improper}{\cfrac{65}{4}} ~\hfill \stackrel{mixed}{7\frac{3}{8}} \implies \cfrac{7\cdot 8+3}{8} \implies \stackrel{improper}{\cfrac{59}{8}} \\\\[-0.35em] ~\dotfill\\\\ \cfrac{65}{4}+\cfrac{59}{8}\implies \cfrac{(2)65~~ + ~~(1)59}{\underset{\textit{using this LCD}}{8}}\implies \cfrac{130+59}{8}\implies \cfrac{189}{8}\implies 23\frac{5}{8}\)
Mr. Rodriguez is packing bags of snacks for his children’s lunchboxes. If he has 20 blueberries and 30 grapes, what is the maximum number of bags of snacks that he can pack so that each bag has the same number of blueberries and grapes?
5 bags
10 bags
50 bags
60 bags
Answers
Answer:
10bags
Step-by-step explanation:
Answer:
10 bags.
Step-by-step explanation:
I got it right on my quiz- Edge2020
Probability please help

Answers
Answer: 7/5
Step-by-step explanation:
Answer:
5/12
Step-by-step explanation:
So, 14 boys and 10 girls. 24 total kids.
14+10=24
10 girls means 10/24
simplified to 5/12
A box of chocolates contains five milk chocolates, three dark chocolates, and 8 white
chocolates. You randomly select and eat three chocolates. The first piece is milk
chocolate, the second is dark chocolate, and thethird is white chocolate.
What is the probability that this event happens?
Answers
Answer:
60/200
Step-by-step explanation:
credit to pawlossolomon
Felipe calculated that he uses about 545 gallons of fuel each year. His car's owner's manual says that it is approved to use regular fuel. How much money can he save each year by switching from premium fuel, at $4.59 per gallon, to regular fuel at $4.18 per gallon?
$0.41
$2,501.55
$223.45
$2,278.10
$4,779.65
Answers
Answer:
$223.45
Step-by-step explanation:
premium fuel: 4.59 x 545
= 2501.55
regular fuel: 4.18 x 545
=2278.1
2501.55-2278.1 = 223.45
The quotient of a number and 8 decreased by 17 is -15. What is the number?
Answers
Answer: 1/4
Step-by-step explanation:

Use the picture below to answer questions 14a and 14b
14a) What is the value of x?
14b) What is the measure, in degrees, of angle BDE?

Answers
Answer:
x = 35, ∠ BDE = 130°
Step-by-step explanation:
(4x - 10) and (x + 95) are corresponding angles and are congruent, then
4x - 10 = x + 95 ( subtract x from both sides )
3x - 10 = 95 ( add 10 to both sides )
3x = 105 ( divide both sides by 3 )
x = 35
Thus
∠ BDE = 4x - 10 = 4(35) - 10 = 140 - 10 = 130°
16y2 – 25
help me i hate this school
Answers
Answer:
(4y)2 - (5)2 which is in the form a2 - b2
= (4y + 5) (4y - 5)
Step-by-step explanation:
a
2
−
b
2
=
(
a
+
b
)
(
a
−
b
)
a
2
-
b
2
=
(
a
+
b
)
(
a
-
b
)
where
a
=
4
y
a
=
4
y
and
b
=
5
b
=
5
.
(
4
y
+
5
)
(
4
y
−
5
)
Mr. Burrows bought a
total of 63 mugs for the
Staff lunch room. The total
was $150.57.
How much would is mugs cost?
Answers
If h(x) = -x(1 - x), find h(-3).
Answers
Answer:
12
Step-by-step explanation:
h(-3)= -(-3)(1-(-3))=
3(4)=12