Evaluate each value expression for the given value of x : 22-x/5 , x=20
HURY!!!!!!!!!!!
Answers
22-20/5
22-4
=18
Answer:
10 maybe sorry if wrong
Step-by-step explanation:
22-x x=20 so that should be 2 then u do 2x5 =10
Related Questions
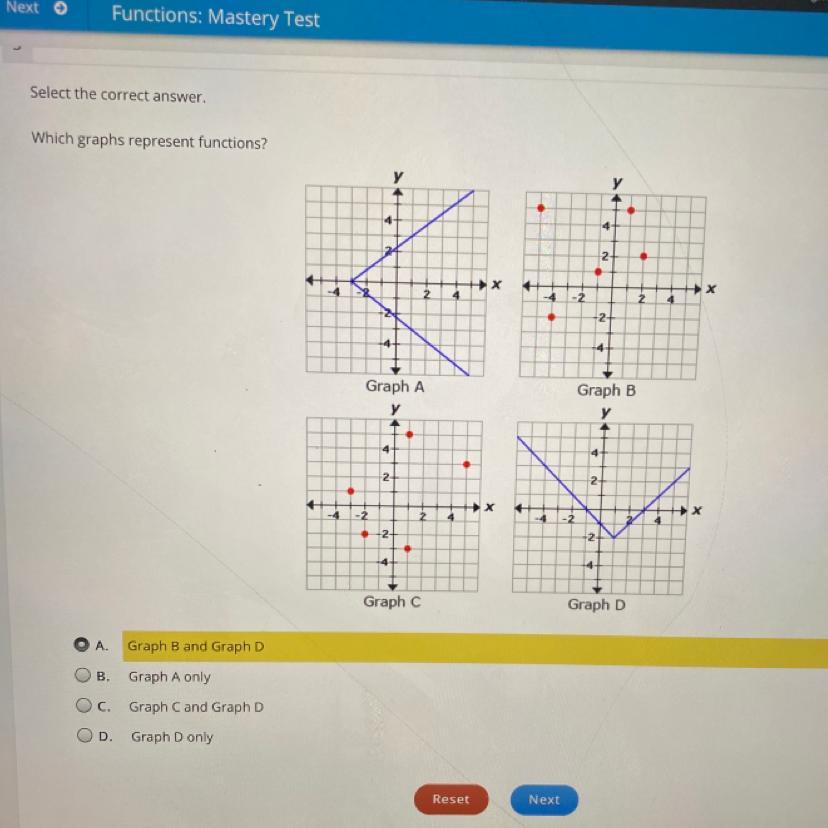
A. Graph B and Graph D
B. Graph A only
C. Graph C and Graph D
D. Graph D only
Answers
Answer:
A is correct answer
Step-by-step explanation:
like me
what is the answer

Answers
Answer:
A
Step-by-step explanation:
Indicate which of the following statements are correct (+) or incorrect (−). In the explicit form of a DE, the lowest derivative is isolated on one side of the equation An ordinary DE consists of only polynomial and/or rational functions A second order ODE is one in which the derivative is equal to a quadratic function 【 In an implicit ODE, the highest derivative is not isolated. [4] b. Solve the following initial value problem y′1+x2=xy3y(0)=−1 [5] c. Solve the following 1st order ODE: tlntdtdr+r=tet [7] d. Find the general solution of the following 2 nd order inhomogeneous ODE: ψ¨+2ψ˙+50ψ=12cos5t+sin5t [2] e. A ham sandwich is dropped from the height of the 381 m tall Empire State Building. The sandwich is effectively a square flat plate of area 0.1×0.1 m and of mass 0.25 kg. The drag on an object of this size falling at a reasonable speed is proportional to the square of its instantaneous velocity v. The velocity of the sandwich will increase until it reaches terminal velocity when the drag exactly equals its weight. The resulting equation of motion for the free-falling sandwich in air is given by Newton's Second Law: dtd(mv)=mg−0.01Av2 Assuming the sandwich falls flat, does not come apart and its mass does not change during its fall, find the equation describing its terminal velocity vf as a function of time.
Answers
a) The statement in part (a) is correct. When in the explicit form of a differential equation, the lowest derivative is isolated on one side of the equation.
b) To solve the initial value problem. Thus, z′−3x2z=3 and by multiplying both sides of the equation by
\(e^∫−3xdx=e^-3x\), we get:
e^-3xz′−3e^-3xx2z
\(=3e^-3x+C\) Know let's multiply both sides by\(x^3\) and get:
\(z′x3−3x2z=3x^3e^-3x+C\) Keeping in mind that
\(z=y3−1\), we have:
\(y3=x+12e3x+Cx3+d\)
where C and d are constants of integration.
c) Here's the solution to the first-order ODE:
Differentiating both sides with respect to t yields:
\(d/dt[tlnt] = dt/dt, d/dt[t] + td/dt[ln(t)]\)
\(= e^t, 1/t*dr/dt + r/t\)
= e^t. \(= e^t.\)
\(dtd(mv)=0\) and the drag on the sandwich exactly equals its weight.
To know more about statement visit:
https://brainly.com/question/33442046
#SPJ11
Joni has a circular garden with a diameter of 16.5. If she uses 2 teaspoons of fertilizer for every 25 square feet of garden how many teaspoons of fertilizer will Joni need for her entire garden?
Answers
Answer:
17.106 Teaspoons of fertilizer
Step-by-step explanation:
Joni has a circular garden with a diameter of 16.5. If she uses 2 teaspoons of fertilizer for every 25 square feet of garden how many teaspoons of fertilizer will Joni need for her entire garden?
Step 1
We calculate the area of the garden.
The garden is circular in shape.
Area of a circle = πr²
r = radius = Diameter/2
Diameter = 16.5 ft
r = 16.5/2
r = 8.25 ft
Area of the circle = π × 8.25²
Area of the circle (Garden) = 213.82464998 ft²
Approximately = 212.825ft²
Step 2
If she uses 2 teaspoons of fertilizer for every 25 square feet of garden how many teaspoons of fertilizer will Joni need for her entire garden?
25 ft² = 2 teaspoons of fertilizer
212.825ft² = x
Cross Multiply
25 ft² × x = 212.825ft² × 2
x = 213.825× 2/25 ft²
x = 17.106 Teaspoons of fertilizer
please help me solve this :)

Answers
In a large package of markers, 28 of the 448 markers are red. What percent of the markers are red?
Answers
Answer:
6.25%
Step-by-step explanation:
percentage of red = ( no. of red markers / total no. of markers) *100
= 28 *100 / 448
= 6.25%
clerks at mosier data systems key in thousands of insurance records each day for a variety of client firms. samples of the work of 20 clerks are gathered. ceo donna mosier carefully examines 100 records entered by each clerk and counts the number of errors. mosier wants to set control limits to include 99.73% of the random variation in the data entry process. which type of process control chart should she use?
Answers
Donna Mosier should use an Individuals control chart to set control limits for the data entry process.
An Individuals control chart is a type of process control chart used to monitor the process when the sample size is one. In this case, Mosier is examining 100 records entered by each of the 20 clerks, making the sample size one.
To set control limits that include 99.73% of the random variation in the data entry process, Mosier can use the following steps:
Calculate the mean and standard deviation of the number of errors for each clerk based on the 100 records examined.Calculate the Upper Control Limit (UCL) and Lower Control Limit (LCL) using the formulas: UCL = mean + 3 * standard deviation and LCL = mean - 3 * standard deviation.Plot the individual data points for each clerk on the Individuals control chart, with the UCL and LCL as the upper and lower boundaries, respectively.Monitor the data points over time to detect any trends, shifts, or out-of-control points that may indicate a process issue.By using an Individuals control chart, Mosier can set control limits to monitor the data entry process and detect any issues before they become major problems.
Learn more about control chart
https://brainly.com/question/26478724
#SPJ4
What is half of the world’s population
Answers
Answer:
3,900,000,000
Step-by-step explanation:
The current global population is 7,800,000,000.
Half of this is 3,900,000,000.
So to find half of the world’s population, divide 7,800,000,000 by two.
So, half of the world’s population is 3,900,000,000
Do the following using the given information: Utility function u(x1+x2) = .5ln(x1) + .25ln(x₂) .251 Marshallian demand X1 = - and x₂ = P₂ . Find the indirect utility function . Find the minimum expenditure function . Find the Hicksian demand function wwww
Answers
Hicksian demand functions are:x1** = 2P₁x₂ ; x₂** = P₂²
Utility function: u(x1+x2) = .5ln(x1) + .25ln(x₂) .The Marshallian demand functions are: x1* = - and x₂* = P₂.
The indirect utility function is found by substituting Marshallian demand functions into the utility function and solving for v(P₁, P₂, Y).u(x1*,x2*) = v(P₁,P₂,Y) ⇒ u(-, P₂) = v(P₁,P₂,Y) ⇒ .5ln(-) + .25ln(P₂) = v(P₁,P₂,Y) ⇒ v(P₁,P₂,Y) = - ∞ (as ln(-) is not defined)
Thus the indirect utility function is undefined.
Minimum expenditure function can be derived from the Marshallian demand function and prices of goods:
Exp = P₁x1* + P₂x2* = P₁(-) + P₂P₂ = -P₁ + P₂²
Minimum expenditure function is thus:
Exp = P₁(-) + P₂²
Hicksian demand functions can be derived from the utility function and prices of goods:
H1(x1, P1, P2, U) = x1*H2(x2, P1, P2, U) = x2*
Hicksian demand functions are:
x1** = 2P₁x₂
x₂** = P₂²
If there are no restrictions on the amount of money the consumer can spend, the Hicksian demand functions for x1 and x2 coincide with Marshallian demand functions.
Learn more about utility function at:
https://brainly.com/question/32708195
#SPJ11
A box has a width of 5 centimeters and a height of 6 centimeters. If the volume is 150 cubic centimeters, what is the length of the box?
A) 5 centimeters
B) 4 centimeters
C) 7 centimeters
D) 3 centimeters
Answers
Answer: A. 5
Step-by-step explanation:
Answer:
A) 5 cm.
Step-by-step explanation:
Volume = length * width * height
150 = length * 5 * 6
Length = 150 / (5*6)
= 150/30
= 5.
the distance from city a to city b is 256.8 miles. the distance from city a to city c is 739.4 miles how much farther is the trip to city c than the trip to city b
Answers
Taking a difference, we can see that the trip to city C is 482.6 mi longer.
How much farther is the trip to city c than the trip to city b?
Here we know that the distance from city a to city b is 256.8 miles, and the distance from city a to city c is 739.4 miles
To find how much farther is the trip to city c than the trip to city b, we just need to take the difference between the two distances above.
That means that we need to take the distance to city c and subtract the distance to city b.
We will get:
739.4 mi - 256.8 mi = 482.6 mi
The trip to city C is 482.6 mi more than the trip to city B.
Learn more about differences at:
https://brainly.com/question/17695139
#SPJ1
is the quotient of two rational numbers always a rational number explain
Answers
In a subsurface system, we have reverse faulting, a pressure is identified at the depth of
2,000 ft with A = 0.82. Given this information, calculate: the total maximum horizontal stress
Shmaz given friction angle 4 = 30°.
Answers
To calculate the total maximum horizontal stress (Shmax) in a subsurface system with reverse faulting, we can use the formula:
Shmax = P / A
where P is the pressure at the given depth and A is the stress ratio. Given: Depth = 2,000 ft, A = 0.8, Friction angle (φ) = 30°
First, we need to calculate the vertical stress (σv) at the given depth using the equation:
σv = ρ g h
where ρ is the unit weight of the overlying rock, g is the acceleration due to gravity, and h is the depth.
Next, we can calculate the effective stress (σ') using the equation:
σ' = σv - Pp
where Pp is the pore pressure.
Assuming the pore pressure is negligible, σ' is approximately equal to σv.
Finally, we can calculate Shmax using the formula:
Shmax = σ' * (1 + sin φ) / (1 - sin φ)
Substituting the given values into the equations, we can calculate Shmax. However, the unit weight of the rock and the value of g are required to complete the calculation.
Learn more about horizontal stress (Shmax) here:
https://brainly.com/question/31642399
#SPJ11
Last year, a gardening store ordered 530,570 potted plants. This year, they ordered 424,456 potted plants. What is the percent of decrease in the number of potted plants ordered annually?
Answers
Answer:
last year = 530570
this year = 424456
decreased plants = 530570-424456 = 106114
decreased percent = 106114÷530570×100%
= 20%
therefore 20% is the decrease precent in the number of potted plants ordered anually
You roll a fair six-sided die and record the result X. You roll the die again and record the result Y.
Answers
The probability of rolling a sum of 10 is \(\frac{1}{18}\)
as we know that total number of outcomes for a dice is equal to six raised to the power times the dice is rolled
that is, total number of outcomes = \(6^{2} = 36\)
also, the sum can be equal to 10 in only two cases, (6,4) and (5,5)
according to the formula
Probability = \(\frac{favorable events}{ total number of outcome} = \frac{2}{36} = \frac{1}{18}\)
What is Probability?The area of mathematics known as probability deals with numerical representations of the likelihood that an event will occur or that a statement is true.An event's probability is a number between 0 and 1, where, roughly speaking, 0 denotes the event's impossibility and 1 denotes certainty. The likelihood that an event will occur increases with its probability.Probability theory, which is widely used in fields of study like statistics, mathematics, science, finance, gambling, artificial intelligence, machine learning, computer science, game theory, and philosophy, has given these ideas an axiomatic mathematical formalization. For example, it can be used to infer information about the expected frequency of events.To learn more about Probability with the given link
https://brainly.com/question/3392790
#SPJ4
Question:
You roll a fair six-sided die and record the result X. You roll the die again and record the result Y. What is the probability of rolling a sum of 10?
find the centroid ( ¯ x , ¯ y ) of the triangle with vertices at ( 0 , 0 ) , ( 5 , 0 ) , and ( 0 , 7 ) .
Answers
The centroid of a triangle is the point where the three medians of the triangle intersect. In this case, the triangle has vertices at (0, 0), (5, 0), and (0, 7).
First, let's calculate the average x-coordinate:
¯x = (0 + 5 + 0) / 3 = 5/3 ≈ 1.67
Next, let's calculate the average y-coordinate:
¯y = (0 + 0 + 7) / 3 = 7/3 ≈ 2.33, the centroid of the triangle with vertices at (0, 0), (5, 0), and (0, 7) is approximately (1.67, 2.33).
In summary, the centroid of the triangle with verticesvertices at (0, 0), (5, 0), and (0, 7) is located at approximately (1.67, 2.33). This point represents the average position of the three vertices and is the intersection point of the medians of the triangle.
The centroid coordinates are found by taking the average of the x-coordinates and the average of the y-coordinates of the three vertices. In this case, we add up the x-coordinates (0 + 5 + 0 = 5) and divide by 3 to get an average of 5/3, which is approximately 1.67. Similarly, we add up the y-coordinates (0 + 0 + 7 = 7) and divide by 3 to get an average of 7/3, which is approximately 2.33. These values represent the x-coordinate (¯x) and the y-coordinate (¯y) of the centroid, respectively. Therefore, the centroid of the triangle is located at approximately (1.67, 2.33).
Learn more about triangles here
brainly.com/question/14366937
#SPJ11
A poll estimates that 47% of likely voters are in favor of additional restrictions on teenage drivers. The poll has a margin of error of plus or minus 5%. Write and solve an absolute-value equation to find the minimum and maximum percent of voters that actually support the restrictions.
Answers
Answer:
5
Step-by-step explanation:
Find the value of x given that 25 x 8 = 22x
Answers
Answer:
Step-by-step explanation:
25*8=200 soooooooooo 200=22x divide 22 on both sides and you get x=100/11
Answer:
25×8=200
200÷22x
x=9,09
therefore x=9,1
All conditions are met for the hypothesis test. based on the sample proportion, the z-test statistic was calculated to be -1.98. what is the p-value for this test?
Answers
The p-value for a z-test statistic of -1.98 can be determined by finding the area under the standard normal distribution curve to the left of -1.98.
In hypothesis testing, the p-value represents the probability of obtaining a test statistic as extreme as, or more extreme than, the observed test statistic under the null hypothesis.
Given that the z-test statistic is -1.98, we want to find the probability associated with this value. To do so, we look up the corresponding area under the standard normal distribution curve.
Using statistical software or a standard normal distribution table, we can find that the area to the left of -1.98 is approximately 0.0244 (or 2.44% when expressed as a percentage).
However, since the z-test statistic is negative, we are interested in the left tail of the distribution. To find the p-value, we consider the area in the left tail, which is 0.0244.
Therefore, the p-value for this test is approximately 0.0244. This means that if the null hypothesis is true, there is approximately a 2.44% chance of observing a test statistic as extreme as -1.98 or more extreme.
It is worth noting that the p-value should be compared to the predetermined significance level (α) to make a decision about rejecting or failing to reject the null hypothesis. If the p-value is less than or equal to α, typically set at 0.05, the null hypothesis is rejected in favor of the alternative hypothesis. If the p-value is greater than α, the null hypothesis is not rejected.
Learn more about p-value here
https://brainly.com/question/17571541
#SPJ11
A forest fire leaves behind an area of grass burned in an expanding circular pattern. if the radius of the circle of burning forest can be modeled by the function r(t) = 2t+1,where t is time in minutes, build a function modeling the area burned asa function of time
Answers
To model the area burned as a function of time, we can use the formula for the area of a circle, which is A = πr^2. In this case, the radius of the circle is given by r(t) = 2t + 1, where t represents time in minutes.
To find the area burned at any given time, we substitute the expression for r(t) into the formula for the area:
A(t) = π(2t + 1)^2
Now, let's simplify this equation step by step:
1. Expand the square:
A(t) = π(4t^2 + 4t + 1)
2. Distribute π to each term inside the parentheses:
A(t) = 4πt^2 + 4πt + π
So, the function modeling the area burned as a function of time is A(t) = 4πt^2 + 4πt + π.
We are given the radius of the circle of burning forest as r(t) = 2t + 1, where t is the time in minutes. To find the area burned, we need to use the formula for the area of a circle, which is A = πr^2. By substituting the expression for r(t) into the formula, we get A(t) = π(2t + 1)^2. Simplifying further, we expand the square and distribute π to each term inside the parentheses, resulting in A(t) = 4πt^2 + 4πt + π.
The function A(t) = 4πt^2 + 4πt + π models the area burned as a function of time, where t represents the time in minutes.
To know more about area visit ;
https://brainly.com/question/30307509
#SPJ11
y+8=x/2 linear or nonlinear
Answers
source: trust me bro
answer : linear
source: trust me people
234 × 673 pls help I'm on my last remaining brain cell
Answers
Answer:
157482
Step-by-step explanation:
234 × 673 = 157482
Answer:
157,482
Step-by-step explanation:
Use a calculator.
please help its due today


Answers
The measure of the angles are: K, L, C, N, O G = -3/4, 65, 8 40.4, 89 and 44 respectively
How to find the measure of the angles?To determine K
9x + 2 = 23 + 5x -1
9x -5x = -1 +2
4x = -3Making x the subject we have
x= -3/4
To find the value of L
Let angle L be x
x + 65 = 130
x=130-65
That is x = 65
To find the m<C
4x + 7 = 3x + 15
Collecting like terms
4x - 3x = 15-7
Making x the subject of the relation we have
x=8
To find the m<N
7x + 6x - 1 = 90
13x = 89
x=89/13
x= 6.9
Substitute x = 6.9 in 6x -1
6(6.9) -1
m<L = 40.4
To find the m<O
5x - 11 = 4x +9
5x-4x = 9 +11
x=20
By substitution in 4x +9 we have
4(20) +9
80+9
m<S = 89
To find the value of the angle G
6x - 4 = 5x +4
6x - 5x = 4+4
x = 8
Therefore the m<G is
5x +4
5(8) +4
40+4 = 44 digress
Learn more about measure of angles in https://brainly.com/question/28451077
#SPJ1
Cindy is designing a rectangular fountain in the middle of a courtyard. The rest of the courtyard will be covered in stone. The part of the courtyard that will be covered in stone has an area of 246 square feet. A. What is the width of the fountain? B. What fraction of the area of the courtyard will be occupied by the fountain?
Answers
The width of the fountain will be 3 feet and the fraction of the area of the courtyard will be occupied by the fountain is 3/44
What is the area?Is the measure of the space occupied by a body bounded by an environment called perimeter, the same is expressed in units of squared side. Example: ft^2, m^2
The formula and procedure to solve this geometry exercise is:
A(rectangle) = length * width
Information about the problem:
A(stone) = 246 ft^2
A(fountain) = ?
Width = ?
Calculating the area of the fountain we get:
Area of fountain = area of the rectangle - 246
Area of fountain = (12 x 22) - 246
Area of fountain = 264 - 246
Area of fountain = 18 ft^2
Calculating the width of the fountain we have:
A(rectangle) = length * width
18 = W x 6
W = 18/6
W = 3 feet
Calculating the fraction of the area of the courtyard will be occupied by the fountain we get:
Fraction = Area of fountain / area of the rectangle
Fraction = 18 ft^2 /264 ft^2
Fraction = 18/264
Simplifying we have:
Fraction = 3/44
Learn more about area at: brainly.com/question/11957651
#SPJ4

Mariah makes $60 per day
selling bracelets, and she
already has $55 in her
account. Write a linear
equation for this situation
and calculate how much
money Marlah will have
after one week of selling
bracelets.
Answers
Answer:
don't know if this works but I'll try
Step-by-step explanation:
Y=60x+55
y is how many days and the 55 is there because that is how much mariah already has made?
Y=-x+1
Y= 2/3x-4
How many solutions does it have?
Answers
Answer:
x = -5
Y = -22/3
Step-by-step explanation:
Y= 2/3x - 4 Y = -x + 1
We put -x + 1 in for y to solve for x
-x + 1 = 2/3x - 4
-5/3x + 1 = -4
-5/3x = -5
x = -5
Now put -5 in for x and solve for y
Y= 2/3(-5) - 4
Y = -10/3 - 4
Y = -22/3
So, there are only one solution x = -5 and y = -22/3
Jake tosses a coin up in the air and lets it fall on the ground. The equation that
models the height (in feet) and time (in seconds) of the parabola is
h(t) = -16t2 + 24 + 6. Approximate the time at which the coin hits the
ground.
Answers
Answer:
\(t = 1.71825\)
Step-by-step explanation:
Given
\(h(t) = -16t^2 + 24t + 6\)
Required
When will the coin hit the ground
When the coin hits the ground, \(h(t) = 0\)
The expression \(h(t) = -16t^2 + 24t + 6\) becomes
\(0 = -16t^2 + 24t + 6\)
Multiply through by -1
\(16t^2 - 24t - 6 = 0\)
Solve using quadratic formula
\(t = \frac{-b\±\sqrt{b^2 - 4ac}}{2a}\)
Where
\(a = 16\)
\(b = -24\)
\(c = -6\)
\(t = \frac{-b\±\sqrt{b^2 - 4ac}}{2a}\)
\(t = \frac{-(-24)\±\sqrt{(-24)^2 - 4 *16 * -6}}{2 * 16}\)
\(t = \frac{24\±\sqrt{576 +384}}{32}\)
\(t = \frac{24\±\sqrt{960}}{32}\)
\(t = \frac{24\±30.984}{32}\)
Split
\(t = \frac{24+30.984}{32}\) or \(t = \frac{24-30.984}{32}\)
\(t = \frac{54.984}{32}\) or \(t = \frac{-6.984}{32}\)
\(t = 1.71825\) or \(t = -0.21825\)
But time can't be negative;
So:
Time to hit the ground is 1.71825 seconds
a scientist begins with 100 milligrams of a radioactive substance that decays exponentially. after 35 hours, 50mg of the substance remains. how many milligrams will remain after 54 hours?
Answers
34.32 milligrams of radioactive substance will remain after 54 hours .
What are functions ?Two Persian mathematicians named Al-Biruni and Sharaf al-Din al-Tusi are credited with providing the earliest known discussion of the idea of function. Functions were first used to describe the desired connection between two varying variables.For example, time affects a planet's position. The concept was first introduced with infinitesimal calculus towards the end of the 17th century, and differentiable functions were considered up until the 19th century (that is, they had a high degree of regularity). When the concept of a function was defined in terms of set theory near the end of the 19th century, the areas in which it could be used were greatly broadened.
CalculationRemember the formula for an exponential function is represented by
\(f(x) = ab^{x}\)
where a is the initial value and bb is the growth rate.
If the base, b > 1, then it shows exponential growth. If it is 0< b < 1, then it shows exponential decay.
Using the formula for exponential function, we substitute the necessary details from the problem to get the rate first:
initial milligrams of that radioactive substances = 100
final amount of that radioactive substances = 50
number of hours = 35
decay rate can be found by =
\(50 = 100b^{35}\\ \\\frac{50}{100} = b^{35}\\ \\ b = 0.5^{\frac{1}{35} }\)
now that we use 54/35 to represent the time needed to find how much of the substance is left:
\(f(x) = 100(0.5)^{\frac{54}{35} }\)
= 100(0.3432050906)
= 34.32
learn more about functions here :
brainly.com/question/12431044
#SPJ4
If you have 66 data observations in a sample, how many classes (bins) does the sturges' rule recommend for you to construct a frequency distribution?
Answers
According to Sturge's rule, number of classes or bins recommended to construct a frequency distribution is k ≈ 7
Sturge's Rule: There are no hard and fast guidelines for the size of a class interval or bin when building a frequency distribution table. However, Sturge's rule offers advice on how many intervals one can make if one is genuinely unable to choose a class width. Sturge's rule advises that the class interval number be for a set of n observations.
Given,
n = 66
We know that,
According to Sturge's rule, the optimal number of class intervals can be determined by using the equation:
\(k=1+log_{2} n\)
Here, n is equal to 66 and by substituting the value to the equation we get:
\(k=1+log_{2} (66)\)
k = 7.0444
k ≈ 7
Learn more about Sturge's rule here: https://brainly.com/question/28184369
#SPJ4
please help me with this problem !

Answers
Answer:
a you cant add if they are not the same denominator
Answer:
Finding the least common denominator\(Answer: A\)
------------------------
Hope it helps...
Have a great day!!