Darius and his friends bought 3 pizzas. Each pizza cost $9.89. They paid for the pizzas with 20 dollar bills.
How much change did they get?
Enter your answer in the box.
$|__|
Answers
Answer:
10.33
Step-by-step explanation:
9.89x3$29.67
40-29.67=$10.33
40-29.67= $10.33 is your answer
Related Questions
I need to know how to solve for question number 4 please?

Answers
= 4
This is the graph

Determine the direction angle (in degrees) for each vector: . Make sure you're using degrees instead of radians. • If you use a decimal approximation, you must be accurate to at least 3 decimal places. a. (5,2) has direction angle: 0: 21.801 b. (-2, 11) has direction angle: 101.31 c. (7,-3) has direction angle: d. (-8, -14) has direction angle: 0 60.26 Hint: Find the magnitude and the direction angle in degrees for: Magnitude: |||| = Direction angle: v = (-8√3,-8) Compute the sum: Hint: n=1 1 n+7
Answers
The given vectors are:(5,2), (-2,11), (7,-3), and (-8,-14).
The direction angle of a vector is the angle between the vector and the positive x-axis measured counterclockwise. Therefore, the direction angle of vector v = (x,y) is given by θ = tan⁻¹(y/x).a. For vector (5,2), direction angle is given by:θ = tan⁻¹(2/5) = 21.801 degrees (rounded to 3 decimal places)b.
For vector (-2,11), direction angle is given by:θ = tan⁻¹(11/-2) = 101.31 degrees (rounded to 3 decimal places)c. For vector (7,-3), direction angle is given by:θ = tan⁻¹(-3/7) = -23.198 degrees (rounded to 3 decimal places)Note that the direction angle here is negative because the vector points towards the negative x-axis.d.
For vector (-8,-14), direction angle is given by:θ = tan⁻¹(-14/-8) = 60.26 degrees (rounded to 3 decimal places)
To know more about vectors visit:-
https://brainly.com/question/29274141
#SPJ11
suppose sat math scores are normally distributed with a mean of 516 and a standard deviation of 115 while act math scores have a normal distribution with a mean of 22 and a standard deviation of 5. anna scored 650 on the sat math and bob scored 29 on the act math. assuming that both tests measure the same kind of ability, who did better in terms of the standardized z-score?
Answers
By assuming that both tests measure the same kind of ability, Bob did better in terms of the standardized Z-score.
We have provide a data about two major math's tests.
First Major test : SAT math score normally distributed with
Mean , μ = 516 and
Standard deviations, σ = 115
Second Major test: ACT math score normally distributed with
Mean, μ = 22
Standard deviations, σ = 5
Anna scores in SAT test 650
i.e x = 650
Using Z-Score formula,
Z = (x - μ)/σ
Z = (650 - 516)/115 = 154/115 = 1.17
Also, Bob score on ACT math test is 29 i.e x = 29
Z-Score = (x - μ)/σ
=> Z = (29 - 22)/5 = 7/5
=> Z = 1.4
Since, Z-Score for Bob's ACT math test is high as compared to Anna's SAT math test.
So, Bob did better in terms of the standardized z-score.
To learn more about Z-Score formula, refer:
https://brainly.com/question/28016497
#SPJ4
Zohar is using scissors to cut a rectangle with a length of 5x – 2 and a width of 3x 1 out of a larger piece of paper. which expression can be used to find the perimeter of the rectangle and what is the perimeter if x = 4? (5x−2) (3x 1); 31 centimeters (5x−2) (3x 1); 36 centimeters 2(5x−2) 2(3x 1); 62 centimeters 2(5x−2) 2(3x 1); 70 centimeters
Answers
The expression that can be used to find the perimeter of the rectangle is 62 centimeters 2(5x−2)+2(3x+1).
What is the perimeter of the rectangle?The perimeter of the rectangle is equal to the 2 times sum of the length and width of the rectangle.
Zohar is using scissors to cut a rectangle with a length of 5x – 2 and a width of 3x + 1 out of a larger piece of paper.
The expression that can be used to find the perimeter of the rectangle is given by;
\(\rm Perimeter \ of \ rectangle = 2 (length +width)\\\\\rm Perimeter \ of \ rectangle = 2 (5x-2+3x+1)\\\\ \rm Perimeter \ of \ rectangle = 2 (7x-1)\\\\ \rm Perimeter \ of \ rectangle = 16x-2\\\\When , x\ =\ 4\\\\ \rm Perimeter \ of \ rectangle = 14x-2\ = 16(4)-2\ =\ 64-2\ =\ 62\\\)
Hence, the expression that can be used to find the perimeter of the rectangle is 62 centimeters 2(5x−2)+2(3x+1).
To know more about the perimeter of the rectangle click the link given below.
https://brainly.com/question/11935054
What is the rule for the nth term of the arithmatic sequence given below:
5/2, 4, 11/2, 7,..……
Answers
Answer:
an = 5/2 +3/2(n -1)
Step-by-step explanation:
The common difference is ...
4 -5/2 = 3/2
The general rule is ...
an = a1 +d(n -1)
For a1 = 5/2 and d = 3/2, the rule for this sequence is ...
an = 5/2 +3/2(n -1)
___
This can be simplified to ...
an = 1 +3/2n
help on math! thanks!

Answers
Answer:
A
Step-by-step explanation:
covert to slope intercept form
x-y=2
-y=-x+2
y=x-2
Answer: C
Step-by-step explanation:
In a class of students, the following data table summarizes how many students play
an instrument or a sport. What is the probability that a student chosen randomly
from the class plays a sport?
Plays a sport
Does not play a sport
Plays an instrument Does not play an instrument
3
8
10
9
Answers
Answer:
3 OR 8 hope this helps!
Step-by-step explanation:
1. The amount paid for different weights of oranges at a farmer’s market are shown below. Oranges (lb) Price ($) 2 3 3 4.50 5 7.50 ? 12 (a) Is the relationship between the price and the weight of the oranges proportional? Use the first three rows of data to answer this question. Show your work and explain. How many pounds of oranges cost $12? Show your work.
due today.
Answers
Answer:
Relationship is proportional ;
8 oranges
Step-by-step explanation:
Oranges (lb) 2 ___ 3 ____5 ___?
Price ($) __ 3 ___4.50___7.50_12
For proportionality :
Oranges = k * price
Where, k = constant of proportionality
2 = 3k
k = 2 /3
k = 0.666666
Using k :
Number of oranges at price = 4.50 should be:
0.66666 * 4.50 = 3
Number of oranges at price = 7.50 should be:
0.66666 * 7.50 = 5
Hence, relationship is proportional
Number of oranges at $12
0.66666 * 12 = 8
9. (09.01 LC) The graphs of f(x) and g(x) are shown below: If f(x) = (x + 7)^2, which of the following is g(x) based on the translation? (5 points)
g(x) = (x + 9)^2
g(x) = (x + 5)^2
g(x) = (x − 9)^2
g(x) = (x − 5)^2
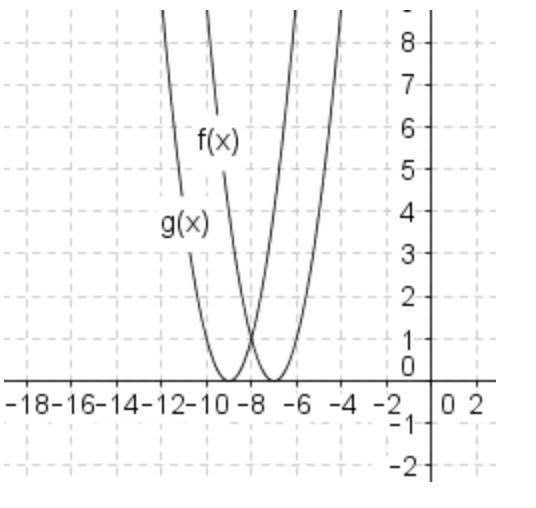
Answers
Hey there! :)
Answer:
g(x) = (x + 9)²
Step-by-step explanation:
If we look at the graph g(x), we can see that its vertex is at (-9, 0).
Recall the transformation form of a parabola is f(x) = ±a(b(x-h))+k where (h , k) are the coordinates of the vertex. In this instance it is (-9, 0), therefore:
g(x) = (x + 9)²
Suppose you have a gambling game that costs two dollars to play. You can win 1 dollar with probability 0.15, and 2 dollars with probability 0.05 and 20 dollars with probability 0.01. What is the expected net gain from playing this game? Document any computations you use in the codeblock provided. If you use a computation in your answer, it must be given here: {r}
Answers
The expected net gain from playing this game is -0.35 dollars. To calculate the expected net gain, we need to multiply each possible outcome by its corresponding probability and sum them up.
Let's denote the winnings as X, where X = 1 represents winning 1 dollar, X = 2 represents winning 2 dollars, and X = 20 represents winning 20 dollars.
Expected net gain = (1 dollar * probability of winning 1 dollar) + (2 dollars * probability of winning 2 dollars) + (20 dollars * probability of winning 20 dollars) - (2 dollars, the cost to play the game)
Expected net gain = (1 * 0.15) + (2 * 0.05) + (20 * 0.01) - 2 = -0.35 dollars.
In this game, there are three possible outcomes with their respective probabilities: winning 1 dollar with probability 0.15, winning 2 dollars with probability 0.05, and winning 20 dollars with probability 0.01. These probabilities indicate the likelihood of winning each corresponding amount.
To calculate the expected net gain, we need to consider the potential winnings and the cost to play the game. The cost to play the game is a fixed amount of 2 dollars.
We calculate the expected net gain by multiplying each possible outcome by its probability and summing them up. For example, the expected gain from winning 1 dollar is (1 * 0.15) dollars, while the expected gain from winning 2 dollars is (2 * 0.05) dollars. Similarly, the expected gain from winning 20 dollars is (20 * 0.01) dollars.
To obtain the final expected net gain, we subtract the cost to play the game (2 dollars) from the sum of the expected gains. If the result is negative, it represents a net loss, while a positive result indicates a net gain.
In this case, the calculations are as follows:
Expected net gain = (1 * 0.15) + (2 * 0.05) + (20 * 0.01) - 2 = -0.35 dollars.
This means that, on average, a player can expect to lose approximately 35 cents per game when playing this particular gambling game.
Learn more about Probability here : brainly.com/question/31828911
#SPJ11
Solve a system of linear equations to find that values of x and y
m<2y m<4x m<(2x + 12) m<(y + 6)
The linear equations are 4x + ___ = 180 and 2x + ___ = 180
The solutions are x = ___ and y = ___
Answers
The solutions to the systems of equations are x = 30 and y = 54
The correct linear equation is expressed as:
4x+y+6=180 ................1
2x+2y+12=180 .................2
The equations can also be written as:
4x + y = 174 .................... 3 × 2
2x + 2y = 168 ................... 4 × 1
______________________________________
8x + 2y = 348
2x + 2y = 168
Subtract both equations
8x - 2x = 348 - 168
6x = 180
x = 180/6
x = 30
Substitute x = 30 into equation 3:
4x + y = 174
4(30) + y = 174
120 + y = 174
y = 174 - 120
y = 54
Therefore the solutions are x = 30 and y = 54
Learn more here: https://brainly.com/question/15165519
Question 3
Solve the linear equation: 2(x - 3) = 12
A
x= 15/2
B
x = 9
с
X=-9
D
x= -15/2
Answer
Answers
+6 +6
2x=18
/2. /2
X=9
The answer is B
Answer:
The answer is B
Step-by-step explanation:
By BODMAS;
2(9 - 3) = 12
2(6) = 12
12 = 12
Evaluate yz + x² x=3.2, y=6.1, z=0.2
Answers
Answer:
Step-by-step explanation:
To evaluate the given expression, we need to substitute the given values for x, y, and z. The expression becomes:
yz + x²
Substituting the given values, we get:
(6.1 * 0.2) + (3.2^2)
This simplifies to:
1.22 + 10.24
Therefore, the value of the expression is approximately 11.46.
11.46
gimme brainlyest gang
A person draws a card from a hat. each card is one color, with the following probabilities of being drawn: 1/25 for white, 1/5 for black, 1/10 for red, and 1/15 for pink. what is the probability of pulling a black or red card, written as a reduced fraction?
Answers
The probability of pulling a black or red card = 3/10
We are informed that a card is pulled from a hat.Probability that a white card will be drawn, P(white) = 1/25
Probability that a blue card will be dealt, P(black) = 1/5
Probability that a black card will be dealt, P(red) = 1/10
Probability that a pink card will be revealed, P(pink) = 1/15
All of these occurrences are distinct from one another and do not relate to one another.
The likelihood that any one of any two events, A and B, with probability P(A) and P(B), will occur is:
P( A ∪ B ) = P(A) + P(B)
Here, event A can be compared to getting a black card, and
incident B is comparable to receiving a red card.
So, P(black or red) = P(black) + P (red)
= (1/5) + (1/10)
= ( 2 + 1 )/10
= 3/10
So, the likelihood that a black or red card will be pulled from the hat:
= 3/10
To know more about Probability visit:
https://brainly.com/question/16969946
#SPJ4
I asked someone to help me earlier but class cut out before they could answer my question

Answers
Given that the boat travels upstream for 60 miles in 4 and downstream for 60 miles in 3 hours.
Let x be the rate of the boat in still water.
Let y be the rate of the boat in the current.
Downstream =x+y.
Upstream =x-y.
Using the speed formula for downstream, we get
\(3(x+y)=60\)Dividing both sides by 3, we get
\(\frac{3\mleft(x+y\mright)}{3}=\frac{60}{3}\)\(x+y=20\)\(x=20-y\)Using the speed formula for upstream, we get
\(4(x-y)=60\)Dividing both sides by 4, we get
\(\frac{4\mleft(x-y\mright)}{4}=\frac{60}{4}\)\(x-y=15\)Substitute x=20-y to compute y value, we get
\(20-y-y=15\)\(-2y=15-20\)\(-y=\frac{-5}{2}=-2.5\)\(y=2.5\)Substitute y=2.5 in x=20-y, we get
\(x=20-2.5=17.5\)Hence the rate of the boat in still water is 17.5 mph and the rate of the boat in the current is 2.5 mph.
What is the slope of the line shown?

Answers
Answer:
\( \frac{1}{2} \)
Step-by-step explanation:
Given line is passing through the points (8, 0) & (0, - 4)
\(slope \: of \: line = \frac{ - 4 - 0}{0 - 8} = \frac{ - 4}{ - 8} = \frac{1}{2} \\ \)
what is the answer to number 2 at the top?

Answers
The equation of the transformed exponential function g(x) is g(x) = 2^-x - 1
Writing an exponential function for the graph of g(x)From the question, we have the following parameters that can be used in our computation:
Parent function: y = 2^x
The graph of the transformed exponential function g(x) passes through the points (-2,3), (-1,1), (0,0), (1,-0.5) and (2, -0.75)
So, we have the following transformation steps:
1st Transformation:
Reflect y = 2^x across the y-axis
So, we have
y = 2^-x
2nd Transformation:
Translate y = 2^-x down by 1 unit
So, we have
y = 2^-x - 1
This means that
g(x) = 2^-x - 1
Hence, the equation of the function g(x) is g(x) = 2^-x - 1
Read more about transformation at
brainly.com/question/1548871
#SPJ1
a student has scores of 84%, 71%, 86%, and 73% on four exams. what grade does the student need on the next exam to have an overall mean of 80%?
Answers
Answer:
Step-by-step explanation:
So, first you add the four exam percentage which will be 314 then you multiply 80 and 5. We use 5 because there are total 5 exams which will give the mean of 80%. After you get the answer for 80x5 which will be 400. Then you do, 400 - 314 which will be 86. So, the answer is 86%. To figure out if that answer is right, You add all the scores percentage and divide that by 5 and you should get 80%.
To have an overall mean of 80% after five exams, the student needs to score 88% on the next exam.
To find the student's overall mean, we need to add up all the scores and divide by the number of exams.
We have 4 exams scores:84%, 71%, 86%, and 73%.
Therefore, the total of the four exam scores is:
84% + 71% + 86% + 73% = 314%
To find the overall mean after five exams, the total score after five exams should be 5 × 80% = 400%.
Therefore, to have an overall mean of 80%, the student must score (400 - 314)% on the fifth exam, which is equivalent to: 86%.
Hence, the student needs to score 86% on the next exam to have an overall mean of 80%.
To learn more about Mean visit:
https://brainly.in/question/15476192
#SPJ1
rewrite z 2 −2 z 4 y 2 z 2−x/2 0 dz dx dy in dx dz dy order.
Answers
We can rewrite the given expression in the desired order as follows:
∫∫∫ z^2 - 2z^(4y^2) * (z^(-x/2)) dz dx dy
First, we integrate with respect to dz from 0 to z^(x/2):
∫∫ z^(2-x/2) - 2z^(4y^2 - x/2 + 1) / (4y^2 - x/2 + 1) dz dx
Next, we integrate with respect to dx from 0 to 1:
∫ z^(2-x/2) / (2-x/2) - 2z^(4y^2 - x/2 + 1) / (4y^2 - x/2 + 1) | 0 to 1 dy
Finally, we integrate with respect to dy from 0 to 1:
∫[0,1] ∫[0,1] ∫[0,1] z^(2-x/2) / (2-x/2) - 2z^(4y^2 - x/2 + 1) / (4y^2 - x/2 + 1) dz dx dy
This is the same expression as the original one, but written in the desired order of integration.
To know more about integration refer here:
https://brainly.com/question/31744185?#
SPJ11
Suppose that a basketball player has the ABILITY to make 60% of his free-throw attempts.
The following dotplots show the proportion of made free-throws in 100 sets of 30 attempts
and in 100 sets of 300 attempts. Which distribution is the one showing the results of 100 sets
of 30 attempts? Explain
Answers
Answer:
the sampling distribution of proportions
A sample is a small group of observations which is a subset of a larger population containing the entire set of observations. The proportion of success or measure of a certain statistic from the sample, (in the scenario above, the proportion of obese observations on our sample) gives us the sample proportion. Repeated measurement of the sample proportion of this sample whose size is large enough (usually greater Than 30) in other to obtain a range of different proportions for the sample is called the sampling distribution of proportion. Hence, creating a visual plot such as a dot plot of these repeated measurement of the proportion of obese observations gives the sampling distribution of proportions
Step-by-step explanation:
Hope this helps:)
find the area of the shaded region.
round to the nearest tenth.

Answers
The area of the shaded region is 294.4 square meters
How to find the area of the shaded region?The given parameters are represented on the figure
By this;
The area of the shaded region is the sum of the area of the major sector and the area of the triangle
The area of the major sector is
A1= ∅/360 * πr²
The area of the triangle is
A2 = 0.5r²sin(x)
So, the total area is
Total = ∅/360 * πr² + 0.5r²sin(x)
This gives
Total = (360 - 130)/360 * 3.14 * 11.1^2 + 0.5 * 11.1^2 * sin(130 deg)
Evaluate
Total = 294.4
Hence, the area of the shaded region is 294.4 square meters
Read more about areas at:
https://brainly.com/question/22972014
#SPJ1
Pls help my teacher is gonna kill me

Answers
Answer:
5³
Step-by-step explanation:
kira rides = 5 miles
cory rides = 5 * 5
tina rides : 5 * 5 * 5
therefore tina rides = 5³
Answer:
The answer would be 5 with 3.
Step-by-step explanation:
A pool measuring 18 meters by 20 meters is surrounded by a path of uniform width, as shown in the figure. If the area of the pool and the
path combined is 1520 square meters, what is the width of the path?
18
20
20+ 2x
18+2x

Answers
By solving a quadratic equation we can see that the width of the path measures 20 meters.
How to find the width of the path?
We know that the pool measures 18 meters by 20 meters, then the area of the pool alone is:
A = 18m*20m = 360 m^2
Now, if the path has a width x, then the rectangle that includes the path and the pool has dimensions:
(18m + 2x) and (20m + 2x)
And its area is given by:
(18m + 2x)*(20m + 2x)
And we know it is equal to 1520 m^2, then (i'm not writting the units in the computation):
(18 + 2x)*(20 + 2x) = 1520
360 + 4x^2 + 76x = 1520
Now we just need to solve that quadratic equation:
4x^2 + 76x - 1520 + 360 = 0
4x^2 + 76x - 1160 = 0
The solutions are:
x = (-76 ± √(76^2 - 4*4*(-1160))/(2*4)
x = (-76 ± 156)/4
We only care for the positive solution, which gives:
x = (-76 + 156)/4 = 20
We conclude that the width of the path measures 20 meters.
If you want to learn more about quadratic equations:
https://brainly.com/question/1214333
#SPJ1
A computer processes tasks in the order they are received. Each task takes an Exponential amount of time with the average of 2 minutes. Compute the probability that a package of 5 tasks is processed in less than 8 minutes.
Answers
The probability that a package of 5 tasks is processed in less than 8 minutes is 0.963.
Let X denote the time required to process a package of five tasks. X is an exponentially distributed random variable with mean 2 minutes.
The probability of X being less than 8 minutes is given by:
P(X ≤ 8) = 1 - P(X > 8)
= \(1 - (1 - e^{(-8/2)}^{5}\)
= 0.963
Therefore, the probability that a package of 5 tasks is processed in less than 8 minutes is 0.963.
To learn more about the probability visit:
https://brainly.com/question/11234923.
#SPJ4
A hypothesis can be differentiated from a theory because it is...
a. a specific prediction arising from the theory. c. it talks about how one specific variable affects d. all of the above.
Answers
A hypothesis can be differentiated from a theory because it is a specific prediction arising from the theory. The statement that is true is, a hypothesis can be differentiated from a theory because it is a specific prediction arising from the theory. A hypothesis is a suggested explanation of a phenomenon or observed data that is testable.
Hypotheses are more detailed, and are written to explain precisely what you think is going to occur in your research and the reason for that prediction. A hypothesis will be rejected if it does not fit the data, whereas a theory will be modified to fit the data.
A hypothesis is more like a forecast, and a theory is more like a law that explains how certain events operate. Thus, we can conclude that a hypothesis is a specific prediction arising from the theory.
Learn more about hypothesis from the given link
https://brainly.com/question/32562440
#SPJ11
if you know this Pls help me!!

Answers
Answer:
The graph in the lower left corner.
Step-by-step explanation:
The graph of \(y=2^x\) is in the upper right-hand corner.
The graph of \(y=-2^x\) (reflected about the x-axis) is in the upper left-hand corner.
The graph of \(y=-\frac{1}{2}(2^x)\) is a vertical compression of \(y=-2^x\), the points are half as far away from the x-axis -- squeezed closer to the x-axis by half.
If f(x) = x + 4 and g(x) = x^2-1, what is
(gºf)(x)?
Answers
Answer:
\(x^2+8x+15\)
Step-by-step explanation:
\(f(x)=x+4 \\\\g(x)=x^2-1 \\\\(g\circ f)(x)=(x+4)^2-1=\\\\x^2+8x+16-1=\\\\x^2+8x+15\)
Hope this helps!
Answer:
x² + 8x + 15
Step-by-step explanation:
(gºf)(x) = g((f(x)) = (x+4)² - 1 = x² + 8x + 16 - 1 = x² + 8x + 15
Suppose that A and B are two independent events for which P(A) = 0.31 and P(B) = 0.76. What is the probability of (A|B), (B|A), (A and B), and (A or B)?
Answers
The probability of (A and B) is 0.2356, the conditional probability of (A|B) is 0.31, the conditional probability of (B|A) is 0.76, and the probability of (A or B) is 0.8144.
Probability is a measure of the likelihood of a particular event occurring. It is expressed as a number between 0 and 1, where 0 indicates that an event is impossible and 1 indicates that an event is certain to occur.
When events A and B are independent, the probability of them occurring together is the product of their individual probabilities.
P(A and B) = P(A) * P(B) = 0.31 * 0.76 = 0.2356
P(A|B) = P(A and B) / P(B) = 0.2356 / 0.76 = 0.31 (It is same as P(A) because events A and B are independent)
P(B|A) = P(A and B) / P(A) = 0.2356 / 0.31 = 0.76 (It is same as P(B) because events A and B are independent)
P(A or B) = P(A) + P(B) - P(A and B) = 0.31 + 0.76 - 0.2356 = 0.8144
Therefore, the probability of (A and B) is 0.2356, the conditional probability of (A|B) is 0.31, the conditional probability of (B|A) is 0.76, and the probability of (A or B) is 0.8144.
To know more about probability refer to:
brainly.com/question/11234923
#SPJ4
Point M is the midpoint of AB. The coordinates of point A are (-6, 3) and the coordinates of M are -2,2). What are the coordinates of polnt B?
Answers
Answer:
Step-by-step explanation:
(x - 6)/2= -2
x - 6 = -4
x = 2
(y + 3)/2 = 2
y + 3 = 4
y = 1
(2, 1) coordinates of B
What are the x- and y-intercepts of the graph of 3x - 4y = 9?
Answers
Answer:
The X and Y intercepts and the Slope are called the line properties. We shall now graph the line -3x-4y-9 = 0 and calculate its properties. Graph of a Straight Line : Calculate the Y-Intercept : Notice that when x = 0 the value of y is 9/-4 so this line "cuts" the y axis at y=-2.25000. y-intercept = 9/-4 = -2.25000.
Step-by-step explanation:
Find the X and Y Intercepts 3x-4y=9. 3x − 4y = 9 3 x - 4 y = 9. Find the x-intercepts. Tap for more steps... To find the x-intercept (s), substitute in 0 0 for y y and solve for x x. 3 x − 4 ⋅ 0 = 9 3 x - 4 ⋅ 0 = 9. Solve the equation. Tap for more steps... Simplify 3 x − 4 ⋅ 0 3 x - 4 ⋅ 0.