Convert 0.1lb/day to oz/wk
Answers
Answer:
1 pound (mass) per day (lb/d) of mass flow=112.00 ounces (mass) per week (oz/wk) in mass flow
Step-by-step explanation:
Related Questions
by rounding each number to 2 significant figures find and approximate answer to 5998 divide 199
Answers
Answer:
I got 30.14
Step-by-step explanation:
i need help ASAPPPPPPPPPPPP

Answers
Answer:
k = 13
Step-by-step explanation:
1. A straight angle is equal to 180 degrees, and a right angle is 90 degrees. With this information and the angles of (4k-7) and (3k+6), we can build an equation of:
\(4k-7+90+3k+6=180\)2. (Solving)
Step 1: Combine like terms.
\(4k - 7 + 90 + 3k + 6 = 180\) \((4k+3k) + (-7+90+6) = 180\) \(7k + 89 = 180\)Step 2: Subtract 89 from both sides.
\(7k + 89 - 89 = 180 - 89\) \(7k = 91\)Step 3: Divide both sides by 7.
\(\frac{7k}{7} = \frac{91}{7}\) \(k = 13\)please help it’s due tomorrow
[x + y = -4
[x - y = 2
Answers
Answer:
which one substitution or elimination
Step-by-step explanation:
Substitution: (-1,-3 )
Elimination: (-1,-3)
i hope this helps you :)
Please mark me brainlest
How can you compare data sets?
Answers
When you compare two or more data sets, focus on four features:
Center. Graphically, the center of a distribution is the point where about half of the observations are on either side.
Spread. The spread of a distribution refers to the variability of the data. ...
Shape. ...
Unusual features.
Answer:
Common graphical displays (e.g., dotplots, boxplots, stemplots, bar charts) can be effective tools for comparing data from two or more data sets.
Step-by-step explanation:
MARK ME AS A BRAINLIST PLZ
hi please help......

Answers
Answer:
9/20
Step-by-step explanation:
45%=45/100
hope this helps
What is the answer pleaseee

Answers
The cross-sectional area of the cylinder with a base diameter of 44cm is approximately 1520.5 cm².
What is the cross-sectional area of the cylinder?A cylinder is simply a 3-dimensional shape having two parallel circular bases joined by a curved surface.
The cross-sectional area of a cylinder is expressed as;
Cross-sectional area A = πr²
From the diagram:
The dameter of the base of the base of the cylinder is 44cm.
We can determine the radius by dividing the diameter by 2:
Radius r = 44 cm / 2
Radius r = 22 cm
Now, plug the value of the radius into the formula to find the cross-sectional area:
Cross-sectional area A = πr²
Cross-sectional area A = π(22 cm)²
Cross-sectional area A = 484π cm²
Cross-sectional area = 1520.5 cm²
Therefore, the cross-sectional area is approximately 1520.5 cm².
Learn about volume of cylinder here: brainly.com/question/16788902
#SPJ1
f(x)=2x1 + 16x2 + 7x3 + 4x4 -> min
Answers
Step-by-step explanation:
f(x)=(2x-1)square=0
it can be 0 or greater than 0
Hence,maximum value of (2x- 1)square=0
maximum value of (2x- 1square)+3=0+3=3
10m 12m 4m7m 4m irregular figures
Answers
Notice that the figure resembles a rectangle with a missing rectangular section.
Since the length of the top part of the rectangle is 10m long, while the bottom lines are 4m each, then the combined length of those two parts is 8m. Then, the width of the missing rectangular section must be 2m long:
Then, to find the total area of the irregular figure, find the area of the greater rectangle and then substract the area of the small, missing rectangle.
The area of the greater rectangle is:
\(10m\times12m=120m^2\)The area of the smaller rectangle is:
\(2m\times7m=14m^2\)Then, the area of the irregular figure, is:
\(120m^2-14m^2=106m^2\)Therefore, the area of the given figure is:
\(106m^2\)
Jalen lost 12 pounds in the first 3 weeks of his diet. After this point, his weight loss rate slowed by half. If he lost a total of 84 pounds, how many weeks did it take him? lbs 100
Answers
Answer:
Step-by-step explanation:
Jalen lost 12 pounds in the first 3 weeks
12/3=4 per week
than the weight loss slowed by half, meaning he lost 6 pounds in 3 weeks
6/3=2 per week
84-12=72 to loose after 3 weeks
72/2=36 weeks
to loose 84 pounds you need 3+36=39 weeks
for 100 lbs
100-12=88
88/2=44
44+12=56 weeks
Mr. Norton uses a fair spinner with 12 equal regions to determine the topic for each day’s warm up lesson in math class. image Mr. Norton plans to spin the spinner 120 times during the school year. What bar graph shows the best prediction for the number of times each topic will be selected?
Answers
Mr. Norton spinning the fair spinner is an illustration of experimental probability
How to determine the bar graph?From the complete question, we have the following sections on the spinner
E = 3
N = 4
R = 3
P = 2
The probability of each section is:
P(E) = 3/12 = 25%
P(N) = 4/12 = 33.3%
P(R) = 3/12 = 25%
P(P) = 2/12 = 16.7%
When the spinner is spinned 120 times, the occurrence of each section is:
E = 25% * 120 = 30
N = 33.3% * 120 = 40
R = 25% * 120 = 30
P = 16.7% * 120 = 20
Next, we plot the appropriate bar graph
See attachment for the bar graph of the distribution
Read more about expected values at:
https://brainly.com/question/15858152

solve r/13.7 = 5 for x
please help asap
Answers
Answer: x=68.5
Step-by-step explanation: You multiply 13.7 and 5 and you should get 68.5 as x.
In the accompanying diagram, angle ACD is an exterior angle of triangle ABC, angle A =3x, angle ACD is =5x, and angle B=50. What is the value of x ?
Answers
The value of x in the triangle ABC is 25.
How to find angles in a triangle?The interior angles of the triangle are given as m∠A = 3x and m∠B = 50°.
The exterior angle of the triangle is given as m∠ACD = 5x.
The sum of angle in a triangle is equals to 180 degrees. Therefore,
3x + 50 + (180 - 5x) = 180
3x + 50 - 5x + 180 = 180
3x - 5x + 50 + 180 = 180
-2x + 230 = 180
subtract 230 from both sides
-2x + 230 - 230 = 180 - 230
-2x = -50
divide both sidesby -2
-2x / -2 = -50 / -2
x = 25
learn more on triangles here: https://brainly.com/question/27100907

Gaurav was conducting a test to determine if the average amount of medication his patients were taking was similar to the national average. He wants to use a 5% significance level for his test to help ensure that his patients do not receive too little or too much medication. If Gaurav were to conduct a test, what probability value would indicate that his null hypothesis (that there is no significant difference between the amount of medication Gaurav's patients are receiving and the national average) would be rejected?
Answers
A probability value equal to or smaller than 0.05 would indicate that Gaurav's null hypothesis should be rejected at the 5% significance level.
In hypothesis testing, the significance level, denoted as alpha (α), is the predetermined threshold used to determine whether to reject the null hypothesis.
Gaurav has specified a 5% significance level, which means he wants to control the probability of making a Type I error (rejecting the null hypothesis when it is true) at 5% or less.
If Gaurav were to conduct a test and calculate the p-value, he would compare it to the significance level of 0.05.
The p-value is the probability of observing a test statistic as extreme as the one obtained, assuming the null hypothesis is true.
If the p-value is less than or equal to the significance level (p ≤ α), it indicates that the observed difference is unlikely to occur by chance alone under the assumption of the null hypothesis.
Gaurav would reject the null hypothesis and conclude that there is a significant difference between the average amount of medication his patients are taking and the national average.
Conversely, if the p-value is greater than the significance level (p > α), it suggests that the observed difference could reasonably occur by chance, and Gaurav would fail to reject the null hypothesis.
This would imply that there is no significant difference between the average medication amounts of Gaurav's patients and the national average.
For similar questions on probability
https://brainly.com/question/25870256
#SPJ11
The cat weighs 7 3/4 pounds.The cats weight is 2/3 the weight of the dog.How much does the dog weigh!
Answers
Answer:
11.625 pounds
Step-by-step explanation:
x = weight of dog
7 3/4 = 2/3(x)
7 3/4 divided by 2/3 = x
x = 11.625 pounds
Answer:
11 5/8
Step-by-step explanation:
let 'd' = dogs weight
7 3/4 equals 2/3d
change 7 3/4 to an improper fraction: 31/4
to isolate 'd', each side needs to be multiplied by 3/2
31/4 × 3/2 = d
93/8 = d
11 5/8 equals dogs weight
Question 2(Multiple Choice Worth 5 points)
(06.03 MC)
What are the solutions of the system of equations y = -(x + 2)² + 1 and y = 3x + 7?
O(-2, 1) and (5,-8)
O(-2, 1) and (-5, -8)
O (2, -3) and (-5, -8)
O(-2, -3) and (-5, -8)
Answers
Step by step solution
Image below

The median weekly income for a student who drops out of high school is 451. Someone with a bachelor's degree from college earns 1053 in that same week. Calculate each person's yearly income and then the difference between them.
Answers
The difference between their yearly incomes is $31,304.
To calculate each person's yearly income, we need to multiply their weekly income by the number of weeks in a year. Assuming there are 52 weeks in a year, the yearly income can be calculated as follows:
For the student who drops out of high school:
Yearly Income = Weekly Income x Number of Weeks
= 451 x 52
= 23,452
For someone with a bachelor's degree:
Yearly Income = Weekly Income x Number of Weeks
= 1053 x 52
= 54,756
The difference between their yearly incomes can be found by subtracting the student's yearly income from the bachelor's degree holder's yearly income:
Difference = Bachelor's Yearly Income - Student's Yearly Income
= 54,756 - 23,452
= 31,304
Therefore, the difference between their yearly incomes is $31,304.
It is important to note that these calculations are based on the given information and assumptions. The actual yearly incomes may vary depending on factors such as work hours, additional income sources, deductions, and other financial considerations.
Additionally, it is worth considering that educational attainment is just one factor that can influence income, and there are other variables such as experience, job type, and market conditions that may also impact individuals' earnings.
For more such answers on incomes
https://brainly.com/question/28414951
#SPJ8
What value of x makes the equation true? 6 6x + 8 = 3x - 19 : A. X=-9 B. -- 3 C. = -3 D. x= 3
Answers
Answer:
A. x = -9
Step-by-step explanation:
6x + 8 = 3x - 19 <== subtract 3x from both sides
-3x -3x
3x + 8 = -19 <== subtract 8 from both sides
- 8 - 8
3x = -27 <== divide both sides by 3
/3 /3
x = -9
Hope this helps!
Answer:
\(\tt x=-9\)
Step-by-step explanation:
\(\tt 6x + 8 = 3x - 19\)
Subtract 8 from both sides:-
\(\tt 6x+8-8=3x-19-8\)
\(\tt 6x=3x-27\)
Subtract 3x from both sides:-
\(\tt 6x-3x=3x-27-3x\)
\(\tt 3x=-27\)
Divide both sides by 3:-
\(\tt \cfrac{3x}{3}=\cfrac{-27}{3}\)
\(\tt x=-9\)
The vaule of x that makes the equation true is A) x= -9.
I am stumped >:( ahhhhhhh
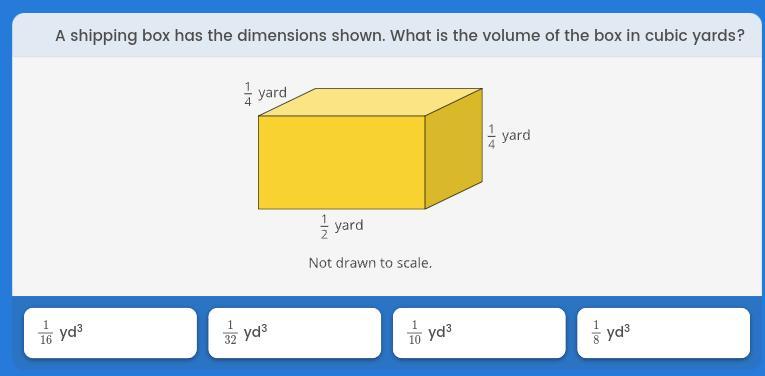
Answers
Answer:
\(\frac{1}{32} yards^{3}\)
Step-by-step explanation:
\(V=w*h*l\)
↓
\(V= \frac{1}{4} *\frac{1}{4} *\frac{1}{2}\)
=
\(\frac{1}{32} yards^{3}\\\)
Hope this helps!
Please answer this correctly without making mistakes

Answers
Answer:
1 Cup
Step-by-step explanation:
A quart is 4 cups
Answer:
The answer is one.
Step-by-step explanation:
There are four cups in a quart, so this means that in 1 cup there is \(\frac14\) quart.
A law firm is going to designate associates and partners to a big new case. The daily rate charged to the client for each associate is $600 and the daily rate for each partner is $1100. The law firm assigned a total of 10 lawyers to the case and was able to charge the client $8000 per day for these lawyers' services. Determine the number of associates assigned to the case and the number of partners assigned to the case.
There were ____associates assigned to the case and ____
partners assigned to the case.
Answers
Answer: x = 7
Thus 7 associates and 10 partners were assigned
Step-by-step explanation:
7 associates and 10 partners were assigned
Let "x" be the number of associates assigned to
case
Let "y" be the number of partners assigned to the case
The law firm assigned a total of 17 lawyers to the case
Therefore,
x + y = 17
x = 17 - y --------- eqn 1
daily rate charged to the client for each associate is $800
daily rate for each partner is $1800
They was able to charge the client $23600 per day for these lawyers' services
Therefore,
800x + 1800y = 23600 ------- eqn 2
Let us solve eqn 1 and eqn 2
Substitute eqn 1 in eqn 2
800(17 - y) + 1800y = 23600
13600 - 800y + 1800y = 23600
1000y = 23600 - 13600
1000y = 10000
Divide both sides by 1000
y = 10
Substitute y = 10 in eqn 1
x = 17 - 10
x = 7
Thus 7 associates and 10 partners were assigned
At a basketball game, for every 2 baskets team A scored, team B scored 5 baskets. The ratio of the number of baskets scored by team A to the number of baskets for team B is choices: 2 to 3, 2 to 5, 3 to 2, 5 to 2
Answers
The ratio of the number of baskets scored by team A to the number of baskets by team B is 2: 5. Then the correct option is B.
In a basketball game, team B scored 5 baskets for every 2 that team A scored.
The utilization of two or more additional numbers that compares is known as the ratio.
Assume that Team A scored two baskets while Team B netted five.
The problem statement states that for every two baskets Team A scored, Team B scored five. This may be expressed as the ratio shown below:
Ratio = 2x : 5x
Ratio = 2: 5
Thus, the correct option is B.
More about the ratio link is given below.
https://brainly.com/question/13419413
#SPJ1
An HP laser printer is advertised to print text documents at a speed of 18 ppm (pages per minute). The manufacturer tells you that the printing speed is actually a Normal random variable with a mean of 17.42 ppm and a standard deviation of 3.25 ppm. Suppose that you draw a random sample of 12 printers. Part i) Using the information about the distribution of the printing speeds given by the manufacturer, find the probability that the mean printing speed of the sample is greater than 18.12 ppm. (Please carry answers to at least six decimal places in intermediate steps. Give your final answer to the nearest three decimal places).
Answers
Answer:
0.227 = 22.7% probability that the mean printing speed of the sample is greater than 18.12 ppm.
Step-by-step explanation:
To solve this question, we need to understand the normal probability distribution and the central limit theorem.
Normal Probability Distribution:
Problems of normal distributions can be solved using the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Central Limit Theorem
The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean \(\mu\) and standard deviation \(\sigma\), the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean \(\mu\) and standard deviation \(s = \frac{\sigma}{\sqrt{n}}\).
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
Mean of 17.42 ppm and a standard deviation of 3.25 ppm.
This means that \(\mu = 17.42, \sigma = 3.25\)
Sample of 12:
This means that \(n = 12, s = \frac{3.25}{\sqrt{12}}\)
Find the probability that the mean printing speed of the sample is greater than 18.12 ppm.
This is 1 subtracted by the p-value of Z when X = 18.12.
\(Z = \frac{X - \mu}{\sigma}\)
By the Central Limit Theorem
\(Z = \frac{X - \mu}{s}\)
\(Z = \frac{18.12 - 17.42}{\frac{3.25}{\sqrt{12}}}\)
\(Z = 0.75\)
\(Z = 0.75\) has a pvalue of 0.773.
1 - 0.773 = 0.227
0.227 = 22.7% probability that the mean printing speed of the sample is greater than 18.12 ppm.
3 6 9 12 15 18 21 24 27 30 is odd or even numbers?
Answers
Answer: Half of them are even and half of them are odd.
Step-by-step explanation:
The even numbers are 6, 12, 18, 24, and 30. An even number is defined as a number that is divisible by 2, meaning it has no remainder when divided by 2. For example, 6 divided by 2 equals 3 with no remainder, so 6 is even.
The odd numbers are 3, 9, 15, 21, and 27. An odd number is defined as a number that is not divisible by 2, meaning it has a remainder of 1 when divided by 2. For example, 9 divided by 2 equals 4 with a remainder of 1, so 9 is odd.
Therefore, out of the given numbers, half of them are even and half of them are odd.
________________________________________________________
The ages of grandparents of students in Mr. Keyes' third period class are listed below.52 54 57 61 56 6167 64 63 57 60 50A. Create the five-number summary that represents the data set.B. Create a box plot that represents the data set.
Answers
Given the data set (ages of grandparents):
52, 54, 57, 61, 56, 61, 67, 64, 63, 57, 60, 50
Let's create a five-number summary that represents the given data set and also create a box plot.
A) A five number summary of a data set consists of the following:
• Minimum value
,• First quartile
,• Median
,• Third quartile
,• Maximum value
Let's determine the five-number summary of the given data set.
• Minimum value:
The minimum value is the smallest number from the given data set.
Thus, the minimum is = 50
• First quartile:
The first quartile is also called the lower quartile. It is the median of the lower half of the data set.
To find the first quartile, list out the lower half of the data set after arranging the data in acsending order.
Arrange in ascending order: 50, 52, 54, 56, 57, 57, 60, 61, 61, 63, 64, 67
Lower half: 50, 52, 54, 56, 57, 57
Median of lower half:
\(\frac{54+56}{6}=\frac{110}{2}=55\)Therefore, the first quartile is = 55
• Median:
Median is the middle term of the data set.
50, 52, 54, 56, 57, 57, 60, 61, 61, 63, 64, 67
The middle terms are = 57 and 60
To find the median, divide the sum of the middle terms by 2.
Thus, we have:
\(\frac{57+60}{2}=\frac{117}{2}=58.5\)Therefore, the median of the data set is 58.5
• Third Quartile:
The third quartile is also called the upper quartile. It is the median of the upper half of the data set.
Upper half of data set = 60, 61, 61, 63, 64, 67
Median of upper half =
\(\frac{61+63}{2}=\frac{124}{2}=62\)Therefore, the third quartile is 62
• Maximum value:
The maximum value is the greatest number in the given data set.
The greatest number in the data set is 67.
Therefore, the maximum value is 67.
We have the five-number summary that represents the data set below:
• Minimum = 50
,• First quartile = 55
,• Median = 58.5
,• Third quartile = 62
,• Maximum = 67
b) Let's create a box plot that represents the data set.
We have the box plot below:

There are 49 dogs signed up for a dog show. There are 36 more small dogs than large dogs. How many small dogs have signed up to compete?
Answers
Answer:
85 dogs have signed up to compete
Step-by-step explanation:
its 85 small dogs because 49+36=85
Find a . b.
-
a = <2,4>, b = <2, 5>
<4,9>
24
<4, 20>
16
Answers
Answer: What is this
Step-by-step explanation:
So sir or ms but um i cannot read this and i am a Harvard Graduate.
IF U GET CORRECT U GET BRAINLIEST
The last five houses built in town sold for the prices shown below. Find the mean price. Use pencil and paper. If a new house built in the town sold for $160,000, how would that affect the mean?
prices: $181,00; $187,00; $144,00; $176,00; $175,00
what is the mean price of the houses??
Answers
Answer:
172,600
Step-by-step explanation:
hii so basically the mean is the same as average so add all together and divide by how many prices in this case there originally were
so 181,000+187,000+144,000+176,000+175,000=863,000
then divide by 5
863,000/5=172,600
hope this helped !
Arrange the steps in order to simplify the expression

Answers
Answer:
Step-by-step explanation:
For step explanation:
1. write the problem
2. distinguishing the neg sign
3. distributing 3
4. moving like terms next to each other through commutative property
5. Combining like terms
6. getting rid of parentheses

The graph shows the speed of a car while it is
slowing down to a stop.
a) Using an appropriate triangle with two of its
vertices on the curve, estimate the distance
travelled by the car during this time.
b) Is your answer an underestimate or an
overestimate?
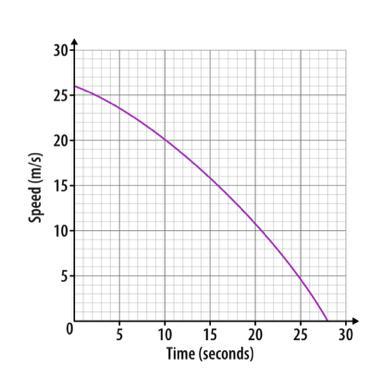
Answers
-2\tfrac{1}{2}-\Big(-4\tfrac{3}{5}\Big)
−2 /2/1 −(−4 3/5)
Answers
The value of the fraction expression -2 1/2 - (-4 3/5) is 2 1/10
How to evaluate the expressionFrom the question, we have the following parameters that can be used in our computation:
−2 /2/1 −(−4 3/5)
Express properly
So, we have the following representation
-2 1/2 - (-4 3/5)
Remove the brackets
This gives
-2 1/2 - (-4 3/5) = -2 1/2 + 4 3/5
Express the denominator as 10
So, we have
-2 1/2 - (-4 3/5) = -2 5/10 + 4 6/10
Evaluate the difference
-2 1/2 - (-4 3/5) = 2 1/10
Hence, the solution is 2 1/10
Read more about fraction at
https://brainly.com/question/1622425
#SPJ1