consider the plane curve defined by the Paramatric equations X= 7 + t sin (t) X=49+ t cos (t) Calculate the Arc length of the curve over the domain ort R 211
Answers
The arc length of the plane curve defined by the Paramatric equations X= 7 + t sin (t) X=49+ t cos (t) over the domain of R 211 is approximately 58.5.
To calculate the arc length of the given plane curve, we can use the formula:
L = ∫√(dx/dt)² + (dy/dt)² dt
where L is the arc length and dx/dt and dy/dt are the derivatives of X and Y with respect to t, respectively.
First, let's find dx/dt and dy/dt:
dx/dt = cos(t) + t cos(t)
dy/dt = -sin(t) + t sin(t)
Now, we can substitute these into the arc length formula and integrate over the given domain:
L = ∫√(cos(t) + t cos(t))² + (-sin(t) + t sin(t))² dt from 2 to 11
Simplifying the integrand:
L = ∫√(cos²(t) + 2t cos²(t) + t² cos²(t) + sin²(t) - 2t sin(t) sin(t) + t² sin²(t)) dt
L = ∫√(1 + t²) dt
Using the substitution u = 1 + t², du/dt = 2t, and dt = du/2t:
L = ∫√u * 1/(2t) du from 5 to 122
L = 1/2 ∫u^(1/2) * u^(-1/2) du from 5 to 122
L = 1/2 ∫1 du from 5 to 122
L = 1/2 * (122 - 5)
L = 58.5
Therefore, the arc length of the curve over the domain of R 211 is approximately 58.5.
More on arcs: https://brainly.com/question/15601180
#SPJ11
Related Questions
Find the area of the circle. Round your answer to the nearest hundredth. It is a whole circle with a diameter of twelve, use the formula a=pi r ^2. I'll give 45 points and brainliest quick pls I have 15 minutes
Answers
Answer: 113
Step by Step explanation:
12/2=6
inches.π⋅6^2≈113
The area of the circle with diameter 12 units is 113.04 square units.
What is Circle?A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre.
The given circle has a diameter of 12.
We have to find the area of the circle.
The formula for Area of circle=πr²
Radius of circle = Diameter/2
=12/2
Radius=6
Now let us put r value in Area of circle formula
A=3.14×6²
=3.14×36
=113.04 square units.
Hence, the area of the circle with diameter 12 units is 113.04 square units.
To learn more on Circles click:
https://brainly.com/question/11833983
#SPJ5
Graph -8 + x = 4y - 16.
Answers
If a, a²+ 1 and a+6 are in an AS, find the possible values of a.
it's of optional math. If someone know how to solve this. please help me I have been trying this for like half hour
Answers
a, a² + 1 and a + 6 are all in arithmetic progression, in which there is a fixed difference d between consecutive terms. This mean we have
a² + 1 = a + d
a + 6 = a² + 1 + d
Eliminate d and solve for a :
(a² + 1) - (a + 6) = (a + d) - (a² + 1 + d)
a² - a - 5 = -a² + a - 1
2a² - 2a - 4 = 0
a² - a - 2 = 0
(a - 2) (a + 1) = 0
a - 2 = 0 or a + 1 = 0
a = 2 or a = -1
Johnny is budgeting for his trip to the toy store. He does not want to spend more than $80 on Christmas presents. If he wants to buy a toy car that costs $32.25 and some dolls that cost $4.50 each, he can buy ______________ dolls. Use the inequality to help solve the problem.
Answers
The inequality that can be used to represent the number of doll Johnny can buy is x ≤ 10.61
How to solve inequality?Johnny is budgeting for his trip to the toy store. He does not want to spend more than $80 on Christmas presents.
He wants to buy a toy car that costs $32.25 and some dolls that cost $4.50 each. The number of dolls he can buy can be represented with the inequality as follows:
let
x = number of dolls
Therefore,
4.50x + 32.25 ≤ 80
subtract 32.25 from both sides of the inequality
4.50x + 32.25 ≤ 80
4.50x + 32.25 - 32.25 ≤ 80 - 32.25
4.50x ≤ 47.75
divide both sides by 4.50
4.50x/ 4.50 ≤ 47.75 / 4.50
x ≤ 10.6111111111
x ≤ 10.61
learn more on inequality here: https://brainly.com/question/24501286
#SPJ1
solve for R
D = RT
PLZ HELP ME
Answers
Answer:
Distance = Rate x Time
Is that what you are trying to figure out?
Answer:
R=D/T
Step-by-step explanation:
since D equals R times T, R would equal D divided by T
Expand. If necessary, combine like terms. (5x-6)^2=
Answers
Answer:
25x²-60x+36
Step-by-step explanation:
(5x-6)²
Expand the brackets.
(5x-6)(5x-6)
5x(5x-6)-6(5x-6)
Multiply.
25x²-30x-30x+36
Add or subtract the like terms.
25x²-60x+36
Perfect squares trinomials are those expressions which are found by squaring binomial expressions. The expansion of the given binomial expression, (5x - 6)² is (25x² + 36 - 60x).
What are perfect squares trinomials?Perfect squares trinomials are those expressions which are found by squaring binomial expressions. A few examples of perfect squares trinomials are,
a² + b² + 2ab = (a + b)²
a² + b² - 2ab = (a - b)²
The expansion of the given binomial expression, (5x - 6)² can be done as,
(5x - 6)²
= (5x)² + 6² - 2(5x)(6)
= 25x² + 36 - 60x
Hence, The expansion of the given binomial expression, (5x - 6)² is (25x² + 36 - 60x).
Learn more about Perfect square trinomials:
https://brainly.com/question/88561
#SPJ2
Which method would it be possible to prove the triangles below are similar (if possible)?

Answers
Answer:
It’s either SAS or AA.
Calculate the experimental probability of flipping a coin 25 times, and getting heads 8 times.
Answers
Answer:
68 percent
Step-by-step explanation:
Answer:
68%
Step-by-step explanation:
How many solutions are there to the inequality x1 + x2 + x3 ≤ 11, where x1, x2, and x3 are nonnegative integers? [Hint: Introduce an auxiliary variable x4 such that x1 + x2 + x3 + x4 = 11.]
Answers
The number of nonnegative integer solutions to the inequality x1 + x2 + x3 ≤ 11 is C(14,3) = 364.
We can solve this inequality by introducing an auxiliary variable x4, such that x1 + x2 + x3 + x4 = 11. Here, x1, x2, x3, and x4 are all nonnegative integers.
We can interpret this equation as follows: imagine we have 11 identical objects and we want to distribute them among four boxes (x1, x2, x3, and x4). Each box can contain any number of objects, including zero. The number of solutions to this equation will give us the number of nonnegative integer solutions to the original inequality.
We can use a technique known as stars and bars to count the number of solutions to this equation. Imagine we represent the 11 objects as stars: ***********.
We can then place three bars to divide the stars into four groups, each group representing one of the variables x1, x2, x3, and x4. For example, if we place the first bar after the first star, the second bar after the third star, and the third bar after the fifth star, we get the following arrangement:
| ** | * | ****
This arrangement corresponds to the solution x1=1, x2=2, x3=1, and x4=7. Notice that the number of stars to the left of the first bar gives the value of x1, the number of stars between the first and second bars gives the value of x2, and so on.
We can place the bars in any order, so we need to count the number of ways to arrange three bars among 14 positions (11 stars and 3 bars). This is equivalent to choosing 3 positions out of 14 to place the bars, which can be done in C(14,3) ways.
Therefore, the number of nonnegative integer solutions to the inequality x1 + x2 + x3 ≤ 11 is C(14,3) = 364.
Click the below link, to learn more about solutions of the inequality :
https://brainly.com/question/22010462
#SPJ11
find the 5th derivative by using calculus with full workings of 7g^8+2g^7+4g^6+3g^5+g^2+5g+276.3
Answers
This is an exercise in applying the power rule, which says
\(f(x) = x^n \implies f'(x) = nx^{n-1}\)
Starting with
\(7g^8 + 2g^7 + 4g^6 + 3g^5 + g^2 + 5g + 276.3\)
we have
• first derivative
\(56g^7 + 14g^6 + 24g^5 + 15g^4 + 2g + 5\)
• second derivative
\(392g^6 + 84g^5 + 120g^4 + 60g^3 + 2\)
• third derivative
\(2352g^5 + 420g^4 + 480g^3 + 180g^2\)
• fourth derivative
\(11760g^4 + 1680g^3 + 1440g^2 + 360g\)
• fifth derivative
\(\boxed{47040g^3 + 5040g^2 + 2880g + 360}\)
At Cheng's Bike Rentals, it costs 31 to rent a bike for 6 hours.
How many hours of bike use does a customer get per dollar?
Answers
Answer:
0.2hrs/dollars
Step-by-step explanation:
Given parameters:
Cost of bike = $31
Time duration = 6hrs
Unknown:
Hours customers get per dollar = ?
Solution:
The number of hours customer gets per dollar is;
Number of hrs/dollar = \(\frac{time duration }{cost}\)
Number of hrs/dollar = \(\frac{6hrs}{31}\)
Number of hrs/dollar = 0.2hrs/dollars
a=2x3^4x5 b=2^3x3^2x5^5 write down the night common factor of A and B PLEASE HELP ASAP PLEASEE!
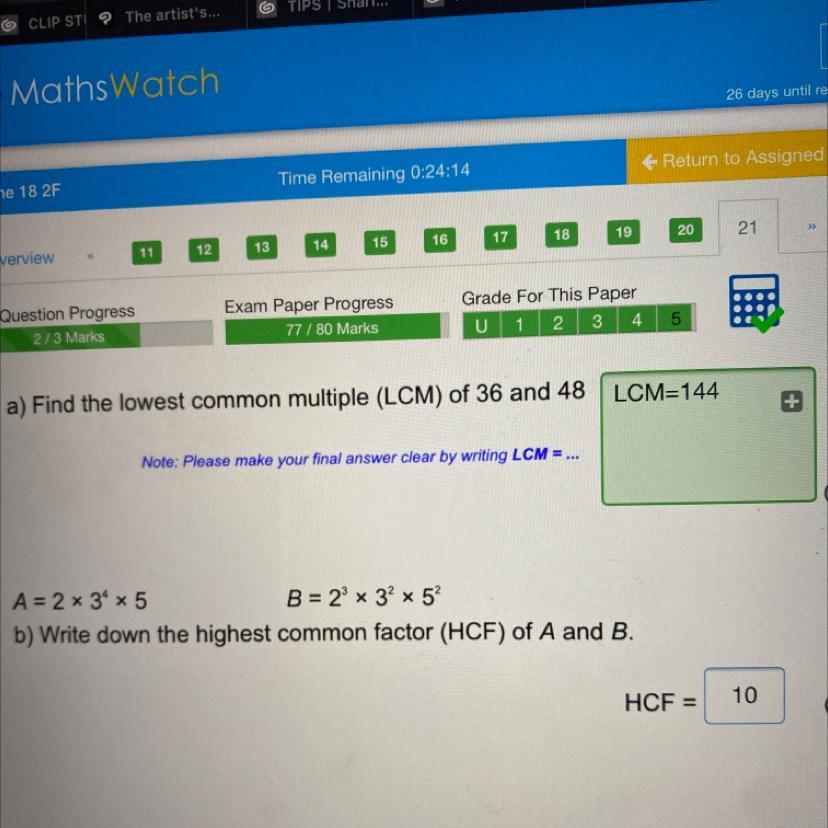
Answers
Answer:
The HCF of \(a=2^1 \cdot 3^4 \cdot 5^1\) and \(b = 2^3 \cdot 3^2 \cdot 5^2\) is \(90\).
Step-by-step explanation:
To calculate the HCF of two numbers given their prime factorization, we take the lowest exponent of every prime when comparing the two numbers.
So the HCF of \(a=2^1 \cdot 3^4 \cdot 5^1\) and \(b = 2^3 \cdot 3^2 \cdot 5^2\) is \(2^1 \cdot 3^2 \cdot 5^1 = 90\).
What would be the perimeter of this figure?

Answers
Answer:
40.9ft
Step-by-step explanation:
The perimeter is when you add all the sides together.
8 + 10 + 10 + 4 + 8.9 = 40.9ft
5. in which number is the 3-1/100 the value of the 3 in 43,781? a. 345 b. 237, 895 c. 5,732
Answers
The number in which the value of 3 is 3-1/100 is 345. The correct option is a.
The given number is 43,781.
The value of the 3 in 43,781 is 3,000.
The value of 3-1/100 is 2.99.
We are supposed to find out in which number 2.99 is the value of the 3 in 43,781.
Given options are:
a. 345
b. 237,895
c. 5,732
We'll calculate the values of 3-1/100 in each option.
a. The value of 3-1/100 in 345 is 2.99.
So, the correct option is a.
To know more about value refer here:
https://brainly.com/question/9796766
#SPJ11
As a model system he decides to study this question in a rocky intertidal zone where mussels are the dominant competitor, barnacles are the inferior competitor and the sea-star is the main predator. He hypothesizes that the sea-star prefers foraging on the bigger mussels thereby preventing the mussels from competitively excluding the barnacles. He finds two sites that appear to be similar in everything except for that in one the sea-star is present and in the other it is absent. He then compares the numbers of the two competing species between the two sites. This study design can be viewed as
Answers
This study design can be viewed as an observational comparative study.
In an observational comparative study, the researcher compares groups or conditions that already exist and observes any differences or relationships between them. In this case, the researcher is comparing two sites in a rocky intertidal zone, one with the presence of sea-stars and the other without sea-stars.
The researcher is interested in examining the impact of sea-star predation on the interactions between mussels and barnacles, the dominant and inferior competitors, respectively. By comparing the numbers of the competing species between the two sites, the researcher can analyze the potential influence of sea-star predation on the competitive exclusion of barnacles by mussels.
This study design allows for the examination of natural differences and associations without direct manipulation by the researcher.
Learn more about hypothesizes here : brainly.com/question/28331914
#SPJ11
What is the common difference in the arithmetic sequence 30, 27, 24, 21, 18,
Answers
what is this can someone tell me

Answers
Answer:
A. y+4=f(x)
Step-by-step explanation:
if you were to make this a y= equation then you would have to move the 4 to the other side by subtracting 4 from both sides therefore making the value of f(x) - 4
you should try it out in a graphic calculator like Desmos, it's an online graphic calculator
hi! please help in math!
i need the solution/explanation on how you got the answer
(y + 3) = -8(x - 4)
what is the slope?
Answers
Answer:
slope m = - 8
Step-by-step explanation:
the equation of a line in point- slope form is
y - b = m(x - a)
where m is the slope and (a, b ) a point on the line
y + 3 = - 8(x - 4) ← is in point- slope form
with slope m = - 8
The slope is :
↬ -8Solution:
Given: \(\bf{y+3=-8(x-4)}\)
To determine the slope, it's important to know the form of the equation first.
There are 3 forms that you should be familiar with.
The three forms of equations of a straight line are:
Slope Intercept (y = mx + b)Point slope (y-y₁) = m(x - x₁)Standard form (ax + by = c)This equation matches point slope perfectly.
The question becomes, how do you work with point slope to find slope?
Point slopeIn point slope, m is the slope and (x₁, y₁) is a point on the line.
Similarly, the slope of \(\bf{y+3=-8(x-4)}\) is -8.
Hence, the slope is -8.The sum of three consecutive odd integers is 153. What is the value of the largest of the integers?
Help me pls
Answers
the value of largest integer when the sum of 3 consecutive odd integers is 153 is 53.
We know that odd consecutive numbers means the difference between the numbers will be 2
So, let the first odd number be x
Second number will be = x + 2
Third number will be = x + 2 + 2 = x + 4
Now, we are given that, the sum of these odd integers is 153
So, we get the equation as:
x + x + 2 + x + 4 = 153
3 x + 6 = 153
3 x = 153 - 6
3 x = 147
x = 147 / 3
x = 49
Largest integer = x + 4 = 49 + 4 = 53
Therefore, we get that, the value of largest integer when the sum of 3 consecutive odd integers is 153 is 53.
Learn more about integers here:
https://brainly.com/question/17695139
#SPJ9
4y 8x - 16 as a function
Answers
Answer:
The function form is:
f(x) = 2x - 8
which value of n makes the equation true
\(-\frac{1}{2}n=-8\)
Answers
Answer:
Step-by-step explanation:
nothing makes it true
Find the area of the circle. Round to the nearest tenth. Use 3.14 or 22/7 for π.
Answers
The area of a circle is 158.3 sq. m.
Consider we need to find the area of the given circle.
The radius of a circle is 7.1 m.
The area of a circle is the place occupied by the circle in a two-dimensional plane. Alternatively, the space occupied within the circumference of a circle is called the area of the circle.
The area of the circle is:
\(A = \pi r^{2}\) , where r is the circle's radius.
Putting r = 7.1 , π = 3.14, we get
\(A = 3.14 *(7.1)^{2}\)
A = 158.2874
A ≈ 158.3
So, the area of a circle is 158.3 sq. m.
Read more about circle:
https://brainly.com/question/20489969
#SPJ4

Find the area of hexagon DEFGHI.

Answers
Step-by-step explanation:
Break it up into two trapezoids as shown
area = trap1 + trap2
= 2 * (7+3) / 2 + 3 * ( 7 + 3) / 2 = 10 + 15 = 25 units^2

const erit to miguel be dibiv art sis IW You deposit $3,000 in a
savings account at 6% interest. If the interest is compounded:
001 = dipnel .02 = thbiW ISVenA Yearly: Answer: $5,372.54
Answers
After one year of compounding at a 6% interest rate, you would have approximately $3,180 in your savings account.
To calculate the amount of money you will have in the savings account after one year with compound interest, we can use the formula:
A = P(1 + r/n)^(nt)
Where:
A is the final amount
P is the principal amount (initial deposit)
r is the annual interest rate (as a decimal)
n is the number of times the interest is compounded per year
t is the number of years
Let's solve it step by step:
Given:
P = $3,000 (principal amount)
r = 6% = 0.06 (annual interest rate as a decimal)
n = 1 (compounded annually)
t = 1 (one year)
Using the formula:
A = 3000 * (1 + 0.06/1)^(1*1)
A = 3000 * (1 + 0.06)^1
A = 3000 * (1.06)^1
A ≈ $3180
So, after one year of compounding at a 6% interest rate, you would have approximately $3,180 in your savings account.
It seems there was a discrepancy in the answer provided. Based on the given information, the correct calculation yields $3,180, not $5,372.54.
Learn more about interest rate from
https://brainly.com/question/25720319
#SPJ11
What is the equation of the line that passes through the point (–5,–6) and has a slope of zero?
Answers
Answer:
y = -6
Step-by-step explanation:
y = mx + b
-6 = -5(0) + b
b = -6
Find the volume for 2 different spheres by randomly choosing different radii.
Using the same radii values, find the volume of 2 cylinders where the height of the cylinder is the same as the diameter of the sphere.
Answers
Answer:
The volume of a sphere of radius r is:
S = (4/3)*pi*r^3
The volume of a cylinder of radius r and height h is:
C = pi*r^2*h
For this problem the height of the cylinders will be equal to the diameter of the spheres, which is equal to two times the radius.
First, let's use the radius: r = 2.
The volume of the sphere will be:
S = (4/3)*3.14*(2)^3 = 33.49
The volume of the cylinder, where h = 2*2 = 4, is:
C = 3.14*(2^2)*4 = 50.24
Now, let's choose the radius r = 3.
The volume of the sphere will be:
S = (4/3)*3.14*3^3 = 113.04
The volume of a cylinder with this radius and h = 3*2 = 6, is:
C = 3.14*(3^2)*6 = 169.56
Write the sample space for rolling a 6 sided die.
Answers
Answer:
Sample space = { 1, 2, 3, 4, 5, 6}
Explanation:
The sample space is the set with all the possible outcomes of the experiment. So if we roll a 6 sided die, we have the possible outcomes:
{ 1, 2, 3, 4, 5, 6}
Because we have 6 possible results. one for every side of the die
Therefore, the sample space is { 1, 2, 3, 4, 5, 6}
Please help!
Identify the slope of the function: f(x)=2(3x-7)
A:3
B:6
C:7
D:2
Answers
Answer:
The slope is 6
Step-by-step explanation:
f(x)=2(3x-7)
Distribute the 2
f(x)=2*3x-2*7
f(x) = 6x -14
This is in slope intercept form
y = mx+b where m is the slope and b is the y intercept
The slope is 6 and the y intercept is -14
The slope is 6
what was the most inaccurate version of pi? explain who, when and what the value was.
Answers
The value of pi is known to over 31 trillion decimal places, thanks to the use of powerful computers and sophisticated algorithms.
Describe about the history of pi?The history of pi dates back thousands of years, and over time, various civilizations have attempted to calculate its value with varying degrees of accuracy. One of the most inaccurate versions of pi was recorded by the ancient Babylonians around 2000 BC.
The Babylonians calculated the value of pi as 3.125, which is off by more than 6% from the actual value. It is believed that the Babylonians arrived at this value by using a rough approximation of a circle as a hexagon. They measured the perimeter of the hexagon and divided it by the diameter to get their approximation of pi.
This value was later refined by the ancient Egyptians and Greeks, who were able to calculate pi with greater accuracy. The Greek mathematician Archimedes, for instance, was able to calculate pi to within 1% accuracy by using a method of exhaustion.
It wasn't until the development of calculus in the 17th century that mathematicians were able to derive an exact formula for pi. Today, the value of pi is known to over 31 trillion decimal places, thanks to the use of powerful computers and sophisticated algorithms.
To know more about Greek symbols visit:
brainly.com/question/7269995
#SPJ1
A scientist adds drops of liquid to a test tube. The test tube has marks every ml.
Each drop contains 0.14 mL. Between which two marks on the test tube will the
liquid be after the sixth drop is added?
a. 1/5 and 2/5, between 0.2 and 0.4 mL's
b.2/5 and 3/5, between 0.4 and 0.6 ml's
c.3/5 and 4/5, between 0.6 and 0.8 mL's
d. 4/5 and 5/5, between 0.8 and 1 ml
Answers
Answer:
A scientist adds drops of liquid to a test tube. The test tube has marks every ml.
Each drop contains 0.14 mL. Between which two marks on the test tube will the
liquid be after the sixth drop is added?
a. 1/5 and 2/5, between 0.2 and 0.4 mL's
b.2/5 and 3/5, between 0.4 and 0.6 ml's
c.3/5 and 4/5, between 0.6 and 0.8 mL's
d. 4/5 and 5/5, between 0.8 and 1 ml