chegg many of a bank’s customers use its automatic teller machine to transact business after normal banking hours. during the early evening hours in the summer months, customers arrive at a certain location at the rate of one every other minute. this can be modeled using a poisson distribution. each customer spends an average of 92 seconds completing his or her transactions. transaction time is exponentially distributed.
Answers
The given scenario can be modeled using a Poisson distribution.
A Poisson distribution is commonly used to model the number of events occurring in a fixed interval of time or space, given the average rate at which those events occur. It is often used to describe rare or random events, such as the arrival of customers at a bank's automatic teller machine.
In this context, the Poisson distribution helps us understand the likelihood of a certain number of customers arriving during a specific time frame. The fact that customers arrive at a rate of one every other minute means that the average rate λ (lambda) is 0.5 customers per minute.
To calculate the probability of a specific number of customers arriving during a given time frame, we can use the Poisson probability formula:
P(x;λ) = (e^(-λ) * λ^x) / x!
Where:
- P(x;λ) represents the probability of x customers arriving during the specified time frame,
- e is the base of the natural logarithm (approximately 2.71828),
- λ is the average rate of arrival (0.5 customers per minute in this case),
- x is the number of customers we want to calculate the probability for, and
- x! denotes the factorial of x.
Furthermore, we are given that each customer spends an average of 92 seconds (or 1.53 minutes) completing their transactions. This transaction time can be modeled using an exponential distribution.
The exponential distribution is commonly used to model the time between events in a Poisson process. In this case, it helps us understand the time it takes for a customer to complete their transaction. The average transaction time is 1.53 minutes.
The exponential distribution has a probability density function (pdf) given by:
f(x;λ) = λ * e^(-λx)
Where:
- f(x;λ) represents the probability density function,
- e is the base of the natural logarithm (approximately 2.71828),
- λ is the rate parameter (the reciprocal of the average transaction time), and
- x is the time we want to calculate the probability density for.
By understanding and applying the concepts of the Poisson distribution and the exponential distribution, we can model and analyze the arrival rate of customers at the bank's automatic teller machine and the time it takes for them to complete their transactions.
More on Poisson Distribution: https://brainly.com/question/30388228
#SPJ11
Related Questions
Identify the domain and range of the function. Enter your answers as numbers in the boxes; do not include units. Enter the range values in ascending order separated by commas. Age Admission Price under 5 free 5–17 $25 18–64 $40 65 and over $35 What is the domain?
Answers
Given that:
The tables values which represents Age and Admission Price is
Age Admission Price
under 5 free
5–17 $25
18–64 $40
65 and over $35
To find:
Domain and range of the function.
Solution:
Domain is the set of input values.
Here, domain is age because age is an independent variable.
Age cannot be negative, so
\(Domain:\{Age\geq 0\}=[0,\infity)\)
Therefore, the domain is all number greater than or equal to 0.
Range is the set of input values.
Here, range is Admission Price because Admission Price is a dependent variable.
Admission Prices are free (i.e., 0), $25, $40 and $35. So
\(Range:\{0,25,35,40\}\)
Therefore, range is {0,25,35,40}.
Solve the system of equations -4x-y=18
Answers
Explanation: You need to get y by itself so you will move -4x to the other side by adding it on both side so it can cancel out on the side that it was originally which was the y side.
This will give you -y=4x+18
Now you need to make y become a positive so you will divide both side by -1 ( the 1 comes from in front of the y there is always an invisible 1 if there is no number in front of the letter (x,y,etc))
When you divide everything by -1 it will get you : y=-4x-18
Ned made the design at the right. Use a protractor. Find and write the measure of each of the 3 angles.
Answers
Using a protractor we were able to find that the measure of each angle in the design is 60 degrees, 120 degrees, and 60 degrees.
To find the measure of each angle in the design, we can use a protractor. A protractor is a tool used to measure angles, usually in degrees.
Starting with the first angle, we can place the protractor so that its baseline is aligned with one of the lines that forms the angle, and then read the degree measurement at the point where the other line intersects the protractor. We repeat this process for the other two angles.
After measuring each angle, we can see that the first angle measures 60 degrees, the second angle measures 120 degrees, and the third angle measures 60 degrees.
It's important to note that the sum of the angles in any triangle always add up to 180 degrees. So, in this design, where the three angles form a triangle, the sum of their measurements would be 60 + 120 + 60 = 240 degrees, which is not possible. This means that the design is not a triangle, and must be some other shape with straight sides.
In conclusion, using a protractor we were able to find that the measure of each angle in the design is 60 degrees, 120 degrees, and 60 degrees.
Find out more about protractor
brainly.com/question/29284105
#SPJ4
Write the expanded expression as a simplified expression with exponents:7xxyyyy=
Answers
Answer:
7x²y⁴
Explanation:
Given the expanded expression:
\(7xxyyy\)We have two x(s) and 4 y(s), thus:
\(\begin{gathered} 7xxyyyy=7\times x^2\times y^4 \\ =7x^2y^4 \end{gathered}\)please help me
the formula
A=1/2 X bX h
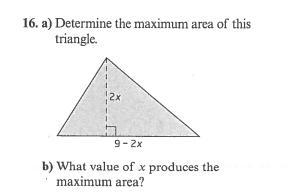
Answers
Answer:
−4 cot ( −45 )
Step-by-step explanation:
5x + 10 = 7x + 18
Answer answer answer answer answer
Answers
Answer:
My answer would most likely be x = -4.
Answer:
x = -4
Step-by-step explanation:
Solve for x.. 5x+18=45
Answers
Answer: x=5.4
Step-by-step explanation:
5x+18=45
-18 on both sides
45-18=27
5x=27
divide 5 on both sides
x=5.4
Answer: x=5.4
Step-by-step explanation:
5x=27
x=5.4
A company estimates that it will need $97,000 in 13 years to replace a computer. If it establishes a sinking fund by making fixed monthly payments into an account paying 3.2% compounded monthly, how much should each payment be? (Round your answer to the nearest cent. Do not include any symbols. Example: 56789.12)
Answers
Thus, the company must consider fixed monthly payments of amount $497.92 into the account to reach $97,000 in 13 years.
To find the monthly payment needed to reach $97,000 in 13 years, we can use the sinking fund formula:
FV = PMT * [(1 + i)^n - 1] / i
where:
FV = future value ($97,000)
PMT = monthly payment (unknown)
i = monthly interest rate (3.2% compounded monthly, which is 0.032 / 12 = 0.002667)
n = number of months (13 years * 12 months/year = 156 months)
Rearrange the formula to solve for PMT:
PMT = FV * i / [(1 + i)^n - 1]
PMT = 97,000 * 0.002667 / [(1 + 0.002667)^156 - 1]
PMT ≈ 497.92
So, the company should make fixed monthly payments of approximately $497.92 into the account to reach $97,000 in 13 years.
Know more about the sinking fund formula
https://brainly.com/question/29076086
#SPJ11
what was Tomas's first error

Answers
Answer:
6 + 6y + 6 = 12 + 6
Step-by-step explanation:
2(3) + 6y = 12
Multiply 2(3).
6 + 6y = 12
Subtract 6 on both sides, so y variable is isolated.
6 + 6y - 6 = 12 - 6
6y = 6
Divide by 6 on both sides.
6y/6 = 6/6
y = 1
If you roll a 6-sided die 84 times, what is the best prediction possible for the number of times you will roll an odd number?
Answers
Answer:
42
Step-by-step explanation:
Given that:
Sample space = {1, 2, 3, 4, 5,6}
Probability of obtaining an odd number in a single roll :
Probability = required outcome / Total possible outcomes
Required outcome = {1,3,5} = 3
Total possible outcomes = 6
P(odd number) = 3/6 = 1/2
Number of odds on 84 die rolls :
1/2 * 84 = 42 times
Find the measure of the missing angle using the exterior angle sum theorm.

Answers
Answer:
28°
Step-by-step explanation:
Theorem: the exterior angle of a triangle is equal tobthe sum of opposite interior angles of the triangle
therefore,
55 = unknown angle + 27
let the unknown angle be x
55 = x + 27
isolating x
x = 55 - 27
x = 28
thus, the missing angle is 28°
Graph the solution to the following system of inequalities.
y-2x-9
y<2x+7
Answers
Answer:
Tb bight by icing I jb Yves j by by by navy by iffy i by by by
A surveyer wants to find the distance from points A and B and to an inaccessible point C. These three points form a triangle. Because points he can be cited from both A and B, he knows that the measure of angle a equals 65°. Also, it is known that the distance from A-to-C is 90 m and the distance from B to C is 120 m. What is the measure of angle B
Answers
Answer:
42.8°
Step-by-step explanation:
The sine rule states that for a triangle with lengths of a, b and c and the corresponding angles which are opposite the sides as A, B and C, then the following rule holds:
\(\frac{a}{sin(A)}=\frac{b}{sin(B)} =\frac{c}{sin(C)}\)
Given that points A, B and C forms a triangle with angle A = 65°, distance from A-to-C = 90 m and the distance from B to C = 120 m.
The distance from A to C is the side opposite to angle B. Hence let b = distance from A to C = 90 m.
The distance from B to C is the side opposite to angle A. Hence let a = distance from B to C = 120 m.
Therefore using sine rule:
\(\frac{a}{sin(A)}=\frac{b}{sin(B)} \\\\\frac{120}{sin(65)}=\frac{90}{sin(B)} \\\\sin(B)=\frac{90*sin(65)}{120} \\\\sin(B) =0.6797\\\\B=sin^{-1}(0.6797)\\\\B=42.8^o\)
what equation is equivalent to 6(3p-2)=20?
Answers
Answer:
18 p - 32 = 0
Q4 (15 points)
A borrowing sovereign has its output fluctuating following a uniform distribution U[16, 24]. Suppose that the government borrows L = 6 before the output is known; this loan carries an interest rate ri.
The loan is due after output is realized. 0.5 of its output.
Suppose that if the government defaults on the loan, then it faces a cost equivalent to c =
The loan is supplied by competitive foreign creditors who has access to funds from world capital markets, at a risk-free interest rate of 12.5%.
** Part a. (5 marks)
Find the equilibrium rī.
** Part b. (5 marks)
What is the probability that the government will repay its loan?
* Part c. (5 marks)
Would the borrowing country default if r = r? Prove it.
Answers
a. The equilibrium interest rate, is determined by the risk-free interest rate, the probability of repayment, and the cost of default.
b. The probability of the government repaying its loan can be calculated using the loan repayment threshold and the distribution of the output.
c. If the interest rate, r, is equal to or greater than the equilibrium interest rate, the borrowing country would default.
a. To find the equilibrium interest rate, we need to consider the risk-free interest rate, the probability of repayment, and the cost of default. The equilibrium interest rate is given by the formula: r = r + (c/p), where r is the risk-free interest rate, c is the cost of default, and p is the probability of repayment.
b. The probability that the government will repay its loan can be calculated by determining the percentage of the output distribution that exceeds the loan repayment threshold. Since 0.5 of the output is required to repay the loan, we need to calculate the probability that the output exceeds L/0.5.
c. If the interest rate, r, is equal to or greater than the equilibrium interest rate, the borrowing country would default. This can be proven by comparing the repayment threshold (L/0.5) with the loan repayment amount (L + Lr). If the repayment threshold is greater than the loan repayment amount, the borrowing country would default.
Calculations and further details would be required to provide specific numerical answers for each part of the question.
Learn more about probability here: brainly.com/question/13604758
#SPJ11
chloe lives in an area where she runs the air conditioner during the 4 hottest months of the year. the air conditioner runs for 7 hours per day at a cost of 21 cents per hour. how much does she spend per year on utility bills for the air conditioner?(assume there are 30 days in each month)
Answers
Chloe runs her air conditioner for a total of 4 months, or 120 days. Chloe spends $176.40 per year on utility bills for the air conditioner during the four hottest months.
The reason for this expense is the need for cooling during these months, and the cost associated with running the air conditioner for 7 hours per day at a rate of $0.21 per hour.
To calculate the annual cost of running Chloe's air conditioner, we need to consider the number of hours per day, the cost per hour, and the number of days the air conditioner is used.
1. First, let's determine the total number of hours the air conditioner runs during the four hottest months:
7 hours/day * 30 days/month * 4 months = 840 hours
2. Now, we can calculate the total cost by multiplying the number of hours by the cost per hour:
840 hours * $0.21/hour = $176.40
At 7 hours per day, that's a total of 840 hours of use. At a cost of 21 cents per hour, Chloe spends 0.21 x 840 = $176.40 per year on utility bills for her air conditioner.
Learn more about utility bills here:
brainly.com/question/26060750
#SPJ11
What is nine more than a number y, if that number is 4? Evaluating Algebraic Expressions
Answers
Answer:
13
Step-by-step explanation:
9 more than a number y is
y + 9
If the number y is 4, then you have
4 + 9 = 13
The expression is y +9 and the evaluation is 13.
What is addition?Combining objects and counting them as one big group is done through addition. In arithmetic, addition is the process of adding two or more integers together. Addends are the numbers that are added, and the sum refers to the outcome of the operation.
Let y be the required number.
According to question,
the algebraic expression
y + 9
Here, y =4
Then y + 9
= 4 +9
= 13
Therefore, the expression is y +9 and the evaluation is 13.
To learn more about the addition;
brainly.com/question/29464370
#SPJ3
a real estate agent gets a 6% Commission on Lots Heelys and sells. He gets 3% on Lots listed by someone else that he sells. One week you sold a $90,000 lot listed with another company and a $65,500 lot He listed. Find his Commission.
Answers
Answer:
I hope you have a good new year
Each turn in a game, you randomly draw a card from a deck and replace it. The table shows the number of times you draw each type of card. How many times can you expect to not draw a point card in 30 turns?
Card Type Frequency
Point 6
Resource 3
Defense 9
times ?
Answers
The number of times you can expect not to draw a point card in 30 turns is 20.
What is Probability?
Probability is simply the possibility of getting an event. Or in other words, we are predicting the chance of getting an event.
The value of probability will be always in the range from 0 to 1.
We have,
Total number of turns = 6 + 3 + 9 = 16
Number of times point card drawn = 6
Probability of finding the point card = 6/18 = 1/3
If the number of turns is 30,
Number of times point card is drawn = 1/3 × 30 = 10
Number of times point card is not drawn = 30 - 10 = 20
Hence there are 20 times that the drawn card is not point card.
Learn more about Probability here :
https://brainly.com/question/11234923
#SPJ1
If 20 chickens laid 120
eggs. At this rate, how
many eggs would 30
chicken lay?
Answers
Answer:
180 eggs is the correct amount.
Which of the following functions is equivalent to f(x) = 2( x - 3)2 + 4
-
O f(x) = 2x2 - 6x + 4
O f(x) = 2x2 – 12x + 22
O f(x) = 2x2 + 18x + 4
O f(x) = 2x2 + 12x – 22
Answers
Answer:
\(\implies f(x) = 2x^2-12x + 22 \)
Step-by-step explanation:
Given :-
\( f(x) = 2(x-3)^2+4\)And we need to find out the function from the given options which is equivalent to the given function . For that firstly simplify the whole square term , that is ,
Using Identity :-
\(\implies (a-b)^2= a^2-2ab + b^2\)
Whole square term :-
\(\implies (x-3)^2= x^2+9-6x \)
Now multiply the constant term outside the bracket to it . As ,
\(\implies f(x) = (2x^2+18-12x )+ 4 \)
Add the constant terms .
\(\implies f(x) = 2x^2-12x + 22 \)
Hence the second option is correct .
A recipe for chocolate chip muffins states that it yields 12 muffins, with 340 calories per muffin. you instead decide to make mini-muffins, and the recipe yields 19 muffins. if you eat 4,how many calories will you consume?
Answers
You consume 860 calories for eating 4 muffins.
What is calories?
An energy unit is a calorie. A calorie, also known as a kilocalorie, is one thousand calories of energy. Energy is measured in joules. The kilojoule, or 1000 joules, is the unit of energy.
Given:
A recipe for chocolate chip muffins states that it yields 12 muffins, with 340 calories per muffin.
You instead decide to make mini-muffins, and the recipe yields 19 muffins.
A recipe for chocolate chip muffins states that it yields 12 muffins, with 340 calories per muffin, so one batch of standard size muffins has 12(340) = 4080 calories.
If the same amount of ingredients is made into 19 minimuffins, that's 4080/19 = 215 calories per minimuffin.
Eating 4 of these will be a consumption of 4(215) = 860 calories.
Hence, you consume 860 calories for eating 4 muffins.
To know more about calories, click on the link
https://brainly.com/question/24555200
#SPJ4
30-60-90 right triangles

Answers
Answer:
i think it is either 31 or 15.5
Step-by-step explanation:
Answer:
Step-by-step explanation:
pythagoras theorem.
a squared plus b squared equals hypotenuse squared
a=7
b=24
49+576=625
square root is 25
that's your answer. c=25.
A cone is 8.4cm high.............

Answers
Answer:
Correct option is
B
2.1 cm
Step-by-step explanation:
If the volume of a square based pyramid is 300 centimeters cubed with a height of 9 centimeters, then what is the length of the base?
A: 5.77
B: 7
C: 10
D: 100
Answers
Answer:
the answer is c
Step-by-step explanation:
1/3a^2h
=1/3x10^2x9
=300 feet^3
Martin needs to ship a box that weighs 12,000 grams. How should be move the decimal point to convert this number
to kilograms?
O 3 places to the left
O 3 places to the right
O 2 places to the left
O 2 places to the right
Answers
(1 gram=0.001 kilograms)
Find all values of c so that v (-1, 2, c) and w -(7, 8, 9) are orthogonal. (Enter your answers as a comma-separated list.)
Answers
The values of c for which the vectors v(-1, 2, c) and w(-7, 8, 9) are orthogonal are c = -1 and c = 17.
In order for two vectors to be orthogonal, their dot product must be zero. The dot product of two vectors v = (v1, v2, v3) and w = (w1, w2, w3) is given by v · w = v1w1 + v2w2 + v3w3.
Let's calculate the dot product of v(-1, 2, c) and w(-7, 8, 9):
v · w = (-1)(-7) + (2)(8) + (c)(9)
= 7 + 16 + 9c
= 23 + 9c
For the vectors to be orthogonal, the dot product must be zero, so we have:
23 + 9c = 0
Solving this equation for c, we get:
9c = -23
c = -23/9
Therefore, the vectors v(-1, 2, c) and w(-7, 8, 9) are orthogonal when c = -23/9.
However, we need to find all the values of c for which the vectors are orthogonal. Since the equation 9c = -23 only gives us one solution, we need to explore further. Let's substitute c = -23/9 back into the dot product equation:
v · w = 23 + 9(-23/9)
= 23 - 23
= 0
The dot product is zero, which means that the vectors v(-1, 2, c) and w(-7, 8, 9) are orthogonal when c = -23/9.
So, the only values of c for which the vectors v and w are orthogonal are c = -23/9 and c=17.
Learn more about vectors here:
https://brainly.com/question/24256726
#SPJ11
2. Write a ratio expressing the slope of the line through each pair of points.
(-6, 1) and (-2, 0)

Answers
Answer:
Step-by-step explanation:
m = \(\frac{-1}{4}\)

A. 0-5
B. 5-10
C. 10-15
D.15-20

Answers
Answer:
the answer is Its letter C.
Step-by-step explanation:
letter C C C C C. c. c.
What is the slope and y-intercept of y=6.75?
Answers
Step-by-step explanation:
There's no slope and y-intercept in such a question, maybe you should try typing a valid question for an effective answer.