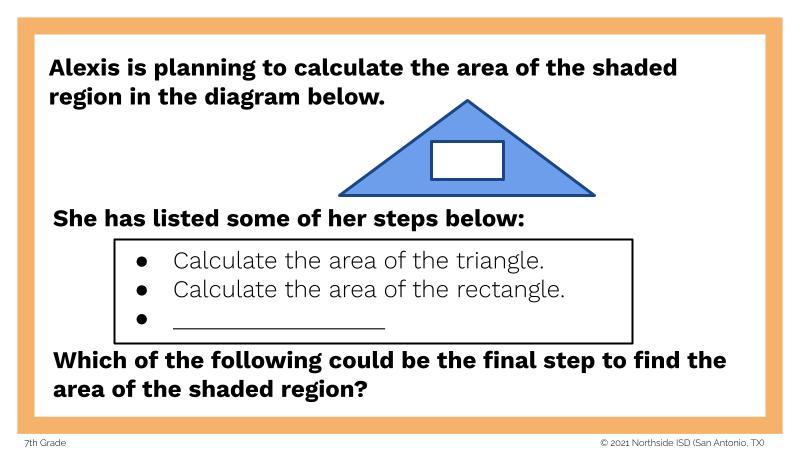
Answers
Answer:
subtract the area of the rectangle from the area of the triangle
Related Questions
creating a discussion question, evaluating prospective solutions, and brainstorming and evaluating possible solutions are steps in_________.
Answers
Creating a discussion question, evaluating prospective solutions, and brainstorming and evaluating possible solutions are steps in problem-solving.
What is problem-solving?
Problem-solving is the method of examining, analyzing, and then resolving a difficult issue or situation to reach an effective solution.
Problem-solving usually requires identifying and defining a problem, considering alternative solutions, and picking the best option based on certain criteria.
Below are the steps in problem-solving:
Step 1: Define the Problem
Step 2: Identify the Root Cause of the Problem
Step 3: Develop Alternative Solutions
Step 4: Evaluate and Choose Solutions
Step 5: Implement the Chosen Solution
Step 6: Monitor Progress and Follow-up on the Solution.
Let us know more about problem-solving : https://brainly.com/question/31606357.
#SPJ11
Indicate which variable you think should be the predictor (x) and which variable should be the response (y). Explain your choices.
a. You have collected data on used cars for sale. The variables are price and odometer readings of the cars.
b. Research is conducted on monthly household expenses. Variables are monthly water bill and household size.
c. A personal trainer gathers data on the weights and time spent in the gym for each of her clients.
Answers
a. Predictor (x): odometer readings. Response (y): price.
b. Predictor (x): household size. Response (y): monthly water bill.
c. Predictor (x): time spent in the gym. Response (y): weight.
a. In this case, we want to predict the price of a used car based on its odometer reading. The price is the response variable, and the odometer reading is the predictor variable. It is reasonable to assume that the more miles a car has on it, the lower its value will be, all else being equal.
b. In this case, we want to predict the monthly water bill based on household size. The monthly water bill is the response variable, and the household size is the predictor variable. It is reasonable to assume that the more people living in a household, the higher their monthly water bill will be, all else being equal.
c. In this case, we want to predict a client's weight based on the time they spend in the gym. Weight is the response variable, and time spent in the gym is the predictor variable. It is reasonable to assume that the more time a person spends in the gym, the more likely they are to lose weight or maintain a healthy weight, all else being equal.
Learn more about variable here
https://brainly.com/question/15078630
#SPJ11
suppose goop purchases 150 gallons of raw material. what is the probability that they will run out of raw material?
Answers
The probability that they will run out of raw material is 0.78 or 78% if the order quantity is 150 gallons.
We have following information,
the demand for the polymer is distributed normally .
Mean (μ) = 250 gallons and Standard deviations (σ) = 125 gallons
Selling price of poymer = $25 per gallons
cost price of raw material for polymer= $ 10 per gallons
Salvage value = $(-5)/ gallon
Now, Using the formula for normally distributed sample,
Z = ( X - μ ) /σ
where , X---> sample mean
μ ----> population mean
σ----> standard deviations
Z -----> Z-value
from question it is clear X = 150
putting all the values of variables in above formula we get, Z = ( 150-250)/125 = -100/125
= - 0.8
(-0.8) value of z = 0.21 = 21% , the value of z is measure from normal distribution table.
They will run out of raw material if demands exceds this quantity or
Probability ( out of stock ) = 1 - 0.21 = 0.79 = 79%
So, the probability that they will run out of raw material is 0.78 or 78% if the order quantity is 150 gallons.
To learn more about Normal distribution , refer:
https://brainly.com/question/4079902?utm
# SPJ4
#Complete Question :
Goop Inc needs to order a raw material to make a special polymer. The demand for the polymer is forecasted to be Normally distributed with a mean of 250 gallons and a standard deviation of 125 gallons. Goop sells the polymer for $25 per gallon. Goop's purchases raw material for $10 per gallon and Goop mrust spend $5 per gallon to dispose off all unused raw material due to government regulations. (One gallon of raw material yields one gallon of polymer.) That is to say, the salvage value is $(-5)/gallon. If demand is more than Goop can make, then Goop sells only what they made and the rest of demand is lost. ?
1. Suppose Good purchases 150 gallons of raw material. What is the probability that they will run out of raw material? ?
Simplify (4y+6)-(3y-5)
Answers
Answer:
y+1
Step-by-step explanation:
4y-3y+6-5
In function apart defined below, how many of the parameters are considered input parameters?voidapart(double x, int wholep, double fracp){*wholep = (int)x;fracp = x - wholep;}
Answers
Out of the three parameters, x is an input parameter, while wholep and fracp are considered both input and output parameters.
In the function apart defined in question, there are three parameters: x, wholep, and fracp.
Among these parameters, x is considered an input parameter because it represents the input value that is passed into the function.
The parameters wholep and fracp can be considered both input and output parameters. They are passed by reference (using pointers) and can be modified within the function. The function updates the values of wholep and fracp based on the calculation, which means they can be considered as output values. However, they are also initially provided as input values when the function is called.
Therefore, out of the three parameters, x is an input parameter, while wholep and fracp are considered both input and output parameters.
Learn more about function here:
https://brainly.com/question/28249912
#SPJ11
2x-8x+1=49
???help please show work
Answers
2x-8x = -6x collect like terms
-6x+1=49
Subtract 1 on both sides
-6x=48
X=-8
below the paraboloid z = 18 − 2x2 − 2y2 and above the xy-plane
Answers
Answer:
y
2
=−
2
z
+7
Steps for Solving Linear Equation
z=18−2×2−2y2
Multiply 2 and 2 to get 4.
z=18−4−2y
2
Subtract 4 from 18 to get 14.
z=14−2y
2
Swap sides so that all variable terms are on the left hand side.
14−2y
2
=z
Subtract 14 from both sides.
−2y
2
=z−14
Divide both sides by −2.
−2
−2y
2
=
−2
z−14
Dividing by −2 undoes the multiplication by −2.
y
2
=
−2
z−14
Divide z−14 by −2.
y
2
=−
2
z
+7
Step-by-step explanation:
the given equation defines a paraboloid that lies below the plane z=0. Specifically, it is situated above the xy-plane, which means that the z-values of all points on the surface are greater than or equal to zero.
we can break down the equation z=18-2x^2-2y^2. This equation represents a paraboloid with its vertex at (0,0,18) and axis of symmetry along the z-axis. The first term 18 is the z-coordinate of the vertex and the last two terms -2x^2 and -2y^2 determine the shape of the paraboloid.
Since the coefficient of x^2 and y^2 terms are negative, the paraboloid is downward facing and opens along the negative z-axis. Therefore, all points on the paraboloid have z-values less than 18. Additionally, since the paraboloid is situated above the xy-plane, its z-values are greater than or equal to zero.
the paraboloid defined by the equation z=18-2x^2-2y^2 is situated below the plane z=0 and above the xy-plane. Its vertex is at (0,0,18) and it opens along the negative z-axis.
To know more about paraboloid, visit:
https://brainly.com/question/30925041
#SPJ11
If x < 0 and y = 0, where is the point (x, y) located?
on the x-axis
on the y-axis
Submit
Answers
Answer:the y axis
Step-by-step explanation: use desmos graphing
A shipping container will be used to transport several 70-kilogram crates across the country by rail. The greatest weight that can be loaded into the container is 23500 kilograms. Other shipments weighing 9700 kilograms have already been loaded into the container. What is the greatest number of 70-kilogram crates that can be loaded into the shipping container?
Answers
Subtract what has already been loaded from total amount allowed:
23500 - 9700 = 13,800
Divide the amount left by the weight of each crate:
13,800 / 70 = 197.14
They can load 197 crates.
An economy has a Cobb-Douglas production function: Y=K α
(LE) 1−α
The economy has a capital share of 1/3, a saving rate of 20 percent, a depreciation rate of 5 percent, a rate of population growth of 2 percent, and a rate of labor-augmenting technological change of 1 percent. In steady state, capital per effective worker is: 4 4 6 1 1.6
Answers
Capital per effective worker in steady state is 6.
In the Cobb-Douglas production function, Y represents output, K represents capital, L represents labor, and α represents the capital share of income.
The formula for capital per effective worker in steady state is:
k* = (s / (n + δ + g))^(1 / (1 - α))
Given:
Capital share (α) = 1/3
Saving rate (s) = 20% = 0.20
Depreciation rate (δ) = 5% = 0.05
Rate of population growth (n) = 2% = 0.02
Rate of labor-augmenting technological change (g) = 1% = 0.01
Plugging in the values into the formula:
k* = (0.20 / (0.02 + 0.05 + 0.01))^(1 / (1 - 1/3))
k* = (0.20 / 0.08)^(1 / (2 / 3))
k* = 2.5^(3 / 2)
k* ≈ 6
Therefore, capital per effective worker in steady state is approximately 6.
In steady state, the economy will have a capital per effective worker of 6
To know more about technological change, visit
https://brainly.com/question/15602412
#SPJ11
Find the surface area of the following pyramid using its net. Please answer correctly the 4 questions and I'll give you brainliest :D

Answers
Answer:
The surface is 7.4344433322 acording to the exact dynamiter and angle of the pyrmaids
Step-by-step explanation
Im smart like that :)
Answer:
1. 224
2. 424.8
3. 85.4
4. 960
Step-by-step explanation:
I used the triangle calculator :)
hope it helps!
Among adults in the US, the distribution of albumin levels in cerebrospinal fluid is roughly symmetric with mean =29.5 mg/100ml and Standard deviation of 9.25mg/100ml. Suppose that you select repeated samples of size 20 from this population and calculate the mean for each. How large must the sample be for 99% of their means to lie within 10 µmol/l of the population mean?
Answers
Rounding up gives us a sample size of 7. Thus, we would require a sample size of 7 for 99 percent of the samples' means to be within 10 µmol/L of the population mean. Therefore, option B is correct.
The distribution of albumin levels in cerebrospinal fluid among adults in the US is roughly symmetric with a mean of 29.5 mg/100 ml and a standard deviation of 9.25 mg/100 ml. If repeated samples of size 20 are taken from this population and the mean is calculated for each, how large should the sample be for 99% of their means to lie within 10 µmol/L of the population mean.Solution:The mean of the population is 29.5 mg/100 ml and the standard deviation is 9.25 mg/100 ml, and we're looking for the number of samples required for 99 percent of the samples' means to be within 10 µmol/L of the population mean. The sample size formula is used for this problem.The sample size formula is:$$n
=\left(\d fraction{z*\sigma}{E}\right)^2$$Where, n is the sample size z is the level of significanceσ is the population standard deviation E is the margin of error The formula for margin of error is:E
= z*σ/√nWe require that the sample mean lies within 10 µmol/L of the population mean with a probability of 0.99.Using a standard normal distribution table, we find that the value of z for a 0.995 probability is 2.58.Accordingly, we have:E
= 10 µmol/Lz
= 2.58σ
= 9.25 mg/100 ml The formula for margin of error is:E
= z*σ/√n Solving for n by plugging in the above values we have:$$n
=\left(\dfraction{z*\sigma}{E}\right)^2$$$$n
=\left(\d fraction{2.58*9.25}{10}\right)^2$$$$n
=6.31$$.Rounding up gives us a sample size of 7. Thus, we would require a sample size of 7 for 99 percent of the samples' means to be within 10 µmol/L of the population mean. Therefore, option B is correct.
To know more about Rounding visit:
https://brainly.com/question/29022222
#SPJ11
simpson's rule can only be applied when the function is normally integrable.
T/F
Answers
True . Simpson's rule can only be applied when the function being integrated is normally integrable. This requirement is necessary to ensure that the method is accurate and reliable, and that the approximation of the integral is meaningful.
Simpson's rule is a numerical method for approximating the value of a definite integral. It can only be applied when the function being integrated is integrable, which means that it has a well-defined integral over the given interval. In other words, the function must be continuous and bounded over the interval. If the function is not integrable, Simpson's rule (or any numerical integration method) cannot be used to find the value of the integral.
Simpson's rule is based on approximating the area under a curve by using a series of parabolic arcs. The method is relatively simple and accurate, but it requires that the function being integrated is well-behaved. This means that the function must be continuous and bounded over the interval of integration. If the function is not continuous, or if it is unbounded (i.e., it increases or decreases without limit), then Simpson's rule cannot be used to find the value of the integral. One way to check whether a function is integrable is to use the Riemann criterion, which states that a function is integrable over a given interval if and only if the upper Riemann sum and the lower Riemann sum converge to the same value as the partition size approaches zero.
To know more about Simpson's rule visit :-
https://brainly.com/question/30459578
#SPJ11
Need help desperately, work overdue :)

Answers
Answer:
a) £108
btw i use sparx maths too
Step-by-step explanation:
The explicit rule for a sequence and one of the specific terms is given. Find the position of the given
term
f(n) -5.5n - 52;25
25 is the BLANK th term
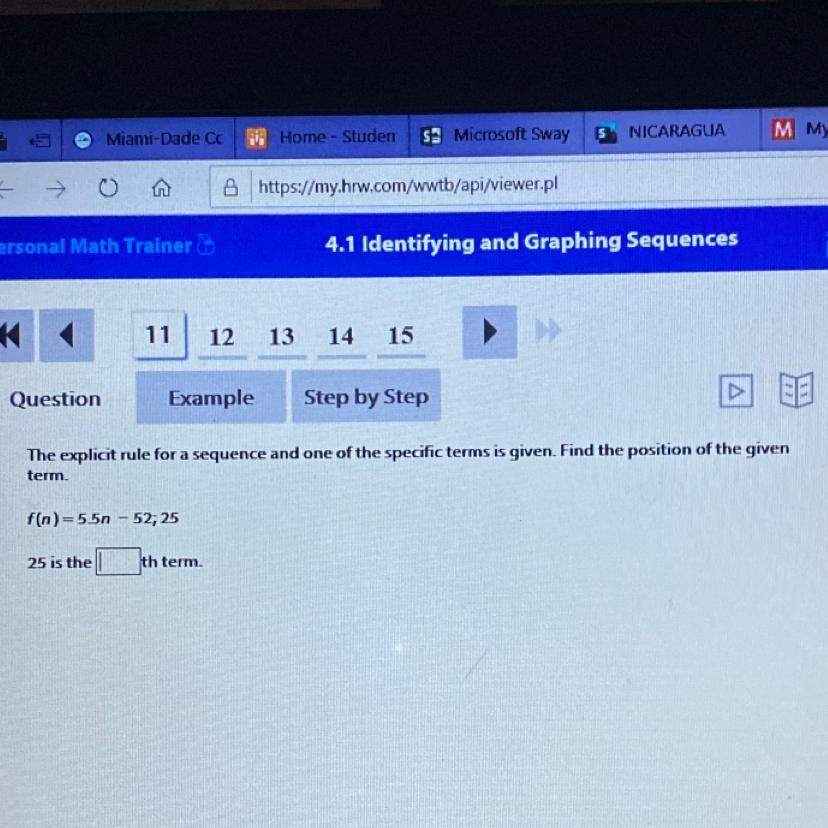
Answers
Answer:
25 is the 14th term.
Step-by-step explanation:
The nth term of a sequence is given by the following explicit rule:
f(n) =5.5n - 52
We need to find the position n of the term of value 25. In other words, find n in the equation:
5.5n - 52=25
Adding 52:
5.5n =25+52=77
Solving:
n=77/5.5
n=14
25 is the 14th term.
A sample of 34 observations is selected from a normal population. The sample mean is 28, and the population standard deviation is 4. Conduct the following test of hypothesis using the 0.05 significance level.
H0: μ ≤ 26
H1: μ > 26
a.Is this a one- or two-tailed test?
One-tailed test
Two-tailed test
b.What is the decision rule?
Reject H0 when z > 1.645
Reject H0 when z ≤ 1.645
c.What is the value of the test statistic? (Round your answer to 2 decimal places.)
d.What is your decision regarding H0?
Reject H0
Fail to reject H0
e-1) What is the p-value? (Round your answer to 4 decimal places.)
e-2)Interpret the p-value? (Round your final answer to 2 decimal places.)
Answers
a. The alternative hypothesis (H1) specifies that is greater than 26, indicating a directed alternative, this is a one-tailed test.
b. The alternative hypothesis is one-sided and argues that > 26, hence the critical value is 1.645.
c. The value of the test statistic (z-score) is z ≈ 3.82.
d. We reject the null hypothesis (H0) because the test statistic (z = 3.82) is higher than the crucial value (1.645).
In this case, the p-value is the probability of observing a sample mean of 28 or greater, assuming the population mean is 26.
a. This is a one-tailed test because the alternative hypothesis (H1) states that μ is greater than 26, indicating a directional alternative.
b. The decision rule for a one-tailed test at a significance level of 0.05 is to reject the null hypothesis (H0) if the test statistic is greater than the critical value. In this case, the critical value is 1.645 because the alternative hypothesis is one-sided and states that μ > 26.
c. The value of the test statistic (z-score) can be calculated using the formula:
z = (x - μ) / (σ / √n)
where x is the sample mean, μ is the population mean, σ is the population standard deviation, and n is the sample size.
In this case:
x = 28
μ = 26
σ = 4
n = 34
Substituting the values into the formula:
z = (28 - 26) / (4 / √34) ≈ 3.82
d. Since the test statistic (z = 3.82) is greater than the critical value (1.645), we reject the null hypothesis (H0).
e-1. To calculate the p-value, we need to find the area under the standard normal distribution curve to the right of the test statistic (z = 3.82). We can use a standard normal distribution table or a calculator to find this area.
The p-value is the probability of observing a test statistic as extreme as the one calculated (or more extreme) under the null hypothesis.
e-2. Interpreting the p-value: The p-value represents the probability of obtaining a sample mean as extreme as the one observed (or more extreme) if the null hypothesis is true.
learn more about hypothesis from givn link
https://brainly.com/question/606806
#SPJ11
At work, Julie focuses on measuring performance and takes the necessary corrective actions. Julie is engaged in the ________ function of the management process.
Answers
Julie, in her role at work, focuses on measuring performance and taking corrective actions, which indicates her involvement in the controlling function of the management process.
The controlling function of the management process involves monitoring and evaluating performance to ensure that organizational goals are achieved effectively and efficiently. It includes activities such as measuring performance, comparing it to predetermined standards or targets, identifying deviations or variances, and taking corrective actions as necessary. Julie's emphasis on measuring performance and implementing necessary corrective actions indicates her engagement in the controlling function. By monitoring performance and taking corrective actions, Julie plays a vital role in ensuring that the organization stays on track, addresses any performance gaps, and maintains alignment with established objectives and standards.
Learn more about measuring here:
https://brainly.com/question/28103959
#SPJ11
Let V = span { sin^2 x, cos^2 x} be a subspace of the vector space of all functions mapping R into R. Let B = {sin^2x, cos^2x) and C = (cos 2x, l}, (a) Find the change-of-coordinates matrix from B to C for vector space V. (b) Find the change-of-coordinates matrix from C to B for vector space V.
Answers
Therefore, the change-of-coordinates matrix from B to C is: [ 1 ] [ -1 ]
(a) To find the change-of-coordinates matrix from B to C, we need to express the vectors in C (cos 2x, 1) as linear combinations of the vectors in B (sin^2 x, cos^2 x).
Let's express (cos 2x, 1) in terms of B:
(cos 2x, 1) = a(sin^2 x, cos^2 x)
Expanding the right side using scalar multiples a and b:
(cos 2x, 1) = a(sin^2 x, cos^2 x) = (asin^2 x, acos^2 x)
Comparing corresponding components, we have the following equations:
cos 2x = asin^2 x
1 = acos^2 x
Dividing the first equation by the second equation, we get:
(cos 2x) / 1 = (asin^2 x) / (acos^2 x)
cos 2x = (sin^2 x) / (cos^2 x)
cos 2x = tan^2 x
Using a trigonometric identity, tan^2 x = sec^2 x - 1, we have:
cos 2x = sec^2 x - 1
To know more about change-of-coordinates matrix,
https://brainly.com/question/31052618
#SPJ11
Aiden misses 10% of the free throws he attempts in a season. How many total free throws did he attempt if he missed 66?
Answers
Answer:
660 free throwsStep-by-step explanation:
Let the total is x.
10% or total is 66, find x:
0.1x = 66x = 66/0.1x = 660If there is no joint variability between two variables, then the r value will be?
Answers
Answer:
If r=0, there is absolutely no relationship between the two variables.
Step-by-step explanation:
Brookfield Street and Bloomington Avenue intersect. If Brookfield Street is 5 meters wide and Bloomington Avenue is 6 meters wide, what is the distance between two opposite corners of the intersection? If necessary, round to the nearest tenth.
Please respond
Thank you!
Answers
Answer:7.8
Step-by-step explanation:
\(\sqrt{6^2+5^2}\)
evaluate the equation / expression
7.81025
Round 7.81025 to the required place
7.8
Answer:
7.8m
Step-by-step explanation:
To find:-
The distance between opposite corners of intersection.Answer:-
From the given data i made a figure to represent the given situation. To find the distance between the opposite corners we would have to use Pythagoras theorem, .
The distance between the corners represents the hypotenuse of the right angled triangle and another two sides are represented by 5m and 6m .
According to Pythagoras theorem,
\(\rm\implies a^2+b^2 = h^2 \\\)
Here a is 5m and b is 6cm .
\(\rm\implies (5m)^3+(6m)^2 = h^2 \\\)
\(\rm\implies 25m^2+36m^2=h^2\\\)
\(\rm\implies 61m^2=h^2\\\)
\(\rm\implies h =\sqrt{61m^2} \\\)
\(\rm\implies \red{ h = 7.8 \ m } \\\)
Hence the distance between the opposite corners is 7.8 m

can someone find a limit at a location where there is a hole in a graph, such as where a point has been removed or where a graph abruptly stops? why or why not? give an explanation that includes the consideration of (in) the limit from the left, (ii) the limit from the right, and (iii) the general limit.
Answers
Yes, it is possible to find a limit at a location where there is a hole in a graph , such as where a point has been removed or where a graph abruptly stops.
This is because the limit of a function is defined as the value that the function approaches as x approaches a given value. In the case of a hole in the graph, the limit is found by looking at the limit from the left, the limit from the right, and the general limit.
The limit from the left is the limit of the function as x approaches the hole from the left. The limit from the right is the limit of the function as x approaches the hole from the right. The general limit is the overall limit of the function at the point where the hole appears. By considering all of these limits, it is possible to determine the overall limit of the function at the point where the hole is present.
Learn more about limits:
https://brainly.com/question/30451475
#SPJ4
Solve for x. Round your answer to two decimal places. Show your work for full credit.
Answers
Answer:
sin(angle) = opposite/hypotenuse
Sin(39) = 9/ hypotenuse
hypotenuse = 9/sin(39) = 14.3011
x = 14.30
Step-by-step explanation:
A ski instructor recorded the amount of snow that fell each Saturday during ski season. He displayed the data in a line plot.
What is the range in this set of data?
Answers
the range in this set of data is 25 inches. To find the range of snowfall amounts, we need to look at the line plot and identify the highest and lowest amounts of snowfall that were recorded.
The range in a set of data is the difference between the maximum and minimum values in the set.
To find the range of snowfall amounts, we need to look at the line plot and identify the highest and lowest amounts of snowfall that were recorded.
Let's say that the highest amount recorded was 30 inches and the lowest amount recorded was 5 inches. Then the range would be:
range = highest amount - lowest amount
range = 30 - 5
range = 25
Therefore, the range in this set of data is 25 inches.
Learn more about data here
https://brainly.com/question/14893265
#SPJ1
I WILL GIVE BRAINLIEST PLSSSSSSSSSSS
The area of a rectangle is 3/4 square yards. If the width of the rectangle is 3/5, what is the length?
EXPRESSION :
ANSWER:
Answers
Answer:
L = 5/4 yards
L = 1 1/4 yards
L = 1.25 yards
Step-by-step explanation:
area = Length * Width
3/4 = L * 3/5
Divide both sides by 3/5
(3/4) / (3/5) = L
When dividing by a fraction flip it over and multiply
3/4 * 5/3 = L
The 3 cancels
5/4 = L
L = 5/4 yards
as a proper fraction
L = 1 1/4 yards
As a decimal
L = 1.25 yards
A mass attached to a vertical spring has position function given by s(t)=5sin(2t) where t is measured in seconds and s in inches:
Find the velocity at time t=1
Find the acceleration at time t=1
Answers
The acceleration of the mass at time t=1 is approximately -19.42 inches/second^2.
To find the velocity and acceleration of the mass attached to a vertical spring at time t=1, we need to differentiate the position function with respect to time.
First, we can find the velocity function v(t) by taking the derivative of the position function s(t) with respect to time t:
v(t) = s'(t) = 5cos(2t) * 2 = 10cos(2t)
Plugging in t=1, we get:
v(1) = 10cos(2) ≈ -3.42 inches/second
Therefore, the velocity of the mass at time t=1 is approximately -3.42 inches/second.
Next, we can find the acceleration function a(t) by taking the derivative of the velocity function v(t) with respect to time t:
a(t) = v'(t) = -20sin(2t)
Plugging in t=1, we get:
a(1) = -20sin(2) ≈ -19.42 inches/second^2
Therefore, the acceleration of the mass at time t=1 is approximately -19.42 inches/second^2.
Learn more about acceleration here
https://brainly.com/question/460763
#SPJ11
Send help plsssss math is difficult

Answers
what is a number in exponential notation that decreases in value as the exponent increases in value
Answers
Answer:
Any fraction
Step-by-step explanation:
Example:
(2/3)² = 4/9
2/3 > 4/9
Answer:
Any Fraction
Step-by-step explanation:
Example:
(1/3)² = 1/9
1/3 > 1/9
Calculate the divergence of the vector field F(x, y, z) = −4 sin(11xy)i – cos(x^6 y)j. (Give an exact answer. Use symbolic notation and fractions where needed.)
Answers
The divergence of the vector field F(x, y, z) = −4 sin(11xy)i – cos(x^6 y)j is 11x cos(11xy) - 6x^5 y sin(x^6 y).
Divergence is a measure of the outflow of a vector field from a point or region of space. In 3D space, it is represented as the scalar dot product of the vector field with the del operator, and is given by the expression div F = ∇·F, where ∇ is the del operator and F is the vector field. The del operator can be represented in terms of the partial derivatives of the vector field with respect to its three independent variables, x, y, and z.
The vector field in this question is F(x, y, z) = −4 sin(11xy)i – cos(x^6 y)j. Therefore, we need to find the divergence of this vector field. Using the formula for divergence, we have:
div F = ∇·F= ∂Fx/∂x + ∂Fy/∂y + ∂Fz/∂z = (−44xy cos(11xy)) + (−6x^5 y sin(x^6 y)) + 0= -44xy cos(11xy) - 6x^5 y sin(x^6 y)
Hence, the divergence of the vector field F(x, y, z) = −4 sin(11xy)i – cos(x^6 y)j is 11x cos(11xy) - 6x^5 y sin(x^6 y).
Learn more about divergence here:
https://brainly.com/question/32236109
#SPJ11
Mr. Adams divides 223 markers equally among the 26 students in his class. He puts the extra markers in a box. What is the least number of markers he puts in the box?
Answers
Answer:
15
Step-by-step explanation:
Answer:
15
Step-by-step explanation: