Express the ratio below in it’s simplest form
3.5:2.5
Answers
\(the \:simpliest\:form\:of\:the\:of\:ratio\:is\: \boxed{\:5\::\:7\: }\)
\(3.5:2.5 = \frac{25}{10} \cdot \frac{35}{10} = \frac{25 \cdot 10}{10} :\frac{35 \cdot 10}{10} \Rightarrow 25 : 35 = \frac{25}{5} :\frac{35}{5} \Rightarrow 5 : 7\)
\(I \:hope\:that\:I\:helped\:you\:!\:\)
Related Questions
On March 1, a company purchased 10 footballs for $5 each. On March 11, the company purchased an additional 10 footballs for $7 each. On March 20, it sold 9 footballs for $20 each. If the company is using the averaging method, its ending inventory on March 20 would be?
Answers
The company's ending inventory on March 20, using the averaging method, would be $75.
Based on the information given, the company purchased a total of 20 footballs - 10 for $5 each and 10 for $7 each. The total cost of these purchases would be (10 * $5) + (10 * $7) = $50 + $70 = $120.
Since the company sold 9 footballs on March 20, the cost of the sold footballs would be 9 * $5 (as per the averaging method). Therefore, the cost of the sold footballs is $45.
To calculate the ending inventory, we subtract the cost of the sold footballs from the total cost of the purchases. Thus, the ending inventory would be $120 - $45 = $75.
In conclusion, the company's ending inventory on March 20, using the averaging method, would be $75.
To know more about cost visit:
brainly.com/question/14566816
#SPJ11
Suppose there is a colony of bacteria that decays at a rate of -17% per hour. When will half of the population be left
Answers
To find when half of the population of bacteria will be left given a decay rate of -17% per hour, you can use the formula for exponential decay:
Final Population = Initial Population * (1 + Decay Rate)^Time
In this case, you want to find the time when half of the population is left, so:
0.5 * Initial Population = Initial Population * (1 - 0.17)^Time
Now, you can solve for Time:
0.5 = (1 - 0.17)^Time
0.5 = 0.83^Time
To solve for Time, you can take the logarithm of both sides of the equation:
log(0.5) = log(0.83^Time)
Using the logarithm property, you can move Time to the front of the equation:
log(0.5) = Time * log(0.83)
Finally, solve for Time by dividing both sides by log(0.83):
Time = log(0.5) / log(0.83)
After calculating, you'll find that Time ≈ 3.94 hours. So, it will take approximately 3.94 hours for half of the bacterial population to be left, given a decay rate of -17% per hour.
Learn more about colony of bacteria at https://brainly.in/question/9750692
#SPJ11
i have no idea about how to do it.

Answers
The blanks are filled as follows
Step one
Equation 2x + y = 18 Isolate y,
y = 18 - 2x
How to complete the stepsStep Two:
Equation 8x - y = 22, Plug in for y
8x - (18 - 2x) = 22
Step Three: Solve for x by isolating it
8x - (18 - 2x) = 22
8x - 18 + 2x = 22
8x + 2x = 22 + 18
10x = 40
x = 4
Step Four: Plug what x equals into your answer for step one and solve
y = 18 - 2x
y = 18 - 2(4)
y = 18 - 8
y = 10
So the solution to the system of equations is x = 40 and y = 10
Learn more about system of equations at
https://brainly.com/question/25976025
#SPJ1
Smoothie Activity
6. Using the relative frequency table, create a segmented bar graph by employee type using technology or by hand. If using Excel technology the columns may need to be switched after inserting the chart. Click on the chart and the "Chart Design" ribbon will pop up. Then select "Switch Row/Column." (10 points)

Answers
By answering the presented question, we may conclude that I used the following procedures to produce this graph.
What is graphs?Mathematicians use graphs to visually display or chart facts or values in order to express them coherently. A graph point usually represents a connection between two or more items. A graph, a non-linear data structure, is made up of nodes (or vertices) and edges. Glue the nodes, also known as vertices, together. This graph contains vertices V=1, 2, 3, 5, and edges E=1, 2, 1, 3, 2, 4, and (2.5), (3.5). (4.5). Statistical graphs (bar graphs, pie graphs, line graphs, and so on) are graphical representations of exponential development. a logarithmic graph shaped like a triangle.
I used the following procedures to produce this graph:
I classified the personnel as full-time, part-time, and temporary.
I estimated the proportion of employees who assessed the company's work-life balance as "very good" or "excellent" for each employee category, as well as the percentage who rated it as "good" or "fair/poor."
I used the following procedures to produce this graph:
I classified the personnel as full-time, part-time, and temporary.
I estimated the proportion of employees who assessed the company's work-life balance as "very good" or "excellent" for each employee category, as well as the percentage who rated it as "good" or "fair/poor."
I made the segmented bar graph using these percentages.
The graph was made using Excel technology. You may make a similar graph with Excel or any other software that supports segmented bar graphs.
To know more about graphs visit:
https://brainly.com/question/11950136
#SPJ1
Find the value of x in the trapezoid below.
X
63°
X = = [?]°
Answers
The value of x in the trapezoid is 117°.
What is trapezoid ?A trapezoid is a quadrilateral a four-sided polygon with two parallel sides and two non-parallel sides. The parallel sides are called the bases of the trapezoid, and the non-parallel sides are called the legs.
In a trapezoid, the opposite angles are supplementary. Therefore, we can set up an equation:
x + 63° = 180°
Solving for x, we get:
x = 180° - 63° = 117°
Therefore, the value of x in the trapezoid is 117°.
Learn more about trapezoid here : brainly.com/question/26487711
#SPJ1
(w+9)+3 write an expression equivalent
Answers
Answer:
\(\displaystyle 12 + w\)
Explanation:
Just combine like-terms to arrive at your answer:
\(\displaystyle \boxed{12 + w} \Rightarrow [w + 9] + 3\)
I am joyous to assist you at any time.
Help please it’s confusing ⚠️⚠️

Answers
The continuous random variable V has a probability density function given by: 6 f(v) = for 3 ≤ ≤7,0 otherwise. 24 What is the expected value of V? Number
Answers
The expected value of the continuous random variable V is 5. The expected value of V is 5, indicating that, on average, we expect the value of V to be around 5.
To calculate the expected value of a continuous random variable V with a given probability density function (PDF), we integrate the product of V and the PDF over its entire range.
The PDF of V is defined as:
f(v) = 6/24 = 0.25 for 3 ≤ v ≤ 7, and 0 otherwise.
The expected value of V, denoted as E(V), can be calculated as:
E(V) = ∫v * f(v) dv
To find the expected value, we integrate v * f(v) over the range where the PDF is non-zero, which is 3 to 7.
E(V) = ∫v * (0.25) dv, with the limits of integration from 3 to 7.
E(V) = (0.25) * ∫v dv, with the limits of integration from 3 to 7.
E(V) = (0.25) * [(v^2) / 2] evaluated from 3 to 7.
E(V) = (0.25) * [(7^2 / 2) - (3^2 / 2)].
E(V) = (0.25) * [(49 / 2) - (9 / 2)].
E(V) = (0.25) * (40 / 2).
E(V) = (0.25) * 20.
E(V) = 5.
Therefore, the expected value of the continuous random variable V is 5.
The expected value represents the average value or mean of the random variable V. It is the weighted average of all possible values of V, with each value weighted by its corresponding probability. In this case, the expected value of V is 5, indicating that, on average, we expect the value of V to be around 5.
Learn more about random variable here
https://brainly.com/question/17217746
#SPJ11
Let X 1 ,X 2 ,…,Xn be iid Bern(p) random variables, so that Y=∑ i=1n X i is a Bin(n,p) random variable. (a) Show that Xˉ =Y/n is an unbiased estimator of p. (b) Show that Var( Xˉ )=p(1−p)/n. (c) Show that E{ Xˉ (1− Xˉ )}=(n−1)[p(1−p)/n]. (d) Find the value of c such that c Xˉ (1− Xˉ ) is an unbiased estimator of p(1−p)/n.
Answers
a) X is an unbiased estimator of p. b) The Var(X) is p(1-p)/n. c) The E[X(1-X)] is (n-1)[p(1-p)/n]. d) The value of c is c = 1/(n-1).
(a) To show that X = Y/n is an unbiased estimator of p, we need to show that E[X] = p.
Since Y is a sum of n iid Bern(p) random variables, we have E[Y] = np.
Now, let's find the expected value of X:
E[X] = E[Y/n] = E[Y]/n = np/n = p.
Therefore, X is an unbiased estimator of p.
(b) To find the variance of X, we'll use the fact that Var(aX) = a^2 * Var(X) for any constant a.
Var(X) = Var(Y/n) = Var(Y)/n² = np(1-p)/n² = p(1-p)/n.
(c) To show that E[X(1-X)] = (n-1)[p(1-p)/n], we expand the expression:
E[X(1-X)] = E[X - X²] = E[X] - E[X²].
We already know that E[X] = p from part (a).
Now, let's find E[X²]:
E[X²] = E[(Y/n)²] = E[(Y²)/n²] = Var(Y)/n² + (E[Y]/n)².
Using the formula for the variance of a binomial distribution, Var(Y) = np(1-p), we have:
E[X²] = np(1-p)/n² + (np/n)² = p(1-p)/n + p² = p(1-p)/n + p(1-p) = (1-p)(p + p(1-p))/n = (1-p)(p + p - p²)/n = (1-p)(2p - p²)/n = 2p(1-p)/n - p²(1-p)/n = 2p(1-p)/n - p(1-p)²/n = [2p(1-p) - p(1-p)²]/n = [p(1-p)(2 - (1-p))]/n = [p(1-p)(1+p)]/n = p(1-p)(1+p)/n = p(1-p)/n.
Therefore, E[X(1-X)] = E[X] - E[X²] = p - p(1-p)/n = (n-1)p(1-p)/n = (n-1)[p(1-p)/n].
(d) To find the value of c such that cX(1-X) is an unbiased estimator of p(1-p)/n, we need to have E[cX(1-X)] = p(1-p)/n.
E[cX(1-X)] = cE[X(1-X)] = c[(n-1)[p(1-p)/n]].
For unbiasedness, we want this to be equal to p(1-p)/n:
c[(n-1)[p(1-p)/n]] = p(1-p)/n.
Simplifying, we have:
c(n-1)p(1-p) = p(1-p).
Since this should hold for all values of p, (n-1)c = 1.
Therefore, the value of c is c = 1/(n-1).
To know more about unbiased estimator:
https://brainly.com/question/32063886
#SPJ4
An 18% tip is left on a $65 dinner bill. 5% tax is added (before tip). What is the total paid?
Answers
Answer: $79.95
Step-by-step explanation:
65 × 0.18 = 11.7
65 × 0.05 = 3.25
11.7 + 3.25 = 14.95
14.95 + 65 = $79.95
What is the purpose of using prefixes in the metric system?
Answers
Answer: to scale the base units so that they can represent anything from a very large numeric value
Step-by-step explanation:
to scale the base units so that they can represent anything from a very large numeric value
The president of a large university believes that on average professors in the U.S. teach 3 classes. A professor at the university believes the number is actually less. The professor takes a random sample of 90 professors at universities in the U.S. and finds that they teach an average of 2.7 classes. The professor enlists you to determine if this data confirms or denies the president's claim.
Answers
Using hypothesis test, the professor's finding of an average of 2.7 classes supports the belief that the number is less than 3, contradicting the president's claim.
How to Confirm or Deny a Claim Using Hypotheses?To determine if the data confirms or denies the president's claim, a hypothesis test can be conducted. The null hypothesis (H₀) would state that the average number of classes taught by professors in the U.S. is 3, while the alternative hypothesis (H₁) would state that it is less than 3.
Using the sample data, a one-sample t-test can be performed with a significance level (α) chosen, such as 0.05. If the test statistic falls within the critical region (rejecting the null hypothesis), it would suggest that the professor's finding of an average of 2.7 classes supports the belief that the number is less than 3, contradicting the president's claim.
Learn more about Hypotheses on:
https://brainly.com/question/29576929
#SPJ1
yolanda has $84 to purchase x pairs of mittens and y scarves as gifts for her relatives. a pair of mittens costs $6 and a scarf costs $4 could yolanda buy 5 pairs of mittens and scarves?
Answers
Answer:
Yes
Step-by-step explanation:
\(6\) × \(5 = 30\)
\(4\) × \(5 = 20\)
\(30 + 20 = 50\)
\(84-50=34\)
Yolanda can purchase 5 pairs of mittens and 5 scarves and still have $34 leftover.
Two events are ________ if the occurrence of one is related to the probability of the occurrence of the other.
Answers
Answer:
Dependent
Step-by-step explanation:
Two events are said to be dependent when the outcome of the first event is related to the other.
When two events, A and B are dependent, the probability of occurrence of A and B is:
P(A and B) = P(A) · P(B|A)
https://brainly.com/question/13604758
#SPJ4
Two events are dependent if the occurrence of one is related to the probability of the occurrence of the other.
A probability-dependent event is an event whose occurrence affects the probabilities of others. Suppose you have 3 red balls and 6 green balls in your pocket. Two balls are drawn one after the other from the bag. A dependent event is an event that depends on what happened before. These events are affected by previously occurring results.In other words, two or more intedependent events are called dependent events. A random change in one event can deviate from another.If two events A and B depend on each other, then the probability of A and B occurring is
P(A and B) = P(A) P(B|A)
Two events are dependent if the occurrence of one is related to the probability of the occurrence of the other.
Learn more about dependent events here
https://brainly.com/question/27692683
#SPJ4
answer this image ( I have to type 20 characters)

Answers
Answer:
So the slope would equal 2
The y intercept would be 3
the equation is y = 2x+3
Step-by-step explanation:
hoped that helped
What is 0 divided by 0
Answers
Answer: undefined
Step-by-step explanation:
Answer:
um.. the answer is "0"
Step-by-step explanation:
8.
Last week, Janet used 4 cups of flour to make pizza dough. This week, she uses 3/8
the amount of flour as last week. Which statement is true about the amount of
flour Janet uses this week?
Janet uses more than 4 cups of flour because 4 is greater than 1.
Janet uses more than 4 cups of flour because 4 is greater than 3/8.
Janet uses less than 4 cups of flour because is less than 1.
Janet uses less than 4 cups of flour because is less than 4.
As
Answers
Answer:
Janet uses less than 4 cups of flour because is less than 4.
Step-by-step explanation:
She is using 3/8 of 4 so less than. Then you use 4 not 1 because there is 4 cups.
Answer:
She uses less than four cups because 3/8 (1.5) is less than 4.
Step-by-step explanation:
Which of the following values for a will make the equation 12 - X = 5 true?
A) -17
B) -7
C) 7
D) 17
Answers
the awnser is X = 7 imple numerical terms are commonly written last.
In order to solve this linear equation, we need to group all the variable terms on one side, and all the constant terms on the other side of the equation.
In our example,
- term , will be moved to the right side.
Notice that a term changes sign when it 'moves' from one side of the equation to the other.
We need to get rid of expression parentheses.
If there is a negative sign in front of it, each term within the expression changes sign.
Otherwise, the expression remains unchanged.
In our example, there are no negative expressions.
X = 7
Answer:
c) 7
Step-by-step explanation:
Given that,
→ 12 - x = 5
Let's solve the problem,
→ 12 - x = 5
→ x = 12 - 5
→ [ x = 7 ]
Hence, the value is 7.
4). Find the general solution of the nonhomogeneous ODE using the method of undetermined coefficients: y" + 2y'- 3y = 1 + xeˣ (b) A free undamped spring/mass system oscillates with a period of 3 seconds. When 8 lb is removed from the spring, the system then has a period of 2 seconds. What was the weight of the original mass on the spring?
Answers
(a) the general solution of the nonhomogeneous ODE is y(x) = c1e^(-3x) + c2e^x + 2 + (3x + 4)e^x, where c1 and c2 are arbitrary constants.
(b) the weight of the original mass on the spring was 72 lb.
a) To find the general solution of the nonhomogeneous ODE y" + 2y' - 3y = 1 + xe^x, we first find the general solution of the associated homogeneous equation, which is y_h'' + 2y_h' - 3y_h = 0. The characteristic equation is r^2 + 2r - 3 = 0, which has roots r = -3 and r = 1. Therefore, the general solution of the homogeneous equation is y_h(x) = c1e^(-3x) + c2e^x, where c1 and c2 are arbitrary constants.
To find the particular solution, we assume a particular form for y_p(x) based on the nonhomogeneous terms. For the term 1, we assume a constant, and for the term xe^x, we assume a polynomial of degree 1 multiplied by e^x. Solving for the coefficients, we find y_p(x) = 2 + (3x + 4)e^x.
Thus, the general solution of the nonhomogeneous ODE is y(x) = c1e^(-3x) + c2e^x + 2 + (3x + 4)e^x, where c1 and c2 are arbitrary constants.
b) To find the weight of the original mass on the spring, we can use the formula for the period of an undamped spring/mass system, T = 2π√(m/k), where T is the period, m is the mass, and k is the spring constant.
Initially, with the original weight on the spring, the period is 3 seconds. Let's denote the original mass as m1. Therefore, we have 3 = 2π√(m1/k).
When 8 lb is removed from the spring, the period becomes 2 seconds. Denoting the new mass as m2, we have 2 = 2π√((m1 - 8)/k).
Dividing the second equation by the first, we get (2/3)² = [(m1 - 8)/k] / (m1/k), which simplifies to 4/9 = (m1 - 8) / m1.
Solving for m1, we have m1 = 72 lb.
Therefore, the weight of the original mass on the spring was 72 lb.
To learn more about homogeneous equation click here: brainly.com/question/12884496
#SPJ11
Someone help and please make sure the answer is right
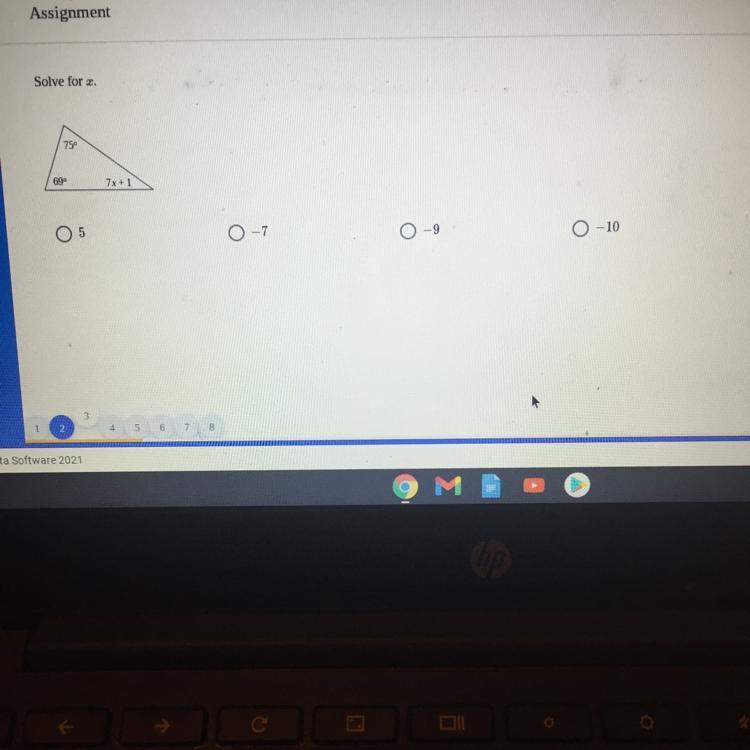
Answers
Answer:
5
Step-by-step explanation:
angles in a triangle will alwasy equal 180*
75+69=144
180-144=36
now you need to find what number subed for x will = 36
7(5)+1= 35+1=36
good luck and hoped this helped
5
Select the correct answer from each drop-down menu.
What is the distance and midpoint between points D and E on the number line?
D
E
+++
-4-3-2 -1 0 1 2 3
distance =
midpoint =
|||||||
4
5
Reset
Next
Answers
Answer:
4.20
Step-by-step explanation:
hope this helps
What Is the fraction 3/8+1/6
Answers
Answer:
13/24
Step-by-step explanation:
3/8 + 1/6
We need to get a common denominator of 24
3/8 *3/3 + 1/6*4/4
9/24 + 4/24
13/24
helpppppppppppppppppppppppppppp





Answers
Answer:
6)180-99=81
81+43=124
180-124=56
b=56
Question 3 of 10What is the median of the following data values?108, 102, 43, 100, 22O A. 100OB. 80C. 95OD. 75SUBM
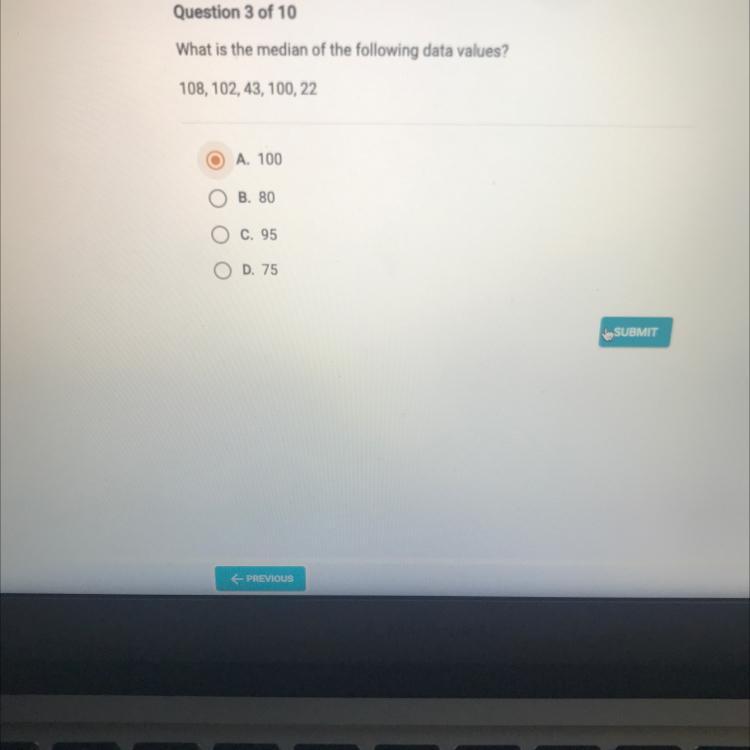
Answers
The median is the value that separates the higher half from the lower half of a data set.
To find the median, first, write the number in ascending order.
22, 43, 100, 102, 108
Since there are 5 numbers in the data set, the median is the 3th number.
Then, the median is 100.
Answer: 100.
What is the length of his lower body in centimeters

Answers
The answer to the question is A: 0.96 centimeters
\(\frac{2}{5}\) of 1.6 is 0.64
If you subtract 0.64 from 1.6 you get 0.96, \(\frac{3}{5}\) of 1.6
Find the area of this shapr

Answers
Answer:
42 m^2
Step-by-step explanation:
we find the area of the rectangle with the formula WxL
7 x 4 = 28 m^2
we find the area of the triangle with the formula 1/2 b x h
1/2 7 x 4 =
3.5 x 4 = 14 m^2
we add the two areas
28 + 14 = 42m^2 (your answer)
How does math come easy to you guys?
Answers
Answer:
An easy way to understand math easily is to study math more and do it more.
Answer:
More practice & write down every step.
Step-by-step explanation:
A printer prints 28 photos in 8 minutes. How many minutes does it take to print 21 photos?
Answers
Answer:
6 min.Step-by-step explanation:
ratio and proportion:
28 photos = 21 photos
8 min x min.
21 (8) = 28 (x)
x = 168 / 28
x = 6 min.
What is the magnitude of ?
V
(9,-4)
Answers
Answer:
The magnitude is sqrt((-4)^2 + (-9)^2) = 9.85. The angle is atan(-9/-4) = 180 deg + 66 deg = 246 deg = -114 deg.
Step-by-step explanation:
hope it help
Answer:
9.85
Step-by-step explanation:
|v|= √9²+(-4)²
=√81+16
=√97
|v|= 9.85
Solve for p.
p/2 − 3.71 = –3.51
p =
Answers
Step-by-step explanation:
mark me as the brainliest
