Find the Maclaurin series expansion of the function f(z) = (2-1)(z-2) in the domain 1 < |z| < 2.
Answers
The expansion for the function f(x) = (2 - 1)*(z - 2) centered at z = 0 in the given domain is:
f(z) = z - 1.
How to find the Maclaurin expansion?Here we want to find the Maclaurin series expansion for the function:
f(z) = (2 - 1)*(z - 2)
We can trivially simplify this, because the first term is equal to 1, so we will get:
f(z) = z - 2
The Maclaurin series expansion of f(z) is a power series centered at z = 0 (or the origin). Since we're given the domain 1 < |z| < 2, which is an annulus centered at the origin, we can express f(z) as a Laurent series.
To determine the Laurent series expansion of f(z), we'll expand it as a series of powers of (z - 0) = z. However, we need to exclude the terms with negative powers of z since the domain does not include z = 0 (so it is not really a laurent series)
Let's express f(z) as a Laurent series:
f(z) = z - 2 = z - 2(1) = z - 2 + 2(1)
The term "2(1)" can be considered as a constant term in the Laurent series expansion. Now, let's focus on the term "z - 2". We can express it as a power series of z:
z - 2 = z - 2(1) = z - 2z⁰
Therefore, the Laurent series expansion of f(z) in the given domain is:
f(z) = z - 2 + 2(1) + 0z² + 0z³ + ...
Simplifying further, we have:
f(z) = z - 2 + 2 = z - 1
Thus, the Laurent series expansion of f(z) = (2 - 1)(z - 2) in the domain 1 < |z| < 2 is f(z) = z - 1.
Learn more about power series at:
https://brainly.com/question/14300219
#SPJ4
Related Questions
3x + 5.9 = 14.6
What's the answer?
Answers
Answer: X=2.9
Step-by-step explanation:
(x^2+3x)=(x^2+x+2)
is it a or b
a. x=2
b. x=1
Answers
Answer:
b. x = 1
Step-by-step explanation:
(1^2+3x)=(1^2+1+2) is equal to 4
Find the area of the circle

Answers
Answer:
A ≈ 27.34
Step-by-step explanation:
Answer:
27.34
Step-by-step explanation:
The table shows the number of cups of water required when cooking different amounts of rice.
Amount of
Rice
(cups) Amount of
Water
(cups)
2 5
3 7.5
5 12.5
8 20
Which statements apply to the ratio of rice and water? Choose two options.
The amount of rice is the dependent value.
The amount of water is the dependent value.
The amount of rice is the independent value.
The amount of water is the independent value.
The values cannot be labeled as dependent or independent without a given equation.
Answers
Answer:
C The amount of rice is the independent value
Step-by-step explanation:
The table in your question was formatted a bit off but im assuminng that it was meant to show that the amount of rice is the x value and the amount of water was the y value.
The x value is always the independant variable and the y value is the dependant variable. This is because the value of y will depend on x. In other words, the amount of water needed is going to depend on the amount of rice used.
Hope this helps
What is the vertex of the absolute value function below?

Answers
Answer: (-3,2)
Step-by-step explanation:
(-3,2) is where the line changes.
Answer:
B
Step-by-step explanation:
edge 2021
While reading about a research study, which of the following would tell you that an association claim is being
made?
a. The presence of a scatterplot or bar graph
b. The measurement of two variables
c. The use of a correlation coefficient
d. The interrogation of internal validity
Answers
The answer to this question is c.The use of a correlation coefficient.
The use of a correlation coefficient would tell you that an association claim is being made in a research study. A correlation coefficient is a statistical measure that shows the strength and direction of a relationship between two variables. It ranges from -1 to 1, with 0 indicating no relationship and -1 or 1 indicating a perfect negative or positive relationship, respectively.
A scatterplot or bar graph may be used to visually display the relationship between two variables, but they do not necessarily indicate that an association claim is being made. The measurement of two variables is necessary for any type of research study, but it does not inherently imply that an association claim is being made.
Interrogation of internal validity is a process used to ensure that the study's results accurately reflect the relationship between the variables being studied. It is important for any research study but does not specifically indicate that an association claim is being made.
Therefore,the answer to this question is c.The use of a correlation coefficient.
Know more about correlation coefficient here,
https://brainly.com/question/29704223
#SPJ11
Chebyshev's theorem says that for any set of observations, the proportion of values that lie within k standard deviations of the mean is?
Answers
Chebyshev's theorem says that for any set of observations, the proportion of values that lie within k standard deviations of the mean is 1 - 1/k².
What do you mean by standard deviation?In statistics, Standard deviation is a measure of the variation of a set of values.
σ = standard deviation of population
N = number of observation of population
X = mean
μ = population mean
We know that Chebyshev's theorem states that for a large class of distributions, no more than 1/k² of the distribution will be k standard deviations away from the mean.
This means that 1 - 1/k² of the distribution will be within k standard deviations from the mean.
Lets k = 1.8, the amount of the distribution that is within 1.8 standard deviations from the mean is;
1 - 1/1.8² = 0.6914
= 69.14%
Hence, Chebyshev's theorem says that for any set of observations, the proportion of values that lie within k standard deviations of the mean is 1 - 1/k².
Learn more about standard deviation and variance:
https://brainly.com/question/11448982
#SPJ1
What is the range of the set: 49, 68, 62, 47, 39, 54, 43
Answers
so ... 68 - 39 = 29
The range of the given numbers in the data set is 29.
What is the range of the set?Range is the difference between the highest and lowest values of a set of observations.Range is a measure of variation.
Range = highest value - lowest value
68 - 39 = 29
To learn more about range, please check: https://brainly.com/question/12372689
A stock fell 80 points on Monday and rose 65 points on Tuesday. By how
much has the value of the stock changed over the two-day period?
Answers
answer this please :(

Answers
A sample of 11 individuals shows the following monthly incomes.
Individual
Income ($)
1
1,500
2
2,000
3
2,500
4
4,000
5
4,000
6
2,500
7
2,000
8
4,000
9
3,500
10
3,000
11
43,000
a. What would be a representative measure of central location for the above data? Explain.
b. Determine the mode.
c. Determine the median.
d. Determine the 60th percentile.
e. Drop the income of individual number 11 and compute the standard deviation for the first 10 individuals.
Answers
a. The representative measure of central location for the given data would be the mean income. b. The mode is the value that appears most frequently in the data set. c. The median is the middle value in the sorted data set. d. The 60th percentile represents the value below which 60% of the data falls. e. After dropping the income of individual number 11, the standard deviation can be calculated for the remaining 10 individuals.
a. To find the representative measure of central location, we calculate the mean income. We add up all the incomes: $11,500 + $22,000 + $32,500 + $44,000 + $54,000 + $62,500 + $72,000 + $84,000 + $93,500 + $103,000 + $114,300 = $692,800. Dividing this sum by 11 (the number of individuals) gives us the mean income of $62,981.82.
b. The mode is the value that appears most frequently in the data set. In this case, there is no value that appears more than once, so there is no mode.
c. To determine the median, we first sort the incomes in ascending order: $11,500, $22,000, $32,500, $44,000, $54,000, $62,500, $72,000, $84,000, $93,500, $103,000, $114,300. The median is the middle value, which in this case is the 6th value, $62,500.
d. The 60th percentile represents the value below which 60% of the data falls. To determine the 60th percentile, we arrange the data in ascending order and locate the value that corresponds to the 60th percentile. In this case, the 60th percentile falls between the 6th and 7th values, which are $62,500 and $72,000. To interpolate the exact value, we can use the formula: 60th percentile = lower value + (0.6 × difference between the lower and higher values). Using this formula, the 60th percentile is approximately $67,500.
e. After dropping the income of individual number 11 ($143,000), we are left with the incomes of the first 10 individuals. To compute the standard deviation, we first calculate the mean of the remaining incomes (excluding the dropped income), which is $57,480. We then calculate the squared difference between each income and the mean, sum up these squared differences, divide by 10 (n-1), and take the square root of the result. This will give us the standard deviation for the first 10 individuals.
Learn more about standard deviation here:
https://brainly.com/question/13498201
#SPJ11
What additional information can be used to prove ZDX=XYZ by SSS?
A) DX=YZ
B) DZ=YX
C) XZ=XY
D) XZ=ZD

Answers
Answer:
The answer is going to be A. DX = YZ
Step-by-step explanation:
To furnish a cafeteria, a school can spend $5200 on tables and chairs. Tables cost $200 and chairs cost $40. Each table will have 8 chairs around it. Write and solve a system of equations for x and y. How many tables and chairs will the school purchase?
Answers
The number of tables is 100 and the number of chairs purchased is 800.
Given,
In the question:
To furnish a cafeteria, a school can spend $5200 on tables and chairs. Tables cost $200 and chairs cost $40. Each table will have 8 chairs around it.
To write and solve a system of equations for x and y and how many tables and chairs will the school purchase?
Now, According to the question:
We need to form an equation from this given information and solve it for x to find the number of tables and chairs that the school will purchase.
The number of tables is x, so number of chairs is 8x
Total cost of tables=200x
Total cost of chairs=40 × 8x=320x
Total expenditure=200x + 320x=520x
520x = 5200
x = 100
Therefore, the number of tables is 100 and the number of chairs purchased is 800.
Learn more about Equation at:
https://brainly.com/question/14686792
#SPJ1
What is the formula for varies inversely?
Answers
Inverse variation is the relationships between variables that are represented in the form of \(y=\frac{k}{x}\), where \(x\) and \(y\) are two variables and \(k\) is the constant value. It states that if one quantity's value rises, the other quantity's value falls.
We notice that the variation in values of one quantity depends on the variation in values of another quantity in our day-to-day lives. A variable's inverse variation in relation to another variable is known as inverse variation. It exemplifies the opposite relationship that exists between two quantities. As a result, a variable and another variable are inversely proportional.
In everyday life, there are numerous real-world examples of inverse variation. For instance: If a train travels at a constant speed for a greater distance, it takes longer to complete the journey, and vice versa. On the other hand, if more people are assigned to a job, it takes less time to complete it.
To know more about inverse variation,
brainly.com/question/14109259
A regression table mainly shows us Select one: a. How we should run the regression analysis.b. what the coefficients values are, and whether each coefficient is significant (likely to be different from from 0)c. that the smallest coefficients always have the greatest impact on the dependent variable.d. if a large constant term is important.e. all of the above.
Answers
A regression table mainly shows us that what the coefficients values are, and whether each coefficient is significant (likely to be different from from 0) .
A regression table is a statistical technique that describes how strongly a dependent variable and one or more independent variables are correlated (s). A field or variable you are attempting to predict or comprehend could be a dependent variable.
Regression allows researchers to predict or explain the variation in one variable based on another variable. The variable that researchers are trying to explain or predict is called the response variable. It is also sometimes called the dependent variable because it depends on another variable.
What is interpretation of regression statistics table?
In statistics, regression is a technique that can be used to analyze the relationship between predictor variables and a response variable. When you use software (like R, SAS, SPSS, etc.) to perform a regression analysis, you will receive a regression table as output that summarize the results of the regression.
A regression table primarily reveals the values of the coefficients as well as the significance of each coefficient (how likely it is that it will depart from 0).
To learn more about regression visit:
brainly.com/question/7656407
#SPJ4
Please help. Will mark Brainliest.
Write |3x-5|-|5x+1| as a piecewise function
Answers
Answer:
f(x) = 2x + 6, x < - 1/5
-8x + 4, -1/5 ≤ x ≤ 5/3
-2x - 6, x>5/3
Step-by-step explanation:
3x-5 ≥ 0, 5x + 1 ≥ 0
3x ≥ 5, 5x ≥ -1
x ≥ 5/3, x ≥ -1/5
Intervals: x < -1/5, -1/5 ≤ x ≤ 5/3, x>5/3
Equations:
-(3x - 5) + (5x + 1) = -3x + 5 + 5x + 1 = 2x + 6
-(3x - 5) -(5x + 1) = -3x + 5 - 5x - 1 = -8x + 4
3x - 5 -(5x + 1) = 3x - 5 - 5x - 1 = -2x - 6
Piecewise Function:
f(x) = 2x + 6, x < - 1/5
-8x + 4, -1/5 ≤ x ≤ 5/3
-2x - 6, x>5/3
Here is your graph (and the piecewise functions to the right):

Determine the missing step for solving the quadratic equation by completing the square. 0 = –6x2 + 24x – 5
Answers
Step-by-step explanation:
Check the above photos....



Need help on this question help please

Answers
(a) Determine whether each relation is an equivalence relation. Justify your answer. If the relation is an equivalence relation, then describe the partition defined by the equivalence classes.
(b) The domain is a group of people. Person x is related to person y under relation S if x is currently the spouse of person y. You can assume that there is no polygamy and that there is at least one person who is married to another person in the group.
(c) The domain is the set of all integers. xEy if x + y is even. An integer z is even if z = 2k for some integer k.
Answers
a) Equivalence relations are those which satisfy three properties: symmetric, reflexive, and transitive. If these three conditions are met, then a relation is an equivalence relation.
If the relation satisfies these three conditions, we can say it is an equivalence relation. This relation has no equivalence class as there is only one element. For every x belongs to X, (x,x) belongs to R. Thus, R is a reflexive relation.
For every x, y belongs to X, if (x,y) belongs to R then (y,x) belongs to R. Thus, R is a symmetric relation. For every x, y, and z belongs to X, if (x,y) belongs to R and (y,z) belongs to R then (x,z) belongs to R. Thus, R is a transitive relation.
b) If we consider S as the relation of persons who are spouses of each other, then it is an equivalence relation as it satisfies the three properties that an equivalence relation must satisfy.
Therefore, the spouses in the domain would be partitioned into equivalence classes, where each equivalence class contains a couple that are spouses of each other.
c) We know that an integer z is even if z=2k for some integer k. Thus, xEy if x+y=2k. It is an equivalence relation, as it satisfies the three conditions of an equivalence relation. In this relation, there are two equivalence classes: {2k,2k}, {2k+1,2k-1}.
To learn more about integer, refer below:
https://brainly.com/question/15276410
#SPJ11
Use your knowledge about linear function to determine if the two point (-2,-3) and (2,-1) are belong to the same function? If they do, then what is the function? Show your work.
Answers
Yes, the two points (-2,-3) and (2,-1) belong to the same linear function.
To determine the function, we can use the slope-intercept form of an linear equation, which is y = mx + b, where m is the slope and b is the y-intercept.
First, we need to find the slope of the line using the formula m = (y2 - y1) / (x2 - x1). Plugging in the values of the given points, we get:
m = (-1 - (-3)) / (2 - (-2)) = 2 / 4 = 1/2
Now, we can use one of the given points and the slope to find the y-intercept. Let's use the point (2,-1) and plug in the values into the equation y = mx + b:
-1 = (1/2)(2) + b
Solving for b, we get:
b = -1 - 1 = -2
Therefore, the equation of the linear function is y = (1/2)x - 2.
So, the two points (-2,-3) and (2,-1) belong to the same function, which is y = (1/2)x - 2.
To know more about linear function click on below link:
https://brainly.com/question/21107621#
#SPJ11
an 8 inch candle burns 1/4 inch every hour. a 12 inch large candle burns 1/2 inch every hour. after how many hours will the two candles be the same height?
Answers
Answer:
69
\
Step-by-step explanation:
Complete the proof that
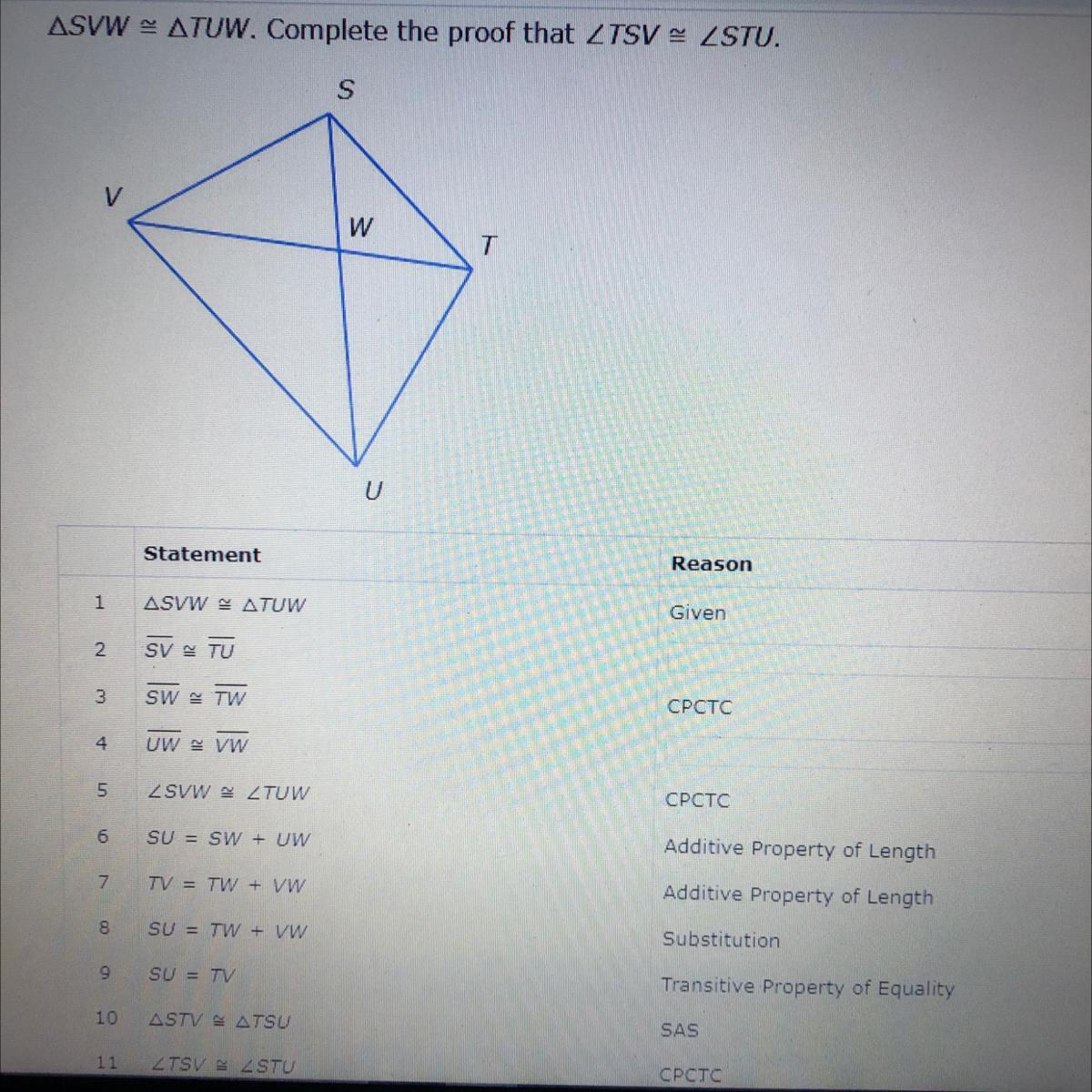
Answers
Answer:
The two column proof is presented as follows;
Statement \({}\) Reason
ΔSCW ≅ ΔTUW \({}\) Given
\(\overline {SV}\) ≅ \(\overline {TU}\) \({}\) CPCTC
\(\overline {SW}\) ≅ \(\overline {TW}\) \({}\) CPCTC
\(\overline {WV}\) ≅ \(\overline {UW}\) \({}\) CPCTC
∠SVW ≅ ∠TUW \({}\) CPCTC
SU = SW + UV \({}\) Additive property of Length
TU = TW + VW \({}\) Additive property of Length
SU = TW + VW \({}\) Substitution
SU = TV \({}\) \({}\) Transitive property
ΔSTV ≅ ΔTSU \({}\) SAS
∠TSV ≅ ∠STU \({}\) CPCTC
Step-by-step explanation:
The two column proof is presented as follows;
Statement \({}\) Reason
ΔSCW ≅ ΔTUW \({}\) Given
\(\overline {SV}\) ≅ \(\overline {TU}\) \({}\) Congruent Parts of Congruent Triangles are Congruent
\(\overline {SW}\) ≅ \(\overline {TW}\) \({}\) Congruent Parts of Congruent Triangles are Congruent
\(\overline {UV}\) ≅ \(\overline {VW}\) \({}\) Congruent Parts of Congruent Triangles are Congruent
∠SVW ≅ ∠TUW \({}\)Congruent Parts of Congruent Triangles are Congruent
SU = SW + UV \({}\) Additive property of Length
TU = TW + VW \({}\) Additive property of Length
SU = TW + VW \({}\) Substitution
SU = TV \({}\) Transitive property
ΔSTV ≅ ΔTSU \({}\) Side-Angle-Side rule of congruency
∠TSV ≅ ∠STU \({}\) Congruent Parts of Congruent Triangles are Congruent
Kay and Karen went on a fishing trip.Kay caught 20 fish and Karen caught 12 fish. What is the value of the ratio
Answers
describe how the graphs of y=1/2x +7 is the same and different from the graph of y= 12x + 7
Answers
Answer:
the slope of the line for y=12x+7 is steeper compared to the other equation
they both have the same y-intercept of y=7
Step-by-step explanation:
i just plugged it into desmos and looked at the graph
hope this helps <3

T/F the square root of a number will always have two outcomes one is positive and the other is negative.
Answers
we have only one outcome that is neither positive nor negative, then the statement is false.
What is square root?
A value known as the square root of a number is one that, when multiplied by itself, yields the original number. An alternative to square rooting a number is to use it. Therefore, the concepts of squares and square roots are connected. The original number is equal to the square root of any integer, which is equivalent to a number.
Let's assume that m is an integer that is positive, such that (m.m) = (m2) = m.
This seems to be true because:
-2*-2 = 2*2 = 4
So √4 = 2 and -2
But particularly the square root of zero is:
√0 = 0
So here we have only one outcome that is neither positive nor negative, then the statement is false.
Learn more about square roots, by the following link.
https://brainly.com/question/428672
#SPJ1
If cos(6x-15) = sin(9x) what is the value of x
Answers
The value of X in the trigonometric equation cos(6x-15) = sin(9x) is X = 7
What is trigonometric equation?The equations that involve the trigonometric functions of a variable are called trigonometric equations
From trigonometric rules the relation
Sin x = Cos (90 - x)
Cos x = Sin ( 90 - x)
Substitute into the equation
Cos ( 6x -15) = Sin( 90 -(6x-15))
= Sin(90-6x+15)
= Sin (105 -6x) = Sin (9x)
Evaluate the equation
= 105 - 6x =9x
Collect the like terms
= 105 = 9x + 6x
15x = 105
Divide both sides by 15
15x/ 15 = 105/15
x = 7
Therefore, the value of X in the trigonometric is X =7
Learn more about trigonometric equation here: https://brainly.com/question/24349828
#SPJ1
Help me fast asap this is due by the end of class

Answers
Answer:
D
Step-by-step explanation:
-12/6= -2
x^10/x^8= x^2
y^3/y= y^2
Solve the equation using the distributive property and properties of equality. One-half (x + 6) = 18 What is the value of x? 6 7 and one-half 14 and one-half 30
Answers
Answer:
The value of x is 30.
We have to find the value of x in the given equation.
Using distributive property
We have,
\(1/2(x+6)=18\\a.(b+c)=a.b+a.c\\1/2(x+3)=18\\1/2x=15\\x=3\)
other are also solve by this methode;)
The required solution of the expression \(1/2(x+6) = 18\) is 30.
To solve the equation using the distributive property and properties of equality. One-half (x + 6) = 18 and value of x to be determined.
The equation is the well-organized link of the variables of two expressions that contain equal between them.
distributive properties are used to evaluate the math problem easily by distributing numbers to the numbers present in parenthesis. eg, if we apply the distributive property of multiplication to solve the expression
a( b + c ) = a.b + a.c
\(1/2(x+6) = 18\)
Using distributive property a( b + c ) = a.b + a.c
\(1/2.x+1/2*6 = 18\\1/2x+3=18\\1/2x=18-3\\1/2x=15\\x=2*15\\x=30\\\)
Thus, The required solution of the expression \(1/2(x+6) = 18\) is 30.
Learn more about the equation here:
https://brainly.com/question/10413253
#SPJ5
Find the derivative of y with respect to z.y = ln (sinh 9z)
Answers
The given function is:
\(y=\ln (\sinh 9z)\)Differentiate w. r. t. z to get:
\(\begin{gathered} \frac{dy}{dz}=\frac{d}{dz}(\ln \sinh 9z) \\ =\frac{1}{\sinh9z}\frac{d}{dz}(\sinh 9z) \\ =\frac{1}{\sinh9z}\cosh 9z(\frac{d}{dz}9z) \\ =\frac{9\cosh 9z}{\sinh 9z} \\ =9\cot h9z \end{gathered}\)Hence the derivative is 9cot9z.
50 POINTS AND BRAINLYEST Triangle NMO has vertices at N(−5, 2), M(−2, 1), and O(−3 , 3). Determine the vertices of image N′M′O′ if the preimage is reflected over x = −1.
N′(5, −2), M′(2, 1), O′(3, 3)
N′(−5, 5), M′(−2, 3), O′(−3, 7)
N′(3, 2), M′(0, 1), O′(1, 3)
N′(−5, −2), M′(−2, −1), O′(−3, −3)
Answers
Answer:
N'(3, 2), M'(0, 1), O'(1, 3)
Step-by-step explanation:
Please sketch the graphs of the two triangles and the line of reflection to confirm my answer.
Since NMO is reflected over the line
x = -1, the y-coordinates of N'M'O' will remain the same. Since the x-coordinate of N is at -5, which is 4 units to the left of the line of reflection, the x-coordinate of N' is at -1 + 4 = 3. Since the x-coordinate of M is at -2, which is 1 unit to the left of from the line of reflection, the x-coordinate of M' is at -1 + 1 = 0. Since the x-coordinate of O is at -3, which is 2 units to the left of the line of reflection, the x-coordinate of O' is at -1 + 2 = 1. So the vertices of N'M'O' are at (3, 2), (0, 1), and (1, 3).