Angela is following this recipe to make biscuits.
Angela uses 1.1 litres of syrup.
How much sugar is needed in kg?
Recipe: Makes 14 biscuits
110 g margarine
100 g sugar
275 ml syrup
200 g oats
50 g sultanas
Answers
Answer:
0.4 kg
Step-by-step explanation:
A proportion can be used to find the amount of sugar.
x/(1.1 L) = (0.1 kg)/(0.275 L)
x = (1.1)(0.1)/0.275 kg = 0.4 kg
Angela needs 0.4 kg of sugar for her biscuits.
Related Questions
Match the vocab word

Answers
Answer:
1). Algebraic expression - a letter or symbol used to represent an unknown.
2). Coefficient - a numerical value.
3). Constant - the constant preceding the variables in a product.
4). Expression - a mathematical expression containing one or more variables.
5). Variable - a mathematical phrase that cannot be determined true or false.
Solve 2+2+2+2+8+8+8
Good answer get brainliast
Answers
\(2+2+2+2+8+8+8\)
Add up the 2's:
\(2+2+2+2\\=8\)
Add up the 8's:
\(8+8+8\\=24\)
Add both numbers together:
\(8+24\\=\fbox{32}\)
Answer:
32
Step-by-step explanation:
2+2+2+2=8
8+8+8=24
24+8=32
Can anybody pls help! Offering 25 points! This is due soon!

Answers
Answer: A' (1, 5)
B' (-3, 5)
C' (-3, 2)
D' (1, 2)
Step-by-step explanation:
To reflect across the y-axis the original point (x, y) must become (-x, y) which is essentially taking the opposite of x
We can do that with each of the point of the rectangle ABCD to make the reflect rectangle A'B'C'D'
A (-1, 5) to A' (1, 5)
B (3, 5) to B' (-3, 5)
C (3, 2) to C' (-3, 2)
D (-1, 2) to D' (1, 2)
in the time series design, if a researcher notes that every time that sampled inviduals are observed on the DV that the average score increases. can the researcher attribute variation on the DV to treatment
Answers
In a time series design, a researcher collects data on a dependent variable (DV) at multiple time points before and after the implementation of a treatment. If the researcher notes that every time sampled individuals are observed on the DV, the average score increases, it may be tempting to attribute this variation to the treatment.
However, caution should be exercised when making such conclusions. While the observed trend in the DV may be associated with the treatment, it's essential to consider alternative explanations, such as maturation, history, or regression to the mean. Maturation refers to the natural developmental processes that occur in participants over time, which might contribute to the observed changes. History refers to external events that could impact the DV, unrelated to the treatment. Regression to the mean occurs when extreme scores naturally become closer to the average over time, which might be mistaken as a treatment effect.
To confidently attribute variation in the DV to the treatment, the researcher should consider using a control group and a comparison group design. This allows for the comparison of changes in the DV between those who received the treatment and those who did not, reducing the likelihood of confounding variables.
In summary, although the increasing average scores in a time series design may suggest a relationship between the treatment and the DV, the researcher should be cautious when attributing this variation solely to the treatment. Other factors and potential confounding variables must be considered before making any definitive conclusions.
Learn more about treatment here:
https://brainly.com/question/31477810
#SPJ11
If 4x-4t=-10 is a true equation, what would be the value of 4x-4y+9?
Answers
Answer:
uhh... that is impossible to know since we don't know the value 'y'
Step-by-step explanation:
In a drawer, there are 11 pairs of socks, 6 of which are white, and 8 t-shirts, 7 of which are white. If you randomly select one pair of socks and one t-shirt, what is the probability that both are white
Answers
The probability of both the socks and the t-shirt being white is 21/44.
The probability of selecting a white pair of socks from the drawer is 6/11. The probability of selecting a white t-shirt from the drawer is 7/8. To find the probability of both events occurring together, we multiply the two probabilities:
P(white socks and white t-shirt) = (6/11) * (7/8) = 0.4773
Therefore, the probability of randomly selecting one pair of white socks and one white t-shirt from the drawer is approximately 0.4773 or 47.73%.
Hi there! To find the probability that both the socks and the t-shirt you randomly select are white, you'll need to multiply the individual probabilities for each item being white.
For the socks, there are 6 white pairs out of a total of 11 pairs. So, the probability of selecting a white pair is 6/11.
For the t-shirts, there are 7 white ones out of a total of 8. So, the probability of selecting a white t-shirt is 7/8.
Now, multiply these probabilities together: (6/11) * (7/8) = 42/88. Simplify the fraction to get 21/44.
So, the probability of both the socks and the t-shirt being white is 21/44.
Learn more about probability here
https://brainly.com/question/24756209
#SPJ11
What is the domain of 4-2x?
Answers
A fireman’s ladder leaning against a house makes an angle of 62 with the ground. If the ladder is 3 feet from the base of the house, how long is the ladder?
Answers
In the given scenario ladder is 6.52 feet long.
Given that,
The angle between ground and ladder = 62 degree
The distance of ladder from ground and ladder = 3 feet
We have to find the length of ladder.
Since we know that,
The trigonometric ratio
cosθ = adjacent/ Hypotenuse
Here we have,
Adjacent = 3 feet
Hypotenuse = length of ladder
Thus to find the length of ladder we have to find the value of hypotenuse.
Therefore,
⇒ cos62 = 3/ Hypotenuse
⇒ 0.46 = 3/ Hypotenuse
⇒ Hypotenuse = 3/0.46
= 6.52
Thus,
length of ladder = 6.52 feet.
Learn more about the triangle visit;
brainly.com/question/1058720
#SPJ1

If you marked the midpoints of the edges of a cube and sliced off all its corners through the midpoints of its edges, how many and what type of faces would the truncated figure have?
Answers
The truncated figure formed by slicing off the corners of a cube through the midpoints of its edges would have 14 faces. The types of faces in the truncated figure are:
6 square faces: These are the original faces of the cube that remain unchanged after truncation.
8 hexagonal faces: These faces are formed by the truncation of the corners of the cube. Each hexagonal face is created by joining the midpoints of three adjacent edges of the cube.
Therefore, the truncated figure would have 6 square faces and 8 hexagonal faces, totaling 14 faces in total.
Learn more about truncated here:
https://brainly.com/question/25863850
#SPJ11
Ballio made 8 identical bags using 3 yards of fabric. How much fabric did Ballio use for each bag?
Answers
For each bag, BALLIO would have needed 2.25 (or 2 1/4) yards of fabric.
What is unitary method?"A way to find a multiple unit value from a single unit value as well as the reverse."
In every case, we first count the unit or amount value before figuring out the more or less amount value.
This method is referred to as a unified procedure for this reason.
By dividing the set value by the quantity of sets, one can find numerous set values.
By dividing several set values by the total number of sets, one can determine a set value.
According to our question-
9 bags you are making
must be the same
divide the nine bags by the four yards
2.25 yards per bag
learn more about unitary method click here:
brainly.com/question/24587372
#SPJ1
Can somebody plz answer both correct and just write which one I circle thanks!! (Only if u remember)
(WILL MARK BRAINLIEST) :D

Answers
Answer:
7. +6
8. -2
9. +1
10. +2
11. -4
12. +4
13. -3
14. -2
15. +1
16. -5
17. -5
18. 0
Step-by-step explanation:
Determine the x-intercepts of the following equation.
(x+1) (x+2)=y
Answer: (-1, 0)(-2,0)
Answers
You rent an apartment that costs
$
800
$800 per month during the first year, but the rent is set to go up 12.5% per year. What would be the rent of the apartment during the 10th year of living in the apartment? Round to the nearest tenth (if necessary).
Answers
Answer:
2,309.2
This could be wrong but I'm pretty sure it's right
Which of the following are like radicals? Check all
of the boxes that apply.
3x√x²y
O -12x√√x³y
O-2x√xy³²
Ox√yx²
-x√x²y
O 2√xy
Answers
The like radicals are 3x√x²y and x√yx², and the answer is:
3x√x²y, -2x√xy³², x√yx², 2√xy
What is expression?An expression is a combination of numbers, variables, and mathematical operations, such as addition, subtraction, multiplication, and division. It does not have an equal sign and can be simplified or evaluated by applying the appropriate rules of arithmetic.
According to the given information:
The like radicals have the same radicand (expression under the radical sign) and the same index (the number outside the radical sign). Checking each expression:
3x√x²y: The radicand is x²y and the index is 2.-12x√√x³y: The expression can be simplified as -12x√(x√(x^2 * y)) = -12x√x³√y, which has a different index than the first expression, so it is not a like radical.-2x√xy³²: The radicand is xy³² and the index is 2.x√yx²: The expression can be simplified as x√(y*x²) = x√(x²y), which has the same radicand and index as the first expression, so it is a like radical.-x√x²y: The expression can be simplified as -x√(x²y) = -x|x|√y, which has a different coefficient and a different index than the first expression, so it is not a like radical.2√xy: The radicand is xy and the index is 2.Therefore, the like radicals are 3x√x²y and x√yx², and the answer is:
3x√x²y, -2x√xy³², x√yx², 2√xy
To know more about radicals,expression visit:
https://brainly.com/question/3796764
#SPJ1
Identify the quadrant or axis that the following points lie on, if the point lies on an axis, specify which part (positive or negative) of which axis (X or Y)

Answers
Given point (-1, 9), we should determine the quadrant it belongs to and also determine which is positive or negative between x and y.
from the above graph, we can see that the point is in the first quadrant.
x is negative
y is positive

The line plot below shows the amount of water ( in gallons) students drank in a week. What is the difference in the lowest amount of water and the highest amount of water students drank
Answers
The difference in the lowest amount of water and the highest amount of water students drank is 1/2
How to determine the difference?From the complete question (see attachment for line plot), we have:
Least = 1/8
Highest = 5/8
The difference is then calculated as:
Difference = Highest - Least
This gives
Difference = 5/8 - 1/8
Evaluate
Difference = 4/8
Simplify
Difference = 1/2
Hence, the difference in the lowest amount of water and the highest amount of water students drank is 1/2
Read more about line plots at:
https://brainly.com/question/15853311
#SPJ4

How do I find the answer for the shaded region

Answers
find the zeros
p(x)=5x3 −5x 2 −10x
and then place them on a graph
Answers
The polynomial function p(x) = 5x³ - 5x² - 10x have zeros of 0, -1 and 2
Zeros of a PolynomialThe zeros of a polynomial p(x) are all the x-values that make the polynomial equal to zero. They are interesting to us for many reasons, one of which is that they tell us about the x-intercepts of the polynomial's graph. We will also see that they are directly related to the factors of the polynomial.
The polynomial function given is
p(x) = 5x³ - 5x² - 10x
The zeros of the function are 0, 2, -1
To test the zeros if they are the roots of the polynomial
p(0) = 5(0)³ - 5(0)² - 10(0)
p(0) = 0
p(2) = 5(2)³ - 5(2)² - 10(2)
p(2) = 0
p(-1) = 5(-1)³ - 5(-1)² - 10(-1)
p(-1) = 0
The zeros are 0, -1, and 2 to the polynomial
Learn more on zeros of a polynomial here;
https://brainly.com/question/20896994
#SPJ1
Please show me step by step how to do this

Answers
Answer:
You know that the beginning salary is $32,000, and it is raised by $1,000 per year.
a) We want to find a recursive relation, let's try to find a pattern:
S₁ = salary on the first year = $32,000
S₂ = salary on the second year = $32,000 + $1,000 = $33,000
S₃ = salary on the third year = $33,000 + $1,000 = $34,000
and so on.
We already can see that the recursive relation is: "the salary of the previous year plus $1,000", this can be written as:
Sₙ = Sₙ₋₁ + $1,000
Such that S₁ = $32,000
b) Your salary in the fifth year is S₅
Let's construct it:
S₃ = $34,000
S₄ = $34,000 + $1,000 = $35,000
S₅ = $35,000 + $1,000 = $36,000
Your salary on the fifth year is $36,000
c) When we have a recursive relation like:
Aₙ = Aₙ₋₁ + d
The sum of the first N elements is given by:
Sum(N) = N*(2*A₁ + (N - 1)*d)/2
Then the sum of your salary for the first 20 years is:
S(20) = 20*(2*$32,000 + (20 - 1)*$1,000)/2
S(20) = $830,000
7. The basketball team is selling hats and gloves. They will earn $10 for every hat they sell and $8 for every pair of gloves. They want to sell a total of 40 items. Create two unique equations that model the number of hats, h, and the number of gloves, y, that the team is selling.
Answers
Answer:
5h+35y=390$
20h+20y=360$
Step-by-step explanation:
there are many answers possible.
The following are just examples:
5h+35y=390$
20h+20y=360$
please help:
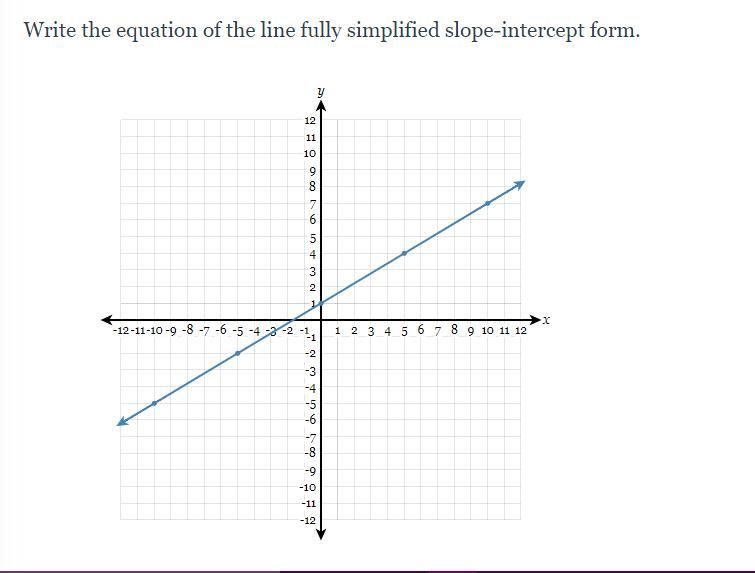
What is an equation in slope-intercept form for the line given?

Answers
The equation of the line passing through points (-1, 2) and (1, -1) is y = -1.5x + 0.5
How to solve an equationAn equation is an expression that shows the relationship between two or more numbers and variables.
The standard form of a linear equation is:
y = mx + b
Where m is the slope of the line and b is the y intercept
The line given passes through the point (-1, 2) and (1, -1). The equation is:
y - 2 = [(-1-2)/(1 - (-1)](x - (-1)]
y - 2 = -(3/2)(x + 1)
y = -(3/2)x - (3/2)
y = -1.5x + 0.5
The equation of the line is y = -1.5x + 0.5
Find out more on equation at: https://brainly.com/question/2972832
#SPJ1
b) Use Newton's method to find 3/5 to 6 decimal places. Start with xo = 1.8.
c) Consider the difference equation n+1 = Asin(n) on the range 0 ≤ n ≤ 1. Use Taylor's theorem to find an equilibrium
Answers
b) Using Newton's method starting with xo = 1.8, we find 3/5 ≈ 0.6.
c) Using Taylor's theorem, the equilibrium point for n₊₁ = Asin(n) on 0 ≤ n ≤ 1 is A = 1.
b) Using Newton's method to find 3/5 (0.6) to 6 decimal places:
Newton's method is an iterative numerical method for finding the roots of a function. To find the root of a function f(x) = 0, we start with an initial guess x₀ and iteratively improve the guess using the formula:
xₙ₊₁ = xₙ - f(xₙ) / f'(xₙ)
where f'(xₙ) is the derivative of f(x) evaluated at xₙ.
In this case, we want to find the root of the function f(x) = x - 3/5. We start with an initial guess x₀ = 1.8 and apply the Newton's method formula:
x₁ = x₀ - f(x₀) / f'(x₀)
To find the derivative f'(x), we differentiate f(x) = x - 3/5 with respect to x, which gives f'(x) = 1.
Substituting these values, we get:
x₁ = 1.8 - (1.8 - 3/5) / 1
Simplifying the expression:
x₁ = 1.8 - (9/5 - 3/5) / 1
x₁ = 1.8 - (6/5) / 1
x₁ = 1.8 - 6/5
x₁ = 1.8 - 1.2
x₁ = 0.6
Therefore, after one iteration, we find that the approximate value of 3/5 to 6 decimal places using Newton's method starting with x₀ = 1.8 is x₁ = 0.6.
c) Using Taylor's theorem to find an equilibrium point for the difference equation n₊₁ = A sin(n) on the range 0 ≤ n ≤ 1:
Taylor's theorem allows us to approximate a function using a polynomial expansion around a given point. In this case, we want to find an equilibrium point for the difference equation n₊₁ = A sin(n) on the range 0 ≤ n ≤ 1.
To find an equilibrium point, we need to find a value of n for which n₊₁ = n. Substituting n₊₁ = A sin(n) into this equation, we get:
A sin(n) = n
Expanding sin(n) using its Taylor series expansion, we have:
n + n³/3! + n⁵/5! + ...
Ignoring higher-order terms, we can approximate sin(n) as n. Substituting this approximation into the equation, we get:
n ≈ A n
This implies that A = 1, as n cannot be zero.
Therefore, the equilibrium point for the difference equation n₊₁ = A sin(n) on the range 0 ≤ n ≤ 1 is A = 1.
To learn more about Taylor's theorem visit : https://brainly.com/question/28168045
#SPJ11
Rewrite the expression as a simplified expression containing one term.

Answers
Answer:
-(cos α)/(sin α)
= -cot α
Step-by-step explanation:
use trigonometric identities

The length of a rectangular speaker is three times its width, and the height is four more than the width. Write an expression for the volume v of the rectangular prism in terms of its width w.
Answers
Answer:
\(V=(3W^{3} + 12W^{2} ) units^{3}\)
Step-by-step explanation:
we know that
The volume of a rectangular prism is equal to
\(V=LBH-- >\)\(EquationA\)
where
L is the length
W is the width
H is the height
we know that
\(L=3W--- > EquationB\)
\(H = W + 4----- > EquationC\)
substitute equation B and equation C in equation A
\(V=(3W)(W)(W+4)\)
Apply distributive property
\(V=(3W^{3} + 12W^{2} ) units^{3}\)
Pls mark me Brainliest
please answer this question it would be highly appreciated

Answers
He owes 200 so the first part of the equation would be the starting value of -200.
You would then multiply 25 by the number of months he has given them money and add that to the -200, this would make the -200 a smaller value every month meaning he owes his parents less money.
The answer is A. y = -200 + 25x
What is the slope of the line containing the points (-2, 1) and (4, -3)?
Answers
Step-by-step explanation:
the slope is always the ratio "y coordinate charge / x coordinate change" when going from one point on the line to another.
so, when going from (-2, 1) to (4, -3)
x changes by +6 (from -2 to 4).
y changes by -4 (from 1 to -3).
therefore, the slope is
-4/+6 = -4/6 = -2/3
Find cos (330°). Your answer MUST be in radical form.
Answers
Step-by-step explanation:
square root 3 divided by
2
A candy store uses 10. 3 grams of sugar each hour. How many grams of sugar will the store use in 10 hours?
Answers
The candy store will use 103 grams of sugar in 10 hours.
To find out how many grams of sugar the store will use in 10 hours, we can simply multiply the amount of sugar used in one hour (10.3 grams) by the number of hours (10).
To solve the problem, we use a simple multiplication formula: the amount used per hour (10.3 grams) multiplied by the number of hours (10) to find the total amount of sugar used in 10 hours.
We can interpret this problem using a rate equation: the rate of sugar usage is 10.3 grams/hour, and the time period is 10 hours. Multiplying the rate by the time gives the total amount of sugar used.
So the calculation would be:
10.3 grams/hour x 10 hours = 103 grams
Therefore, the candy store will use 103 grams of sugar in 10 hours.
Learn more about Basic Maths:
https://brainly.com/question/17083065
#SPJ4
help plsssss!!!! i dont get it

Answers
Answer:
sin2θ = \(\frac{1320}{3721}\)
Step-by-step explanation:
Given
sinθ = \(\frac{11}{61}\) = \(\frac{opposite}{hypotenuse}\)
This is a right triangle with legs 11 and x ( adjacent ) and hypotenuse 61
Using Pythagoras' identity in the right triangle to find x
x² + 11² = 61²
x² + 121 = 3721 ( subtract 121 from both sides )
x² = 3600 ( take the square root of both sides )
x = \(\sqrt{3600}\) = 60
Then cosθ = \(\frac{adjacent}{hypotenuse}\) = \(\frac{60}{61}\)
Using the identity
sin2θ = 2sinθcosθ , then
sin2θ = 2 × \(\frac{11}{61}\) × \(\frac{60}{61}\) = \(\frac{1320}{3721}\)
this is a geo question i do not understand someone please help me