a turn consists of rolling a standard die and tossing a fair coin. the game is won when the die shows a $1$ or a $6$ and the coin shows heads. what is the probability the game will be won before the fourth turn? express your answer as a common fraction.
Answers
The probability that the game will be won before the fourth turn is \(\frac{91}{216}\).
What is probability?Probability refers to the possibility of the occurrence of an event.
P(E) = probability of occurrence of an event E = \(\frac{Number Of Favourable Outcomes}{Total Number Of Outcomes}\)
Now,
Probability of winning the game in first turn = \(\frac{1}{3}\frac{1}{2}=\frac{1}{6}\)
Probability of losing the first game but winning the second one = \(\frac{5}{6}\frac{1}{6} = \frac{5}{36}\)
Probability of losing the first two games but winning the third one = \(\frac{5}{6}(\frac{5}{6})\frac{1}{6} = \frac{25}{216}\)
Thus, the probability of winning the game before fourth turn = \(\frac{1}{6}+\frac{5}{36}+\frac{25}{216}=\frac{91}{216}\)
Hence, The probability that the game will be won before the fourth turn is \(\frac{91}{216}\).
To learn more about probability, refer to the link: https://brainly.com/question/25870256
#SPJ4
Related Questions
In a group of 304 people, the ratio of adult women to adult men is 3:4 and the ratio of adult men to children is 1:3. How many children are there
Answers
Answer:
192
Step-by-step explanation:


The number of successive win on a mobile phone game similar to Pokémon follows a Poisson distribution, with a mean of 27 wins per hour. Find the probability that there will be 90 or more wins in the next three hours of playing.
Answers
The probability of having 90 or more wins in the next three hours of playing is 0.0506 or 5.06% (approx).
We are required to find the probability of having 90 or more wins in the next three hours of playing a mobile phone game similar to Pokémon, given that the number of successive win follows a Poisson distribution with a mean of 27 wins per hour.
The given mean of Poisson distribution is λ = 27.
The Poisson distribution formula is:P(X = x) = (e^-λ λ^x) / x!
We need to calculate the probability of having 90 or more wins in 3 hours.
We can combine these three hours and treat them as one large interval, for which λ will be λ1 + λ2 + λ3= (27 wins/hour) * 3 hours= 81 wins.
P(X ≥ 90) = 1 - P(X < 90)
To calculate P(X < 90), we can use the Poisson distribution formula with λ = 81.P(X < 90) = Σ (e^-81 * 81^k) / k!, k = 0, 1, 2, ....89= 0.9494
Using the above formula and values, we get P(X ≥ 90) = 1 - P(X < 90)= 1 - 0.9494= 0.0506
Therefore, the probability of having 90 or more wins in the next three hours of playing is 0.0506 or 5.06% (approx).
Hence, the probability of having 90 or more wins in the next three hours of playing is 0.0506 or 5.06% (approx).
Learn more about probability
brainly.com/question/31828911
#SPJ11
A null hypothesis is that the mean nose lengths of men and women are the same. The alternative hypothesis is that women have a shorter mean nose length than men. Alpha for the problem is.05. A statistical test is done and the p-value is 0.04. Which of the following is the most appropriate way to state the conclusion?
Answers
The appropriate way to state the conclusion of the given statement is:
The results of the statistical test suggest that women have a shorter mean nose length than men (p = 0.04, α = 0.05).
Given:
A null hypothesis is that the mean nose lengths of men and women are the same.
The alternative hypothesis is that women have a shorter mean nose length than men.
Alpha for the problem is.05.
A statistical test is done and the p-value is 0.04.
Hence based on the condition we frame it as:
The results of the statistical test suggest that women have a shorter mean nose length than men (p = 0.04, α = 0.05).
Hence we get the required answer,
Learn more about Null hypothesis here:
brainly.com/question/15980493
#SPJ4
the area of the shaded region is $78$ square inches. all angles are right angles and all measurements are given in inches. what is the perimeter of the non-shaded region?
Answers
The perimeter of the non-shaded region in the figure can be calculated to be 14 inches.
Given a figure.
The middle portion of the figure is not shaded.
It is required to find the perimeter of the non-shaded region.
It is given that:
The whole area of the shaded region = 78 inches²
The area of the small square which is situated at the bottom is:
Area of small square = 2 × 4
= 8 inches²
So, the area of the rest of the shaded area = 78 - 8
= 70 inches²
Now, the area of the whole region without the small square is:
Area = 10 × (10 - 2)
= 80 inches²
So, the area of the non-shaded region = 80 - 70
= 10 inches²
The width of the non-shaded rectangle is 2 inches.
So, length = 5 inches.
So, the perimeter is:
P = 2(5 + 2)
= 14 inches
Hence, the perimeter is 14 inches.
Learn more about Area and Perimeter here :
https://brainly.com/question/11957651
#SPJ12
The figure is given below.

Express (x+3)^2 as a trinomial in standard form.
Answers
Answer:
x^2+6x+9
Step-by-step explanation:
(x+3)^2
(x+3)(x+3)
x^2+3x+3x+9
x^2+6x+9
Simplify the expression.
r8 (ra)-3 (write without negative exponent) =
Answers
Answer:
r^5/a^3
Step-by-step explanation:
r^8(ra)^-3
r^8/(ra)^3
r^8/r^3a^3
r^8-3/a^3
r^5/a^3
I’m stuck on this question

Answers
The accumulated amount after 25 years is , $70,702.80.
Now, We can use the formula for compound interest to find the accumulated amount after 25 years:
A = P(1 + r/k)^(kt)
Where A is the accumulated amount, P is the principal , r is the interest rate, n is the number of times the interest is compounded per year, and t is the time period.
In this case, we have:
P = $25,300
r = 0.045 (
k = 12 (monthly compounding)
t = 25
Substituting these values into the formula, we get:
A = $25,300(1 + 0.045/12)^(12 x 25)
A ≈ $70,702.80
Therefore, the accumulated amount after 25 years is ,
$70,702.80.
Learn more about the compound interest here:
brainly.com/question/26457073
#SPJ1
Bag with only red marbles and blue marbles.
The probability of randomly choosing a blue marble is 7 over 9
There are 45 marbles in total in the bag and each is equally
likely to be chosen.
Work out how many red marbles there must be.
Answers
Answer:
There are 35 red marbles
Please help! x+25=100
Answers
Help me please with the answer

Answers
Your selections are correct.
The coefficients of the x terms are opposites, so x will be eliminated when we add these two equations for both examples.
Find the value of x!

Answers
××1=× 123 =×/1= the value of x is one
Tell whether the p = 6 is a solution of p + 5 ≤ 12.
Answers
p + 5 ≤ 12
6 + 5 ≤ 12
11 ≤ 12
Since 11 is less than or equal to 12, the inequality is true when p = 6. Therefore, p = 6 is a solution of the inequality p + 5 ≤ 12
7. What is the slope of the line that passes through the pair of points (2, 5) and (8, 3)?
1/3
-1/3
3
-3
Answers
Answer:
The answer would be -1/3.
Step-by-step explanation:
If you plug the coordinates into slope formula (y 2 - y 1 )/ (x 2 - x 1) , you'd have 3-5 over 8-2, and once you solve that, you'll have -1/3 as your slope. Hope this helps ! :D
PLEASE MARK AS BRAINLIEST!! <3
if nm = 8x - 14 and jk = x squared + 1, find JK
Answers
A parallelogram in which adjacent sides are perpendicular to each other is called a rectangle. The length of Jk can be either 10 or 26.
What is a rectangle?A parallelogram in which adjacent sides are perpendicular to each other is called a rectangle. A rectangle is always a parallelogram and a quadrilateral but the reverse statement may or may not be true.
Since in a rectangle the opposite sides are equal, therefore, the sides NM and JK will be equal.
JK = MN
8x - 14 = x² + 1
0 = x² - 8x + 15
x = 5, 3
Hence, the length of Jk can be either 10 or 26.
The complete questions are:
Quadrilateral JKMN is a rectangle, if NM= 8x - 14 and JK= x squared + 1, find JK.
Learn more about Rectangle:
https://brainly.com/question/15019502
#SPJ1
A water balloon is catapulted into the air so that its height h, in meters, after t seconds is given by the equation h(t) = –4.9t² + 27t + 2.5.
Answers
1. The value of h(1) is 24.5 m
2. The maximum height of the balloon is 39.59 m.
3. The balloon burst after 5.5979 sec it hits the ground
What is Equation?Equations are mathematical statements with two algebraic expressions flanking the equals (=) sign on either side.
It demonstrates the equality of the relationship between the expressions printed on the left and right sides. LHS = RHS is a common mathematical formula.
Coefficients, variables, operators, constants, terms, expressions, and the equal to sign are some of the components of an equation. The "=" sign and terms on both sides must always be present when writing an equation.
Given:
h(t) = –4.9t² + 27t + 2.5.
1. For t= 1 hour
h(1) = –4.9(1)² + 27(1) + 2.5.
h(1) = 24.5m
2. Vertex formula (-b/2a, h(-b/2a))
Vertex = -27/ 2(4.9) = 2.755
So, h(2.755) = 39.59 m
3.–4.9t² + 27t + 2.5. = 0
solving the above equation for t we get
t= -0.877 sec
t = 5.5979 sec
Learn more about Equation here:
https://brainly.com/question/29657983
#SPJ1
The line that passes through the point (2, -8) and has a slope of -1 is given by the equation y+___=___(x-___).
Answers
Answer:
y + 8 = - 1(x - 2)
Step-by-step explanation:
The equation of a line in point- slope form is
y - b = m(x - a)
where m is the slope and (a, b) a point on the line
Here m = - 1 and (a, b) = (2, - 8) , thus
y - (- 8) = - 1 (x - 2) , that is
y + 8 = - (x - 2)
A= (a, b}
B = {1,2,3}
Select the expression that is an element of AxBxB.
a. (1,2,3)
b. (a, a,1)
c. (b,2^2)
d. (2.1.1)
Answers
The expression that is an element of AxBxB is (1,2,3)
The given data is A= (a, b}, B = {1,2,3}
The product of two matrices A and B is defined if the number of columns of A is equal to the number of rows of B. If both A and B are square matrices of the same order, then both AB and BA are defined.
\(& \ A \times B=\left\{\begin{array}{c}(a, 1),(a, 2),(a, 3),(b, 1) \\(b, 2),(b, 3)\end{array}\right. \\\)
\(& A \times B \times B=\left\{\begin{array}{l}(a, 1,1),(a, 1,2),(a, 1,3), \\(a, 2,1),(a, 2,2),(a, 2,3),\end{array}\right. \\& (a, 3,1),(a, 3,2),(a, 3,3) \\ & (b, 1,1),(b, 1,2),(b, 1,3) \\& \left.\begin{array}{l}(b, 2,1),(b, 2,2),(b, 2,3) \\(b, 3,1),(b, 2),(b, 3,3)\end{array}\right\} \\\)
\(& \therefore(b, 2,3) \in D \times B \times B \\\\& (a, a, 1) \notin A \times B \times B \\\\& \left(b, 2^2\right) \quad \forall A \times B \times B \\\\& (2,1,1) \notin A \times B \times B \\\\&=(b, 2,3) \in \cap \times B \times B \\&\end{aligned}\)
The union of two sets X and Y is equal to the set of elements that are present in set X, in set Y, or in both the sets X and Y.
The intersection of sets can be denoted using the symbol ‘∩’. As defined above, the intersection of two sets A and B is the set of all those elements which are common to both A and B. Symbolically, we can represent the intersection of A and B as A ∩ B.
Therefore, the expression that is an element of AxBxB is (1,2,3).
For more such questions on sets
https://brainly.com/question/28492445
#SPJ4
2.5x-1.5x=21 Solve for x:
Answers
Answer:
2.5x-1.5x=21
2.5x-1.5x=21
1x=21
x=21 ANSWER.
Step-by-step explanation:
MARK ME AS A BRAINLIST PLZ
\(\bold{Hello~!}\)
\(2.5x~-~1.5x~=21:~x = 21\)
If you want to solve for x ? EXPLANATION :\(\sf{Add~similar~elements:~2.5x~ -~ 1.5x = x\)
\(\sf{x = 21}\)
Hope It Helps!
#LearnWithBrainly
\(\underline{Answer~:}\)
\(\sf{Miwsow}\)
Please, follow me. and like of my answers ! And, Brainliest is Appreciate !
\(2\sqrt} 5+3\sqrt} 75\)
Answers
Explanation
2x10 + 3x150
20 + 450
470
For a moving object, the force acting on the object varies directly with the object's acceleration. When a force of 90 N acts on a certain object, the acceleration of the object is 10 m/s² . If the force is changed to 81N , what will be the acceleration of the object?
Answers
Answer:
9 1/10th m/s2 :)
Step-by-step explanation:
The reason it is 1/10 is because the Number is 81 not 80, therefore 1/10 because there it is 1 left in 80 making it a fraction not a whole :) i hope this helps sorry if the explanation is too complicated...
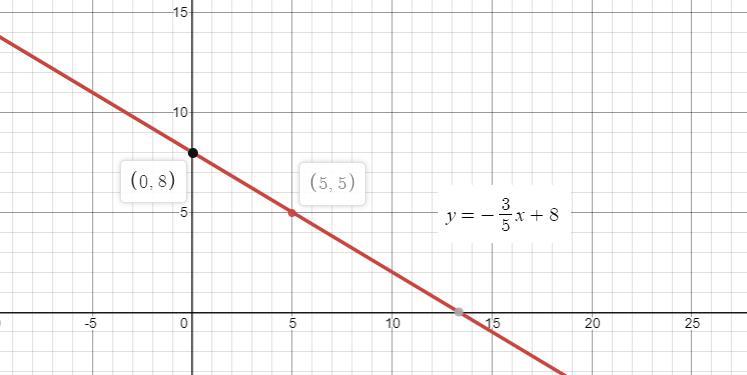
what is the answer?
Answers
The given function is-
\(y=-\frac{3}{5}x+8\)To graph this linear function, we just need two points. Let's find the corresponding values of y when x = 0 and x = 5.
For x = 0.
\(y=-\frac{3}{5}(0)+8=8\)So, the first point is (0, 8).
For x = 5.
\(y=-\frac{3}{5}(5)+8=-3+8=5\)The second point is (5, 5).
Then, we graph these points to draw a straight line through them.
The image below shows the graph.
two garden beds are shown. the perimeters of the two gardens are equal.
Part A
write an equation that sets the perimeter equals then solve the equation.
Part B
the side length of a garden cannot be a negative number or zero. what value(s) of X make the equation you wrote in the first part true in this context if this problem

Answers
The perimeter are given by the sum of the expressions of the lengths of
the sides which are each equal to 4·x + 6.
Response:
Part A
The equation of the perimeters is; 4·x + 6 = 4·x + 6The solution is; x = xPart B
The values of x are; 0 < x < ∞Which methods can be used to evaluate the given figures?Part A
Given parameters;
Perimeter of the rectangular garden = Perimeter of the triangular garden
Therefore;
Perimeter of the rectangular garden = 2 × (x + 2) + 2 × (x + 1) = 4·x + 6
Perimeter of the triangular garden = x + 3 + 2·x + 1 + x + 2 = 4·x + 6
The equation that sets the perimeters equal is therefore;
4·x + 6 = 4·x + 6
The solution of the above equation is therefore;
x = x
Part B
The values of x that make the first part of the equation true are;
0 < x < ∞Learn more about the perimeter of geometric figures here:
https://brainly.com/question/2122650
x⁴+8x³+34x²+72x+81 factories it.
Answers
Answer:
The expression x⁴ + 8x³ + 34x² + 72x + 81 cannot be factored further using simple integer coefficients. It does not have any rational roots or easy factorizations. Therefore, it remains as an irreducible polynomial.
t ÷ 16 = 80 what is t ?
Answers
Answer:
T=1280
Step-by-step explanation:
T / 16=80
multiply 80 by 16
t= 1280
so if we divide 1280 by 16 we get 80
Answer:
\( \longmapsto \sf \: t \div 16 = 80\)
\( \longmapsto \sf \: t = 80 \times 60\)
\( \longmapsto \sf \: t = 1280\)
Select the correct answer.
The graph of function f is shown.
Function g is represented by the table.
x -1 0 1 2 3
g(x) 15 3 0
Which statement correctly compares the two functions?
A.
They have the same x- and y-intercepts.
B.
They have the same y-intercept and the same end behavior as x approaches ∞.
C.
They have different x- and y-intercepts but the same end behavior as x approaches ∞.
D.
They have the same x-intercept but different end behavior as x approaches ∞.
Answers
Answer:
The answer will be A
hope this helps :)
Find the slope of the line on the graph. Reduce all fractional answers to lowest terms.
-4. -1. -2

Answers
Answer:
-1
Step-by-step explanation:
Please help me with this !!!

Answers
Answer:
Step-by-step explanation:
I assume that QP = 18 cm and m∠Q = 72°

Answer:
\(\fbox {P = 6.76 cm}\)
Step-by-step explanation:
Finding the missing angle :
22° + ∠R + 72° = 180°∠R = 180° - 94°∠R = 86°Applying the Law of Sines :
\(\boxed {\frac{sin P}{P} = \frac{sin R}{PQ}}\)
Substituting the known values :
sin 22° / P = sin 86° / 18P = sin 22° × 18 / sin 86°P = 0.374606593 × 18 / 0.99756405P = 6.76 cmFive years ago, someone used her $40,000 saving to make a down payment for a townhouse in RTP. The house is a three-bedroom townhouse and sold for $200,000 when she bought it. After paying down payment, she financed the house by borrowing a 30-year mortgage. Mortgage interest rate is 4.25%. Right after closing, she rent out the house for $1,800 per month. In addition to mortgage payment and rent revenue, she listed the following information so as to figure out investment return: 1. HOA fee is $75 per month and due at end of each year 2. Property tax and insurance together are 3% of house value 3. She has to pay 10% of rent revenue for an agent who manages her renting regularly 4. Her personal income tax rate is 20%. While rent revenue is taxable, the mortgage interest is tax deductible. She has to make the mortgage amortization table to figure out how much interest she paid each year 5. In last five years, the market value of the house has increased by 4.8% per year 6. If she wants to sell the house today, the total transaction cost will be 5% of selling price Given the above information, please calculate the internal rate of return (IRR) of this investment in house
Can you show the math as far as formulas go?
Answers
Given the following information: Five years ago, someone used her $40,000 saving to make a down payment for a townhouse in RTP. The house is a three-bedroom townhouse and sold for $200,000 when she bought it. After paying down payment, she financed the house by borrowing a 30-year mortgage.
Mortgage interest rate is 4.25%. Right after closing, she rent out the house for $1,800 per month. In addition to mortgage payment and rent revenue, she listed the following information so as to figure out investment return: 1. HOA fee is $75 per month and due at end of each year 2. Property tax and insurance together are 3% of house value 3. She has to pay 10% of rent revenue for an agent who manages her renting regularly 4. Her personal income tax rate is 20%. While rent revenue is taxable, the mortgage interest is tax deductible. She has to make the mortgage amortization table to figure out how much interest she paid each year 5. In the last five years, the market value of the house has increased by 4.8% per year 6.
To know more about interest visit:
https://brainly.com/question/30393144
#SPJ11
What is the area of the trapezoid

Answers
The area of the Trapezium given in the question is 28ft²
The area of a trapezium is calculated using the relation :
Area = h/2(b1 + b2)Using the parameters given for our compuation:
height, h = 4
b1 = 9
b2 = 5
Inputting the parameters into our formula :
Area = 4/2(5 + 9)
Area = 2(14)
Area = 28ft²
Therefore, the area of the Trapezium is 28ft²
Learn more on trapezium :https://brainly.com/question/30042904
#SPJ1
Write the equation of a line that passes the point (-6,9)and is perpendicular to a line that passes through the points (-2,1) and (6,7).
Answers
Answer:
y = 4/3x + 1.
Step-by-step explanation:
The equation of the lines passing through (2,1) and (6,7) is:
y - 7 = (7-1)/(6- -2)(x - 6)
y - 7 = 6/8(x - 6)
y = 3/4(x - 6) + 7
y = 3/4x + 5/2.
So the line perpendicular to it has slope -1/3/4 = -4/3:
y - 9 = -4/3(x + 6)
y = -4/3 x - 8 + 9
y = 4/3x + 1.