A math teacher gave her class two tests. The results were 42 percent of the class passed the first test and 50 percent of the class passed thesecond test. Also, 60 percent of the students in the class who passed the first test passed the second test. What percentage of the class passed both tests? Are the events the class passed the first test and the class passed the second test independent? (1 point)
A) A total of 30 percent passed both tests. The events are independent.
B) A total of 25. 2 percent passed both tests. The events are independent.
C) A total of 25. 2 percent passed both tests. The events are not independent.
D) A total of 30 percent passed both tests. The events are not independent.
Answers
The answer is option C) A total of 25.2 percent passed both tests. The events are not independent.
To solve this problem, we can use a Venn diagram. Let P(A) be the probability of passing the first test, P(B) be the probability of passing the second test, and P(A and B) be the probability of passing both tests.
From the problem, we know:
P(A) = 0.42
P(B) = 0.50
P(B | A) = 0.60 (the probability of passing the second test given that the student passed the first test)
We can use the formula P(B | A) = P(A and B) / P(A) to find P(A and B):
0.60 = P(A and B) / 0.42
P(A and B) = 0.60 x 0.42
P(A and B) = 0.252
Therefore, 25.2% of the class passed both tests.
To determine if the events are independent, we can compare P(B) to P(B | A). If they are equal, the events are independent. If they are not equal, the events are dependent.
P(B) = 0.50
P(B | A) = 0.60
Since P(B) is not equal to P(B | A), the events are dependent.
Therefore, the answer is option C) A total of 25.2 percent passed both tests. The events are not independent.
To know more about probability refer here:
https://brainly.com/question/11234923
#SPJ11
Related Questions
Figure ABCD is dilated from point Q, the center of dilation.
Which figure is a dilation of figure ABCD?

Answers
Therefore , the solution of the given problem of quadrilateral comes out to be EFHG is dilation of figure ABCD.
What is quadrilateral ?A quadrilateral is a four-sided shape with four corners that is used in mathematics. The term is either derived from the Latin words "quad" or "portfolio of inventive (meaning "side"). The three factors of a rectangle are four corners, four regions, and four corners. The two main types of concave and convex shapes are convex and concave. The subcategories of trapezoids, rectangular shapes, angles, rhombuses, but also squares are also considered to be convex quadrilaterals.
Here,
A transition that changes a figure's size is a dilation. Each point's coordinates are multiplied by a scale factor, which is a fixed value higher than zero, to achieve this.
A magnification occurs when the scale factor is higher than 1; a reduction occurs when the scale factor is between 0 and 1. (a reduction). The spot around which the figure is resized is the centre of dilation.
Figure ABCD is enlarged from position Q in this instance.
This indicates that the corresponding point in the dilated figure is created by multiplying each point in EFHG by a scale factor.
The amount of scaling is determined by the scale factor. The dilated figure will be bigger than ABCD if the scale factor is greater than 1, and smaller than ABCD if the scale factor is between 0 and 1.
So, EFHG is dilation of figure ABCD.
To know more about quadrilateral visit:
https://brainly.com/question/29934291
#SPJ1
ak-r=on, solve for k
Answers
Answer:
U need to copy and paste more of the question
Step-by-step explanation:
Start copy at the top go all the way to the bottem of the question and paste into brainly.
use ctrl c for copy and crtl v for paste
A classmate simplified a rational equation 1-2/x-2 = x+1/X+2 1 - 2/x-2 = x+1/X+2
A): Explain the error in this simplification.
B): Show your work as you correct this error.
Answers
A rational equation is defined as the quotient of two polynomials, with the denominator not being zero. It is a type of algebraic expression that involves variables and rational functions with rational exponents.
The error in the given rational equation is that it's not allowed to cancel out the x-2 and X+2 terms, as they are in the denominators. It is because of the fact that it may make the denominator zero, which would result in undefined expressions. Therefore, we need to find the least common multiple (LCM) of the denominators and then convert the equation into equivalent forms with the same denominator.B):
Correction of the error1-2/x-2 = x+1/X+2Given rational equation can be re-written as:((1 - 2)/(x - 2)) = ((x + 1)/(X + 2))The LCM of x - 2 and X + 2 is (x - 2) (X + 2).Multiplying the numerator and denominator of the left side by (X + 2) and the numerator and denominator of the right side by (x - 2) we get,(1 - 2)(X + 2) = (x + 1) (x - 2)Now simplify the equation:2X - 3x = -3The given rational equation becomes 2X - 3x = -3 after correction.Note: You should always double-check if the obtained solution satisfies the initial equation.
To know more about consecutive integers visit:
https://brainly.com/question/1767889
#SPJ11
Suppose there are 145 units of a substance at t= 0 days, and 131 units at t = 5 days If the amount decreases exponentially, the amount present will be half the starting amount at t = days (round your answer to the nearest whole number) The amount left after t = 8 days will be units (round your answer to the nearest whole number).
Answers
The amount left after t = 8 days will be approximately 53 units, if the amount has exponential decay.
To solve this problem, we can use the formula for exponential decay:
N(t) = N₀ * e^(-kt),
where:
N(t) is the amount of substance at time t,
N₀ is the initial amount of substance,
e is the base of the natural logarithm (approximately 2.71828),
k is the decay constant.
We can use the given information to find the value of k first. Given that there are 145 units at t = 0 days and 131 units at t = 5 days, we can set up the following equation:
131 = 145 * e^(-5k).
Solving this equation for k:
e^(-5k) = 131/145,
-5k = ln(131/145),
k = ln(131/145) / -5.
Now we can calculate the amount of substance at t = 8 days. Using the formula:
N(8) = N₀ * e^(-kt),
N(8) = 145 * e^(-8 * ln(131/145) / -5).
To find the amount left after t = 8 days, we divide N(8) by 2:
Amount left after t = 8 days = N(8) / 2.
Let's calculate it:
k = ln(131/145) / -5
k ≈ -0.043014
N(8) = 145 * e^(-8 * (-0.043014))
N(8) ≈ 106.35
Amount left after t = 8 days = 106.35 / 2 ≈ 53 (rounded to the nearest whole number).
To know more about Exponential decay refer-
https://brainly.com/question/13674608#
#SPJ11
i need help somebody please

Answers
Answer:
\(3y + 3y - 12\)
Step-by-step explanation:
This is because if you were to combine like terms, the two beginning values would add up to 6y since adding two y's is just y & 3+3 is 6. & 12 is common sense lol.
Dana has to pay 15% tax on any income above £10,500 per year. Dana is paid £24,000 annually. Calculate the amount of money she receives after tax each month.
Answers
Answer:
0.4375
Step-by-step explanation:
u divided 10,500 and 24,000 and u will get 0.4375
If the sum of an infinite geometric series is \( \frac{15625}{24} \) and the common ratio is \( \frac{1}{25} \), determine the first term. Select one: a. 625 b. 3125 c. 25 d. 125
Answers
The first term of the infinite geometric series is 625.Let's dive deeper into the explanation.
We are given that the sum of the infinite geometric series is \(\( \frac{15625}{24} \)\)and the common ratio is\(\( \frac{1}{25} \).\)The formula for the sum of an infinite geometric series is \(\( S = \frac{a}{1 - r} \)\), where \( a \) is the first term and \( r \) is the common ratio.
Substituting the given values into the formula, we have \(\( \frac{15625}{24} = \frac{a}{1 - \frac{1}{25}} \).\)To find the value of \( a \), we need to isolate it on one side of the equation.
To do this, we can simplify the denominator on the right-hand side.\(\( 1 - \frac{1}{25} = \frac{25}{25} - \frac{1}{25} = \frac{24}{25} \).\)
Now, we have \(\( \frac{15625}{24} = \frac{a}{\frac{24}{25}} \).\) To divide by a fraction, we multiply by its reciprocal. So, we can rewrite the equation as \( \frac{15625}{24} \times\(\frac{25}{24} = a \).\)
Simplifying the right-hand side of the equation, we get \(\( \frac{625}{1} = a \).\)Therefore, the first term of the infinite geometric series is 625.
In conclusion, the first term of the given infinite geometric series is 625, which corresponds to option (a).
learn more about geometric series here here
https://brainly.com/question/30264021
#SPJ11
Write an equation of the line with a slope of −27 and a y-intercept of 5.
An equation is y= __
Answers
Answer: -9
Step-by-step explanation:
m=1
b=5
slope=1
y=(0,5)
The equation of the line is y = -27x + 5 with a slope of −27 and a y-intercept of 5.
What is a linear equation?It is defined as the relation between two variables, if we plot the graph of the linear equation we will get a straight line.
If in the linear equation, one variable is present, then the equation is known as the linear equation in one variable.
It is given that:
The slope of −27 and a y-intercept of 5.
As we know,
The slope-intercept form of the line is:
y = mx + c
m = -27
c = 5
y = -27x + 5
The equation is y = -27x + 5
Thus, the equation of the line is y = -27x + 5 with a slope of −27 and a y-intercept of 5.
Learn more about the linear equation here:
brainly.com/question/11897796
#SPJ2
Write the ratio in its simplest form
400 g: 2 kg
Answers
Answer: divide them both by 2 and you get 200:1
Step-by-step explanation:
Translate the following verbal expression into an algebraic expression ... "The quotient of a number and four decreased by five"
Answers
Answer:
n/(4-5)
Step-by-step explanation:
A quotient of a number and... means that the number is being divided by whatever comes after. In this case the number that comes after is '4 decreased by 5', which is 4 - 5 as the -5 takes 5 away so it 'decreases it by 5'. Therefore is the expression is 'the quotient of a number and four decreased by five' then it is expressed in the following way (using n for number):
n/(4-5)
Hope this helped!
-3x^2-9x+357=9x solve by completing the square
Answers
Answer:
\(x_1 = -3 + 8\sqrt{2} = 8.3137\\\\x_1 = -3 - 8\sqrt{2} = -14.3137\\\)
Step-by-step explanation:
\(-3x^2-9x+357=9x\\\\\mathrm{Move}\:357\:\mathrm{to\:the\:right\:side}\\\\-3x^2-18x=-357\\\\\mathrm{Divide\:both\:sides\:by\:}-3\\\\\dfrac{-3x^2-18x}{-3}=\dfrac{-357}{-3}\\\\x^2+6x=119\)
\(\mathrm{Rewrite\:in\:the\:form}\:\left(x+a\right)^2=b\)
\(\left(x+a\right)^2=x^2+2ax+a^2\)
\(\mathrm{Rewrite\:in\:the\:form}\:x^2+2ax+a^2\\\\\mathrm{Comparing terms }2ax=6x\\\\a=3\\\\\mathrm{Add\:}a^2=3^2\mathrm{\:to\:both\:sides}\\\\x^2+6x+3^2=119+3^2\\\\\left(x+3\right)^2=128\\\\\)
\(\mathrm{Taking square roots on either side:}\\\\(x + 3) = \pm \sqrt{128}\\\\x = -3 \pm \sqrt{128}\)
\(128 = 64 \cdot 2\\\\\sqrt{128} = \sqrt{64} \cdot \sqrt{2}\\\\= 8\sqrt{2}\)
So the two roots are
\(x_1 = -3 + 8\sqrt{2} = 8.3137\\\\x_1 = -3 - 8\sqrt{2} = -14.3137\\\)
factoring integers with sublinear resources on a superconducting quantum processor
Answers
Factoring integers with sublinear resources on a superconducting quantum processor can be achieved using quantum approximate optimization algorithm" (QAOA) or quantum phase estimation algorithm (QPE) approach.
Factoring integers with sublinear resources on a superconducting quantum processorFactoring large integers is a problem of significant importance in the field of cryptography.
Classical computers have limited efficiency in solving this problem, and their performance is expected to decline further as the size of the integers to be factored increases.
In contrast, quantum computers are expected to have exponential speedup in factoring large integers, thanks to Shor's algorithm.
Recently, researchers have been exploring the possibility of using superconducting quantum processors to factor integers with sublinear resources.
One approach is to use a so-called "quantum approximate optimization algorithm" (QAOA), which maps the problem of factoring integers to a problem of finding the ground state of a certain Hamiltonian.
The QAOA can be implemented using a superconducting quantum processor, with the problem Hamiltonian implemented by a set of two-qubit gates.
Another approach is to use the quantum phase estimation algorithm (QPE) to estimate the eigenvalues of the problem Hamiltonian.
This can be used to factor integers by finding the period of a certain function using the quantum Fourier transform, as in Shor's algorithm. QPE can be implemented on a superconducting quantum processor using phase estimation circuits, which require only polynomial resources.
Learn more about superconducting quantum processors on:
brainly.com/question/29235650
#SPJ1
the determinant of a is the product of the pivots in any echelon form u of a, multiplied by (1)r, where r is the number of row interchanges made during row reduction from a to u.
Answers
The determinant of a is the product of the pivots in any echelon form u of a, multiplied by (1)r, where r is the number of row interchanges made during row reduction from a to u is False.
Given:
The determinant of a is the product of the pivots in any echelon form u of a, multiplied by (1)r, where r is the number of row interchanges made during row reduction from a to u.
Definition of det A:
det A = (-1)^r * (product of pivots in echelon form) if A is invertible or 0 when A is not invertible.
From definition of det A we can simply say the given statement is false - This can only hold if A is an invertible matrix, but the problem does not state this.
Reduction to an echelon form may also include scaling a row by a nonzero constant, which can change the value of the determinant.
Therefore the determinant of a is the product of the pivots in any echelon form u of a, multiplied by (1)r, where r is the number of row interchanges made during row reduction from a to u is False.
Learn more about the determinant and pivots here:
https://brainly.com/question/17409182
#SPJ1
HELP ME!! FIND X AND Y.

Answers
Answer:
x = 26
y = 72°
Step-by-step explanation:
Finding x:Alternates angles are equal.(4x - 16)° = (3x + 10)°
Subtract 3x to both sides
4x - 3x - 16 = 10
x - 16 = 10
Add 16 to both sides
x = 10 + 16
x = 26
Finding y:Angles on a straight lines add up to 180 degrees.(4x - 16)° + y° = 180°
4x - 16 + y = 180
4(26) - 16 + y = 180
104 - 16 + y = 180
88 + y = 180
Subtract 88 to both sides
y = 180 - 88
y = 72°
\(\rule[225]{225}{2}\)
This shows a figure.
What is the value of x?
57
63
86
95

Answers
Answer:
57
Step-by-step explanation:
Straight line is 180 degrees
x+2x+9=180
3x=171
x=171/3
x=57
pls mark as brainliest
A web music store offers two versions of a popular song. the size of the standard version is 2.3 mb. the size of the high quality version is 4.8 mb. yesterday there were 1460 downloads of the song for a total of download size of 5458 mb how many downloads of the high quality version where there
Answers
The number of downloads of the high quality version that was made was 600.
Calculations and Parameters
This is a great system of equations problem
If we call the number of standard version downloads (S)
The high quality downloads (H).
For number of songs downloaded: S + H = 910For download size: 2.8(S) + 4.4(H) = 3044.To find S, we can rearrange the first statement to H = 910 - S,
Then we would substitute (910 - S) wherever you see an H in the second equation so that you have only S's in your equation.
Thus:
2.8(S) + 4.4(910 - S) = 3044
2.8S + 4004 - 4.4S = 3044
-1.6S = -960
s = 600
Read more about systems of equations here:
https://brainly.com/question/14323743
#SPJ4
what is the area of this figure

Answers
Answer:
478km2
Step-by-step explanation:
(13km×25km)+[11km×(7km+4km)]+(8km×4km)
=325km2+121km2+32km2
=478km2
hope this helps
Britney’s weight is 5\6 of Joan’s weight. If the two girls together weight a total of 121 kg, how much does Britney weigh alone?
Answers
Britney weighs 55 kg alone.
Let's start by setting up an equation using the information given in the question.
Let B be Britney's weight and J be Joan's weight.
We know that Britney's weight is 5/6 of Joan's weight, so we can write:
B = (5/6)J
We also know that the two girls together weigh a total of 121 kg, so we can write:
B + J = 121
Now we can substitute the first equation into the second equation to solve for B:
(5/6)J + J = 121
Multiplying both sides by 6 to get rid of the fraction:
5J + 6J = 726
11J = 726
Dividing both sides by 11:
J = 66
Now we can use the first equation to find Britney's weight:
B = (5/6)J = (5/6)66 = 55
Find out more about equation
brainly.com/question/14269995
#SPJ11
a combination of values that, when arranged correctly, result in a final value.
Answers
Combinations and sequences are widely used in mathematics to explain and assess situations in which the order or selection of components might influence the final result.
What is combination?A combination in mathematics is a selection of elements from a set with distinct members, where the order of selection is irrelevant. A combination is a mathematical approach for determining the number of potential arrangements in a set of objects when the order of the selection is irrelevant. You can choose the components in any order in combinations. A combination is a method of picking elements from a collection when the order of selection is irrelevant. Assume we have three integers P, Q, and R. Combination defines how many possibilities we may choose two numbers from each set.
Here,
A combination is a grouping of things from a bigger set in which the order of the components is irrelevant. A sequence is a collection of elements that are ordered in a precise order. Combinations and sequences are frequently used in mathematics to describe and evaluate situations where the order or selection of components might impact the final outcome, such as in combinatorics, probability, and statistics.
To know more about combination,
https://brainly.com/question/20211959
#SPJ4
Complete question:
A combination of values that, when arranged correctly, result in a final value. true/false
Please help me with this question so I can better help my son to understand this better. I have attached the graph that were working from below? The graph shows f(x)and its transformation g(x).Enter the equation for g(x) in the box.g(x) =
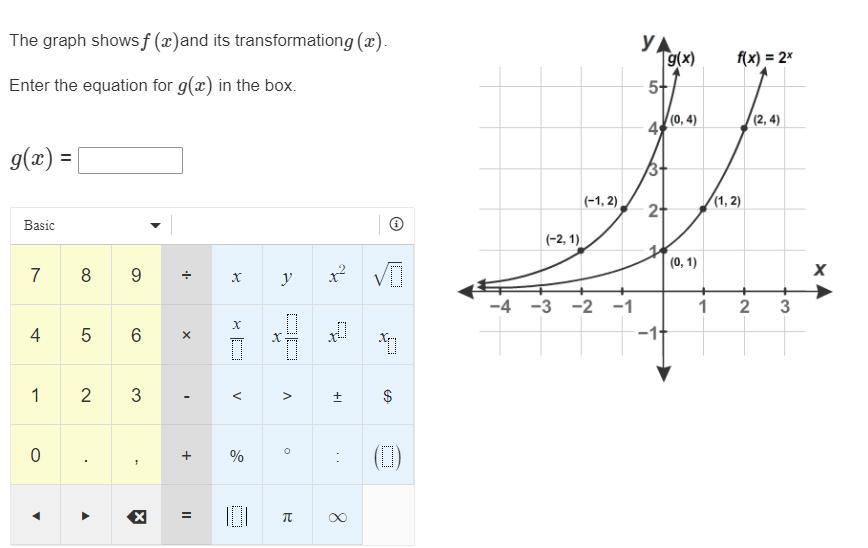
Answers
Answer
g(x) = 2ˣ⁺² or 2^(x + 2)
\(g(x)=2^{x+2}\)
Explanation
When a function f(x) is translated horizontally along the x-axis by a units, the new function is represented as
f(x + a) when the translation is by a units to the left.
f(x - a) when the translation is by a units to the right.
Looking at the coordinates of the points given on f(x) and g(x), we can see that g(x) is just f(x) translate 2 units to the left.
Hence, if f(x) = 2ˣ
g(x) = f(x + 2) = 2ˣ⁺² or 2^(x + 2)
Hope this Helps!!!
1.
a) Carol and Conrad together have less than $50. Carol has $10 more than Conrad how much money might Conrad have.
b) How much money might Carol have
Answers
B)$25
They both add up less than $50, and there is many other ways of what it could be
find a particular solution to the nonhomogeneous differential equation y′′ 4y′ 5y=10x 5e−x.
Answers
y_p(t) = 2 - e^(-t) + te^(-t)
How to find particular solution?
To find a particular solution, we can use the method of undetermined coefficients.
First, complementary solution is y_c(t) = e^(-2t)(c1 cos(t) + c2 sin(t)).
Next, we can guess that the particular solution has the form:
y_p(t) = (a0 + a1t) + (b0 + b1t)e^(-t)
where a0, a1, b0, and b1 are constants that we need to determine.
Taking the first and second derivatives of y_p(t), we get:
y'_p(t) = a1 - b1e^(-t)
y''_p(t) = b1e^(-t)
Substituting y_p(t), y'_p(t), and y''_p(t) into the nonhomogeneous equation, we get:
b1e^(-t) + 4(a1 - b1e^(-t)) + 5(a0 + a1t + b0e^(-t) + b1te^(-t)) = 10x + 5e^(-x)
Simplifying the left-hand side and equating the coefficients of like terms on both sides, we get the following system of equations:
a1 - 4b1 + 5b1 = 0 (coefficient of e^(-t))
-4a1 + 5a0 + 5b0 = 10 (coefficient of 1)
5a1 + 5b1 = 5 (coefficient of te^(-t))
-5b1 = 5e^(-x) (coefficient of e^(-x))
a1 = 0 (coefficient of x)
Solving for the constants, we get:
a1 = 0
b1 = -e^(x)
a0 = 2
b0 = 0
Therefore, the particular solution to the nonhomogeneous differential equation is:
y_p(t) = 2 - e^(-t) + te^(-t)
Learn more about differential equation
brainly.com/question/14620493
#SPJ11
A set of n = 25 pairs of scores (x and y values) has a pearson correlation of r = 0. 80. how much of the variance for the y scores is predicted by its relationship with x?
Answers
The amount of variance for y that is predicted by its relationship with x is 64%.
How much variance is predicted?
Variance measures the rate of dispersion of a data point around the dataset. It can be calculated by finding the square of the standard deviation of a dataset. Variance measures the variation of a data set.
Correlation is a statistical measure used to measure the linear relationship that exists between two variables. The greater the correlation coefficient is closer to one, the greater the linear relationship that exists between the two variables. A positive correlation occurs when the two variables move in the same direction.
Variance = (correlation coefficient²) x 100
(0.80²) x 100
0.64 x 100 = 64%
To learn more about correlation, please check: https://brainly.com/question/27246345
#SPJ1
63+ (2x+8)+(x + 7) = 180
Answers
63+ (2x+8)+(x + 7) = 180
63+3x+15=180
78+3x=180
3x=102
x=34
Hey there!
63 + (2x + 8) + (x + 7) = 180
DROP the PARENTHESES:
63 + 2x + 8 + x + 7 = 180
CONVERT TO:
63 + 2x + 8 + 1x + 7 = 180
COMBINE the LIKE TERMS 1:
71 + 3x + 7 = 180
COMBINE the LIKE TERMS 2:
78 + 3x = 180
3x + 78 = 180
SUBTRACT 78 to BOTH SIDES:
3x + 78 - 78 = 180 - 78
SIMPLIFY IT:
3x = 180 - 78
3x = 102
DIVIDE 3 to BOTH SIDES:
3x/3 = 102/3
SIMPLIFY IT:
x = 102/3
x = 34
Therefore, your answer should be:
x = 34
Good luck on your assignment & enjoy your day day!
~Amphitrite1040:)
wich statements are true about boundary classes in object-oriented analysis?
Answers
The statements that are true about object-oriented analysis are: b) Control classes receive or handle system events, c) encapsulate behavior of use case, d) implement the controller pattern.
Object-oriented analysis and design (OOAD) is a technical method for analyzing and planning an application, system, or business using object-oriented programming and visual modeling to direct stakeholder communication and product quality throughout the software development process.
Modern software engineering practices typically involve iterative, incremental OOAD. Analysis models for OOA and design models for OOD are the byproducts of OOAD activities, respectively. The goal is for these to continuously improve and change, guided by important factors like risks and business value.
To learn more about object-oriented analysis: https://brainly.com/question/30551374
#SPJ4
Note that the full question is:
Which statements are true about control classes in object-oriented analysis? Select all that apply.
a) Control classes manage data structures.
b) Control classes receive or handle system events.
c) Control classes encapsulate behavior of use case.
d) Control classes implement the controller pattern.
Seth's family plans to drive 220 220 miles to their vacation spot. They would like to complete the drive in 4 4 hours. Find the average speed in miles per hour needed to make the trip in the desired time.
Answers
Answer: 55 miles per hour
Step-by-step explanation:
Here is the complete question:
Seth's family plans to drive 220 miles to their vacation spot. They would like to complete the drive in 4 hours.
Find the average speed in miles per hour needed to make the trip in the desired.
From the question, Seth's family plans to drive 220 miles to their vacation spot and they would like to complete the drive in 4 hours.
Average speed is calculated as distance travelled divided by the time taken. This will be:
Speed = Distance/Time
Average speed = 220/4
Average speed = 55 miles per hour
OMG HELP NOW PLZZ <3

Answers
Answer:
I think it would be Maxine's, since they did more tests.
X + 5 < -2 help me please
Answers
Answer:
x<-7
Step-by-step explanation:
subtract 5 from both sides
Answer:x<-7 I hope this helpful mark the other guy brainlist
Step-by-step explanation:
x+5<-2
Move the constant to the right-hand side and change its sign
x<-2-5
Calculate the difference or subtract 5 from both sides
x<-7
Logan is taking a loan out to buy a $4000 right for his wife. He has two finance options listed below. Which should he choose? Option a- a five year loan with a %7 interest rate compounded quarterly l. Option b- an eight year loan with a 5.5% interest rate compounded annually
Answers
Answer:
The most convenient option for Logan is A, since he will pay a lower amount than if he chose option B.
Step-by-step explanation:
Given that Logan is taking a loan out to buy a $ 4000 right for his wife, and he has two finance options, Option A, which gives a five year loan with a% 7 interest rate compounded quarterly, and Option B, which gives an eight year loan with a 5.5% interest rate compounded annually, to determine which option should be chosen, the following calculation must be performed:
Option A:
X = 4,000 (1 + 0.07 / 3) ^ 5x3
X = 5,653.49
Option B:
X = 4,000 (1 + 5.5 / 1) ^ 8x1
X = 6,138.75
Thus, the most convenient option for Logan is A, since he will pay a lower amount than if he chose option B.
When it was first started, the Clinton High Cooking Club had 101010 members. Each year after the club started, the number of members increased by a factor of approximately 1.21.21, point, 2.
Answers
After one year, the club had approximately 123123 members. After two years, the club had approximately 149149 members. After three years, the club had approximately 180180 members.To calculate the number of members after three years, multiply the initial number of members (101010) by the factor of 1.21.21 three times
1. To calculate the number of members after one year, multiply the initial number of members (101010) by the factor of 1.21.21:
101010 x 1.21.21 = 12312
2. To calculate the number of members after two years, multiply the initial number of members (101010) by the factor of 1.21.21 twice:
101010 x 1.21.21 x 1.21.21 = 149149
3. To calculate the number of members after three years, multiply the initial number of members (101010) by the factor of 1.21.21 three times:
101010 x 1.21.21 x 1.21.21 x 1.21.21 = 180180
After one year, the club had approximately 123123 members. After two years, the club had approximately 149149 members. After three years, the club had approximately 180180 members.
Learn more about number here
https://brainly.com/question/10547079
#SPJ4