A function is defined by f(x)= x/4. What is f(-8)?
- 2
- -1/2
- 1/2
- 2
Answers
Answer:
need this as quick as possible pls
Step-by-step explanation:
Answer:
\(f(-8)=-2\)
Step-by-step explanation:
We have the function:
\(f(x)=\frac{x}{4}\)
And we want to find f(-8).
So, simply substitute -8 for x. This yields:
\(f(-8)=\frac{-8}{2}\)
Divide:
\(f(-8)=-2\)
Hence, our answer is -2.
Related Questions
henry subracts 16 from it and gets an answer of 13.9 what was the orginal number
Answers
Answer:
29.9 is the answer for that question
Answer:
the original number would have been 29.9
Step-by-step explanation:
13.9+16=29.9
For the following set of coupled differential equations: dx/dt= 2.x1 +x2
Answers
The solution to the coupled differential equations dx/dt= 2.x1 +x2 is x1 = t^2 + 2t and x2 = t^2, We can then integrate both sides of the equation to get the following: ln(2.x1 +x2) = t + c
To solve this set of differential equations, we can use the method of separation of variables. This method involves separating the variables in each equation so that they can be solved independently. In this case, we can separate the variables as follows: dx/(2.x1 +x2) = dt
We can then integrate both sides of the equation to get the following: ln(2.x1 +x2) = t + c
where c is an arbitrary constant. We can then exponentiate both sides of the equation to get the following 2.x1 +x2 = e^t.e^c
We can then substitute the initial conditions into this equation to get the following 2.x1 +x2 = e^t.1
where x1(0) = 0 and x2(0) = 0. This gives us the following solution for x1 and x2 x1 = t^2 + 2t and x2 = t^2
Here are some additional explanations:
The method of separation of variables is a general method for solving differential equations. It can be used to solve a wide variety of differential equations, including coupled differential equations.The initial conditions are the values of x1 and x2 at time t = 0. In this case, the initial conditions are x1(0) = 0 and x2(0) = 0.The solution to the coupled differential equations is x1 = t^2 + 2t and x2 = t^2. This solution can be verified by substituting it back into the differential equations.To know more about variable click here
brainly.com/question/2466865
#SPJ11
number of solutions of quadratic equations

Answers
Answer:
Discriminant = 120
Number of x intercepts = 2
=========================================================
Explanation:
The equation is of the form ax^2+bx+c with:
a = -7
b = 8
c = 2
The discriminant is
d = b^2 - 4ac
d = 8^2 - 4(-7)(2)
d = 64 + 56
d = 120
The discriminant is positive, meaning there are 2 different x intercepts.
If d = 0, then we'd have only one x intercept.
If d < 0, then there wouldn't be any x intercepts.
Help please
Use it standard algorithm solve this question
26829
X 890
Answers
Answer:
Step-by-step explanation:
26829 times 890
is 23877810
7/12 x 4x3 =? I need help
Answers
Cyphon, this is the solution:
We have to multiply the following fractions:
7/12 * 4/3
7 * 4 / 12 * 3
28/36
Simplifying, we have:
7/9 (Dividing by 4 numerator and denominator)
We have to add the following fractions:
- 1/2 + 4/9
Let's find the lowest common denominator between 2 and 9, as follows:
2, 4, 6, 8, 10, 12, 14, 16, 18, 20, 22
9, 18, 27, 36
The lowest common denominator is 18
Hi can anyone help ༼ つ ◕_◕ ༽つ

Answers
Answer:
B. 16/25
Step-by-step explanation:
6
10
+
4
100
=
6 × 10
10 × 10
+
4
100
=
60
100
+
4
100
=
60 + 4
100
=
64
100
=
64 ÷ 4
100 ÷ 4
=
16
25
Answer:
\(\huge\boxed{\sf \frac{16}{25} }\)
Step-by-step explanation:
Given fraction:\(\displaystyle \frac{6}{10} +\frac{4}{100}\)
To equalize the denominator, we will have to multiply the fraction 6/10 by 10.
\(\displaystyle =\frac{6 \times 10}{10 \times 10} +\frac{4}{100} \\\\\ =\frac{60}{100} +\frac{4}{100}\)
Now, that the denominator is equal, we can add both the fractions.
\(\displaystyle= \frac{60+4}{100} \\\\=\frac{64}{100}\)
Divide both numerator and denominator by 2.
\(\displaystyle = \frac{64 \div 2}{100 \div 2} \\\\= \frac{32}{50}\)
Divide again.
\(\displaystyle= \frac{32 \div 2}{50 \div 2} \\\\= \frac{16}{25} \\\\\rule[225]{225}{2}\)
Victoria moved into a new apartment and called to install her cable. They told her they charge $45 to install the cable box and $60 for each month of service. Which equation represents how much victoria will owe after x months?
Answers
Answer:
\(y=45+60x\)
Step-by-step explanation:
Step one:
given data
The charge is $45 to install the cable box and $60 for each month of service
Required
The linear expression for the situation.
Step two:
let the number of months be x and let the total charges be y
Hence the expression for the total charges is
\(y=45+60x\)
a rectangle has a length of 25x^3 and a width of 5x^2. Fkns the area.
Answers
Answer:
Step-by-step explanation:
I hope you want the area.
Area = L * W
L = 24x^3
W = 5x^2
Area = 24x^3 * 5x^2
Area = 120 * x^(3 + 2)
Area = 120 * x^5
Note
When you multiply numbers or letters that have powers, if the base for both of them is the same, the powers are added.
The above answer is an example x^2 * x ^3 are letters (x) that are raised to the 2nd power and the 3rd power. You add the powers.
Numbers can do the same thing
2^5 * 2^3 = 2^82*8 = 2562^5 = 642^3 = 864 * 8 = 256
How can you summarize the ruler placement postulate? you can measure the between points a and b by using as zero.
Answers
The summary of the ruler placement postulate is: A direct relationship exists between the set of points on the line and the collection of real numbers, where each point is matched to a single real number and The magnitude of the gap between two points is equal to the absolute value of the subtraction of the two associated values.
The ruler placement postulate states that if two points are given, it is possible to measure the distance between them by taking the point of reference as zero. This means that any point can be chosen as an origin, and the distance between the two points can be calculated by measuring the number of units between them. This postulate also applies to curves, and the distance between two points on a curve can be measured by taking a point of reference as zero and measuring the number of units between them. This postulate is essential in the measurement of distances between points and curves in geometry.
To know more about ruler placement postulate refer to the link brainly.com/question/25097538
#SPJ4
if a normal distribution has mean 0 and standard deviation 1, what is the 97.5th percentile?
Answers
Therefore, the 97.5th percentile of a normal distribution with mean 0 and standard deviation 1 is 1.96.
The 97.5th percentile represents the value below which 97.5% of the data falls. In a normal distribution with mean 0 and standard deviation 1, the 97.5th percentile corresponds to a z-score of 1.96.
A z-score represents the number of standard deviations a data point is from the mean. In this case, a z-score of 1.96 means that the value corresponding to the 97.5th percentile is 1.96 standard deviations above the mean.
Using a standard normal distribution table or calculator, we can find that the area under the curve to the left of a z-score of 1.96 is 0.975. This means that 97.5% of the data falls below a z-score of 1.96.
To know more about normal distribution,
https://brainly.com/question/29308006
#SPJ11
9. (-/1 Points] DETAILS LARCALC11 13.6.015. Find the gradient of the function at the given point. F(x, ) = 3x + 5y2 + 3, (4.1) Vf(4, 1) = Need Help? Read It
Answers
To find the gradient of the function \(F(x, y) = 3x + 5y^2 + 3\) at the point (4, 1), we need to calculate the partial derivatives with respect to x and y.
The gradient of a function is a vector that points in the direction of the steepest increase of the function at a given point. It is represented as a vector with its components being the partial derivatives of the function.
First, let's find the partial derivative with respect to x (denoted as ∂F/∂x):
∂F/∂x = 3
Next, let's find the partial derivative with respect to y (denoted as ∂F/∂y):
∂F/∂y = 10y
At the point (4, 1), we can substitute the values into the partial derivatives:
∂F/∂x = 3
∂F/∂y = 10(1) = 10
Therefore, the gradient of the function F(x, y) at the point (4, 1) is represented by the vector (3, 10).
Learn more about function, below:
https://brainly.com/question/30721594
#SPJ11
Evaluate -6y - 5 for y = 1/2
Answers
Answer:
15
Step-by-step explanation:
-6y-5
Substitute 1/2 for y.
-6y-5
-6(1/2)-5
-3(-5)
15
Mrs. Mack is looking at the blueprints for a new factory. On the blueprint, the factory floor is a rectangle with a length of 0.3 meter and a width of
0.25 meter. The factory will be built by using a scale factor of 140.
Mrs. Mack wants to know the area of the factory floor. She decides to do this by first determining the dimensions of the factory floor.
What will be the length of the factory floor?
Enter your answer in the box
Answers
Answer:
42 meters
Step-by-step explanation:
The length of the factory floor will be 42 meters. This is found by multiplying the length of 0.3 meters by the scale factor of 140, which yields 42 meters.
Answer:
42 meters
Step-by-step explanation:
its correct :)
Point K is on line segment \overline{JL} JL . Given JL=4x,JL=4x, JK=2x+3,JK=2x+3, and KL=x,KL=x, determine the numerical length of \overline{KL}. KL .
Answers
Answer:
KL = 3
Step-by-step explanation:
Point K is on line segment \overline{JL} JL .
Given:
JL=4x
JK=2x+3
KL=x
Determine the numerical length of KL .
Note that:
J-------K-------L
JK + KL = JL
Step 1
We solve for x
JL = JK + KL
4x = 2x + 3 + x
4x = 3x + 3
Collect like terms
4x - 3x = 3
x = 3
Step 2
We solve for KL
KL = x
x = 3
Hence,
KL = 3
Answer:
3
Step-by-step explanation:
It's correct
what is the quotient?
- 4/5 divided by 2
o -1 2/5
o - 2/5
o 1/2
o 1 3/5 ✨
Answers
\( - \frac{4}{5} \div 2 = - \frac{4}{5} \times \frac{1}{2} = - \frac{2 }{5} \\ \)
So the second one is the correct answer.
Answer:
- 2/5
Step-by-step explanation:
divide
add - sign
hope this helps
Question 2 of 3
Draw a triangle with vertices (1, 3), (2, 5), and (4, 3). Then perform the following transformation: a reflection
across the y-axis.
Answers
Answer:
To reflect a triangle across the y-axis, you need to negate the x-coordinate of each vertex.
The original triangle with vertices (1, 3), (2, 5), and (4, 3) will be reflected across the y-axis to become a new triangle with the following vertices: (-1, 3), (-2, 5), and (-4, 3).
Keep in mind that a reflection across the y-axis means that the object is flipped along the y-axis. Therefore, the x-coordinate of each vertex is multiplied by -1, which flips the object horizontally.
A bricklayer lays 4 bricks the first day of the job. On each day thereafter, he lays three times the number of bricks he laid on the previous day. If he continues this pattern, how many bricks in total will he have laid at the end of the fifth day? Responses 10 bricks 10 bricks 24 bricks 24 bricks 324 bricks 324 bricks 484 bricks
Answers
At the end of the fifth day, a bricklayer has laid 324 bricks on the job.
What is multiplication?Multiplication is a mathematical arithmetic operation.
It is also a process of adding the same types of expression to some number of times.
Example - 2 × 3 means 2 is added three times, or 3 is added 2 times.
Given, a bricklayer lays 4 bricks on the first day on the job.
On each day thereafter, he lays three times the number of bricks he laid on the previous day.
And he continued his pattern.
Let x be the number of bricks he laid in the previous day.
Then according to the question,
The number of bricks in total is = 3x
For the second day, x =4
Number of the bricks he laid on the second day = 12
Now the x =12 for the third day.
Number of the bricks he laid on the third day = 36
Now x = 36 for the fourth day.
Number of the bricks he laid on the fourth day = 108
Now the x = 108 for the fifth day.
Number of the bricks he laid on the fifth day = 324
Therefore, 324 bricks he has laid at the end of the fifth day.
To learn more about the multiplication;
https://brainly.com/question/19634536
#SPJ1
ummmm yea its hardddddddd

Answers
Answer: The correct answer is D. This is because 3 times 5 equals 15. 2 times 12 is 24. 24 plus 15 equals 39. Therefor, the first equation checks out. Now lets move on to the next equation. Remeber. x is equal to 5 and y is equal to 12. 5 times 5 equals 25. 25 minus y, or 12, equals 13. The correct answer is D (5, 12.)
Please mark my answer the brainliest if it was helpful!
Every manager has different skills, and specialties of expertise. It is quite possible that you could be in a situation where you see something that someone else does not see, especially if they are working under you with less education or experience than you. If a loan officer working under you recommends a loan to a customer, describe (A) when you would authorize that loan, and (B) when you would deny the loan in each of the following cases and give your rationale. You disagree with your subordinate because you do not think restaurants are good investments in general, so you have a bad feeling about this customer's ability to repay the loan based on your experience with the market rather than any financial facts. You disagree with your subordinate because you think medical operations are high risk for legal problems, so you have a bad feeling about this customer's ability to repay the loan based on your experience with the law rather than any financial facts. You disagree with your subordinate because you think members of their family are business failures, so you have a bad feeling about this customer's ability to repay the loan based on your experience with social history rather than any financial facts. You disagree with your subordinate because you just have a bad feeling about this customer's ability to repay the loan based on your gut instinct rather than any financial facts.
Answers
As a manager, it is important to make loan authorization decisions based on financial facts and objective analysis rather than personal biases or gut feelings.
As a responsible manager, it is essential to make loan authorization decisions based on objective criteria rather than personal biases or gut feelings. In the case where you disagree with your subordinate's recommendation to provide a loan to a customer intending to invest in a restaurant, it is important to evaluate the customer's financial facts such as their credit history, business plan, financial projections, and ability to generate sufficient revenue to repay the loan. Personal opinions about the viability of restaurants as investments should not be the sole basis for denial.
Similarly, if your subordinate recommends a loan for a medical operation, your decision should be based on the customer's financial stability, business plan, potential profitability, and risk management strategies.
When evaluating a loan application, it is crucial to focus on financial facts rather than personal opinions about family members. Assess the customer's creditworthiness, financial history, business plan, and repayment capacity to make an informed decision.
Learn more about manager here:
https://brainly.com/question/32150882
#SPJ11
What is the value of x
I will give brainliest

Answers
Answer:
52
Step-by-step explanation:
answer = fifty two
x = 52
Answer:
X+90+90+128=360(total sum of quadrilateral)
X+180+128=360
X+308=360
X=360-308
X=52
The patient's recovery time from a particular surgical procedure is normally distributed with a mean of 7 days and a standard deviation of 7.92 days. What is the probability that it will take more than 11 day to a randomly selected patient to recover from the surgical procedure? QUestion 7 The patient's recovery time from a particular surgical procedure is normally distributed with a mean of 20 days and a standard deviation of 2.24 days. Let X - is the number of days a randomly selected patient needs to recover from the surgical procedure. What is the upper bound of the 90% confidence interval of X ? QUESTION 8 The time needed to find a parking space is normally distributed with a mean of 15 minutes and a standard deviation of 4.89 minutes. 90% of the time, it takes more than how many minutes to find a parking space?
Answers
The upper bound of the 90% confidence interval for the recovery time is approximately 23.696 days.
It takes more than approximately 21.257 minutes to find a parking space 90% of the time.
To find the probability that it will take more than 11 days to recover from the surgical procedure, we need to calculate the area under the normal distribution curve to the right of 11 days.
Mean (μ) = 7 days
Standard deviation (σ) = 7.92 days
We can standardize the value of 11 days using the z-score formula:
z = (x - μ) / σ
z = (11 - 7) / 7.92
z = 0.506
Using a standard normal distribution table or a calculator, we can find the probability corresponding to the z-score of 0.506. The probability is approximately 0.3051.
Therefore, the probability that it will take more than 11 days to recover is approximately 0.3051 or 30.51%.
Question 8:
To find the upper bound of the 90% confidence interval for the number of days needed to recover from the surgical procedure, we need to calculate the z-score corresponding to the desired confidence level and then find the corresponding value using the standard deviation.
Given:
Mean (μ) = 20 days
Standard deviation (σ) = 2.24 days
For a 90% confidence interval, the z-score corresponding to the upper tail probability of 0.10 (1 - 0.90) is approximately 1.645.
Using the formula for the upper bound of the confidence interval:
Upper bound = μ + (z * σ)
Upper bound = 20 + (1.645 * 2.24)
Upper bound ≈ 23.696
Therefore, the upper bound of the 90% confidence interval for the recovery time is approximately 23.696 days.
Question 9:
To find the time it takes more than 90% of the time to find a parking space, we need to calculate the z-score corresponding to the desired upper tail probability and then find the corresponding value using the standard deviation.
Mean (μ) = 15 minutes
Standard deviation (σ) = 4.89 minutes
For a probability of 90%, the upper tail probability is 1 - 0.90 = 0.10.
Using a standard normal distribution table or a calculator, we can find the z-score corresponding to the upper tail probability of 0.10, which is approximately 1.282.
Using the formula for the upper bound:
Upper bound = μ + (z * σ)
Upper bound = 15 + (1.282 * 4.89)
Upper bound ≈ 21.257
Therefore, it takes more than approximately 21.257 minutes to find a parking space 90% of the time.
Learn more about approximately from
https://brainly.com/question/27894163
#SPJ11
Point M is on line segment
L
N
‾
LN
. Given
L
N
=
9
,
LN=9,
M
N
=
4
x
,
MN=4x, and
L
M
=
5
x
,
LM=5x, determine the numerical length of
M
N
‾
.
MN
Answers
The value of x from the given expression is 1
Addition postulateIf the point M is on the line LN, then the equivalent addition postulate is expressed as;
LM+MN = LN
Given the following
LN=9,
MN =4x
LM=5x
Substitute
5x + 4x = 9
9x = 9
x =9/9
x = 1
Hence the value of x from the given expression is 1
Learn more on addition postulate here: https://brainly.com/question/2134445
#SPJ1
a data set consists of the data given below plus one more data point. when the additional point is included in the data set the sample mean of the resulting data set is 32.083. what is the value of the additional data point?
Answers
The value of the additional data point is \($19.17$\).
What is the value of the additional data point?Let us first find the mean of the given data:
\(Mean = \frac{\sum_{i=1}^{n} x_i}{n}=\frac{39 + 45 + 43 + 42 + 44}{5}= 42.6\)
Now let's find the value of the additional data point. Let the value of the additional data point be x. Therefore, the new sum of data is
\($(39+45+43+42+44+x)$\).
Total numbers of data are 6 (five given in the set and one additional data point).So, the mean of the resulting data set is given by:
\(32.083 = \frac{(39+45+43+42+44+x)}{6}\)
Multiplying both sides of the equation by 6 we get:
\(6 \times 32.083 = (39+45+43+42+44+x)\)
We have the value of \($39+45+43+42+44$\) which is \($213$\).
Therefore, substituting all the values, we get:
\(193.83 + x = 213\)
On subtracting \($193.83$\) from both sides, we get the value of
\(x. x = 213 - 193.83 = 19.17\)
Therefore, the value of the additional data point is \($19.17$\)
See more about sample mean at: https://brainly.com/question/29441200
#SPJ11
"Just these three Please
show work please too!
Given f(x)= f(x)= = = X find lim f(f(x)) x➜0
[0,-/1 6. Use the Intermediate Value Theorem to determine if 3 tan 0=1+0 has a solution in [0,
Given m(t)=2.5t²+6t.
Answers
The limit of f(f(x)) as x approaches 0 does not exist.
Does the limit of f(f(x)) as x approaches 0 exist?When we evaluate f(f(x)), we substitute f(x) into f(x). However, the given function f(x) is not provided, as there is an error in the equation. Therefore, we cannot determine the specific value of f(f(x)). As a result, the limit of f(f(x)) as x approaches 0 does not exist since we don't have enough information about the function f(x).
Learn more about limit
brainly.com/question/12211820
#SPJ11
DIRECTION: solve the following with the complete solution, write the theorem if necessary.
plss beg i need serious answer I'm buriéd with work.
deadline now until 6:00 pm... it's 4:50 here.
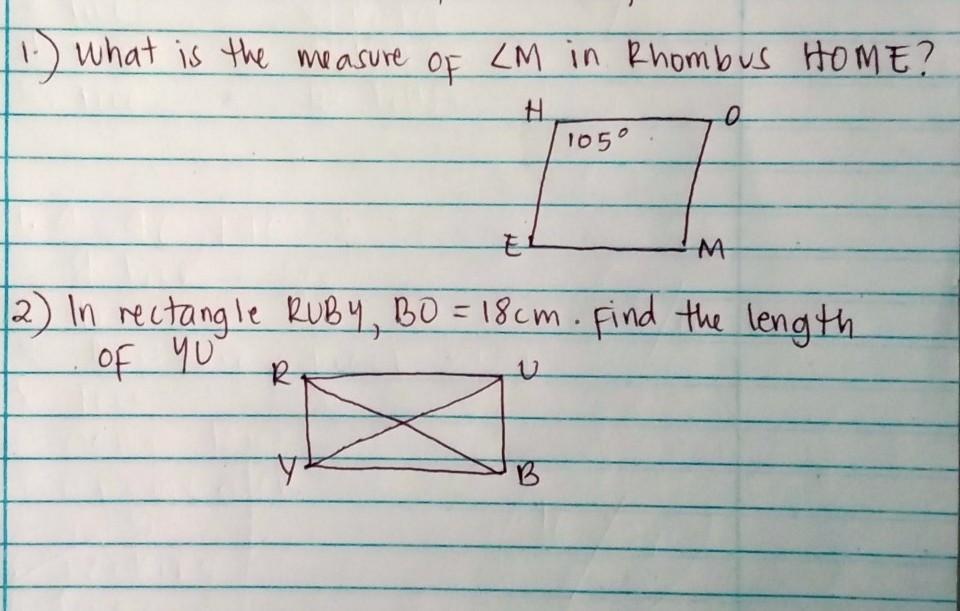
Answers
Answer:
1) Property of rhombus
Opposite angles are congruentm∠M = m∠H = 105°2) Property of rectangle
Diagonals are congruent and bisect each otherYU = 2*BO = 2*18 cm = 36 cmhelpppppppppp meeeee

Answers
Answer:
4.5
Step-by-step explanation:
A triangle has interior angles of 50°, 55°, and 75° with side lengths of 3.1 m, 3.2 m, and 4.0 m. A second
triangle has side lengths of 10.3 m, 10.5 m, and 12.0 m. What must the interior angles of the second triangle
be for the two shapes to be similar?
Answers
The interior angles of the second triangle will be 50°, 55°, and 75° respectively.
What are similar triangles?
If the corresponding angles and sides of two triangles are congruent, then the two triangles are said to be similar.
In other words, similar triangles have the same shape but may differ in size.
Given, a triangle has interior angles of 50°, 55°, and 75° with side lengths of 3.1 m, 3.2 m, and 4.0 m.
And, a second triangle has side lengths of 10.3 m, 10.5 m, and 12.0 m.
The corresponding sides of both triangles are 3.1 m, 3.2 m, and 4.0 m with 10.3 m, 10.5 m, and 12.0 m respectively.
Then, the angles of both triangles are also corresponding to each other.
So we get, the interior angles of the second triangle will be 50°, 55°, and 75° respectively.
To learn more about similar triangles
https://brainly.com/question/14285697
#SPJ13
find the average value of f over the given rectangle. f(x, y) = 4ey √x+ey , r = [0, 6] ⨯ [0, 1]
Answers
The resulting expression with respect to x ∫[0 to 6] (8/(3(1 + e))) * [(x + e)^(3/2) - x^(3/2)] dx.
The average value of the function f(x, y) = 4ey √(x+ey) over the rectangle r = [0, 6] ⨯ [0, 1] can be determined by evaluating the double integral of f(x, y) over the given region and dividing it by the area of the rectangle.
To find the average value, we start by calculating the double integral:
∬[r] f(x, y) dA
Where dA represents the differential area element.
Since the region r is a rectangle defined by [0, 6] ⨯ [0, 1], we can set up the double integral as follows:
∫[0 to 6] ∫[0 to 1] f(x, y) dy dx
Now, let's compute the inner integral with respect to y:
∫[0 to 6] 4e^y √(x + ey) dy
To evaluate this integral, we can use the u-substitution method. Let u = x + ey, then du = (1 + e) dy. The bounds of integration for y become u(x, 0) = x and u(x, 1) = x + e.
Substituting the values, the inner integral becomes:
∫[0 to 6] (4/(1 + e)) √u du
= (4/(1 + e)) ∫[x to x + e] √u du
Next, we evaluate this integral with respect to u:
(4/(1 + e)) * (2/3) * u^(3/2) | [x to x + e]
= (8/(3(1 + e))) * [(x + e)^(3/2) - x^(3/2)]
Now, we integrate the resulting expression with respect to x:
∫[0 to 6] (8/(3(1 + e))) * [(x + e)^(3/2) - x^(3/2)] dx
Evaluating this integral will give us the average value of the function over the given rectangle. However, due to the complexity of the calculations involved, providing an exact numerical result within the specified word limit is not feasible. I recommend using numerical methods or software to evaluate the integral and obtain the final average value.
Learn more about expression here
https://brainly.com/question/1859113
#SPJ11
A spinner with nine equal-sized sections numbered one to nine is spun once. What is the probability that the spinner will land on a number that is not a multiple of three?
A. 1/3
B. 2/3
C. 7/9
D. 5’9
Answers
Answer:
2/3.
Step-by-step explanation:
Total number of possible outcomes on one spin = 9.
Total of non multiples of 3:
1, 2, 4, 5,7 and 8
= 6
Answer is 6/9 = 2/3.
Answer:
B. 2/3
Step-by-step explanation:
Probability is written like a fraction where on the bottom you have ALL the possibilities. There's 9 numbers on the spinner, so there's a total of 9 different ways for it to land.
9 ways total. That goes on the bottom of the fraction.
On top of the fraction you put however many number of ways the event in question can happen. That goes on top.
A multiple of three is 3, 6, 9. There are 3 ways the spinner can land on a multiple of three. So there are 6 ways to land on NOT a multiple of three (1, 2, 4, 5, 7, 8) 6 ways, so 6 goes on top.
6/9 is the answer. But always simplify fractions first, like you do.
6/9 simplifies to 2/3
The answer is
B. 2/3
given the equation 12x-6y=-36 identify the slope and y intercept
Answers
Let's isolate y first
\(\\ \rm\Rrightarrow 12x-6y=-36\)
\(\\ \rm\Rrightarrow 6y=12x+36\)
\(\\ \rm\Rrightarrow y=\dfrac{12}{6}x+\dfrac{36}{6}\)
\(\\ \rm\Rrightarrow y=2x+6\)
Compare to y=mx+b
slope=m=2y intercept=b=6