a cylinder has20,7.5cm surface and another cylinder has 20,20,7.5cm (a)Which shape has the greater surface area? (b)By how much is it greater?. (c)Which shape has the greater volume? d) By how much is it greater?
Answers
(a) Cylinder 2 has the greater surface area.
(b) Cylinder 2 has a surface area 471.2389 cm² greater than Cylinder 1.
(c) Both cylinders have the same volume.
(d) There is no difference in volume between the two cylinders.
Define surface area and volume of cylinder?The sum of the curved and flat surfaces that make up a cylinder is its surface area.
The formula for calculating a right circular cylinder's surface area is,
A = 2πr² + 2πrh, where h is the height of the cylinder and r is the radius of the circular base.
The volume of a cylinder is how much space it covers. The volume of a right-angle cylinder can be calculated using the formula,
V = πr²h, where h is the height and r is the radius of the circular base.
Here question given that,
For Cylinder 1:
Surface area = 20,7.5 cm = 471.2389 cm² (rounded to 4 decimal places)
Volume = πr²h = π(7.5/2)²(20) = 1767.1459 cm³ (rounded to 4 decimal places)
For Cylinder 2:
Surface area = 20.20.7.5 cm = 942.4778 cm² (rounded to 4 decimal places)
Volume = πr²h = π(7.5/2)²(20) = 1767.1459 cm³ (rounded to 4 decimal places)
(a) Cylinder 2 has the greater surface area.
(b) Cylinder 2 has a surface area that is 942.4778 - 471.2389 = 471.2389 cm² greater than Cylinder 1.
(c) Both cylinders have the same volume.
(d) There is no difference in volume between the two cylinders.
To know more about cylinder, visit:
https://brainly.com/question/23935577
#SPJ9
Related Questions
In triangle ABC, AB = BC. If mA = 7x and mzC = 2x + 50, find mzB. I’m
Answers
Answer: if someone can figure that out i will give you a cookie cuz that stuff looks a lil hard.
Step-by-step explanation:
YOO ITS 4AM AND I HAVE CLASS IN TWO HOURS I NEED HELP
-4(7a+7)-2a=92
Answers
- 4 ( 7a + 7 ) - 2a = 92
- 28a - 28 - 2a = 92
- 28a - 2a - 28 = 92
Collect like terms
- 30a - 28 = 92
Add both sides 28
- 30a - 28 + 28 = 92 + 28
- 30a = 120
Divide both sides by - 30
- 30a ÷ - 30 = 120 ÷ - 30
a = - 4ABCD with vertices B (2,1), C (2,5), and D (6,1) has its orthocenter at which point?
A. (0,0)
B. (2,1)
C. (2,5)
D. (6,1)
Answers
Step-by-step explanation:
d is the answer as far as I can tell from a little experience
The ratio of the sides of a triangle are 4:6:8. If the perimeter is 216 meters, what is the length of the longest side?
Answers
Answer:
i just need points sorry
Step-by-step explanation:
Write a paragraph proof that 1+1=2
(Fun fact: it took over 72 pages just to prove that 1+1=2)
Answers
Answer:
The theorem here is essentially that
if a and 3 are disjoint sets with
exactly one element each, then their
union has exactly two elements. ...
Peano shows that it's not hard to
produce a useful set of axioms that
can prove 1+1=2 much more easily
than Whitehead and Russell do.
Which point best approximates 45?

Answers
Answer:
C
Step-by-step explanation:
The square root of 45 is:
6.708
Meaning that C is the correct point.
Hope this helps! :)
Because root 45 is 6.708203932499369
So round that to 2 SF is 6.70 which is closer to 7 than 6 rounded up so it would be C as it’s nearest to 7 but not on 7.
Read the numbers and decide what the next number should be. 1 1.25 7 7.50 2 2.25 8
Answers
(5) Suppose that A is an n x n matrix with and 2 is an eigenvalue. (a) Find the corresponding eigenvalue for -34². (b) Find the corresponding (c) Find the corresponding (d) Find the corresponding eigenvalue for A-¹. eigenvalue for A + 71. eigenvalue for 8.A.
Answers
a. The corresponding eigenvalue for -3\(4^2\)A is -23104
d. The corresponding eigenvalue for A+71I is 73
c. The corresponding eigenvalue for 8A is 16
d. The corresponding eigenvalue for \(A^-1\) is λ
How to calculate eigenvalueLet v be an eigenvector of A corresponding to the eigenvalue 2, That is,
Av = 2v.
We have (\(-34^2A\))v
= \(-34^2\)(Av)
= \(-34^2\)(2v)
= -23104v.
Hence, the eigenvalue is -23104 corresponding to the eigenvector v.
We have (A+71I)v
= Av + 71Iv
= 2v + 71v
= 73v.
Therefore, 73 is an eigenvalue of A+71I corresponding to the eigenvector v.
We have (8A)v = 8(Av)
= 16v.
Thus, 16 is an eigenvalue of 8A corresponding to the eigenvector v.
Let λ be an eigenvalue of \(A^-1\), and let w be the corresponding eigenvector, i.e.,
\(A^-1w\) = λw.
Multiplying both sides by A,
w = λAw.
Substituting v = Aw,
w = λv.
Therefore, λ is an eigenvalue of \(A^-1\) corresponding to the eigenvector v.
Learn more on eigenvalue on https://brainly.com/question/15586347
#SPJ4
(a) To find the corresponding eigenvalue for (-34)^2, we can square the eigenvalue 2:
(-34)^2 = 34^2 = 1156.
Therefore, the corresponding eigenvalue for (-34)^2 is 1156.
(b) To find the corresponding eigenvalue for A + 71, we add 71 to the eigenvalue 2:
2 + 71 = 73.
Therefore, the corresponding eigenvalue for A + 71 is 73.
(c) To find the corresponding eigenvalue for 8A, we multiply the eigenvalue 2 by 8:
2 * 8 = 16.
Therefore, the corresponding eigenvalue for 8A is 16.
(d) To find the corresponding eigenvalue for A^(-1), we take the reciprocal of the eigenvalue 2:
1/2 = 0.5.
Therefore, the corresponding eigenvalue for A^(-1) is 0.5.
Learn more about eigenvalue from :
https://brainly.com/question/15586347
#SPJ11
x+y=5
3x-y=7
solve this equation
Answers
X= (5,0)
Y= (0,5)
Slope is 3 and y intercept is (0,7)
NEED DONE ASAP PLS!!!

Answers
3√64 × 2^-1
= 4 × 1/2
= 2
The answer is 2.
Please do mark me Brainliest
which graph represents the inequality y < 2x +3 correctly

Answers
Answer:
im not sure , sorry
Step-by-step explanation:
Answer:
Option C
Step-by-step explanation:
Graphing inequality:
1) First plot y = 2x + 3 as a dotted line as y is less than x
Put x = 0, y = 2*0 +3 = 0 + 3 = 3 ⇒ (0, 3)
Put x = 1 , y = 2*1 + 3 = 2 +3 = 5 ⇒(1 , 5)
Put x = -1, y =2*(-1)+3 ⇒ -2+3 = 1 ⇒ (-1,1)
Mark these three points, and draw a dotted line.
2) Shade the area bellow the dotted line as y < 2x + 3
Check: take a point in the shaded area. (1 , -1)
-1 < 2*1 + 3
-1 < 2 +3
-1 < 5
The condition is satisfied.
alex works for his father assembling clock motors he is paid a piecework rate of $8.50 per motor. this week he assembled 21 motors
Answers
Answer:
178.5
Step-by-step explanation:
21×8.50 is 178.5 just that
At the end of month, a woman pays $714 in rent.If the rent consititutes 21% of her monthly income, what is her hourly wage given the fact that she works 34 hours per week
Answers
Explanation: 714 divided 21% divided 34X 4.35
= 3400 divided 147.9
= $23
Helen has only 10 questions left to do of her Math homework. She
has 120 minutes before it's her bedtime and it takes 5 minutes to do
each Math question. Describe the pattern and determine how much
time she will have available after her homework is complete.
A)Start at 120 and add 5 each time. She will have 70 minutes of free time after she finishes her math questions.
B) Start at 120 and subtract 5 each time. She will have 70 minutes of free time after she finishes her math questions.
C)Start at 120 and subtract 5 each time. She will have 75 minutes of free time after she finishes her Math questions.
D) Start at 120 and subtract 5 each time. She will not have enough time to complete all of her math questions.
Answers
B. Start at 120 and subtract 5 each time. She will have 70 minutes of free time after she finishes her math questions
A school trip to a museum cost $2,056. A total of 125 chaperones and students went on the trip. Adult admission to the museum costs $23, and student admission costs $16. How many chaperones and students went to the museum?
Answers
Answer:
8 adults and 117 studentsStep-by-step explanation:
Set the following equations based on the question:
c + s = 12523c + 16s = 2056Solve the system by elimination, multiply the first equation by 23 and subtract the second one:
23c + 23s - 23c - 16s = 23*125 - 20567s = 819s = 819/7s = 117Find the value of c:
c + 117 = 125c = 8Answer:
Chaperones = 8 and Student = 117
Step-by-step explanation:
Let,
Chaperones = x
Student = y
x + y = 125
=> x = 125 - y (1)
23x + 16y = 2056
=> 23(125 - y) + 16y = 2056
=> 2875 - 23y + 16y = 2056
=> 2875 - 7y = 2056
=> 2875 - 2056 = 7y
=> 819 = 7y
=> 819/7 = y
=> 117 = y
From 1
=> x = 125 - 117
=> x = 8
A motor racing circuit has length 5 5/6 miles.A straight section of the circuit has length 1 1/4 miles.What fraction of the circuit is the straightest section
Answers
Answer:
Dear user,
Answer to your query is provided below
The fraction of the circuit is (3/14)
Step-by-step explanation:
Explanation of the same is attached in image

Answer:
3/14
Step-by-step explanation:
4. Create a Python program (Filename: optimization.py) to perform the following optimization problem. Minimize x
3
−2cos(x)+9 s.t. 0≤x≤2 This optimization is to find the minimum value of x
3
−2cos(x)+9 when 0≤x≤2. This optimization problem can be approximately solved by simply searching in the feasible range. In the program, you can simply define a list x=[0,0.01,0.02,…,1.98,1.99,2.0] and also define an objective function as f(x)=x
3
−2cos(x)+9, and search for the minimum f(x) of different values in the list x.
Answers
Here's a Python program (Filename: optimization.py) to perform the optimization problem: Minimize x
3
−2cos(x)+9 s.t. 0≤x≤2The optimization problem is to find the minimum value of x
3
−2cos(x)+9 when 0≤x≤2. This optimization problem can be approximately solved by simply searching in the feasible range. In the program, you can simply define a list x = [0, 0.01, 0.02, …, 1.98, 1.99, 2.0]. Also, define an objective function as f(x) = x
3
−2cos(x)+9 and search for the minimum f(x) of different values in the list x.```python
import math
x = [0.01*i for i in range(201)]
min_val = 1e18
opt_x = 0
def f(x):
return x**3 - 2*math.cos(x) + 9
for xi in x:
if xi>=0 and xi<=2:
fval = f(xi)
if fval
To know more about optimization problems: https://brainly.com/question/14160739
#SPJ11
x+9;x=6
find the value of why for the given value of x
Answers
Answer:
-5
Step-by-step explanation:
Answer:
15
Step-by-step explanation:
x+9=6 when x=6
6+9=15
15=15
HELP!!!!
Find the equation of the line below.
O A y= 1/6 x
O B.y= 1/3 x
O C. y = 3x
0 D. y=6x
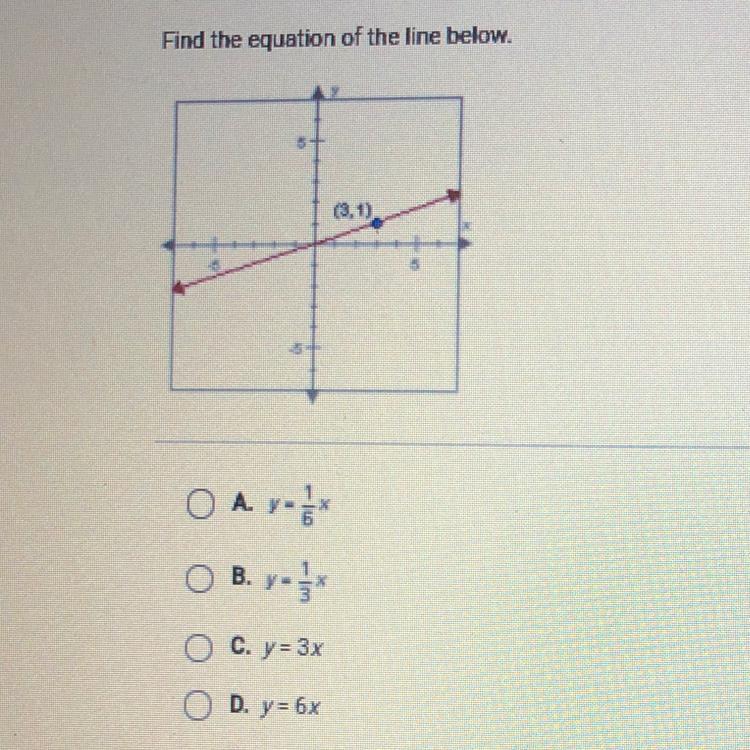
Answers
This meal costs $19.00 .A sales tax is applied, followed by an automatic tip of 18 %.What is the total with tax and tip?
Answers
The total cost of he meat with tax and tip is $ 22.42
How to find the totalTo calculate the total cost with tax and tip, we need to follow these steps:
multiply the meal cost by the tip rate. when the tip rate is 18%, we have:
Tip amount = $19.00 * 0.18 = $3.42
Add the meal cost, sales tax, and tip amount to get the total cost:
Total cost = Meal cost + Sales tax + Tip amount
= $19.00 + $3.42
= $ 22.42
Therefore, the total cost with tax and tip is $22.42
Learn more about total cost at
https://brainly.com/question/5168855
#SPJ1
Cole jarred 8 liters of jam after 2 days. How much jam did Cole jar if he spent 5 days making jam? Assume the relationship is directly proportional.
Answers
A rainwater was 2/3 full of water. After 60 liters of water were used from the barrel, it was 5/12 full. How much water does the barrel hold when full?
Answers
Answer: 240 liters
=======================================================
Work Shown:
x = capacity of barrel in liters
This value of x is some positive real number. It represents the most amount of water the barrel can hold.
The problem states that the barrel is 2/3 full to start with. This means, we start off with (2/3)x liters of water. Then we subtract off 60 of them to get to 5/12 full, meaning we have (5/12)x liters left.
We can form this equation
(2/3)x - 60 = (5/12)x
Multiply both sides by 12 to clear out the fractions
(2/3)x - 60 = (5/12)x
12*( (2/3)x - 60 ) = 12*(5/12)x
12*(2/3)x - 12*60 = 12*(5/12)x
8x - 720 = 5x
From here we solve for x
8x - 5x = 720
3x = 720
x = 720/3
x = 240
The barrel's full capacity is 240 liters
--------------------------
Check:
2/3 of 240 = (2/3)*240 = 160
The barrel starts off with 160 liters of water inside.
We then use 60 of them to be left with 160-60 = 100 liters
Note how 100/240 = (5*20)/(12*20) = 5/12, showing that 100 liters out of 240 total reduces to the fraction 5/12. In other words, when we say "5/12 full" we mean 100 liters full. This helps confirm we have the right answer.
There are 20 students in a 5th grade class. Four of these students will be selected to be patrols. In how many ways can the four patrols be selected?
Answers
Answer:
4845 ways
Step-by-step explanation:
We need to form groups of four students among the total of 20 students, and the order of the students inside the group does not matter, so we have a combination problem.
To solve this problem, we just need to find the combination of 20 choose 4:
C(20, 4) = 20! / (16! * 4!) = 20 * 19 * 18 * 17 / (4 * 3 * 2) = 4845
There are 4845 ways of selecting this group of four patrols.
Name the property that justifies the statement : 14x × 0 = 0
Answers
the zero property of multiplication
Explanation
the zero property of multiplication says, the product of any number and zero, is zero,
so in this case the number is (14x)
so
14x*0=0
I hope this helps you
find the range of the function f(x)=2x+4
Answers
Answer:
(0,4)
Step-by-step explanation:
this is highest and only Y intercept. the range of a graph is all of the Y intercepts. and the domain of a graph is all of the x-intercepts

According to flightstats com, American Airlines flights from Dallas to Chicago are on time 80% of the time. Suppose 20 flights are randomly selected, and the number of on-time flights is recorded. (a) Explain why this is a binomial experiment (b) Determine the values of n and p. (c) Find and interpret the probability that exactly 12 flights are on time. (d) Find and interpret the probability that fewer than 12 flights are on time. (e) Find and interpret the probability that at least 12 flights are on time. (f) Find and interpret the probability that between 10 and 12 flights, inclusive, are on time.
Answers
As, in our question we are doing 20 trials to get the number of on-time flights. Hence, this is a binomial experiment.
The value of p=0.8 and n=20
The probability that exactly 12 flights are on time = 0.1772
The probability that exactly fewer than 12 flights are on time = 0.1049
The probability that at least 12 flights are on time = 0.8951
The probability that between 10 and 12 flights, are on time = 0.1795
(a) Binomial probability is the probability of exactly 'k' successes on 'n' repeated trials in an experiment which has two possible outcomes.
As, in our question we are doing 20 trials to get the number of on-time flights. Hence, this is a binomial experiment.
(b) As, the probability of success on an individual trial is p and here success means the flight is on-time so the value of p be,
p = 80% = 0.8
As, we made 20 trials and n is the total number of trials then,
the value of n be,
n = 20
(c) Now, if the probability of success on an individual trial is p ,
Then the binomial probability is C(n,x)⋅p^x⋅(1−p)^(n−x)
The probability that exactly 12 flights are on time, be
P(X=12) = C(20,12)⋅(0.8)^12⋅(1−0.8)^(20-12)
P(X=12) = C(20,12)⋅(0.8)^12⋅(0.2)^(8)
P(X=12) = 10,07,760×0.0687×0.00000256
P(X=12) = 0.1772
(d) Now, the probability that exactly fewer than 12 flights are on time, be
P(X<12) = 1 - P(X>12)
So, P(X>12) = P(X=13)+P(X=14)+P(X=15)+P(X=16)+P(X=17)+P(X=18)+P(X=19)+P(X=20)
So, P(X>12) = 0.0545 + 0.0364 + 0.1746 + 0.2182 + 0.2054 + 0.1369 + 0.0576 + 0.0115
P(X>12) = 0.8951
P(X<12) = 1 - 0.8951
P(X<12) = 0.1049
(e) Now, the probability that at least 12 flights are on time, be
P(X>12)=0.8951
(f) The probability that between 10 and 12 flights, are on time, be
P(X=10)+P(X=11)+P(X=12) = 0.0020 + 0.0003 + 0.1772
P(X=10)+P(X=11)+P(X=12) = 0.1795
Learn more about Probability here https://brainly.com/question/13604758
#SPJ1
A bakery records the number of cakes, x it makes and the corresponding total price, p, of the cakes, in dollars. Number of Cakes (x) Price (p) 1 12 2 24 3 36 4 48 Write an equation that represents the relationship between x and p?
Answers
The equation that represents the relationship between the number of cakes (x) and the price (p) is p = 12x.
From the given data, we can observe that the price of the cakes is directly proportional to the number of cakes made. As the number of cakes increases, the price also increases proportionally.
The equation p = 12x represents this relationship, where p represents the price of the cakes and x represents the number of cakes made. The coefficient 12 indicates that for every unit increase in the number of cakes (x), the price (p) increases by 12 units.
For example, when x = 1, the price (p) is 12. When x = 2, the price (p) is 24, and so on. The equation p = 12x can be used to calculate the price of the cakes for any given number of cakes made.
Learn more about equation here:
https://brainly.com/question/29657992
#SPJ11
**MARKING BRANLIEST PLS HELP FAST**

Answers
MODELING REAL LIFE After earning interest, the balance of an account is $420. The new balance is 7/6 of the original balance. How much interest did it earn?
Answers
Answer:
It earned $60 in interest
Step-by-step explanation:
Find the volume of the solid generated by revolving the region bounded by the graphs of
y sex, y >= 0 and x >= 0 about the x-axis.
Answers
The volume of the solid generated by revolving the region bounded by the graphs of y = x, y ≥ 0, and x ≥ 0 about the x-axis is 0 cubic units.
To find the volume using the disk method, we integrate the cross-sectional areas of the disks formed by revolving the region about the x-axis. The region bounded by y = x and y = 0 represents the area under the curve y = x in the positive x-axis region.
The radius of each disk is given by the value of y, which is equal to x in this case. The volume of each disk can be expressed as dV = πx^2 * dx.To determine the limits of integration, we consider the x-values where the curves intersect. In this case, y = x intersects y = 0 at the origin (0, 0). Therefore, the integral for the volume is V = ∫(0 to c) πx^2 * dx, where c represents the x-value where the curves intersect.
Evaluating the integral, we have V = π∫(0 to c) x^2 * dx. Integrating x^2 with respect to x gives V = π * [x^3/3] evaluated from 0 to c. Since c represents the x-value where the curves intersect, we have c = 0. Substituting the limits of integration, the volume simplifies to V = π * (0^3/3 - 0^3/3) = 0.
Therefore, the volume of the solid generated by revolving the region bounded by the graphs of y = x, y ≥ 0, and x ≥ 0 about the x-axis is 0 cubic units.
Learn more about volume here
https://brainly.com/question/32619305
#SPJ11