A bag of tokens contains 6 red, 6 green, and blue tokens. What is the probability that a randomly selected token is purple? Answer in a fraction
Answers
Answer:
The probability that a randomly selected token is red and green is 27/200 in fraction form and 0.135 in decimal form.
Step-by-step explanation:
What is probability?
Probability is defined as the possibility of an event being equal to the ratio of the number of favorable outcomes and the total number of outcomes.
Given that a bag of tokens contains 9 red, 6 green, and 5 blue tokens.
Total = 9 red + 6 green + 5 blue = 9 + 6 + 5 = 20
Total token = 20
P(E₁) is 9 out of 20 are red.
P(E₂) is 6 out of 20 are green.
⇒ P(E₁) = 9/20
⇒ P(E₂) = 6/20
Required probability P(E) = P(E₁)×P(E₂)
Required probability P(E) = 9/20 × 6/20
Required probability P(E) = 54/400
Required probability P(E) = 27/200 = 0.135
Therefore, the probability that a randomly selected token is red and green is 27/200.
Related Questions
HELP PLEASE! Which reason is the justification for the statement that angle A ≅ angle B?
A) Vertical angles are congruent.
B) Linear angles are equal.
C) Intersecting lines form opposing angles.
D) Lines intersect at one point.

Answers
in a standard inch ruler how many 3/8 are in 6 inches
Answers
Nikhil and Mae work at the same company. Nikhil has been at the company 4 time+s as long as Mae. Nikhil's time at the company is 8 more than 2 times Mae's time. The following system of equations models the scenario: x = 4y x = 8 + 2y How many years has each person been employed by the company? Nikhil has been with the company for 16 years, while Mae has been there for 4 years. Nikhil has been with the company for 24 years, while Mae has been there for 6 years. Nikhil has been with the company for 20 years, while Mae has been there for 5 years. Nikhil has been with the company for 12 years, while Mae has been there for 3 years
Answers
Applying the system of equations given, we can conclude that: a. Nikhil has been with the company for 16 years, while Mae has been there for 4 years.
How to Apply a System of Equations?To solve the system of equations, let's substitute the value of x from the first equation into the second equation:
x = 4y
8 + 2y = 4y
Simplifying the equation, we get:
8 = 2y
Dividing both sides by 2:
4 = y
Now, substitute the value of y back into the first equation to find x:
x = 4y
x = 4(4)
x = 16
Therefore, Nikhil has been employed by the company for 16 years, and Mae has been employed for 4 years.
The correct answer is option a. Nikhil has been with the company for 16 years, while Mae has been there for 4 years.
Learn more about System of Equations on:
https://brainly.com/question/13729904
#SPJ1
Example 1: Every Number Has an Opposite
Locate the number 8 and its opposite on the number line. Explain how they are related to zero.
-8-7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7
Exercises 2-3
2. Locate and label the opposites of the numbers on the number line.
9
-2
4
a.
b.
C.
8
Answers
Answer:
To locate the number 8 and its opposite on the number line, we can use the given number line:
-8 -7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7
Number 8 is located to the right of zero. Its opposite can be found by moving an equal distance to the left of zero. Since the number line is symmetric about zero, the opposite of 8 would be -8.
-8 -7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7
↑ ↑
So, the opposite of 8 is -8. They are related to zero as equal distances on opposite sides of zero on the number line.
Now, let's address exercises 2-3:
a. To locate the opposite of 9, we need to move an equal distance to the left of zero. The opposite of 9 is -9.
-8 -7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7
↑
b. To locate the opposite of -2, we need to move an equal distance to the right of zero. The opposite of -2 is 2.
-8 -7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7
↑
c. To locate the opposite of 4, we need to move an equal distance to the left of zero. The opposite of 4 is -4.
-8 -7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7
↑
So, the opposites of the given numbers are:
9 → -9
-2 → 2
4 → -4
They are related to zero as equal distances on opposite sides of zero on the number line.
Step-by-step explanation:
tell which property of equality was used. 5m + 4 = 19 5m + 43= 19-3.
Answers
The value of m from the given equation is 3
Given the expression
5m + 4 = 19
Subtract 4 from both sides using the subtraction law of equality.
5m + 4 - 4 = 19 - 4
5m = 19 - 4
5m = 15
Divide both sides by 5 using the Division law of equality
5m/5 = 15/5
m = 3
Hence the value of m from the given equation is 3
Learn more on linear equation here: https://brainly.com/question/2159099
Can anyone help me please
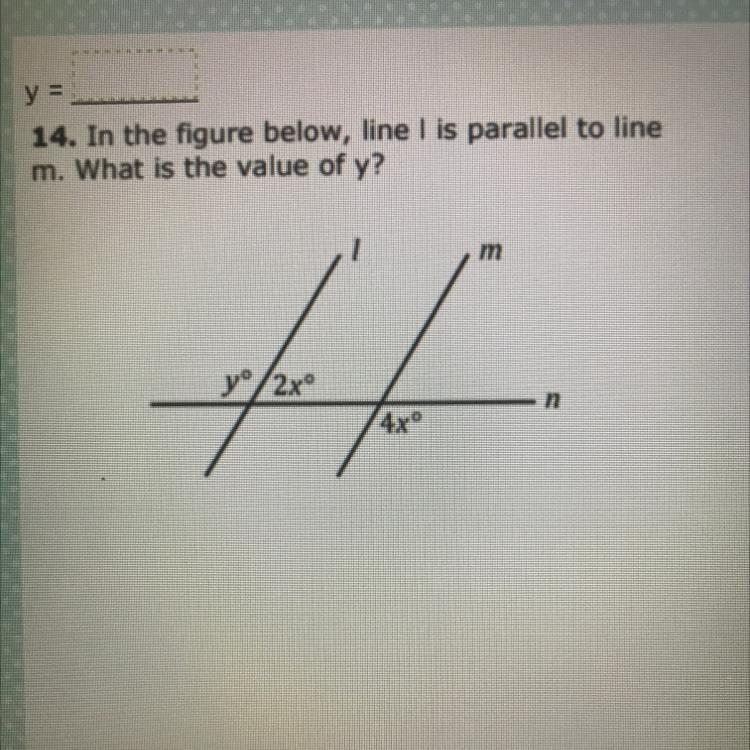
Answers
Answer:
y = 120
Step-by-step explanation:
y = 4x (corresponding angles)
y + 2x = 180
substitute the value of y into the second equation
4x + 2x = 180
6x = 180
x = 30
now that we have the value of x, plug it into either equation and solve for y
y = 4 (30)
y = 120
Solve using substitution
y = 5x– 1
y = 3 + 5
Answers
Answer:
(9/5, 8)
Step-by-step explanation:
y = 3 + 5
y=8
y = 5x– 1
8=5x– 1
9=5x
x=9/5
(9/5, 8)
Explanation in photo:

Help with Venn Diagram / Set Operation Question (100 points)

Answers
Answer:
1)
Find the union of A and B:
A∪B = {2,3,4,5,6,7,9,10,11,13,14,15,18,19,20}Find the complement of above:
(A∪B)\(^{C}\) = U\(A∪B) = {1,2,3,4,...,18,19,20}\ {2,3,4,5,6,7,9,10,11,13,14,15,18,19,20} = {1,8,12,16}2)
Find the intersection of A∩C:
A∩C = {5,6,7,14,20}Find the complement of above:
(A∩C)\(^C\) = U\(A∩C) = {1,2,3,4,...,18,19,20}\ {5,6,7,14,20} = {1,2,3,4,8,9,10,11,12,13,15,16,17,18,19}There are 24 fluid ounces in 3 cups how many cups are equal to 144 fluid ounces?
Answers
Answer:
18 cups
Step-by-step explanation:
Answer:
18 cups
Step-by-step explanation:
24/3 = 8
144/8 = 18 cups
EXREAMLY URGENT!! WILL FOREVER THANK YOU!!!! PLS JUST TAKE A LOOK!!!!! 1. What is the ratio of the sides of triangle XYZ?

Answers
Answer:
Dear Laura Ramirez
Answer to your query is provided below
The ratio of triangle XYZ is 1:√3 :2.
Step-by-step explanation:
A 30-60-90 right triangle (literally pronounced "thirty sixty ninety") is a special type of right triangle where the three angles measure 30 degrees, 60 degrees, and 90 degrees. The triangle is significant because the sides exist in an easy-to-remember ratio: 1:√3 :2.
Write your answer as an integer or as a decimal rounded to the nearest tenth.

Answers
Applying the sine rule the value of side w is calculated to be 6.2
How to find the size of wThe size of w is calculated using the sine rule which states that the ratio of a side and the opposite angles are equal
the formula is
Sin A / a = Sin B / b = Sin C / c
applying the formula for the problem
Sine W / w = Sine X / x
plugging in the values
Sine 38 / w = Sine 84 / 10
cross multiplying
w = 10 x Sin 38 / Sine 84
x = 6.185
x = 6.2 to the nearest tenth
Learn more about sine rule at:
https://brainly.com/question/29625488
#SPJ1
In ΔQRS, q = 50 cm, s = 27 cm and ∠S=110°. Find all possible values of ∠Q, to the nearest 10th of a degree. PLS
Answers
Answer:
2000
Step-by-step explanation:
2hwhjfwftwrd ggg gggggg gggggggggg ggggg
Your total monthly bill (T
) from the Electric and Gas Company depends on how much electricity and how much gas you use each month. For every kilowatt hour of electricity (k
) you use, you are charged $0.25
and for each therm (t
) of gas used you are charged $0.97
.
Which of the following equations represents the relationship between electricity and gas used and your bill?
Answers
The equation representing the relationship between Electricity and Gas used, and your Bill is:
T = 0.25 * k + 0.97 * t
Step-by-step explanation:
Make a plan:
We need to find the equation that represents the relationship between the total monthly bill (T), The Kilowatt Hours of Electricity used (k), and the Terms of Gas used (t).
T = 0.25 * k + 0.97 * t
Solve the problem:
1 - Write the Equation for the cost of Electricity:
0.25 * k
2 - Write the Equation for the Cost of Gas:
0.97 * t
3 - Combine the Equations to Represent the total Monthly Bill (T):
T = 0.25 * k + 0.97 * t
Draw the Conclusion:Hence, The equation representing the relationship between Electricity and Gas used, and your Bill is:
T = 0.25 * k + 0.97 * t
I hope that helps!
For my option I choose b or d can I please get some help

Answers
Amount invested = $2500
rate = 3% = 0.03
n = number of times compounded
n = annually = 1
time = 4 years
To get the future value after 4 years, we will apply the compund interest formula:
\(\begin{gathered} FV\text{ = P(1 +}\frac{r}{n})^{nt} \\ \text{where FV = future value} \\ P\text{ = principal = 2500, r = rate, t = time} \\ n\text{ = number of times compounded} \end{gathered}\)substitute the values into the formula:
\(\begin{gathered} FV\text{ = 2500(1 + }\frac{0.03}{1})^{1\times4} \\ FV\text{ = 2500(1+0}.03)^4 \\ \\ FV\text{ = 2500(1+0}.03)^4\text{ (option A)} \end{gathered}\)A rectangular prism with a square base has a height of 17.2cm and a volume of 24.768cm². What is the length of its base?
Answers
The formula for the volume of a rectangular prism is:
V = lwh
where V is the volume, l is the length, w is the width, and h is the height.
We know that the height of the rectangular prism is 17.2 cm, and the volume is 24.768 cm³. We also know that the base is a square, so the length and width are the same. Let's call the length of the base x.
Then, we can write:
V = lwh
24.768 = x * x * 17.2
24.768 = 17.2x²
x² = 1.44
x = 1.2
Therefore, the length of the base of the rectangular prism is 1.2 cm.
The formula for the volume of a rectangular prism is V = lwh, where l is the length, w is the width, and h is the height.
In this problem, we know that the height is 17.2 cm, and the volume is 24.768 cm³. We also know that the base is a square, so the length and width are the same.
Therefore, we can write the equation:
24.768 = x² * 17.2
To solve for x, we can divide both sides by 17.2, and then take the square root of both sides:
x² = 24.768 / 17.2
x² = 1.44
x = sqrt(1.44)
x = 1.2
Therefore, the length of the base of the rectangular prism is 1.2 cm.
A bucket contains 72 red, 48 blue, 48 green, and 48 yellow crayons. The art teacher also has 120 pieces of drawing paper. What is the largest number of identical kits the art teacher can make with all of the crayons and all of the paper?
Answers
The art teacher can make a maximum of 24 identical kits using all the crayons and drawing paper for proper distribution.
To determine the largest number of identical kits the art teacher can make using all the crayons and drawing paper, we need to find the greatest common divisor (GCD) of the quantities.
The GCD represents the largest number that can divide all the quantities without leaving a remainder.
The GCD of the quantities of crayons can be found by considering the prime factorization:
72 = 2³ × 3²
48 = 2⁴ × 3
48 = 2⁴ × 3
48 = 2⁴ × 3
The GCD of the crayons is 2³ × 3 , which is 24.
Now, we need to find the GCD of the quantity of drawing paper:
120 = 2³ × 3 × 5
The GCD of the drawing paper is also 2³ × 3 , which is 24.
Since the GCD of both the crayons and drawing paper is 24, the art teacher can make a maximum of 24 identical kits using all the crayons and drawing paper.
Each kit would contain an equal distribution of crayons and drawing paper.
Learn more about distribution here:
brainly.com/question/30034780
#SPJ1
Below you have the estimates of a multiple linear regression that satisfies the CLRM assumptions. The independent variable is Y.How do you interpret the coefficient on X5? O For each unit that X% increase, Y decreases 0.084 units. O For each unit that X5 increases, Y decreases 0.084 units on averageO For each unit that X5 increases, Y decreases 0.084 units on average, holding the value of X2 constant. O For each unit that X5 increases, Y decreases 0.084 units on average, holding the value of X3 constant. O For each unit that X5 increases, Y decreases 0.084 units on average, holding the value of X4 constant. O For each unit that X5 increases, Y decreases 0.084 units on average, holding the value of X2, X3 and X4 constant.
Answers
Answer:
Step-by-step explanation:
Historical evidence indicates that times between fatal accidents on scheduled American domestic passenger flights have an approximately exponential distribution. Assume that the mean time between accidents is 43 days. What is the variance of the times between accidents
Answers
Answer:
The variance of the times between accidents is of 1849 days squared.
Step-by-step explanation:
Exponential distribution:
The exponential probability distribution, with mean m, is described by the following equation:
\(f(x) = \mu e^{-\mu x}\)
In which \(\mu = \frac{1}{m}\) is the decay parameter.
The variance of the exponential distribution is:
\(Var = \frac{1}{\mu^2}\)
Assume that the mean time between accidents is 43 days.
This means that \(m = 43, \mu = \frac{1}{43}\)
What is the variance of the times between accidents?
\(Var = \frac{1}{(\frac{1}{43})^2} = 43^2 = 1849\)
The variance of the times between accidents is of 1849 days squared.
1. Select all equations that have two solutions.
A.x² = 16
B. 4x² = 0
C. x² = -16
D. 3x + 2 = 14
Ex² - 1 = 24
F) (x + 8) (x - 8) = 0
Answers
A) x² = 16 (solutions: x = 4, x = -4)
B) 4x² = 0 (solutions: x = 0)
F) (x + 8)(x - 8) = 0 (solutions: x = -8, x = 8)
7. Of 101 randomly selected adults
over 30 who frequent a very large
mall, 35 admitted to having lost their
car at the mall. Construct a 95%
confidence interval for the true
percentage of all adults over 30 who
shop at that mall who admit to having
lost their car at the mall.
Answers
Answer:
The 95% confidence interval for the true percentage of all adults over 30 who shop at that mall who admit to having lost their car at the mall is (25.37%, 43.93%).
Step-by-step explanation:
In a sample with a number n of people surveyed with a probability of a success of \(\pi\), and a confidence level of \(1-\alpha\), we have the following confidence interval of proportions.
\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)
In which
z is the z-score that has a p-value of \(1 - \frac{\alpha}{2}\).
Of 101 randomly selected adults over 30 who frequent a very large mall, 35 admitted to having lost their car at the mall.
This means that \(n = 101, \pi = \frac{35}{101} = 0.3465\)
95% confidence level
So \(\alpha = 0.05\), z is the value of Z that has a p-value of \(1 - \frac{0.05}{2} = 0.975\), so \(Z = 1.96\).
The lower limit of this interval is:
\(\pi - z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.3465 - 1.96\sqrt{\frac{0.3465*0.6535}{101}} = 0.2537\)
The upper limit of this interval is:
\(\pi + z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.3465 + 1.96\sqrt{\frac{0.3465*0.6535}{101}} = 0.4393\)
As percentages:
0.2537*100% = 25.37%
0.4393*100% = 43.93%
The 95% confidence interval for the true percentage of all adults over 30 who shop at that mall who admit to having lost their car at the mall is (25.37%, 43.93%).
To purchase $14,500 worth of restaurant equipment for her business, Debra made a down payment of $1300 and took out a business loan for the rest. After 2 years of paying monthly payments of $585.04, she finally paid off the loan.
(a) What was the total amount Debra ended up paying for the equipment (including the down payment and monthly payments)?
(b) How much interest did Debra pay on the loan?
Answers
The total amount Debra ended up paying for the equipment was $28,541.60 and the amount of interest Debra paid on the loan was $14,041.60
(a) To find the total amount Debra ended up paying for the equipment (including the down payment and monthly payments), we need to add the down payment to the total amount of the loan, and then add the total amount of the monthly payments made over the two years.
Total amount of the loan = $14,500 - $1,300 (down payment) = $13,200
Total amount paid = Down payment + Total amount of the loan + Total amount of monthly payments
Total amount paid = $1,300 + $13,200 + ($585.04 x 24) [since there are 24 monthly payments in 2 years]
Total amount paid = $1,300 + $13,200 + $14,041.60
Total amount paid = $28,541.60
Therefore, the total amount Debra ended up paying for the equipment (including the down payment and monthly payments) was $28,541.60.
(b) To find the amount of interest paid on the loan, we need to subtract the total amount borrowed from the total amount paid, and then subtract the down payment. This will give us the total amount of interest paid over the two years.
Total interest paid = Total amount paid - Total amount borrowed - Down payment
Total interest paid = $28,541.60 - $13,200 - $1,300
Total interest paid = $14,041.60
Therefore, the amount of interest Debra paid on the loan was $14,041.60.
To know more about the financial mathematics visit:
brainly.com/question/28221298
#SPJ1
0 8. A function f(x) is said to have the jump discontinuity at a point x = a if lim a)x+a+ c) x→a+ f(x) = lim x→a+ f(x) = lim x→a¯ lim X-a f(x). b) lim x→a f(x) = lim x→a¯ _ f(x) f(x) = f(a) d) lim f(x) → +00 x→a+
Answers
The correct option for the given question is c) lim x→a¯ f(x) = f(a) when the function f(x) is said to have jump discontinuity at a point x=a.
What is jump discontinuity?Jump continuity is a concept in calculus that describes the behaviour of a function at a specific point where the function jumps from one value to another value without any intermediate values. In other words, a function is considered jump continuous at a point if the function approaches a finite limit from both the left and right sides of that point, but the function values on the left and right sides of the point are not equal.
According to the given information:
The correct notation for the left-hand limit as x approaches a from the left side is lim x→a¯, where the horizontal line above the "a" indicates approaching from the left side.
The statement "lim x→a¯ f(x) = f(a)" means that the limit of f(x) as x approaches a from the left side is equal to the value of f(a) at x = a. This indicates that the function f(x) has a jump discontinuity at x = a, where the function jumps from one value to another value at that specific point.
To know more about jump discontinuity visit: https://brainly.com/question/12644479
#SPJ1
what is the system of equations shown in the graph?

Answers
Answer:
bugo.ka pag answer bobo ka bah ha wag kanang umasa dito piste ka
A=1/2bh solve for b.
Answers
Answer:
2A /h = b
Step-by-step explanation:
A=1/2bh
Multiply each side by 2
2A = 2*1/2bh
2A = bh
Divide each side by h
2A/h = bh/h
2A /h = b
Step-by-step explanation: First, get rid of the fraction by
multiplying both sides of the equation by 2.
That gives us 2A = bh.
Don't be thrown off by the capital A in this problem.
Just treat it like any other variable.
To get b by itself on the right side of the equation, we now simply
divide both side of the equation by h and our answer is 2A/h = b.
Take a look back at the formula now in the original problem.
Does it look familiar?
It's the formula for the area of a triangle.
Take a look at the image I have provided below.
I have made the "b" purple so you
can see that we are solving for it.

Please answer this correctly without making mistakes

Answers
Answer:
1 ft 3 in
Step-by-step explanation:
4 + 11 = 15
15 inches in feet is 1 foot 3 inches
Brainlyest to first correct. Report to incorrect
Add.
(−5x^4+6x^3−43)+(6x^5−x^2+12x+12)
Express the answer in standard form.
Enter your answer in the box.
Answers
Answer:
6x^5-5x^4+6x^3-x^2+12x-31
Step-by-step explanation:
(−5x^4+6x^3−43)+(6x^5−x^2+12x+12)
To add, combine like terms
6x^5-5x^4+6x^3-x^2+12x-43+12
6x^5-5x^4+6x^3-x^2+12x-31
Standard form means from the highest power of x to the lowest power of x
Write the slope of tangent and normal to the curve y=f(X) at (x1,y1).
Answers
The slope of the tangent line is:
a = (df(x1)/dx)
The slope of the normal one is:
a' = -1/(df(x1)/dx)
How to find the slope of the tangent line?For any function y = f(x), we define the slope of the tangent line at the point x as the derivative evaluated in x.
So if we want to find the slope of the tangent line at the point (x1, y1), we just need to differentiate and evaluate in x1, then the slope will be given by:
df(x1)/dx
And the normal slope is the slope of the perpendicular line, two slopes are perpendicular if the product is -1, then:
a*(df(x1)/dx) = -1
a = -1/(df(x1)/dx)
Where df/dx reffers to the derivative of f(x).
Learn more about tangent lines at:
https://brainly.com/question/30162650
#SPJ1
Lydia has four straws of different lengths, and she is trying to form a right triangle. The lengths are 8, 9, 15, and 17 units. Which three lengths should she use? Justify your answer.
Answers
The set of 3 lengths that make a right triangle is {8, 15, 17}
Which three lengths should she use?Remember that for any right triangle, the sum of the squares of the two shorter sides must be equal to the square of the longer side.
So if the 3 sides are A, B, and C, such that:
A < B < C
We will have:
A² + B² = C²
Now you only need to try sets of 3 values in that equation, if we use: 8, 15, and 17 we will have:
8² + 15² = 17²
289 = 289
That equationis true, thus, these 3 lengths make a right triangle.
Learn more about right triangles at:
https://brainly.com/question/2217700
#SPJ1
Given a2 = 7 and a5 = −56 of a geometric sequence, what is the recursive equation for the nth term? a an = 2(an − 1), a1 = 3.5 b an = 2(an − 1), a1 = −3.5 c an = −2(an − 1), a1 = 3.5 d an = −2(an − 1), a1 = −3.5
Answers
Answer: an=-2(an-1), a1=3.5
Step-by-step explanation: took the quiz and got it right
An = -3.5(-2)ⁿ⁻¹ and -3.5 are the Nth and first terms of the above sequence, respectively.
What is Geometric sequence?Every term in a geometric series is obtained by multiplying the term before it by the same number. Aₙ = a₁ rⁿ⁻¹ is the general phrase for it. The common ratio is denoted by the number r.
Given a₂ = 7 and a₅ = −56 for a geometric sequence.
From the general formula of geometric sequence's nth term
Aₙ = a₁ rⁿ⁻¹
Thus
A₂ = 7 = a₁ r²⁻¹ = a₁ r
A₅ = -56 = a₁ r⁵⁻¹ = a₁ r⁴
comparing both equations
A₂/ A₅ = a₁ r / a₁ r⁴
=> -7/56 = 1/r³
=> r³ = -8
=> r = -2
putting this value of r in equation 1
a₁ = -7/2
Hence, for the nth term of the sequence
Aₙ = -7/2(-2)ⁿ⁻¹
Therefore, the Nth term and the first term of the given sequence are Aₙ = -3.5(-2)ⁿ⁻¹ and -3.5 respectively.
Learn more about geometric sequence here:
https://brainly.com/question/3985139
#SPJ2
Which is the largest ratio?
5/36, 2:9, 3 to 18, 1:3
Answers
The largest ratio, among the following ratios: 5/36, 2:9, 3 to 18, 1:3 is 1:3.
How the largest ratio is determined:The ratio refers to the relative size of one quantity compared to another.
The ratio, which is the quotient of two quantities or values, can be expressed as a decimal, percentage, or fraction. We can also express the ratio in its standard form (:).
Given Sum of Equivalent
Ratio Ratios Ratios
5/36 36 13.89% or 0.1389
2:9 11 18.18% or 0.1818
3 to 18 21 14.28% or 0.14.28
1:3 4 25% or 0.25
Thus, we can conclude that 1:3 is the largest ratio amont the others.
Learn more about ratios at https://brainly.com/question/2328454.
#SPJ1