(-5,4); m=3
what is the equation of the line?​

Answer:

4 with a remainder of 2.

Step-by-step explanation:

Answer:

Step-by-step explanation:

From the given information:

Let the first expression that contains two digit number i.e. after multiplying them together their result is greater than 8 but less than 10, let those numbers be:

= 5.5 × 1.5

= 8.25

And another expression that is different from the first but exhibit the same properties to be:

= 3.5 × 2.5    (since both entities are two digit numbers)

= 8.75

Answer:

(a) Vector equation of line: r = (5, 1, 3) + t(1, 4, -2). Parametric equations of line: x = 5 + t, y = 1 + 4t, z = 3 - 2t. Two other points on line: (6, 5, 1) and (4, -3, 5).

(b) Parametric equations of line: x = 2 + t, y = 4 - 5t, z = -3 + 4t. Symmetric equations of line: (x-2)/1 = (y-4)/-5 = (z+3)/4. Point of intersection of line with xy-plane: (2, 4, 0).

(3) Equation of plane: 2x + 3y + 4z = 11. Intercepts of plane: x-intercept: (5.5, 0, 0), y-intercept: (0, -2, 0), z-intercept: (0, 0, 1.5).

(4) Equation of plane: 2x - y + 2z = 3. Intercepts of plane: x-intercept: (1.5, 0, 0), y-intercept: (0, 3, 0), z-intercept: (0, 0, 1.5).

Step-by-step explanation:

(a) The vector equation of the line that passes through the point (5, 1, 3) and is parallel to the vector i + 4j – 2k is:

r = (5, 1, 3) + t(1, 4, -2)

The parametric equations of the line are:

x = 5 + t

y = 1 + 4t

z = 3 - 2t

Two other points on the line are:

(6, 5, 1) when t = 1

(4, -3, 5) when t = -2

(b) The parametric equations of the line that passes through the points A(2,4, -3) and B(3,-1, 1) are:

x = 2 + t

y = 4 - 5t

z = -3 + 4t

The symmetric equations of the line are:

(x-2)/1 = (y-4)/-5 = (z+3)/4

The line intersects the xy-plane when z = 0. Substituting z = 0 into the parametric equations, we get:

x = 2 + t

y = 4 - 5t

0 = -3 + 4t

Solving for t, we get t = 3/4. Substituting t = 3/4 into the parametric equations, we get the point of intersection:

x = 2 + 3/4 = 11/4

y = 4 - 5(3/4) = 1/4

z = 0

(3) An equation of the plane through the point (2, 4, -1) with normal vector n= (2,3,4) is:

2x + 3y + 4z = 11

The intercepts of the plane are:

x-intercept: 2x + 3y + 4z = 0, y = z = 0, x = 5.5

y-intercept: 2x + 3y + 4z = 0, x = z = 0, y = 3.66667

z-intercept: 2x + 3y + 4z = 0, x = y = 0, z = 2.75

(4) An equation of the plane that passes through the points P(1,3,2), Q(3,-1,6), and R(5,2,0) is:

2x - y + 2z = 3

The intercepts of the plane are:

x-intercept: 2x - y + 2z = 0, y = z = 0, x = 1.5

y-intercept: 2x - y + 2z = 0, x = z = 0, y = -2

z-intercept: 2x - y + 2z = 0, x = y = 0, z = 1.5

Learn more about parametric equations.

https://brainly.com/question/32656332

#SPJ11

In terms of relative growth rate Exponential growth is characterized by a constant relative growth rate.

Exponential growth is the process of increasing quantity over time. Occurs when the instantaneous rate of change of a quantity over time is proportional to the quantity itself. The exponential growth model has the form

y (t) = C eᵏᵗ, where k is the rate constant.

Relative Growth Rate:Relative growth rate (RGR) is the rate of growth relative to size. That is, the rate of growth per unit time relative to the size at that point in time and y'(t)/y(t) is the relative growth rate of a function y at time t.

1/y dy/dt = 1/y d(C eᵏᵗ)/dt

= 1/y(kC eᵏᵗ)

= 1/y ( ky) ( since, y (t) = C eᵏᵗ)

= k

Therefore a constant relative growth rate.

To learn more about Exponential growth model, refer:

https://brainly.com/question/13223520

#SPJ4

Complete question:

In terms of relative growth rate, what is the defining property of exponential growth? Choose the correct answer below.

A. The relative growth rate at time t is the slope of the exponential function at time t.

B. dy dt If y represents a population, then the relative growth rate can be represented by dy/dt

C. The relative growth rate is proportional to the size of the population

D. The relative growth rate is constant.

A statistically significant negative correlation coefficient of r = -0.91 was obtained from a sample of n = 30. This indicates a strong and significant negative relationship between the variables.

The researcher conducted a correlation analysis and found a correlation coefficient (r) of -0.91, which indicates a strong negative relationship between the variables under investigation. The negative sign indicates that as one variable increases, the other variable tends to decrease, and vice versa.

The statistical significance of the correlation coefficient is crucial in determining whether the observed relationship is likely to be due to chance or if it truly exists in the population. In this case, the report should highlight that the obtained correlation coefficient is statistically significant.

To establish statistical significance, the researcher likely conducted a hypothesis test, such as a t-test or a permutation test, to determine the probability of obtaining a correlation coefficient as extreme as -0.91 under the null hypothesis of no correlation. Since the obtained correlation coefficient is statistically significant, it suggests that the observed negative relationship is unlikely to be a result of chance and provides evidence for a genuine association between the variables in the population from which the sample was drawn.

Reporting this finding is important for conveying the strength and significance of the negative relationship to the readers, providing valuable insights for further analysis and interpretation of the variables under investigation.

Learn more about correlation coefficient here:

https://brainly.com/question/29704223

#SPJ11

Answer:

620 cm2

Step-by-step explanation:

The base of the prism is a rectangle with 24 cm x 10 cm, so the base area is:

B = 24 * 10 = 240 cm2

The cross section is a triangle with base = 24 cm and height = 5 cm, so its area is:

C = 24 * 5 / 2 = 60 cm2

The sides are rectangles with 10 cm x 13 cm, so their area is:

D = 10 * 13 = 130 cm2

The total surface area is:

S = B + 2C + 2D = 240 + 2*60 + 2*130 = 620 cm2

Answer:

13 years old

Step-by-step explanation:

2020 - 2007 = 13

Answer: 85.1

Step-by-step explanation:

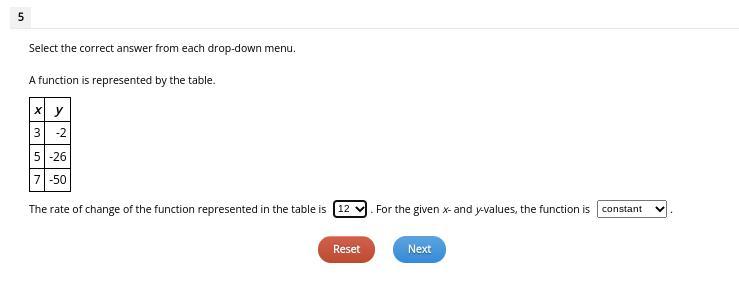
Answer:

so sorry i don't know the answer

Answer:

0.5*13*15 = 97.5 square units

Step-by-step explanation:

If the diagonals of the rhombus are 13 and 15 then its area is 0.5*13*15 = 97.5 square units

The area of the rhombus is,

A. 97.5 square units

To multiply means to add a number to itself a particular number of times. Multiplication can be viewed as a process of repeated addition.

Given that;

Rhombus ABCD with the following dimensions:

diagonal 1 (d1) = BD = 15

diagonal 2 (d2) = AC = 13

AB = 9.9

Hence, Area of the rhombus;

Since we know the length of the 2 diagonals, area of the rhombus can be calculated using;

A = 1/2 × d1 × d2

A = 1/2 × 15 × 13

A = 1/2 × 195

A = 97.5 square units

Thus, The area of the rhombus is,

A. 97.5 square units

Learn more about the multiplication visit:

https://brainly.com/question/10873737

#SPJ7

Answer:

Step-by-step explanation:

The slope intercept form of a line is y=mx+b where m=slope and b=y intercept so we can solve for y from what you were given.

-2y=-16x+8

y=8x-4

So the slope is 8 and the y intercept is -4.

The given statement, "The nullspace of a nonzero 4 x 4 matrix cannot contain a set of four linearly independent vectors" is False.

Explanation: Null space is a linear algebra term used to refer to the set of all vectors that are mapped to the null vector. The solution of Ax=0 is also referred to as the null space or kernel of A. Since the matrix is of size 4x4 and non-zero, it must have at least one non-zero eigenvalue. Any non-zero vector multiplied by this non-zero eigenvalue will result in a non-zero vector, even if it is in the nullspace of the matrix. Because of this, a non-zero 4 x 4 matrix's nullspace can have four or fewer linearly independent vectors.

Hence, the statement "The nullspace of a nonzero 4 x 4 matrix cannot contain a set of four linearly independent vectors" is false.

Learn more about nullspace visit:

brainly.com/question/31745967

#SPJ11

Answer:

There are about 568 miles left of the trip

Step-by-step explanation:

Notice that the 830 miles direct trip would be the hypotenuse of a right angle triangle. The captain goes due North for 605 mi, and then needs to turn west.

Please see the attache image to guide you.

So the question is what is the length of the third side (x) of that right angle triangle which accounts for the distance that has to be sailed due west. So we use the Pythagorean theorem:

\(hyp^2=leg_1^2+leg_2^2\\830^2=605^2+x^2\\x^2=830^2-605^2\\x=\sqrt{830^2-605^2} \\x=568.22\,\,mi\)

Answer: 702

Step-by-step explanation:

From the question, we are informed that the parking lot of a theater has a capacity of 1118 cars and that on Monday, the ratio of the empty parking spots to occupied parking spots is 16:27.

Based on the ratio given, the number of cars that were parked there on that day will be:

= 27/(16 + 27) × 1118

= 27/43 × 1118

= 27 × 26

= 702 cars

The solution to the given initial value problem using Laplace transforms is x(t) = (8/65)cos(7t) + (57/65)sin(7t).

To solve the given initial value problem using Laplace transforms, we first take the Laplace transform of the differential equation and apply the initial conditions.

Applying the Laplace transform to the differential equation x" + x = 8cos(7t), we get:

s^2X(s) + X(s) = 8/(s^2 + 49)

Now, substituting the initial conditions x(0) = 1 and x'(0) = 0, we can solve for X(s):

s^2X(s) + X(s) = 8/(s^2 + 49)

X(0) = 1

sX(0) = 0

Simplifying the equations, we find:

X(s) = (8/(s^2 + 49)) / (s^2 + 1)

X(0) = 1

Using partial fraction decomposition, we can express X(s) as:

X(s) = (8/65) * (1 / (s^2 + 1)) + (57/65) * (s / (s^2 + 1))

Taking the inverse Laplace transform, we obtain the solution:

x(t) = (8/65)cos(7t) + (57/65)sin(7t)

Thus, the solution to the given initial value problem is x(t) = (8/65)cos(7t) + (57/65)sin(7t).

To learn more about “Laplace transform” refer to the https://brainly.com/question/29583725

#SPJ11

13 is the value of z from the given figure.

The properties of the triangle are:

From the given figure,

∠ABE + ∠EBC =. 180

∠EBC = 180- ∠ABE

∠EBC = 180 - 9z -----(1)

∠ECB + 115 = 180

∠ECB = 65-----(2)

IN ∆EBC

∠BEC + ∠ECB + ∠EBC = 180

4Z + 180- 9Z + 65 = 180 ---------(from eq i & ii)

-5Z = -65

Z = -65/-5y

Z = 13

The value of z from the given figure is 13.

Learn more about triangles here:

https://brainly.com/question/2773823

#SPJ1

Complete question:

The factor of the expression will be (x + 1) and (x + 1). Then the product of two binomials will be (x + 1) and (x + 1).

It is a method for dividing a polynomial into pieces that will be multiplied together. At this moment, the polynomial's value will be zero.

The expression is given below.

⇒ x² + 2x + 1

Factorize the expression, then the factor of the expression will be

⇒ x² + x +  x + 1

⇒ x(x + 1)x + 1(x + 1)

⇒ (x + 1)(x + 1)

⇒ (x + 1)²

The product of two binomials will be (x + 1) and (x + 1).

More about the factorization link is given below.

https://brainly.com/question/6810544

#SPJ1

Francesca salary in the first year is $169684.14

Let us assume that P be the first salary of Francesca.

The salary increases by 2% every year.

Let A be the amount she earns after n years at a rate of r.

Using the formula for the compound interest is:

A = P (1 + r)ⁿ

Here, A = $187345

n = 5 years

R = 2%

So, r = 0.02

We need to find the value of P

substituting these values in above equation we get,

187345 = P (1.02)⁵

187345 = 1.10408 × P

P = $169684.14

Therefore,her salary in the first year is $169684.14

Learn more about equation here:

https://brainly.com/question/28243079

#SPJ4

The complete question is:

Each year, Francesca earns a salary that is 2 percent higher than her previous year's salary. In her first 5 years at this job, she earned a total of $187,345. What was Francesca's salary in her 1st year at this job?

Answer:

x = 6

x = 0

Step-by-step explanation:

identify the coefficients

\(9x^2-54x=0\\a=9\\b=-54\\c=0\)

coefficient a = 1

\(9x^2-54x=0\\9/9x^2-54x/9=0/9\\x^2-6x=0\\\rightarrow a=1\\\rightarrow b=-6\\\rightarrow c=0\)

complete the square

\(b=-6\\(\frac{b}{2})^2=(\frac{-6}{2})^2\\(\frac{x}{y})^2=\frac{x^2}{y^2}\\=(\frac{-6}{2})^2=\frac{-6^2}{2^2}\\\frac{-6^2}{2^2}=\frac{36}{4}\\36 \div 4=9\\\\\downarrow\\\\x^2-6x=0\\x^2-6x+9=0+9\\x^2-6x+9=9\\\frac{b}{2}\\\frac{b}{2}=\frac{-6}{2}\\\frac{b}{2}=\frac{\left(-3\cdot 2\right)}{\left(1\cdot 2\right)}\\\frac{b}{2}=-3\\x^2-6x+9=9\\(x-3)^2=9\\\)

solve for x

\((x-3)^2=9\\\sqrt{(x-3)^2}=\sqrt{9}\\x-3=+-\sqrt{9}\\x-3+3=3+-\sqrt{9}\\x=3+-\sqrt{9}\\x=3+-3\\x_1=6\\x_2=0\)

≡ In the end, you have 2 values for x, because you can either subtract 3 by 3 or add 3 by 3 to get six or zero.

To compute the area of the region, you need to integrate over the limits from 0 to π/4 (not π/2) since that's the angle range covered by the portion of the curve y = x that lies within the first quadrant.

To determine the area of the region in the first quadrant bounded by the y-axis, the line y = x, and the two circles x^2 + y^2 = 4 and x^2 + y^2 = 16, we need to analyze the intersection points of these curves and identify the appropriate limits of integration.

Let's start by visualizing the problem. Consider the following description:

The y-axis bounds the region on the left side.

The line y = x forms the right boundary of the region.

The circle x^2 + y^2 = 4 is the smaller circle centered at the origin with a radius of 2.

The circle x^2 + y^2 = 16 is the larger circle centered at the origin with a radius of 4.

To find the intersection points between these curves, we can set their equations equal to each other:

x^2 + y^2 = 4

x^2 + y^2 = 16

Subtracting the first equation from the second, we get:

16 - 4 = y^2 - y^2

12 = 0

This equation has no solutions, indicating that the circles do not intersect. Therefore, the region bounded by the circles is empty.

Now let's consider the region bounded by the y-axis and the line y = x. To find the limits of integration for the area calculation, we need to determine the x-values at which the line y = x intersects the y-axis.

Substituting x = 0 into the equation y = x, we find:

y = 0

Thus, the line intersects the y-axis at the point (0, 0).

To calculate the area of the region, we integrate with respect to x from the point of intersection (0, 0) to the point of intersection of the line y = x with the circle x^2 + y^2 = 4.

To find the x-coordinate of this intersection point, we substitute y = x into the equation of the circle:

x^2 + (x)^2 = 4

2x^2 = 4

x^2 = 2

x = ±√2

Since we are dealing with the first quadrant, the positive value, x = √2, represents the x-coordinate of the intersection point.

Therefore, the limits of integration for the area calculation are from x = 0 to x = √2, which corresponds to the angle range of 0 to π/4.

In summary, to compute the area of the region, you need to integrate over the limits from 0 to π/4 (not π/2) since that's the angle range covered by the portion of the curve y = x that lies within the first quadrant.

To know more about circles, visit the link : https://brainly.com/question/24375372

#SPJ11

Answer:

85

Step-by-step explanation:

Answer:

1 - 330

Blue one  - 424

Yellow one- 181.6

Step-by-step explanation:

Answer:

\(529\pi\) cm²

Step-by-step explanation:

\(d=46 \implies r=23 \\ \\ A=\pi r^2=\pi (23^2)=529\pi\)

A. The y-intercept is at the point (0, 0).

B.  The zeros are x = 0 or x = ±√5

C.

A) The y-intercept of this graph is the point where x = 0.

When  x = 0, the value of y

= 0^4 - 5*0^2

= 0.

Therefore, the y-intercept is at the point (0, 0).

B) The x-intercepts/roots of the function are the values of x that make y = 0.

To find these values, we need to set y = 0 and solve for x:

x⁴ - 5x² = 0

x⁴ = 5x²

x² = x⁴/5

x = ±√(x⁴/5)

We can simplify this expression by factoring x² out of both sides:

x²(x² - 5) = 0

x² = 0 or x² - 5 = 0

x = 0 or x = ±√5

hence the x-intercepts are at x = 0 and x = ±√5.

C) The end behavior of this function refers to how the graph of the function approaches the x-axis as x approaches positive infinity and negative infinity.

x → ∞ f(x) → ∞

x → -∞ f(x) → -∞

D) The graph is attached

Learn more about zeros at:

https://brainly.com/question/29415775

#SPJ1

Answer:

q = 9

Step-by-step explanation:

we can see it is a parallelogram so opposite sides are equal.

q - 3 = 6 ( being opposite sides of parallelogram)

q = 6 + 3

q = 9

Answer:

4+6i

Step-by-step explanation:

Distribute the 2 to the 3i

You get 6i, you can't add 4 to 6i because 4 is real and 6i is imaginary.

It's a complex imaginary number

Real

\(\\ \sf\longmapsto 4+3i^2\)

\(\\ \sf\longmapsto 4+3(-1)\)

\(\\ \sf\longmapsto 4-3\)

\(\\ \sf\longmapsto 1\)

Answer: x = -33

Step-by-step explanation:

27 + 3 = 30

-3 - 30 = -33

Answer:

-33

Step-by-step explanation:

I already took it TwT

The answer to the given question is as follows:

The given information is to be analyzed and observed. The findings are as follows: Most students prefer to learn English because it is a global language and used worldwide, making it the most popular international language among the students. The increasing importance of China and its economy may be the reason why students are taking up Chinese courses. Due to this, Chinese is the second most studied international language after English. A few students are interested in taking up French courses as compared to other courses. Italian is the least preferred international language among the students.

To know more about analysis refer here:

https://brainly.com/question/29926939

#SPJ11

The measure of the bisected angles are-

The given condition is;

Ray EB is the angle bisector of ∠DEF.

Thus,

∠DEB = ∠EBF

∠DEB = 5x - 5 and ∠EBF = x + 7,

Equating both angles;

5x - 5 = x + 7

Solving;

4x = 12

x = 3

Put the value of x in the angles;

∠DEB = 5x - 5

∠DEB = 5×3 - 5

∠DEB = 15 - 5 = 10

and,

∠EBF = x + 7

∠EBF = 3 + 7

∠EBF = 10.

Therefore, the measure of the bisected angles are found as 10° each.

To know more about the angle bisector. here

https://brainly.com/question/24334771

#SPJ9