4x = 16
Step by step please
Pre algebra
Answers
Answer:
Step-by-step explanation:
Just divide four into sixteen. You should get x=4. To check, multiply 4 by 4 (4 x 4), and you should get 16.
Decide by 4 on each side cause it has the variable x
4x/4 = 16/4
4x decide by 4 is 0 so it will leave it with x
Then 16 divided by 4 is 4
So your answer will be x=4 :)
Related Questions
31 19. The oatmeal container shown has a diameter of 3 inches and a height of 9 inches. Which of the following statements are true? Select all that apply. The area of each base is exactly 97 square inches. The volume of the container is exactly 20.251 cubic inches. The volume of the container to the nearest tenth is about 63.6 cubic inches. gn

Answers
Given Data:
The diameter of the container is 3 inches.
The height of the container is 9 inches.
The area of base can be determined as,
\(\begin{gathered} A_b=\frac{\pi}{4}d^2 \\ =\frac{\pi}{4}(3in)^2 \\ =2.25\pi in^2 \end{gathered}\)Thus, option (i) is incorrect.
The volume can be determined as,
\(\begin{gathered} V=A_bh \\ =2.25\pi\times9in^3 \\ =20.25\pi in^3 \end{gathered}\)Thus, option (ii) is correct.
The volume of the container the the nearest tenth can be determine as,
\(\begin{gathered} V=20.25\pi in^3 \\ =63.6in^3 \end{gathered}\)Thus, option (iii) is correct.
Thus, only option (ii) and (iii) are correct.
Which equation describes the same line as y - 6 = -4(x + 1)?
A. y= -4x+2
B. y=-4x-4
C. y = -4x+7
D. y=-4x+3
Answers
We first distribute the -4 in the parenthesis.
-4(x+1) —> -4x - 4
Then we add 6 to both sides to get rid of it on the left. The right side then becomes:
-4x + 2
This is because -4 + 6 is 2
So, we are left with:
y = -4x +2
hi brainly user! ૮₍ ˃ ⤙ ˂ ₎ა
⊱┈────────────────────────┈⊰
\(\large \bold {ANSWER}\)
\(\large \boxed { \large \sf \green{ A. y= -4x+2 }}\)
\(\large \bold {SOLUTION}\)
Convert y - 6 = -4(x + 1) to slope intercept form.
First thing that we need to do is to distribute -4 to all terms within the parenthesis properly.
y - 6 = -4(x + 1) = -4x - 4y - 6 = -4x - 4Hence, we will add 6 both sides:
y - 6 + 6 = -4x - 4 + 6 y = -4x + 2Therefore, the equation describes the same line as y - 6 = -4(x + 1) is y = -4x + 2. (letter A.)
Solve the proportion using the multiplication property of equality.
E 100
OL
m =
(Type an integer or a decimal.)

Answers
the ratio of dividends to the average number of common shares outstanding is:
Answers
The ratio of dividends to the average number of common shares outstanding is known as the dividend yield. It is a measure of the return on an investment in the form of dividends received relative to the number of shares held.
To calculate the dividend yield, you need to divide the annual dividends per share by the average number of common shares outstanding during a specific period. The annual dividends per share can be obtained by dividing the total dividends paid by the number of outstanding shares. The average number of common shares outstanding can be calculated by adding the beginning and ending shares outstanding and dividing by 2.
For example, let's say a company paid total dividends of $10,000 and had 1,000 common shares outstanding at the beginning of the year and 1,500 shares at the end. The average number of common shares outstanding would be (1,000 + 1,500) / 2 = 1,250. If the annual dividends per share is $2, the dividend yield would be $2 / 1,250 = 0.0016 or 0.16%.
In summary, the ratio of dividends to the average number of common shares outstanding is the dividend yield, which measures the return on an investment in terms of dividends received per share held.
To know more about dividend, here
brainly.com/question/3161471
#SPJ11
Hi! can someone help with these questions? I’ll mark brainliest :) Have a nice day and thank you!

Answers
Answer:
7. 32
8. 68
9. 16
10. 29
Step-by-step explanation:
7. =90°-58
=32
8. =68°
9. = (1 + 5x)°
= (1 + 5(16)°
=81
10. = (2x + 3)°
= (2(29) + 3)°
= 61°
Part A: Joseph runs 212 miles on Monday. Each day after that, he runs the same 113 mile route every morning. His goal is to run at least 6 miles by the end of the week. Which inequality represents the least number of days after Monday that Joseph needs to run to reach his goal?
Part B: What is the least number of days after Monday that Joseph needs to run to reach his goal?

Answers
Answer: 325
Step-by-step explanation 212 + 113 = 325
Answer:
Part A - C, 2 1/2 + 1 1/3 x > 6
Part B - number of days = 3
Reduce 4hours 20minutes by 12%
Answers
Step-by-step explanation:
by 12 % seconds
1% of 280 is 2.8
12% of 280 is 33.6
So 280 - 33.6 = 246.4 minutes or 4 hours 10 minutes
if pi is 3.142 what is the area of a circle of radius 3.5 cm
Answers
Answer:
38.4895cm²
Step-by-step explanation:
The area=3.142×(3.5cm)²=38.4895cm²
Answer:
The Area of circle is 34.49cm
Step-by-step explanation:
⟼ Area of a circle = \( \sf \pi {r}^{2} \)
where,
π = 3.142r = radius = 3.5cm⟼ Area = (3.142)(3.5)(3.5)
⟼ Area = (3.142)(12.25)
⟼ Area = 38.4895cm
Zola and her friends are going bowling. The bowling alley charges each person a $14 fee to rent shoes, plus $4.50 per game. Which equation represents the cost, y, for each friend to go bowling in terms of the number of games played, x?
Answers
Answer:
X+14=y
Step-by-step explanation:
A pizza restaurant charges $10.00 for 1 pizza, $20.00 for 2 pizzas, and $28.00 for 3 pizzas. Based on this information, how much will the restaurant charge for 4 pizzas?
Answers
Answer: $36.00
For every extra pizza, it will cost eight dollars more. So, the fourth pizza will cost $36.00.
Plz mark brainliest:)
Write 240 0000 in standard form
Answers
Answer:
2.4 × 10^6
Step-by-step explanation:
Answer:
2.4 × 10⁶
Step-by-step explanation:
is it like example, 1000 'one thousand' like that?
i need some help, can anyone help me out ?
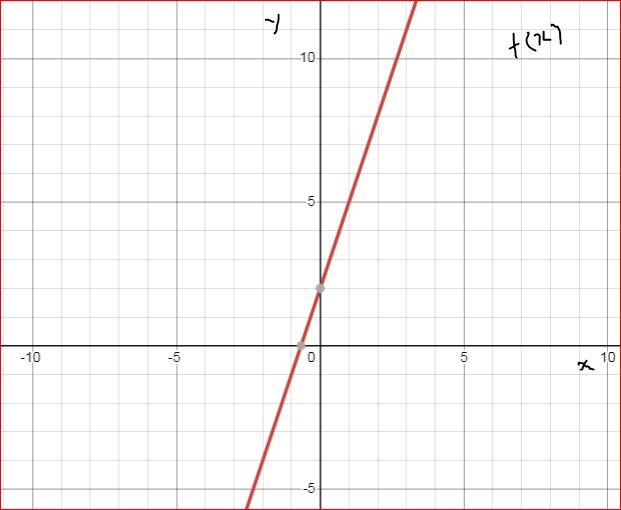
evaluate f(x)=9x+3 when x=5
Answers
Answer:
Step-by-step explanation:
when x=means that you have to put your x into your f(x).
So f(5)=9(5)+3
= 45+3
=48
So f(5)=48
Given:-
\( \sf \: x = 5\)
\( \: \)
Solution:-
\( \sf \: f ( x ) = 9x + 3\)\( \: \)
now put the value of x = 5 in equation :
\( \sf \: f ( 5 ) = 9( 5 ) + 3\)\( \: \)
\( \sf \: f ( 5 ) = 45 + 3\)\( \: \)
\( \fbox{\sf \purple{ f ( 5 ) = 48}}\)\( \: \)
━━━━━━━━━━━━━━━━━━━━━━━
hope it helps:)
How do I find out the exact solutions and approximate solutions?

Answers
Answer: Exact solutions are x = 2√2 and x = -2√2
Approximate solutions are x = 2.828 and x = - 2.828
The attachment shows a portion of the graph with this equation. The "zeros" are the solutions.
Step-by-step explanation: To get values for x, we need to isolate x
2x² -6 = 10 Add 6 to both sides and rewrite the equation as
2x² = 16 divide both sides by 2
x² = 8 Factor 8 as 4(2) so you can "pull out" a square.
To solve for x, take the square roots of both sides.
Remember that square roots are positive and negative values.
\(\sqrt{x^2}\) = ± \(\sqrt{4(2)}\)
x = ± 2√2
x = 2√2 and x = -2√2
We can use a calculator to get approximate values of x.
\(2\sqrt{2}\) = ±2.828427125
Round that to x = 2.828 or 2.83 AND x = - 2.828 for approximate values.

Given a graph for the transformation of f(x) in the format g(x) = f(kx), determine the k value.
Two parabolas open up with f of x passing through negative 3 comma negative 3 and g of x passing through negative 1 comma negative 3
k = negative one third
k = one third
k = −3
k = 3
Answers
k = 1/3 when two parabolas open up with f of x passing through negative 3 negative 3 and g of x passing through negative 1 negative 3.
How is the value of k determined by the given details of the graph?We must consider how the graph of f(x) gets transformed into the graph of g in order to determine the k value for the transformation of f(x) in the format g(x) = f(kx).(x). The vertex of a parabola is its lowest or highest point, depending on whether it is opening up or down, thus we can use the vertex coordinates of f(x) and g(x) to get the k value.
We know the vertices of both parabolas will be the lowest points on each graph because they both open up. Given that g(x) goes through and f(x) passes through (-3, -3), (-1, -3). We stretch or compress f(x) horizontally by a factor of k to convert it to g(x).
The x-coordinates of the parabolas' vertices can be used to determine k because it is where the horizontal compression takes place. The vertex of the quadratic function, f(x), has an x-coordinate of -b/2a, where b and an are its coefficients. Because f(x) is a parabola that is widening up in this instance, an is positive and the vertex's x-coordinate is -(-6)/(2*1) = 3. We can utilize this information to solve for k because the vertex of g(x) has an x-coordinate that is also 3k:
-1 = 3*k*(-3)
-1 = -9k
k = 1/9
Therefore, k = 1/3 is the right response.
Learn more about coordinate geometry here:
brainly.com/question/18269861
#SPJ1

Kaitlin is riding in a bike race that goes through a valley and

Answers
Given:
The altitude for checkpoint 2 is -47.
The altitude for checkpoint 3 is -216.
The altitude for checkpoint 4 is 2,001.
Required:
a) we need to find the altitude of checkpoint 4 higher than checkpoint 2.
b) we need to find the altitude of the top of the hill when that rises 329 feet about the checkpoint 3.
Explanation:
a)
The difference between 2001 and -47 is
\(2001-(-47)=2001+47=2048.\)Checkpoint 4 is 2048 ft higher than checkpoint 2.
b)
Add 329 to the altitude of the checkpoint 3.
\(-216+329=113\)The altitude of the top of the hill is 113 ft.
Final answer:
a) Checkpoint 4 is 2048 ft higher than checkpoint 2.
b) The altitude of the top of the hill is 113 ft.
Find the value of x.

Answers
Answer:
10
Step-by-step explanation:
Answer:
10
Step-by-step explanation:
The angles within the shape will add to 360 degrees. So:
102+100+92+5x+16=360
5x+310=360
5x=50
x=10
solve:
2/3x+15=17
3x+8-x=7
4(2x-6)=2
Answers
Answer:
x = 3
x = (-1)/2
x = 13/4
Step-by-step explanation:
Solve for x:
(2 x)/3 + 15 = 17
Put each term in (2 x)/3 + 15 over the common denominator 3: (2 x)/3 + 15 = (2 x)/3 + 45/3:
(2 x)/3 + 45/3 = 17
(2 x)/3 + 45/3 = (2 x + 45)/3:
1/3 (2 x + 45) = 17
Multiply both sides of (2 x + 45)/3 = 17 by 3:
(3 (2 x + 45))/3 = 3×17
(3 (2 x + 45))/3 = 3/3×(2 x + 45) = 2 x + 45:
2 x + 45 = 3×17
3×17 = 51:
2 x + 45 = 51
Subtract 45 from both sides:
2 x + (45 - 45) = 51 - 45
45 - 45 = 0:
2 x = 51 - 45
51 - 45 = 6:
2 x = 6
Divide both sides of 2 x = 6 by 2:
(2 x)/2 = 6/2
2/2 = 1:
x = 6/2
The gcd of 6 and 2 is 2, so 6/2 = (2×3)/(2×1) = 2/2×3 = 3:
Answer: x = 3
______________________________________________________
Solve for x:
3 x - x + 8 = 7
Grouping like terms, 3 x - x + 8 = (3 x - x) + 8:
(3 x - x) + 8 = 7
3 x - x = 2 x:
2 x + 8 = 7
Subtract 8 from both sides:
2 x + (8 - 8) = 7 - 8
8 - 8 = 0:
2 x = 7 - 8
7 - 8 = -1:
2 x = -1
Divide both sides of 2 x = -1 by 2:
(2 x)/2 = (-1)/2
2/2 = 1:
Answer: x = (-1)/2
_______________________________________
Solve for x:
4 (2 x - 6) = 2
Divide both sides of 4 (2 x - 6) = 2 by 4:
(4 (2 x - 6))/4 = 2/4
4/4 = 1:
2 x - 6 = 2/4
The gcd of 2 and 4 is 2, so 2/4 = (2×1)/(2×2) = 2/2×1/2 = 1/2:
2 x - 6 = 1/2
Add 6 to both sides:
2 x + (6 - 6) = 1/2 + 6
6 - 6 = 0:
2 x = 1/2 + 6
Put 1/2 + 6 over the common denominator 2. 1/2 + 6 = 1/2 + (2×6)/2:
2 x = 1/2 + (2×6)/2
2×6 = 12:
2 x = 1/2 + 12/2
1/2 + 12/2 = (1 + 12)/2:
2 x = (1 + 12)/2
1 + 12 = 13:
2 x = 13/2
Divide both sides by 2:
x = (13/2)/2
2×2 = 4:
Answer: x = 13/4
A choir teacher is dividing 12 sopranos and 9 altos into singing groups. He wants each group to have the same combination of sopranos and altos, with no singers left over. What is the greatest number of groups he can make?
Answers
Answer:
3 groups
Step-by-step explanation:
Sopranos = 12
Altos = 9
Find the highest common factor of 12 and 9
12 = 1, 2, 3, 4, 6, 12
9 = 1, 3, 9
The highest common factor of 12 and 9 is 3
Therefore, the choir teacher can only have 3 groups
12 sopranos / 3 groups
= 4 sopranos per group
9 Altos / 3 groups
= 3 altos per group
Each of the 3 groups Will have 4 sopranos and 3 altos each
one question please help!!! ill mark brainliest!!!!!!!

Answers
Ayden is 1.55 meters tall. At 10 a.m., he measures the length of a tree's shadow to be 21.15 meters. He stands 16.8 meters away from the tree, so that the tip of his shadow meets the tip of the tree's shadow. Find the height of the tree to the nearest hundredth of a meter.
Answers
The height of the tree to the nearest hundredth of a meter = 7.54 m
What are similar triangles?
If two triangles' corresponding angles and sides are identical and their corresponding sides are proportional, or if the ratios between the lengths of the corresponding sides are comparable, then two triangles are said to be similar.
From the figure,
AB= 21.15 meters
AE = AB - BE = 21.15 - 16.8 = 4.35 meters
BC= height, h
The triangles ABC and ADE are similar triangles
So,
AE/DE = AB/BC
4.35 / 1.55 = 21.15/ h
h = ( 21.15 * 1.55 ) / 4.35
= 32.78/4.35 = 7.54 m
So, the height of the tree to the nearest hundredth of a meter = 7.54 m
To learn more about similar triangles from the given link
https://brainly.com/question/2644832
#SPJ1

n a large high school, 37% of the teachers believe that five minutes is not enough time for students to change classes. however, 89% of the students believe that five minutes is not enough time for students to change classes. let and be the sample proportions of teachers and students, respectively, who believe that five minutes is not enough time for students to change classes. suppose 28 teachers and 100 students are selected at random and asked their opinion on the amount of time students have to change class. which of the following is the correct shape and justification of the sampling distribution of ? bimodal because one population proportion is centered at 0.37, while the other is centered at 0.89 approximately normal because the expected number of successes and failures for each sample are all at least 10 not approximately normal because the expected number of successes and failures for each sample are all at least 10 not approximately normal because the expected number of successes and failures for the teacher group are not both at least 10
Answers
The correct statement regarding the shape of the distirbution is given as follows:
Approximately normal because the expected number of successes and failures for each sample are all at least 10.
How to obtain the shape of the distribution?By the Central Limit Theorem, for a proportion p in a sample of size n, the sampling distribution of sample proportion is approximately normal with mean \(\mu = p\) and standard deviation \(s = \sqrt{\frac{p(1 - p)}{n}}\), as long as there are at least 10 successes and 10 failures in the sample, that is, \(np \geq 10\) and \(n(1 - p) \geq 10\).
For teachers, we have that:
np = 0.37 x 28 = 10.36 > 10.n(1 - p) = 0.63 x 28 = 17.64 > 10.For students, we have that:
np = 100 x 0.89 = 89 > 10.n(1 - p) = 100 x 0.11 = 11 > 10.As the conditions are respected, the shape of the sampling distribution is normal.
More can be learned about the central limit theorem at https://brainly.com/question/25800303
#SPJ1
Please help me respond this question

Answers
The correct options are -
g(x) increases at a faster rate over the interval [1,2].f(x) increases at a faster rate over the interval [0,1]Explain the term rate of increase for function?The pace at which one quantity changes in relation to another quantity is known as the rate of change function. Simply said, the rate of increase is calculated by dividing the amount of growth in one item by the equal number of changes in another.The main concept behind rates of growth is how one variable changes as the other grows. Making tables will make this simple to see.For the stated function.
The graph for the function g(x) is given in the question.
As the slope of function g(x) is most steep for the interval [1,2].
Thus, g(x) increases at a faster rate over the interval [1,2].
The graph for the function f(x) is attached,
f(x) = 3x+ 2
From the graph, it is shown that the slope of the function g(x) is most steep for the interval [0,1].
Thus, f(x) increases at a faster rate over the interval [0,1].
To know more about the rate of increase for function, here
https://brainly.com/question/12614336
#SPJ1
HELP DUE IN 15 MINS!
The center of a circle is (3, 2) and a point on the circle is (5, -2). Find the radius and write the equation for the circle.
Answers
Answer:
r = \(2\sqrt{5}\)
\((x - 3)^{2} + ( y - 2)^{2} = 20\)
Is this a live test question or a homework question?
Step-by-step explanation:
the radius is the distance between (3, 2) and (5, -2)
\(r^{2}\) = \({(3 - 5)^{2} + (2 + 2)^{2} }\)
= \((-2)^{2} + 4^{2}\)
= 4 + 16 = 20
r = \(\sqrt{20 } = 2\sqrt{5}\)
Equation of circle: \((x - 3)^{2} + ( y - 2)^{2} = 20\)
Answer:
Radius: \(2\sqrt{5}\)
Equation of circle: \((x-3)^2+(y-2)^2=20\)
Step-by-step explanation:
The radius of a circle is equal to the distance between the center of the circle and any point on the circle. Therefore, we have:
\(r=\sqrt{(5-3)^2+(2-(-2))^2},\\r=\sqrt{2^2+4^2},\\r=\sqrt{20}=\boxed{2\sqrt{5}}\)
The equation of a circle with radius \(r\) and center \((h, k)\) is given by:
\((x-h)^2+(y-k)^2=r^2\).
What we know:
radius of \(2\sqrt{5}\) center at \((3, 2)\)Substituting known values, we get:
\((x-3)^2+(y-3)^2=(2\sqrt{5})^2,\\\boxed{(x-3)^2+(y-2)^2=20}\)
The lines represented by the equations y=-2x-6 and 2y+x=-16 are
Answers
Answer:
Is that the entire question? I want to help but I don't see a full question it looks like half of one
What is the slope of the equation Y = 5/ 4x - 7/4 ?
Answers

x in (-oo:+oo)
y = (5/4)*x-(7/4) // - (5/4)*x-(7/4)
y-((5/4)*x)+7/4 = 0
(-5/4)*x+y+7/4 = 0
y-5/4*x+7/4 = 0 // - y+7/4
-5/4*x = -(y+7/4) // : -5/4
x = (-(y+7/4))/(-5/4)
x = 4/5*(y+7/4)
x = 4/5*(y+7/4)
A van travels 180 miles on 6 gallons of gas. How many gallons will it need to travel 750 miles?
Answers
The van would need approximately 25 gallons of gas to travel 750 miles.
What is a ratio?
A ratio is a quantitative relationship or comparison between two or more quantities. It represents the relative sizes or amounts of different things. Ratios are expressed using two numbers separated by a colon (:) or by using a fraction.
To solve this problem, we can set up a proportion using the information provided. Let's denote the number of gallons needed to travel 750 miles as "x." The proportion can be set up as follows:
180 miles / 6 gallons = 750 miles / x gallons
To solve for x, we can cross-multiply and then divide to isolate x:
180 * x = 6 * 750
180x = 4500
x = 4500 / 180
x ≈ 25
Therefore, the van would need approximately 25 gallons of gas to travel 750 miles.
To learn more about ratio and proportion follow the link:
https://brainly.com/question/12024093
#SPJ4
Bowling The time in which games are played determines the cost per game at Super Strike Bowling.
Games played from 1 pm to 4 pm cost $5
Games played after 4 pm and ending before 8 pm cost $6
Games played from 8 pm until the bowling alley closes at midnight cost $8
Write a step function that models the cost for one game where x represents the number of hours after 12 pm.
Kelly and two friends went bowling after school. They each played one game before 4 pm as well as one game after 4 pm. How much did it cost for all three to bowl?
Answers
As per the unitary method, it would cost $33 for Kelly and her two friends to bowl one game before 4 pm and one game after 4 pm each at Super Strike Bowling.
Bowling is a popular recreational activity enjoyed by many people around the world. Super Strike Bowling charges different rates for games played at different times of the day. To model the cost for one game, we can use a step function, where the value of x represents the number of hours after 12 pm. This function is defined as follows:
Cost per game (C) =
$5 if 1 pm ≤ x < 4 pm
$6 if 4 pm ≤ x < 8 pm
$8 if 8 pm ≤ x ≤ 12 am
Now, let's apply this step function to the scenario of Kelly and her two friends bowling. They each played one game before 4 pm, which cost $5 per game, and one game after 4 pm, which cost $6 per game. Therefore, the total cost for one person to play two games is:
Total cost = ($5 per game) + ($6 per game)
= $11
Since there were three people bowling, we can multiply the total cost by 3 to get the cost for all three to bowl:
Cost for all three to bowl = 3 × $11
= $33
To know more about unitary method here
https://brainly.com/question/28276953
#SPJ1
Could someone help? I got my answer, but I want to check if I’m right.

Answers
Hope it helps you........

Determine whether the underlined numerical value is a parameter or a statistic. Explain your reasoning. for a certain movie, 64.3%.
Answers
The underlined numerical value is a statistic.
It represents a percentage of something related to the movie. Statistics refers to the values calculated from a sample of data and can change depending on the sample chosen. Parameters, on the other hand, refer to values calculated from the entire population and do not change .Discussion :In statistics, parameters and statistics are used to describe sets of data.
Suppose we want to estimate the average age of all Americans. The average age of the entire population is the parameter, while the average age of a sample of Americans is a statistic. Therefore, the parameter is a numerical value that describes a population, while the statistic is a numerical value that describes a sample.In the given case, "64.3%" is a percentage, which is calculated from a sample of data related to the certain movie. As the value is calculated from a sample, it is a statistic and not a parameter.
To know more about statistic visit:
https://brainly.com/question/31538429
#SPJ11
Sara simplified an expression as shown.
image
What property of operations did Sara use to get from Step 3 to Step 4?
A.
Subtracting a number is the same as adding its inverse.
B.
Associative Property of Addition
C.
Commutative Property of Addition
D.
The opposite of a sum is the sum of its opposites.
Sara simplified an expression as shown.
image
What property of operations did Sara use to get from Step 3 to Step 4?
A.
Subtracting a number is the same as adding its inverse.
B.
Associative Property of Addition
C.
Commutative Property of Addition
D.
The opposite of a sum is the sum of its opposites.

Answers
Answer:
B. Associative Property of Addition
Step-by-step explanation: