3/8 x 11 =
3/4 x 2 = ​

The probability of product of a, b, c, d, and e is odd after selected randomly them from the set {1, 2, 3, 4, 5} is equal to 189/625.

Set is equal to ,

{1, 2, 3, 4, 5}

To find the probability that the product of a, b, c, d, and e is odd when selected randomly from the set {1, 2, 3, 4, 5},

consider the possible combinations that result in an odd product.

An odd number can be obtained by multiplying an odd number of odd factors.

Since 5 numbers to choose from, each with an equal probability of being selected,

There are two possibilities for the number of odd factors that will result in an odd product,

Selecting all odd numbers (3 odd factors),

There are 3 odd numbers in the set {1, 3, 5}.

The probability of selecting an odd number is 3/5.

Since we are selecting with replacement, the probability of getting 3 odd numbers is (3/5)³.

Selecting one even number and four odd numbers (1 even and 4 odd factors),

There are 2 even numbers (2 and 4) and 3 odd numbers (1, 3, 5) in the set.

The probability of selecting an even number is 2/5, and the probability of selecting an odd number is 3/5.

Since we are selecting with replacement, the probability of getting 1 even number and 4 odd numbers is (2/5) × (3/5)⁴

To find the overall probability, add the probabilities of the two cases,

P(Odd Product)

= P(3 odd factors) + P(1 even and 4 odd factors)

= (3/5)³ + (2/5) × (3/5)⁴

= 27/125 + 162/625

= 189/625

Therefore, the probability that the product of a, b, c, d, and e is odd when selected randomly from the set {1, 2, 3, 4, 5} is 189/625.

learn more about probability here

brainly.com/question/29190096

#SPJ4

The statement "If f(x) is continuous on the closed interval [a, b], then f(x) must have an absolute maximum and an absolute minimum on the interval" is not true if we replace the closed interval [a, b] with the open interval (a, b).

Counterexample: Let f(x) = 1/x on the open interval (0, 1). Since 1/x is continuous on (0, 1), we can apply the Extreme Value Theorem to conclude that f(x) must have an absolute maximum and an absolute minimum on (0, 1).

However, we can see that f(x) does not have an absolute maximum on (0, 1) since as x approaches 0, f(x) approaches positive infinity.

The Extreme Value Theorem states that if a function is continuous on a closed interval, then it must have an absolute maximum and an absolute minimum on that interval.

However, if the interval is not closed, then the theorem does not necessarily hold. The counterexample above shows that the function 1/x does not have an absolute maximum on the open interval (0, 1), even though it is continuous on that interval. Therefore, the statement is not true if we replace the closed interval [a, b] with the open interval (a, b).

For more questions like Closed interval click the link below:

https://brainly.com/question/30273384

#SPJ11

Without the probability distribution, it is not possible to calculate the standard deviation accurately. Thus, none of the provided options can be considered correct without additional information.

To calculate the standard deviation of the returns, we need the probability distribution of the holding-period returns and their corresponding values. Since the probability distribution is not provided in your question, it is not possible to determine the standard deviation.

To calculate the standard deviation, you would typically need the individual returns and their corresponding probabilities. With that information, you can use the formula for calculating the weighted standard deviation:

σ = √[∑(Ri - E(R))^2 * P(Ri)],

where Ri represents the individual returns, E(R) is the expected return, and P(Ri) is the probability of each return.

Without the probability distribution, it is not possible to calculate the standard deviation accurately. Thus, none of the provided options can be considered correct without additional information.

Learn more about probability distribution

brainly.com/question/15930185

#SPJ11

Red Circle:

First find the diameter

We can do that by counting the squares

Diameter:4

The radius is half of the diameter

4/2=2

Radius of the Red Circles:2

Blue Circle:

Diameter: 2

2/2=1

Radius of the Blue Circle:1

Answer:

3$

Step-by-step explanation:

39.80/ 3 =13.2667

3$

The solutions are:  (A U B)' = {1, 7, 8, 9} and (A ∩ B) x A = {(3, 2), (3, 3), (3, 4), (4, 2), (4, 3), (4, 4)}.

a. (A U B)' represents the complement of the union of sets A and B. To find (A U B)', we need to list all the elements in the universal set U that are not in the union of sets A and B. The union of sets A and B, A U B, includes all the elements that are in either set A or set B (or both). So, A U B = {2, 3, 4, 5, 6}. The complement of A U B, (A U B)', will contain all the elements in the universal set U that are not in the set A U B. Therefore, (A U B)' = {1, 7, 8, 9}.

b. (A ∩ B) x A represents the Cartesian product of the intersection of sets A and B with set A. To find (A ∩ B) x A, we need to list all possible ordered pairs that can be formed by selecting one element from the intersection of sets A and B and pairing it with an element from set A. The intersection of sets A and B, A ∩ B, contains the elements that are common to both sets A and B. In this case, A ∩ B = {3, 4}.

Now, we take each element from A ∩ B and pair it with each element from set A. So, (A ∩ B) x A = {(3, 2), (3, 3), (3, 4), (4, 2), (4, 3), (4, 4)}. Therefore, (A U B)' = {1, 7, 8, 9} and (A ∩ B) x A = {(3, 2), (3, 3), (3, 4), (4, 2), (4, 3), (4, 4)}.

To learn more about universal set, click here: brainly.com/question/29792943

#SPJ11

Answer:

2^3 * 3

2^2 * 3

5 * 7

2 * 7^2

The GCF they all share is 1, or 2^0 * 3^0 * 5^0 * 7^0.

We can rewrite these numbers like so:

2^3 * 3^1 * 5^0 * 7^0

2^2 * 3^1 * 5^0 * 7^0

2^0 * 3^0 * 5^1 * 7^1

2^1 * 3^0 * 5^0 * 7^2

Take the lowest powered form of each factor

2^0 , 3^0 , 5^0 , 7^0

Multiply them together

1

Step-by-step explanation:

Given integral is:∫_0^10( sin⁡(x³)/(100-x²) ) dxWe have to evaluate this definite integral.Let u=x³dudu=3x²dxWe can write integral as:∫_0^100( sin⁡u/(100-u^(2/3)) ) (1/3u^(1/3)du)Let v = u^(1/3)dv=(1/3)u^(-2/3)duAlso, u^(2/3) = v².

Now we can rewrite integral using v as:∫_0^10( sin⁡u/(100-u^(2/3)) ) (1/3u^(1/3)du) = ∫_0^(10^3)(sin(v^3)/(300v^4-100))dvLet w = (10v²)/(√(100+300v^6))Now, we have √(100+300v^6) = (10v²)/w, or w√(100+300v^6) = 10v²dw/dv = (60v^5)/(w(100+300v^6)) - (10v^3)/(w^2√(100+300v^6))dw/dv = (2v³(w^2-15))/(w(√(100+300v^6)))dw = 10v²dv/(w^2-15)Rewriting the integral using w:∫_0^(10/√3)sin(w/(10/√3))^2dwUsing half-angle formula :sin⁡(w/(10/√3)))^2=(1-cos(w/(5/√3))))/2We can now rewrite integral as:∫_0^(10/√3)sin(w/(10/√3))^2dw = (1/2)∫_0^(10/√3)(1-cos(w/(5/√3))))/((w^2-15))dw Using integration by parts :Let u = 1-cos(w/(5/√3)), dv = 1/((w^2-15))dwu' = sin(w/(5/√3))(1/√3), v = (1/√15)arctan(w/√15)Substituting values, we get:- (1/2)sin(w/(5/√3))(1/√15)arctan(w/√15)) + (1/2)∫sin(w/(5/√3))(1/√15)(w/(w^2-15))dwNow, we need to evaluate this integral.

To evaluate this integral, let's use substitution, let z = w/(5/√3), or w = (5/√3)z Then, we can rewrite the integral as:(1/2)∫sin(z)dz/(√3(z^2-3)) = (-1/2)∫d(cos(z))/((z^2-3)^(1/2))Let's use substitution, let t = (z/√3), or z = √3tThen, the integral can be rewritten as:(-1/2√3)∫dt/((t^2-1)^(1/2)) = (-1/2√3)ln|t+(t^2-1)^(1/2)|, or (-1/2√3)ln|z+(z^2/3-1)^(1/2.

To know more about evaluate visit:

https://brainly.com/question/14677373

#SPJ11

Answer:

4.777

Step-by-step explanation:

Circumference of a Circle = 2πr

To solve for the radius = Circumference ÷ 2π

Answer = 30 ÷ (2 ×3.14)

Radius = 4.777

To begin solving this problem, we need to determine the dimensions of the rectangular region that the farmer will fence in. Let's say the length of thr uses, is given by the equation:
e rectangle is L and the width is W. The perimeter of the rectangle, which will be the length of fencing the farme
2L + W = 350

Solving for W, we get:

W = 350 - 2L

Next, we need to divide the rectangular region into three smaller rectangles by placing two parallel fences. Let's say the two fences are placed along the length of the rectangle, dividing it into three sections with widths of x, y, and z. Therefore, we have:

L = x + y + z

Now, we can determine the area of the entire fenced-in region by summing the areas of the three smaller rectangles. The area of a rectangle is given by the equation:

Area = Length x Width

Therefore, the total area of the fenced-in region is:

Area = (xW) + (yW) + (zW)

Substituting W = 350 - 2L and L = x + y + z, we get:

Area = (x(350-2(x+y+z))) + (y(350-2(x+y+z))) + (z(350-2(x+y+z)))

Simplifying this equation, we get:

Area = 350(x+y+z) - 2(x^2 + y^2 + z^2)

To maximize the area, we need to take the derivative of this equation with respect to one of the variables (x, y, or z), set it equal to zero, and solve for the variable. This process is too complicated to do by hand, so we will use a calculator or computer program to find the maximum area.

After finding the maximum area, we can determine the dimensions of the region that give us this maximum area. We do this by using the equations we derived earlier:

W = 350 - 2L

L = x + y + z

With the maximum area and these equations, we can solve for the dimensions of the region that give us the maximum area.

In summary, the farmer should fence in a rectangular region with dimensions that maximize the total area of three smaller rectangles created by placing two parallel fences. The maximum area can be found by taking the derivative of the area equation and setting it equal to zero. The dimensions of the region that give us the maximum area can be found by using the equations we derived earlier.

learn more about dimensions here : brainly.com/question/28688567

#SPJ11

Answer:

12 miles

Step-by-step explanation:

Total miles = Length of trail ×

Number of times she rode

Total miles = 3 miles × 4 times

Total miles = 12 miles

Mika traveled a total of 12 miles.

Answer:

28x + 8y + 2 hope that helps

The statement "In practice, we frequently use a continuous distribution to approximate a discrete one when the number of values the variable can assume is countable but large" is false because we use a discrete distribution to approximate a discrete one when the number of values the variable can assume is countable but large.

In probability and statistics, we frequently use a discrete distribution to model a variable that can only take on certain values. A continuous distribution, on the other hand, is used to represent a variable that can take on any value within a given range.

However, when the number of values the variable can take on is countable but large, we usually use a discrete distribution to model it rather than a continuous one. For example, if we're counting the number of heads that come up in a series of coin flips, we would use a discrete distribution (the binomial distribution) rather than a continuous one.

Learn more about continuous distribution https://brainly.com/question/31503218

#SPJ11

The parametric equations for the tangent line at t=2 are:
x(t) = 1 + 2t
y(t) = 3
z(t) = 16 + 12t

To find the parametric equations for the tangent line at t=2, we first need to find the derivative of each coordinate function with respect to t, and then evaluate them at t=2.

1. Differentiate x(t) = (t-1)^2 with respect to t:
dx/dt = 2(t-1)

2. Differentiate y(t) = 3 with respect to t:
dy/dt = 0 (constant function)

3. Differentiate z(t) = 2t^3 - 3t^2 with respect to t:
dz/dt = 6t^2 - 6t

Now, evaluate the derivatives at t=2:

dx/dt(2) = 2(2-1) = 2
dy/dt(2) = 0
dz/dt(2) = 6(2^2) - 6(2) = 12

Next, find the point (x, y, z) at t=2:
x(2) = (2-1)^2 = 1
y(2) = 3
z(2) = 2(2)^3 - 3(2)^2 = 16

The parametric equations for the tangent line at t=2 are:
x(t) = 1 + 2t
y(t) = 3
z(t) = 16 + 12t

To learn more about parametric equations, refer below:

https://brainly.com/question/28537985

#SPJ11

The months of the birthdays in set notation are { January, April, May }

In mathematics, sets are collections of discrete objects or elements.

Set notation describes the many symbols used while navigating between and within sets. The curly brackets symbol is the most basic way to express a set's elements. A = a, b, c, and d is an illustration of a set.

The birthdays of the five friends are January 18, April 19, April 29, May 11, and May 21.

There are two types of ways in which we can write in set notation.

To know more about set notation, visit https://brainly.com/question/28276299

#SPJ1

Answer:

Move all terms to the left side and set equal to zero. Then set each factor equal to zero.

Exact Form:

x=1/5,3

Decimal Form:

x=0.2,3

Step-by-step explanation:

Let y be 1/x. Then 2y = 2/x.

We wish to simplify (1 + y)(2 - 2y)(2y).

(1 + y)(2 - 2y)(2y)

= 4y(1 + y)(1 - y)

= 4y(1 - y²)

= 4y - y³

= 4/x - 1/x³.

P.S. Writing it as (4x² - 1)/x³ is fine.

76 degrees Fahrenheit was found to be equal to approximately 24.4 degrees Celsius using the formula of conversion.

To convert Fahrenheit to Celsius, you can use the following formula:

Celsius = (Fahrenheit - 32) x 5/9

Plugging in 76 degrees Fahrenheit, we get:

Celsius = (76 - 32) x 5/9

Celsius = 44 x 5/9

Celsius = 220/9

Celsius ≈ 24.4

Therefore, 76 degrees Fahrenheit is approximately 24.4 degrees Celsius.

On the Fahrenheit scale, water freezes at 32 degrees Fahrenheit (°F) and boils at 212 °F, at standard atmospheric pressure.

On the Celsius scale, the freezing point of water is defined as 0 degrees Celsius (°C), and the boiling point of water is defined as 100 °C, at standard atmospheric pressure.

Learn more about Fahrenheit scale here brainly.com/question/31139

#SPJ4

Answer:

False

Step-by-step explanation:

No we can't find the square root of negative number.

Hope it helped you

Please Mark me the brainliest

True, free bilirubin is transported by the blood to the liver.

Macrophages begin the disposal of hemoglobin by separating the heme from the globin. They hydrolyze the globin into free amino acids, which can be used for energy-releasing catabolism or recycled for protein synthesis.

Bilirubin

Bilirubin is a red-orange compound that occurs in the normal catabolic pathway that breaks down heme in vertebrates. This catabolism is a necessary process in the body's clearance of waste products that arise from the destruction of aged or abnormal red blood cells.

Hemoglobin

Hemoglobin is a protein in your red blood cells that carries oxygen to your body's organs and tissues and transports carbon dioxide from your organs and tissues back to your lungs.

Learn more about bilirubin here :-

https://brainly.com/question/28287289

#SPJ4

Answer: 60

Step-by-step explanation:

140km: 7 liters

_______: 3 liters

7/2: 3.5

140/2: 120

120/0.5: 60

Answer:

I would say c, all whole numbers are rational

Step-by-step explanation:

Whole numbers can include all the natural numbers along with zero. It starts can from 0, 1, 2, 3, 4, 5, etc. But all whole numbers can not be natural numbers

Answer:

C. All whole numbers are natural

Step-by-step explanation:

Natural numbers are those that are used for counting, and therefore include 1, 2, 3, 4... and so on, but they do not include 0.

Whole numbers are all numbers without a fractional part, and therefore include 0.

This is why not all whole numbers are natural numbers.

Avogadro's number is a unitless object. It represents a number.

What is unit?

The mass of a particular piece of metal or the length of a particular rod are examples of quantities (specific properties) of objects that have historically been used to define measurement units. An object that has no units is known as unitless.

The proportionality factor that connects the number of constituent particles (often molecules, atoms, or ions) in a sample with the amount of material in that sample is called the Avogadro constant, also known as NA or L. It has a precise value of  6.02214076 × 10²³ reciprocal moles and serves as a SI defining constant.

The value of Avogadro's number is 6.02214076 × 10²³.

One mole of the chemical compound consists of 6.02214076 × 10²³ atoms.

To learn more about Avogadro's number, click on the below link:

https://brainly.com/question/26464742

#SPJ4

Answer:

Step-by-step explanation:

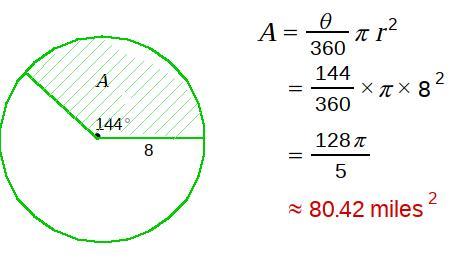
The area of a sector and the properties of circles bounded by a 144° arc in a circle with a radius of 8 miles can be calculated using the formula: Area of sector = (θ/360°) * π * r² where θ is the central angle of the sector and r is the radius of the circle.

In this case, the central angle is 144° and the radius is 8 miles. Plugging these values into the formula, we get: Area of sector = (144°/360°) * π * (8 miles)². Simplifying the equation, we have: Area of sector = (0.4) * π * (8 miles)².

To know more about area of a sector here: brainly.com/question/29055300

#SPJ11

Answer:

Step-by-step explanation:

138° - 80° = 58°

To find the point P on the line y=3x that is closest to the point (50,0), we need to use the formula for the distance between a point and a line. This formula is:

distance = |Ax + By + C| / sqrt(A^2 + B^2)

Where A, B, and C are the coefficients of the equation of the line in the form Ax + By + C = 0.

In this case, the equation of the line y=3x can be written as -3x + y = 0. So we have:

A = -3, B = 1, C = 0

Now we can plug in the values of (50,0) and solve for x and y to find the point P that is closest to it:

distance = |-3x + y| / sqrt((-3)^2 + 1^2)
distance = |-3x| / sqrt(10)

To minimize the distance, we need to minimize |-3x|. This occurs when x = 0. So the point P that is closest to (50,0) is the point (0,0).
To find the point P on the line y = 3x that is closest to the point (50, 0), we need to minimize the distance between P and (50, 0).

Let P(x, y) be a point on the line y = 3x. The distance between P and (50, 0) is given by the distance formula:

D = sqrt((x - 50)^2 + (y - 0)^2)

Since y = 3x, we can rewrite the distance formula as:

D = sqrt((x - 50)^2 + (3x)^2)

To minimize the distance, we can minimize the square of the distance (D^2), as it avoids dealing with the square root:

D^2 = (x - 50)^2 + (3x)^2

Now, we can find the derivative of D^2 with respect to x and set it to 0 to find the critical points:

d(D^2)/dx = 2(x - 50) + 2(3x)^2 * 6x = 0

Solve this equation to find the x-coordinate of the point P. Then, use y = 3x to find the corresponding y-coordinate. Finally, you'll have the coordinates of the point P that is closest to (50, 0).

Visit here to learn more about  derivative : https://brainly.com/question/25324584
#SPJ11

Answer:

answer is b

Step-by-step explanation:

Answer:

-3,3,7 for Acellus

that's it

Remember: a^2 + b^2 = c^2

1

64 + 49 = 113

√113 = \(\sqrt{113}\)

2

100 - 25 = 75

square root of 75 = 5 \(\sqrt{3}\)