3 1/5 greater than 1
Answers
Answer:
When a fraction has a numerator that is greater than or equal to the denominator, the fraction is an improper fraction. An improper fraction is always 1 or greater than 1.
Answer:
3 1/5 > 1
Step-by-step explanation:
Related Questions
The height, h, of an object that is thrown upwards into the air can be represented as h = 0.5at2 + v0t + h0, where a represents the acceleration due to gravity (– 32 ft/s)
2), v0 represents the initial velocity, in feet per second, h0 represents the initial height of the object, in feet, and t represents time, in seconds.
Part A:
A ball thrown upwards from the top of a 64–ft high building with an initial velocity of 48
ft/s.Write an equation that could be used to determine the time, t, in seconds, for the ball to reach the ground. Assume ground level is at a height of 0 ft.
Part B:
Determine the time, t, in seconds, that takes the ball to reach the ground and explain how you determined your answer.
Answers
Answer:
ok so this awnser is v7a
Step-by-step explanation:
What are the projections of the point (0, 3, 3) on the coordinate planes?
On the xy-plane: ( )
On the yz-plane: ( )
On the xz-plane: ( )
Answers
The projections of the point (0, 3, 3) on the coordinate planes are:
On the xy-plane: (0, 3, 0)
On the yz-plane: (0, 0, 3)
On the xz-plane: (0, 3, 0)
The concept of projections onto coordinate planes.
In a three-dimensional Cartesian coordinate system, each point in space is represented by three coordinates: (x, y, z). The xy-plane, yz-plane, and xz-plane are three separate planes that intersect at right angles and divide the three-dimensional space.
When we talk about the projection of a point onto a coordinate plane, we are essentially finding the point on that plane where the original point would "project" onto if we were to drop a perpendicular line from the original point to the plane.
For the point (0, 3, 3), let's consider its projections onto the coordinate planes:
1. Projection on the xy-plane: To find this projection, we set the z-coordinate to zero. By doing so, we "flatten" the point onto the xy-plane, and the resulting projection is (0, 3, 0).
2. Projection on the yz-plane: To find this projection, we set the x-coordinate to zero. By doing so, we "flatten" the point onto the yz-plane, and the resulting projection is (0, 0, 3).
3. Projection on the xz-plane: To find this projection, we set the y-coordinate to zero. By doing so, we "flatten" the point onto the xz-plane, and the resulting projection is (0, 3, 0).
In summary, the projections of the point (0, 3, 3) onto the coordinate planes are:
- On the xy-plane: (0, 3, 0)
- On the yz-plane: (0, 0, 3)
- On the xz-plane: (0, 3, 0)
These projections help us visualize the point's position on each individual plane while disregarding the coordinate orthogonal to that specific plane.
Learn more about coordinate planes here:-
https://brainly.com/question/14568576
#SPJ11
I don’t understand this

Answers
Answer:
find the tan inverse
\( { \tan(?) }^{ - 1} \)
of the value to get the angle.
1d.p = 29.2°

Which Is The Simplified Rational Expression?

Answers
Answer:
1st choice
Step-by-step explanation:
(r² -4r + 5 - r² -2r + 8) / (r - 4)
= (-6r + 13) / (r - 4)
could someone please help with these two questions (I WILL MARK BRAINLAST)
question 1: which of these points lies on the line y = -x + 2
question 2: evaluate: -ab-a if a=-4 and b =-5

Answers
Answer:
1. b
2. -16
Step-by-step explanation:
1. -1+2 is equal to 1 so it works
You plug in each of the coordinates x and y variables on the graph and find one that satisfies the equation.
2. -(-4)(-5) is -20
-(-4) is = 4
-20+4 is -16
An ordinary deck of 52 cards is shuffled. what is the probability that the top four cards have (a) different denominations? (b) different suits?
Answers
The different denomination is 0.6761
The different suits are 0.1055
What are deck of cards consist of?A standard deck of cards has four suites: hearts, clubs, spades, diamonds. Each suite has thirteen cards: ace, 2, 3, 4, 5, 6, 7, 8, 9, 10, jack, queen and king. Thus the entire deck has 52 cards total.
Given:
Deck of cards= 52
Now,
There are 4 denomination in each cards
and there are 4 suits in deck of cards
a) different denominations
=52*48*44*40/(52*51*50*49)
= 0.6761
b) different suits
=52*39*26*13/ /(52*51*50*49)
= 0.1055
Learn more about this concept here:
https://brainly.com/question/17223652
#SPJ4
toni is opening his own starbucks franchise and needs to find the correct dimensions to erect a new sign. The area of his new sign is 16 pi yd^2, what is the circumference of his sign
Answers
The circumference of the sign is 8π yards.
Given that, the area of the sign is 16π yd².Therefore, the radius of the sign is: `r=√(Area/π)=√(16π/π)=√16=4 yards`.So, the circumference of the sign is: `C=2πr=2π(4)=8π yards`.Therefore, the circumference of Toni's new Starbucks franchise sign is 8π yards.
The area of a circle is given by the formula A = πr², where A is the area of the circle and r is the radius. Here, the area of the sign is given as 16π yd². So, we can use the formula A = πr² to find the radius of the sign.r² = A/π = (16π yd²) / πr² = 16The radius of the sign is r = √16 = 4 yards.Now that we know the radius of the sign, we can use the formula for the circumference of a circle to find the circumference of the sign. The circumference of a circle is given by the formula C = 2πr, where C is the circumference of the circle and r is the radius.C = 2πr = 2π(4) = 8π yardsTherefore, the circumference of Toni's new Starbucks franchise sign is 8π yards.
To know more about circumference visit ;-
https://brainly.com/question/28757341
#SPJ11
Considera el descuento indicado en cada tabla y calcula los diferentes porcentajes de descuento del mismo articulo
Answers
The 5% discount is 8.10, Namas divide 42 between the percentage follow me and give me crown.
A discount is a reduction in the price of a product or service offered to customers. It is a common marketing strategy used by businesses to attract customers and increase sales. Discounts can be offered in various forms, such as percentage off, dollar off, or buy-one-get-one-free deals.
Discounts are often used to clear out old inventory, promote new products or services, and to reward customer loyalty. They can be temporary or ongoing, and the amount of the discount can vary depending on the product, service, or promotion. Customers benefit from discounts as they can purchase products or services at a lower price, saving them money. Businesses benefit from discounts as they can increase sales volume, clear out inventory, and attract new customers.
To learn more about Discount visit here:
brainly.com/question/3541148
#SPJ4
Complete Question:-
Consider the discount indicated in each table and calculate the different discount percentages for the same item.

Let u = (1,0, -1), v = (4,3,-2), and w = (2, 3, -2). Find the orthogonal projection of w into the plane spanned by the vectors u and v. Show that the matrix A is orthogonal if and only if its transpose A⁻ is orthogonal.
Answers
The transpose of A⁻¹ is the inverse of the transpose of A⁻¹, which implies that if A⁻¹ is orthogonal, then A is orthogonal. Therefore, we have shown that the matrix A is orthogonal if and only if its transpose A⁻¹ is orthogonal.
To find the orthogonal projection of vector w into the plane spanned by vectors u and v, we need to calculate the projection vector proj_w(uv).
First, we calculate the normal vector n of the plane. The normal vector is obtained by taking the cross product of vectors u and v:
n = u x v
= (1, 0, -1) x (4, 3, -2)
The cross product can be calculated as follows:
n = ((0)(-2) - (-1)(3), (-1)(4) - (1)(-2), (1)(3) - (0)(4))
= (-3, -6, 3)
Next, we normalize the normal vector n to obtain the unit normal vector n:
n = n / ||n||
= (-3, -6, 3) / √(9 + 36 + 9)
= (-3, -6, 3) / √54
= (-1/√6, -2/√6, 1/√6)
Now, we can calculate the projection of vector w onto the plane using the formula:
proj_w(uv) = w - ((w · n) / (n · n)) * n
The dot product of w and n is given by:
w · n = (2)(-1/√6) + (3)(-2/√6) + (-2)(1/√6)
= -2/√6 - 6/√6 - 2/√6
= -10/√6
The dot product of n and n is:
n · n = (-1/√6)(-1/√6) + (-2/√6)(-2/√6) + (1/√6)(1/√6)
= 1/6 + 4/6 + 1/6
= 6/6
= 1
Substituting these values into the projection formula, we have:
proj_w(uv) = (2, 3, -2) - ((-10/√6) / 1) * (-1/√6, -2/√6, 1/√6)
= (2, 3, -2) + (10/√6)(-1/√6, -2/√6, 1/√6)
= (2, 3, -2) + (-10/6, -20/6, 10/6)
= (2, 3, -2) + (-5/3, -10/3, 5/3)
= (2 - 5/3, 3 - 10/3, -2 + 5/3)
= (1/3, 1/3, -1/3)
Therefore, the orthogonal projection of vector w into the plane spanned by vectors u and v is (1/3, 1/3, -1/3).
Now, let's prove the statement that the matrix A is orthogonal if and only if its transpose A⁻¹ is orthogonal.
To prove this, we need to show two conditions:
If A is orthogonal, then A⁻¹ is orthogonal:
If A is orthogonal, it means that A · A⁻¹ = I, where I is the identity matrix.
Taking the transpose of both sides, we have (A · A⁻¹)ᵀ = Iᵀ, which simplifies to (A⁻¹)ᵀ · Aᵀ = I.
This shows that the transpose of A⁻¹ is the inverse of the transpose of A, which implies that if A is orthogonal, then A⁻¹ is orthogonal.
If A⁻¹ is orthogonal, then A is orthogonal:
If A⁻¹ is orthogonal, it means that (A⁻¹) · (A⁻¹)ᵀ = I, where I is the identity matrix.
Taking the transpose of both sides, we have ((A⁻¹) · (A⁻¹)ᵀ)ᵀ = Iᵀ, which simplifies to ((A⁻¹)ᵀ) · (A⁻¹) = I.
Learn more about transpose at: brainly.com/question/32293193
#SPJ11
Question 8 of 25
2 Points
Which of these statements is true for f(x) = 4×(7)^x?
A. It is always decreasing
O B. The y-intercept is (0, 1).
O C. The domain of f(x) is x > 0.
D. The y-intercept is (0,4).
SUBMIT
Answers
Answer: the y-intercept is (0,4)
Step-by-step explanation:
4. What is the rate of change of the linear function that has a graph that passes
through the points (-1, 3) and (-2,-4)?
Answers
The rate of change of the function that has a graph that passes
through the points (-1, 3) and (-2,-4) is slope and is equal to 7.
The slope of a line is outlined because the amendment in y coordinate with relevancy the amendment in x coordinate of that line. cyber web amendment in y coordinate is Δy, whereas cyber web amendment within the x coordinate is Δx. The slope of a line is calculated victimisation 2 points lying on the line. Given the coordinates of the 2 points, we are able to apply the slope of line formula m = y₂ - y₁ / x₂ - x₁ where (x₁ ,y₁) are the coordinate of first point and (x₂ ,y₂) are the coordinate of second point.We have given two points (-1, 3) and (-2,-4) .
Rate of change of graph is given by slope
Using slope formula , we get
m = -4 - 3 / -2 - (-1)
m = -7 / -2 + 1
m = -7 / -1
m = 7
Learn more about slope here :
https://brainly.com/question/17248198
#SPJ9
Katya decided that she could not afford the $48,000 it would cost her to attend college and get her four-year degree. Instead, she entered the full-time workforce four years earlier than her peers who went to college. During those four years, she earned a total of $90,000. However, without a college degree, Katya made an average of $10,000 less than she could have with a degree every year for the 40 additional years of her career. What was the long-term financial cost of Katya deciding to not attend college?
Answers
Answer: $310,000
Step-by-step explanation:
In 40 additional years, the amount she made less than her college counterparts was;
= 10,000 * 40
= $400,000
She however made $90,000 more than them as they went to college;
= 400,000 - 90,000
= $310,000
The long-term financial cost was $310,000.
Find the area of the region that lies above the x-axis, below the curve x=t^2+4t+8,y=e^−t with 0≤t≤1. Give your answer exactly or round to four decimal places.
Answers
The area bounded region that above the x-axis, below the curve x(t) = t² + 4t + 8, \( y = e^{−t}\) with interval 0≤t≤1, is equals to the -6.27453.
The area between two curves is defined as the area that bounded in between two curves and can be calculated using integral calculus. We have two curves with the following equation, x(t) = t² + 4t + 8, \( y = e^{−t}\) with interval, 0≤ t ≤1. We will determine the area of the region that lies inbetween x-axis and curves. The formula for area under the curves is written as below, \(A = \int_{0}^{1} x(t)y'(t) dt \)
Substitute the known values in above formula, \(= \int_{0}^{1} ( t² + 4t + 8) ( - e^{-t}) dt \).
Now, integration by letting \(e^{-t}\)
as first function and (t² + 4t + 8) as second function, \(= [( t² + 4t + 8) e^{-t}]_{0}^{1} - \int_{0}^{1} (2t + 4) e^{-t} \\ \)
\(= [( 1 + 4×1 + 8) e^{-1} - ( 8e^{0})] + [ (2t + 4) e^{-t}]_{0}^{1} - \int_{0}^{1} 2 e^{-t}] \\ \)
\(= [13e^{-1} - 8] + [ (2×1 + 4) e^{-1} - 4]- \int_{0}^{1} 2 e^{-t}] \\ \)
\(= 13e^{-1} - 8 + 6e^{-1} - 4 + 2 e^{-1} - 2 \\ \)
\(= 21e^{-1} - 14 \)
= - 6.27453
Hence, required value is - 6.27453.
For more information about bounded area, visit :
https://brainly.com/question/31390793
#SPJ4
find the number of ways to select 3 pages in ascending index order
Answers
The number of ways to select 3 pages in ascending index order depends on the total number of pages available.
To find the number of ways to select 3 pages in ascending index order, we can use the concept of combinations . In combinatorics, selecting objects in a specific order is often referred to as permutations. However, since the order does not matter in this case, we need to consider combinations instead.
The number of ways to select 3 pages in ascending index order can be calculated using the combination formula. Since we are selecting from a set of pages, without replacement and order doesn't matter, we can use the formula C(n, k) = n! / (k! (n-k)!), where n is the total number of pages and k is the number of pages we want to select.
Learn more about combination formula here:
https://brainly.com/question/13090387
#SPJ11
#6. Please I really need help
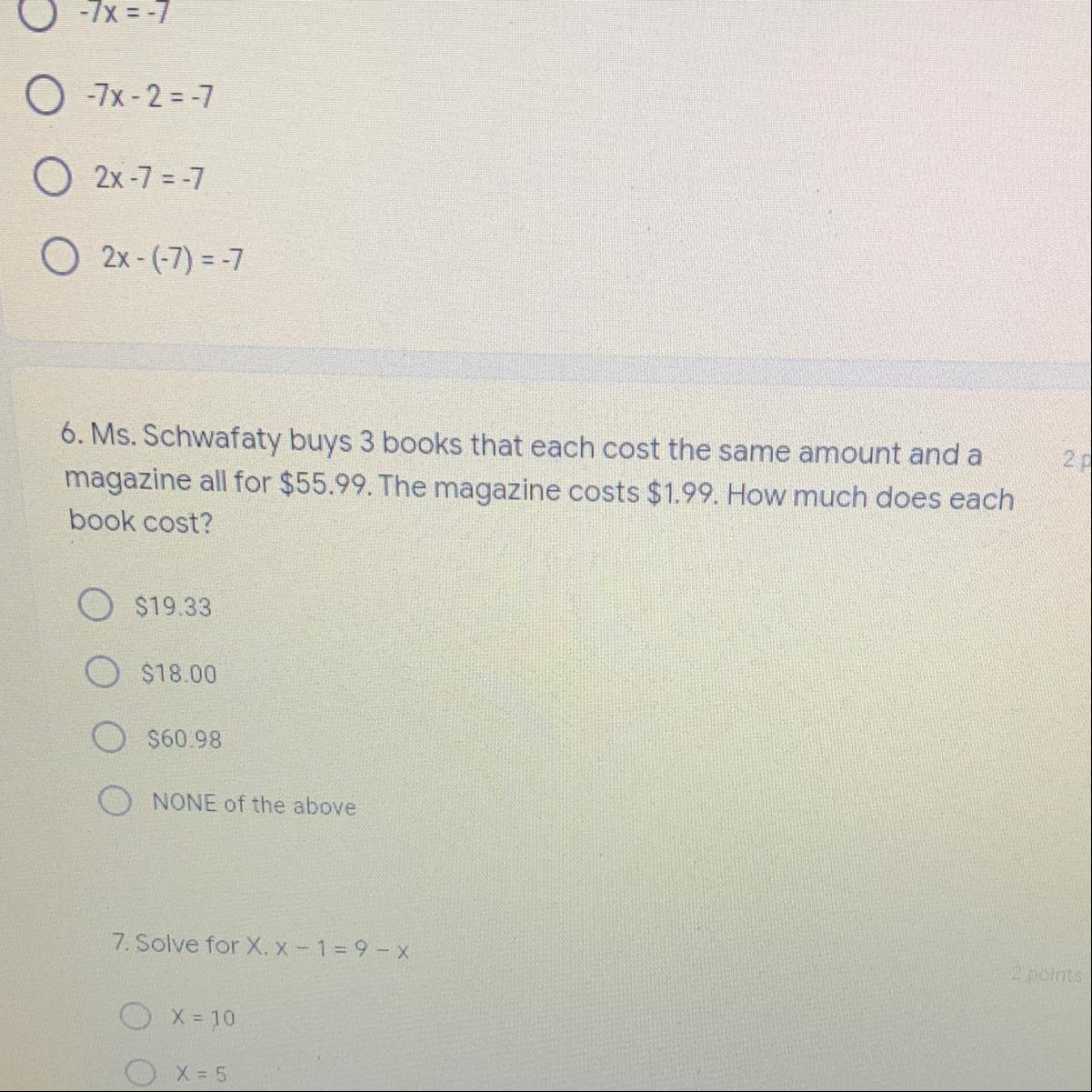
Answers
Answer:
B. $18.00
Step-by-step explanation:
3 books the same cost.
$18.00*3= $54
$ 54+$1.99= 55.99
Hope this helps
How do I determine whether a graph is a function?
Answers
Here's a more detailed explanation: To determine if a graph is a function, you can use the vertical line test. The vertical line test states that if you can draw a vertical line that intersects the graph in more than one place, then the graph is not a function.
Determining whether a graph represents a function is an important concept in mathematics, especially in algebra and calculus. A function is a set of ordered pairs where each input is associated with exactly one output.
So, if you take a ruler or a straight edge and place it vertically on the graph, and it intersects the graph in two or more points, then the graph is not a function. This is because if two points in the graph have the same x-value, they must have different y-values, since functions can only have one output for each input.
On the other hand, if you place a vertical line anywhere on the graph and it intersects the graph in only one point, then the graph is a function.
It's also important to remember that a function must also pass the horizontal line test, which means that no horizontal line can intersect the graph in more than one place.
to know more about graph refer here
https://brainly.com/question/17267403#
#SPJ11
arturo can have pizza for dinner on any two of the next six days. how many different ways can he select the days on which to have pizza?
Answers
the 15 different ways can he select the days on which to have pizza
What is combination?
A combination is a choice made in mathematics from a group of different elements when the order of the choices is irrelevant (unlike permutations). For instance, if three fruits, such as an apple, an orange, and a pear, are supplied, there are three possible pairings of the two: an apple and a pear. Formally speaking, a k-combination of a set S is a subset of S's k unique components. In other words, two combinations are the same if and only if they have the same members. (It is not important how the individuals in each set are arranged.) The quantity of k-combinations for a set with n components
Arturo can have pizza for dinner on any two of the next six days.
he select the days on which to have pizza
So the number of ways c(6 2)
= \(\frac{6!}{4!2!}\)
=\(\frac{30}{2}\)
=15 ways
Hence the 15 different ways can he select the days on which to have pizza.
Learn more about combination, by the following link.
https://brainly.com/question/11732255
#SPJ4
Please help! It’s late! (One question)
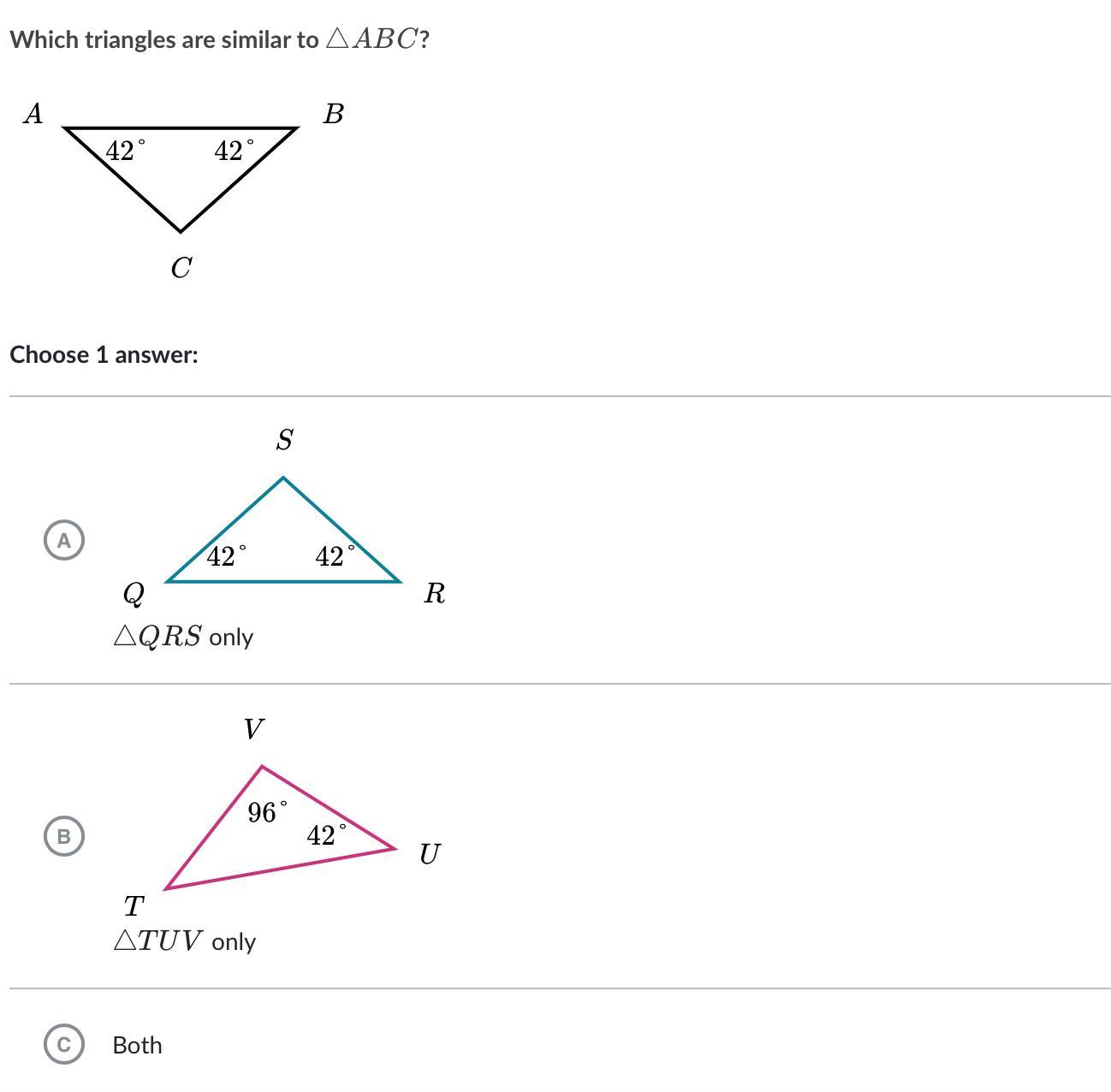

Answers
Explanation: The triangles all have corresponding congruent angles. We can tell this by finding the third angle of the original problem: 96.
Then you can read each triangle by what it states. Angle A is congruent to the corresponding angles in the other triangles, which are Q and T. Angle B is congruent to the corresponding angles in the other triangles, which are R and U. Angle C is congruent to the corresponding angles in the other triangles, which are S and V.
We know heard similar since they have congruent angle measures in corresponding spots.
Isabella deposited $500 into a savings account at a local bank that earned 5% interest per year. How much interest does she earn in her first year?
Answers
Answer:
$25
Step-by-step explanation:
First, converting R percent to r a decimal
r = R/100 = 5%/100 = 0.05 per year,
putting time into years for simplicity,
12 months ÷ 12 months/year = 1 years,
then, solving our equation
I = 500 × 0.05 × 1 = 25
I = $ 25.00
The simple interest accumulated
on a principal of $ 500.00
at a rate of 5% per year
for 1 years (12 months) is $ 25.00.
3. If f(x) = 12x + 14, what is the range value for the domain value 3?
Answers
Answer:50
Step-by-step explanation:
You are given the domain value which is your "x".
So to solve the problem you fill x as 3
Making the equation f(x)=12(3)+14
12 * 3 = 36
36+14=50
There is your answer!
Please help!!! Answer ASAP and show work!!!

Answers
Answer:
B and C. can you select more than one?
Jessi sold half her comic books. Then, she bought 9 more. She now has 11 total. How many did she begin with?
Answers
Answer: she begin with 6.
Step-by-step explanation:
At the beggining she had A books.
She sold half of that, so now she has A/2 books.
She bought 9, so now she has A/2 + 9 books,
And we know that A/2 + 9 = 11 books
So A/2 = 11 - 9 = 3
A = 2*3 = 6
So she started with 6 books
Answer:
She began with 4 books
Step-by-step explanation:
Let the original number of Jessi Comic books be x
When She sold half of the comic books, she has ( x - x/2) = x/2 left
When she bought 9 more, she has 11 in total. That is, x/2 + 9 = 11
x/2 = 11 - 9
x/2 = 2
x = 2 * 2
x = 4
She began with 4 books
The projected worth (in millions of dollars) of a large company is modeled by the equation w = 241(1.06) t. the variable t represents the number of years since 2000. what is the projected annual percent of growth, and what should the company be worth in 2011?
Answers
Answer:
6%- 457 Million
Step-by-step explanation:
w=241(1.06)^11=457 million
what is 6 20/97 as a improper fraction?
Answers
Answer:
120/97
Step-by-step explanation:
When you're turning a mixed fraction into an improper fraction, you keep the denominator (bottem number) the same, but you times the numerator (top number) by the whole number (non-fraction number/left number)
6 * 20 = 120
Therefore it should be 120/97
This can not be simplified though (if I recall)
can you please explain slope, the y-intercept, how to find the equation and how to graph it properly?
Answers
Answer:
Slope is a line on a graph, you find slope with the m=yx+b equation. Y intercept is the point that the line crosses the y axis at, it is represented by b. X and Y in the equation are your coordinates. You graph it by starting at your y-intercept and going up in a straight line following the slope calculated.
Step-by-step explanation:
What’s The Value Of X as A Decimal

Answers
Step-by-step explanation:
8x + 32 = 12x +19
4x = 13
x = 3.25
1/2 minus (1/8+1/8) I need help can somebody give me advice on this
Answers
Answer: 38
Step-by-step explanation:
Subtract 1/8 from 1/2
12 - 18 is 38.
Steps for subtracting fractions
Find the least common denominator or LCM of the two denominators:
LCM of 2 and 8 is 8
Next, find the equivalent fraction of both fractional numbers with denominator 8
For the 1st fraction, since 2 × 4 = 8,
12 = 1 × 42 × 4 = 48
Likewise, for the 2nd fraction, since 8 × 1 = 8,
18 = 1 × 18 × 1 = 18
Subtract the two like fractions:
48 - 18 = 4 - 18 = 38
Cancel CF which is 2
Now you will be with
1/2 - 1/4
Calculate
Solution
1/4
Consider the following LP problem. Maximize z=−2x1−x2+x3 subject to x1+x2+x3≤3x2+x3≥2x1+x3=1x1,x2,x3≥0 (i) Find the dual of this LP problem. [5] (ii) After adding a slack variable s1, subtracting an excess variable e2, and adding artificial variables a2 and a3, Row 0 of the LP problem's optimal tableau is found to be z=4x1+e2+(M−1)a2+(M+2)a3=0 Find the optimal solution to the dual of this LP problem. [3]
Answers
(i) The dual of the given LP problem can be found by following these steps:
1. For each constraint in the primal problem, create a dual variable. In this case, we have three constraints, so we'll have three dual variables: y1, y2, and y3.
2. The objective function of the dual problem will be the sum of the products of the primal variables and their corresponding dual variables. So, the dual objective function is:
Maximize w = 3y1 + 2y2 + y3.
3. For each primal variable x, create a constraint in the dual problem with the coefficient of the corresponding dual variable equal to the coefficient of x in the primal objective function. So, the dual constraints are:
y1 + 2y2 - y3 ≤ -2
y1 + y2 + y3 ≤ -1
y1, y2, y3 ≥ 0.
(ii) To find the optimal solution to the dual problem, we need to solve the optimal tableau of the dual problem. From the given information, we know that Row 0 of the optimal tableau is:
w = 4x1 + e2 + (M-1)a2 + (M+2)a3 = 0.
However, the given information does not provide any details about the values of x1, e2, a2, or a3. Therefore, without this information, we cannot determine the specific optimal solution to the dual problem.
To know more about variable , visit ;
https://brainly.in/question/40782849
#SPJ11
Consider the parent function f (x) below.
1. Graph f(2x) in the color green below.
2. Graph -3f(x) in the color blue below.
3. Graph 2f (2x) in the color red below.
Help asap :)

Answers
In this subsection, we examine the ways in which the graphs of functions alter or transform as a result of certain, specialized adjustments to their formulations.
What is Transformations?We shall present the transformations in the following order: shifts, reflections, and scalings. The transformations we will investigate can be broadly divided into these three types. Consider the full graph of a function f to be the graph shown below.In order for the point (a, b) to be on the graph, f(a) = b, according to the Fundamental Graphing Principle for Functions. To be more specific, we know that f(0) = 1, f(2) = 3, f(4) = 3, and f(5) = 5.To refresh our memories on what g is doing, let's pause for a moment. With an input of x into the function f, we can then get the f as the result (x).G adds 2 to the output of the function f(x). Graphing the points (x, g(x)) is required in order to graph g. The correct response is that we only require the values of f and do not require a formula for f(x) (x). On the graph of y = f, the y values correspond to the values of f(x) (x). We can create the following table, for instance, using the points shown on the f graph.In general, if the pair (a, b) is on the y = f(x) graph, then f(a) = b, and g(a) = f(a) + 2 = b + 2. The result is that (a, b+ 2) is on the graph of g. Alternatively said, we multiply the y-coordinate of each point on the graph of f by 2 to produce the graph of g. Geometrically, a point travels 2 units higher when its y-coordinate is multiplied by 2. Typically, "moving the graph up 2 units" refers to collectively adding 2 to each y-coordinate on a graph. The graph is only two units above its original place, but it still has the same basic structure as previously.To Learn more About transform refer To:
https://brainly.com/question/28025572
#SPJ1

Three boxes each contain a different number of marbles. Box A has 70 marbles, box B has 88 marbles, and box C has 80 marbles. Marbles are to be transferred from box B to box A. What is the least number of marbles that can be transferred so box C has the most marbles?
Answers
it A
Step-by-step explanation: