2+7-8
Using BIDMAS, thank you
Answers
Answer:
\(2 + 7 - 8(additin \: is \: first) \\ = 9 - 8 \\ = 1 \\ thank \: you\)
Related Questions
Shubham, Ashish, and Vinay participated in a long jump competition on their sports day. Shubham jumped 41 m 2 , while Ashish jumped 3 m less than Shubham. On the 4 other hand, Vinay jumped 11m more than Ashish. How 4 long was Vinay's jump?
Answers
Answer:
Vinay jumped = 49m
Step-by-step explanation:
Given:
Shubham jumped = 41m
Ashish jumped = 3m less than Shubham
Vinay jumped = 11m more than Ashish
Find:
Length of Viney's jump
Computation:
Ashish jumped = Shubham jumped - 3m
Ashish jumped = 41m - 3m
Ashish jumped = 38m
Vinay jumped = Ashish jumped + 11m
Vinay jumped = 38m + 11m
Vinay jumped = 49m
For each problem, define a variable and set up an equation, then solve.
Kate's math homework had a set of equations and one work problem. She took 3 minutes to solve each equation then 7 minutes to solve the word problem. if it took her 52 minutes total how many equations did she solve?
Your response will be a number only.
Answers
When Kate's math homework had a set of equations and one work problem, the number of equations will be 15.
How to illustrate the information?It should be noted that an equation is used to illustrate the relationship between the variables.
It should be noted that in this case, Kate's math homework had a set of equations and one work problem and she took 3 minutes to solve each equation.
Let the number of equation be represented by x.
Therefore, the information illustrated shows that the equation will be:
3x + 7 = 52
Collect like terms
3x = 52 - 7
3x = 45.
Divide
x = 45 / 3
x = 15
Therefore, Kate's math homework had a set of equations and one work problem, the number of equations will be 15.
Learn more about equations on:
brainly.com/question/13763238
#SPJ1
prove the identity. sinh(2x) = 2 sinh(x) cosh(x)
Answers
To prove the identity sinh(2x) = 2 sinh(x) cosh(x), we can use the definitions of sinh(x) and cosh(x) and apply trigonometric identities for exponential functions.
We start with the left-hand side of the identity, sinh(2x). Using the definition of the hyperbolic sine function, sinh(x) = (e^x - e^(-x))/2, we can substitute 2x for x in this expression, giving us sinh(2x) = (e^(2x) - e^(-2x))/2.
Next, we focus on the right-hand side of the identity, 2 sinh(x) cosh(x). Again using the definitions of sinh(x) and cosh(x), we have 2 sinh(x) cosh(x) = 2((e^x - e^(-x))/2)((e^x + e^(-x))/2).
Expanding this expression, we get 2 sinh(x) cosh(x) = (e^x - e^(-x))(e^x + e^(-x))/2.
By simplifying the right-hand side, we have (e^x * e^x - e^x * e^(-x) - e^(-x) * e^x + e^(-x) * e^(-x))/2.
This simplifies further to (e^(2x) - 1 + e^(-2x))/2, which is equal to the expression we derived for the left-hand side.
Hence, we have proved the identity sinh(2x) = 2 sinh(x) cosh(x) by showing that the left-hand side is equal to the right-hand side through the manipulation of the exponential functions.
Learn more about Trignometric Identities here: brainly.com/question/24377281
SPJ11
unit 7 right triangles and trigonometry homework 3 similar right triangles and Geometric Mean, pls help.


Answers
The results are listed below:
11) The value of \(x\) is approximately 32.404 units.
12) The value of \(x\) is approximately 10.392 units.
13) The value of \(x\) is approximately 15.875 units.
14) The value of \(x\) is approximately 22.450 units.
15) The value of \(x\) is 11.571 units.
16) The value of \(x\) is 17 units.
17) The value of \(x\) is approximately 21.333 units.
18) The value of \(x\) is 24.857 units.
19) The solution of this system is: \(x \approx 16.155\), \(y \approx 6.462\), \(z\approx 2.4\).
20) The solution of this system is: \(x = 37.5\), \(y = 18\), \(z = 22.5\).
How to calculate missing sides in right triangles
In this question we should apply Pythagorean theorem and algebraic methods to find the missing lengths. Now we proceed to the procedure for each case:
Figure 11We construct the system of equations and find its solution:
\(-x^{2}+y^{2} = 40^{2}\) (1)
\(y^{2}+z^{2} = 62^{2}\) (2)
\(-x^{2}+z^{2} = 12^{2}\) (3)
The solution of this system is: \(x \approx 32.404\), \(y\approx 51.478\), \(z\approx 34.554\). \(\blacksquare\)
Figure 12We construct the system of equations and find its solution:
\(-x^{2} + y^{2} = 3^{2}\) (1)
\(-x^{2}+z^{2} = 36^{2}\) (2)
\(y^{2}+z^{2} = 39^{2}\) (3)
The solution of this system is: \(x \approx 10.392\), \(y \approx 10.817\), \(z \approx 37.470\). \(\blacksquare\)
Figure 13We construct the system of equations and find its solution:
\(x^{2}-y^{2} = 14^{2}\) (1)
\(-y^{2}+z^{2} = 4^{2}\) (2)
\(x^{2}+z^{2} = 18^{2}\) (3)
The solution of this system is: \(x \approx 15.875\), \(y\approx 7.483\), \(z \approx 8.485\). \(\blacksquare\)
Figure 14We construct the system of equations and find its solution:
\(x^{2}-y^{2} = 8^{2}\) (1)
\(-y^{2}+z^{2} = 8^{2}\) (2)
\(x^{2}+z^{2} = 63^{2}\) (3)
The solution of this system is: \(x \approx 22.450\), \(y \approx 20.976\), \(z \approx 58.865\). \(\blacksquare\)
Figure 15We construct the system of equations and find its solution:
\(-x^{2}+y^{2} = 18^{2}\) (1)
\(z^{2} = 28^{2}+18^{2}\) (2)
\(z^{2}+y^{2} = (x+28)^{2}\) (3)
The solution of this system is: \(x = 11.571\), \(y \approx 21.398\), \(z\approx 33.287\). \(\blacksquare\)
Figure 16We construct the system of equations and find its solution:
\(y^{2} = 20^{2}-8^{2}\) (1)
\(x^{2}+y^{2}-z^{2} = 0\) (2)
\((x+8)^{2}-z^{2}= 20^{2}\) (3)
The solution of this system is: \(x = 17\), \(y \approx 18.330\), \(z = 25\). \(\blacksquare\)
Figure 17
We construct the system of equations and find its solution:
\(y^{2} = 16^{2}-12^{2}\) (1)
\((x-12)^{2}+y^{2}-z^{2} = 0\) (2)
\(x^{2}-z^{2} = 16^{2}\) (3)
The solution of this system is: \(x \approx 21.333\), \(y\approx 10.583\), \(z \approx 14.110\). \(\blacksquare\)
Figure 18We construct the system of equations and find its solution:
\(-(x-21)^{2}+y^{2} = 9^{2}\) (1)
\(z^{2} = 21^{2}+9^{2}\) (2)
\(-x^{2}+y^{2}+z^{2} = 0\) (3)
The solution of this system is: \(x = 24.857, y \approx 9.791, z \approx 22.847\). \(\blacksquare\)
Figure 19We construct the system of equations and find its solution:
\(y^{2}-z^{2} = 6^{2}\) (1)
\(x^{2} = 6^{2}+15^{2}\) (2)
\(x^{2}+y^{2}-(15+z)^{2} = 0\) (3)
The solution of this system is: \(x \approx 16.155\), \(y \approx 6.462\), \(z\approx 2.4\). \(\blacksquare\)
Figure 20We construct the system of equations and find its solution:
\(y^{2} = 30^{2}-24^{2}\) (1)
\((x-24)^{2}+y^{2}-z^{2} = 0\) (2)
\(x^{2}-z^{2} = 30^{2}\) (3)
The solution of this system is: \(x = 37.5\), \(y = 18\), \(z = 22.5\). \(\blacksquare\)
To learn more on right triangles, we kindly invite to check this verified question: https://brainly.com/question/7894175
can someone please help me graph this ? not rlly sure

Answers
The graph of the given equation is attached below.
Plotting a line graphThe equation of a line in slope-intercept form is expressed as y = mx + b.
Given an xy plane, we are to plot the graph of the given equation
y = -2/3 x + 1
The given equation has a slope of -2/3 and a y-intercept of 1. This shows that the constructed line must cut the y-axis at the point (0, 1).
The graph of the equation is attached below.
Learn more on line graph here: https://brainly.com/question/26233943
#SPJ1

−8y−13=14y+11 what is the answer?
Answers
Answer:
y = -12/11
Step-by-step explanation:
-8y - 13 = 14y + 11
-8y - 14y = 11 + 13
-22y = 24
y = 24/-22
y = -12/11
Check:
-8*-12/11 - 13 = 14*-12/11 + 11
96/11 - 13 = -168/11 + 11
96/11 - 143/11 = -168/11 + 121/11 = -47/11
Find three consecutive even integers such that the sum of the first and the third is 128.
Answers
9514 1404 393
Answer:
62, 64, 66
Step-by-step explanation:
I like to work "consecutive integer" problems by considering the average, or middle value. If we call that x, then the problem statement tells us ...
(x -2) +(x +2) = 128
2x = 128 . . . . . collect terms
x = 64 . . . . . . . divide by 2
The middle integer is 64, so the three even integers are 62, 64, 66.
Someone please help!
Data is collected on the masses, m kg, of 140 Humboldt penguins. (data in table) a) given that an estimate for the mean mass of the penguins is 4.09kg, find the value of k

Answers
Answer:
k = 6.26Step-by-step explanation:
Find the midpoint of each frequency range, multiply that number by the frequency, sum up and divide by the total frequency.
This will give the mean number.
The mean is:
[17*( 3 + 3.5)/2 + 21*(3.5 + k)/2 + 33*(k + 4)/2 + 54*(4 + 4.5)/2 + 15*(4.5 + 6)/2]/140 = 4.0955.25 + 10.5k + 36.75 + 16.5k + 66 + 229.5 + 78.75 = 140*4.0917k + 466.25 = 572.617k = 572.6 - 466.2517k = 106.35k = 106.35/17k = 6.26Note, I expected the value of k between 3.5 and 4. This is a bit of illogical outcome.
Answer:
k = 3.94 (2 d.p.)
Step-by-step explanation:
The given table is a grouped frequency table with continuous data (no gaps or overlaps between classes).
Mean of grouped data
\(\displaystyle \text{Mean}=\dfrac{\sum fx}{\sum f}\)
(where f is the frequency and x is the class mid-point).
To find an estimate of the mean, assume that every reading in a class takes the value of the class mid-point.
\(\textsf{class mid-point }(x)= \dfrac{\textsf{lower class boundary} + \textsf{upper class boundary}}{2}\)
Calculate the mid-points (x) of each class and fx:
\(\begin{array}{| l | c | c | c |}\cline{1-4} \text{Mass, }m\:\text(kg) & \text{Frequency, }f & \text{Class mid-point, }x & fx \\\cline{1-4} 3 \leq m < 3.5 & 17 & 3.25 & 55.25\\\cline{1-4} 3.5 \leq m < k & 21 & \dfrac{3.5+k}{2} & 36.75+10.5k \\\cline{1-4} k \leq m < 4.0 & 33 & \dfrac{k+4.0}{2} & 16.5k+66\\\cline{1-4} 4.0 \leq m < 4.5 & 54 & 4.25 & 229.5 \\\cline{1-4} 4.5 \leq m < 6 & 15 & 5.25 & 78.75 \\\cline{1-4} \text{Totals} & 140 & & 466.25+27k\\\cline{1-4}\end{array}\)
Given the mean is 4.09 kg, substitute the found values of f and fx (from the above table) into the mean formula and solve for k:
\(\implies 4.09=\dfrac{466.25+27k}{140}\)
\(\implies 572.6=466.25+27k\)
\(\implies 27k=106.35\)
\(\implies k=3.94\:\:(2\: \sf d.p.)\)
Therefore, the value of k is 3.94 (2 d.p.)
The z-value corresponding to a significance level of 0.1388 in a two-tailed test is?
Answers
Step-by-step explanation:
To find the z-value corresponding to a significance level of 0.1388 in a two-tailed test, we need to find the critical values for the test.
Assuming a normal distribution, we can use a standard normal distribution table or calculator to find the critical values.
The significance level for a two-tailed test is split equally between both tails. Therefore, we need to find the z-value that corresponds to a tail area of (1 - 0.1388)/2 = 0.4306.
Using a standard normal distribution table or calculator, we can find that the z-value that corresponds to a tail area of 0.4306 is approximately 1.761.
Therefore, the z-value corresponding to a significance level of 0.1388 in a two-tailed test is +/- 1.761.
Which expression is negative?
a
b
+
+
-1
0
1
Choose 1 answer:
А
a +1
B
a -(-6)
None of the above

Answers
Answer:
I don’t understand
Step-by-step explanation:
i don’t understand
\( \)
\( \rm \: Find \: \frac{dy}{dx} if \: y = sin \: x \degree\)
\(\rm \: Hint :y= sin x° = sin( \frac{\pi \: x}{180} )\)
( Convert degree to ratio )
\( \: \: \: \)
Thanku:)
Answers
Answer:
Given that:
\(\longmapsto{ \large{ \rm{y = \sin \:x \degree}}}\)
We know that,
\( \dashrightarrow{ \boxed{ \red{ \rm{1 \degree = \left ( \frac{\pi}{100} \right)^{c} }}}}\)
So, using this, above given can be written as,
\({ \large{ \longrightarrow{ \rm{y = \sin \left( \frac{\pi x}{180} \right) }}}}\)
On differentiating both sides w.r.t. x, we get:
\({ \large { \longrightarrow{ \rm{ \frac{d}{dx}y = \frac{d}{dx} \sin \left( \frac{\pi x}{180} \right) }}}}\)
We know that,
\({ \dashrightarrow{ \boxed{ \red{ \rm{ \frac{d}{dx} \sin x = \cos x }}}}}\)
So, using the result, we get:
\({ \large{ \longrightarrow{ \rm{ \frac{d}{dx} = \cos \left( \frac{\pi \: x}{180} \: \right) \frac{d}{dx} \left( \frac{\pi \: x}{180} \right) }}}}\)
We know that,
\({ \dashrightarrow{ \boxed{ \red{ \rm{ \frac{d}{dx}k \: f(x) = k \frac{d}{dx} \: f(x)}}}}}\)
So, using this, we get:
\({ \large{ \longrightarrow{ \rm{ \frac{dy}{dx} = \cos \left( \frac{\pi \: x}{180} \right) \: \times \: \left( \frac{\pi}{180} \right) \frac{d}{dx} x}}}}\)
We know that,
\({ \dashrightarrow{ \boxed{ \red{ \rm{ \frac{d}{dx} x= 1 }}}}}\)
So, using this, we get:
\({ \large{ \longrightarrow{ \rm{ \frac{dy}{dx} = \left( \frac{\pi}{180} \right) \: \cos \left( \frac{\pi \: x}{180} \right ) \times 1}}}}\)
Hence:
\({ \large{ \leadsto{ \green{ \rm{ \frac{dy}{dx} = \left( \frac{\pi}{180} \right) \cos \left( \frac{\pi \: x}{180} \right) }}}}}\)
OR\({ \large{ \leadsto{ \green{ \rm{ \frac{dy}{dx} = \left( \frac{\pi}{180} \right) \cos \: x \degree }}}}}\)
\( \: \: \)
Learn More:
\( \boxed{\begin{array}{c|c}\bf f(x)&\bf\dfrac{d}{dx}f(x)\\ \\ \frac{\qquad\qquad}{}&\frac{\qquad\qquad}{}\\ \sf k&\sf0\\ \\ \sf sin(x)&\sf cos(x)\\ \\ \sf cos(x)&\sf-sin(x)\\ \\ \sf tan(x)&\sf{sec}^{2}(x)\\ \\ \sf cot(x)&\sf-{cosec}^{2}(x)\\ \\ \sf sec(x)&\sf sec(x)tan(x)\\ \\ \sf cosec(x)&\sf-cosec(x)cot(x)\\ \\ \sf\sqrt{x}&\sf\dfrac{1}{2\sqrt{x}}\\ \\ \sf log(x)&\sf\dfrac{1}{x}\\ \\ \sf{e}^{x}&\sf{e}^{x}\end{array}}\)
what is the best answer for 441.6 ÷ 12 ?
Answers
Answer:
36.8
Step-by-step explanation:
Your answer would be 36.8 because 12 can go in 441.6 36.8 times also to check the answer you could use multiplication which would be 36.8x12 441.6.Hope it helps you!
\(\rm\red{\overbrace{\underbrace{\tt\color{orange}{\:\:\:\:\:\:\:\:Answer:\:\:\:\:\:\:\:\:\:}}}}\)
\(36.8\)
\(\rm\red{\overbrace{\underbrace{\tt\color{gold}{\:\:\:\:\:\:\:\:ChaEunWoo2009\:\:\:\:\:\:\:\:\:}}}}\)
#CarryOnLearning
Can you help on this

Answers
Answer:
Third option
Step-by-step explanation:
12/11, 12:11, 12 to 11
Answer:
c
Step-by-step explanation:
12/11, 12:11, 12 to 11
hope this helps.
we randomly select 4 numbers from the set of the first 16 prime numbers, without replacement. what is the probability their sum is even? why?
Answers
The probability their sum is even without replacement is 39/52
What is Probability?Probability: Probability is the branch of mathematics concerning numerical descriptions of how likely an event is to occur, or how likely it is that a proposition is true. The probability of an event is a number between 0 and 1, where, roughly speaking, 0 indicates impossibility of the event and 1 indicates certainty.
It is given that if we randomly select 4 numbers from the set of the first 16 prime numbers, without replacement, then the probability that their sum i seven is,
2,3,5,7,11,13,17,23,27,29,31,37,41,43,49,51 are the first 16 prime numbers.
Even numbers are always produced when two odd numbers are joined together.
Odd numbers are always created when Even numbers are added to Odd numbers.
In the list of 16, there is just one even number. If that is one of the options, then the outcome will only be odd. As the same number cannot be selected twice, there is a 15/16 chance that it won't be chosen the first time. There is also a 14/15 chance that it won't be chosen the second time, a 13/14 chance the third time, and a 12/11 chance the fourth time.
By multiplying these four probabilities, we may get the probability of an even sum. The odds of a total that is even are therefore,
32760/43680=39/52=0.75
The probability their sum is even without replacement is 39/52
To learn more about Probability visit:
brainly.com/question/11234923
#SPJ4
The midpoint of \overline{\text{AB}}
AB
is M(1, -3)M(1,−3). If the coordinates of AA are (-6, -7)(−6,−7), what are the coordinates of BB?
Answers
Answer:
the coordinates of BB would be: B(8,1)
The price of pears can be determined by the equation P=0.54n, where P is the price and n is the number of pears. What is the constant of proportionality (unit rate)?
PLEASE NEED THIS ASAP!!!!!!!!!!
Answers
Answer:
The constant of proportionality is 0.54
Step-by-step explanation:
Given:
P = 0.54n
Where,
P = price of pears
n = number of pears
What is the constant of proportionality (unit rate)?
The constant of proportionality is 0.54
This means a unit of pears cost $0.54
If the number of pears bought, n = 50
Then,
P = 0.54n
When n = 50
P = 0.54(50)
= 27
P = 27 when n = 50
The correct answer is:
The constant of proportionality is 0.54
Given f(x) = 5x squared of 2 + 2 and g(x) = 8 - 3x, find the following.
12. Find g[f(-2)].
11. Find flg(x)).
Answers
![Given f(x) = 5x squared of 2 + 2 and g(x) = 8 - 3x, find the following.12. Find g[f(-2)].11. Find flg(x)).](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/GX0jyp5zifQr4nOTP1RthkRlJxcgzONt.png)
Joelle wants to center a painting on a wall that is 16.9 feet long. how much space will be between the end of the wall and the painting?
Answers
The equation to find the amount of space between the end of the wall and the painting is x = 16.9 - 2 * space, where 'x' represents the length of the painting and 'space' represents the unknown space.
To find the amount of space between the end of the wall and the painting, we need to subtract the length of the painting from the length of the wall and divide it by 2.
Given that the wall is 16.9 feet long and the painting is to be centered, we can assume the painting's length is unknown. Let's represent it as 'x'.
Therefore, the equation becomes (16.9 - x) / 2 = space between the end of the wall and the painting.
To solve for x, we can multiply both sides of the equation by 2 to get rid of the fraction, resulting in 16.9 - x = 2 * (space between the end of the wall and the painting).
Next, we simplify the equation to 16.9 - x = 2 * space.
To isolate x, we subtract 2 * space from both sides, resulting in x = 16.9 - 2 * space.
To determine the exact amount of space between the end of the wall and the painting, we would need to know the value of 'space'. Unfortunately, it is not provided in the question.
In summary, the equation to find the amount of space between the end of the wall and the painting is x = 16.9 - 2 * space, where 'x' represents the length of the painting and 'space' represents the unknown space.
Know more about multiply here,
https://brainly.com/question/30875464
#SPJ11
(b) Find the greatest number that divides 300, 560 and 500 without leaving a remainder.
Answers
Greatest number that divides 300, 560 and 500 is 20 .
Given numbers : 300, 560 and 500
First let’s find prime factors of 300,560 and 500
300 = 2^2 *3^1 *5^2
560= 2^4 * 7^1 *5^1
500 = 2^2 * 5^3
So,
Here highest common power of 2 is 2
Here highest common power of 3 is 0
Here highest common power of 5 is 1
Here highest common power of 7 is 0
Thus HCF (300, 560 and 500) = 2^2 * 5^1 * 3 ^0 * 7 ^0
=4*5*1*1
= 20
Know more about HCF,
https://brainly.com/question/26431349
#SPJ1
(0)
A production line operates for two eight-hour shifts each day. During this time, the production line is expected to produce 3,000 boxes. What is the takt time in minutes?
Group of answer choices
.25
.3
3
.6
Answers
The expected number of boxes to be produced is given as 3,000 boxes. So, the correct answer is 0.3, indicating that the takt time in minutes is 0.3 minutes.
The production line operates for two eight-hour shifts each day, which means there are 16 hours of production time available. Since there are 60 minutes in an hour, the total available time in minutes would be 16 hours multiplied by 60 minutes, which equals 960 minutes.
The expected number of boxes to be produced is given as 3,000 boxes.
To calculate the takt time in minutes, we divide the total available time (960 minutes) by the expected number of boxes (3,000 boxes):
\(Takt time = Total available time / Expected number of boxes\)
\(Takt time = 960 / 3,000\)
By performing the calculation, we find that the takt time is approximately 0.32 minutes, which is equivalent to 0.3 minutes rounded to one decimal place.
Learn more about minutes here:
https://brainly.com/question/31148642
#SPJ11
i really need help..im confused

Answers
The marginal frequencies of chocolate and strawberry are 0.75 and 0.25 and chocolate is greater by 0.5
What are marginal frequencies?Marginal frequency is the ratio between either a column total or a row total and the total sample size.
For example, in a table of students classified by sex and area of study, the number of female students, regardless of area of study, would be one marginal frequency.
The marginal frequency of chocolate is calculated as;
The total frequency of chocolate = 30+45 = 75
The grand frequency = 75+25 = 100
marginal frequency of chocolate = 75/100
= 0.75
The marginal frequency of strawberry = 25/100
= 0.25
The difference in the marginal frequencies of chocolate and strawberry = 0.75 - 0.25 = 0.50
Therefore the marginal frequency of chocolate is greater than strawberry by 0.5
learn more about marginal frequency from
https://brainly.com/question/27506923
#SPJ1
The base of an 11 foot ladder is 3 feet from a building if the ladder reaches the flat roof how tall is the building
Answers
The building, the ladder, and the ground form a right triangle as shown below:
The ladder is the hypotenuse of the triangle and the height H and the horizontal distance (3 ft) are the legs.
Applying the Pythagora's Theorem:
\(H^2+3^2=11^2\)Solving for H:
\(\begin{gathered} H^2=11^2-3^2 \\ H=\sqrt{11^2-3^2} \\ H=\sqrt[]{121-9} \\ H=\sqrt[]{112} \\ H\approx10.58 \end{gathered}\)The building is 10.58 feet tall

Where is the graph of f(x)=4[x-3]+2 discontinuos
Answers
Answer:
Below
Step-by-step explanation:
4 [x-3] + 2 = y is not discontinuous anywhere
However 4 / [x-3] + 2 DOES have a discontinuity at x = 3 because this would cause the denominator to be zero <===NOT allowed !!
For numbers 5 - 6, fill in the boxes for the area method, and then put a check mark next to the correct answer.
(5 points per question: 1 point for each blank and 1 point for the correct answer). 5. Fill in the blanks within the area model for (4a - 2b)(12a + 6b) and then put a check next to the answer after simplifying (combining like terms).
4a
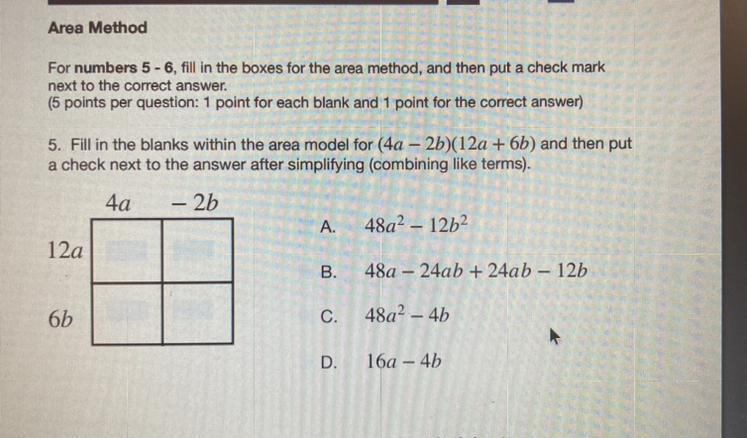
Answers
Answer: 12a + 6b
-2b
-8ab
Check: 48a^2 - 24b^2
Step-by-step explanation:
How many solutions exist for the system of equations in the graph?
Answers
Use elimination/linear combination to solve the following.
please help

Answers
Answer:
Step-by-step explanation:
3x + 4y = 17
5x - 4y = 7
you can thus cancel out 4y
Hence
8x = 24
Hence x = 3
Substituting’
3(3) + 4y = 17
9 + 4y = 17
Hence 17 - 9/4
= y = 2
Hope this helps
Which number is irrational?
A. square root of 5
B. 0.777...
C. 0.454545...
D. 0.3
Answers
Answer:
A
Step-by-step explanation:
The square root of 5 is irrational because it cannot be expressed as a fraction.
Meanwhile, all 3 other answer choices are not irrational because they can be put into fraction form.
Answer:
irrational numbers are number that can't be written in a/b form where a and b are integers and b≠0
if it repeats, it can be written as a fraction
if it terminates, it can also be written as a fraction
if you have a square root that doesn't simplify nicely, we assume it to be irrational
so
A. 0.454545=45/99
B. irrational
C. 7/9
D. 3/10
Please help meeeeee plzzzzz

Answers
tax because the tax shows you what you paid and ect
Find x using the secant-tangent theorem.

Answers
Answer:
Step-by-step explanation:
PLEASE HELP !!!!!! What is the range of f(x)=|x|+7 ??????

Answers
Answer:b
Step-by-step explanation:becayse 7-2 is 11 now you mltiply that a boom