2) Six of the 32 teams are from
Asia. What (simplified)
fraction of the teams are
from Asia?
Answers
Therefore it’s 3/16
Related Questions
Use the graph of the polynomial function to find the factored form of the
related polynomial. Assume it has
no constant factor.

Answers
Answer:
C. X+1 x+8
Here is ur answer
Mark me as brainlest.......
To get to work each morning, Emily takes a horse 10.98 kilometres and a bike 4.06 kilometres. How many kilometres is Emily’s journey in total?
Answers
You have to do 10.98+4.06
i would split this into 2 parts so i would get 10+4 and 0.98+0.06
10+4=14
0.98+0.06= 1.04
then we add 14 to 1.04
14+1.04=15.04
So your answer would that Emilys journey is 15.04km in total
Please help for section d) 100 points, must show all working and step by step

Answers
Answer:
Step-by-step explanation:
(a) and (b) see diagram
(c) you can see from the graph, the purple line hits the parabola twice which is y=6 or k=6
(d) Solving simultaneously can mean to set equal
6x - x² = k >subtract k from both sides
6x - x² - k = 0 >put in standard form
- x² + 6x - k = 0 >divide both sides by a -1
x² - 6x + k = 0
(e) The new equation is the same as the original equation just flipped (see image)
(f) The discriminant is the part of the quadratic equation that is under the root. (not sure if they wanted the discriminant of new equation or orginal. I chose new)
discriminant formula = b² - 4ac
equation: x² - 6x + 6 = 0 a = 1 b=-6 c = 6
discriminant = b² - 4ac
discriminant= (-6)² - 4(1)(6)
discriminant = 36-24
discriminant = 12
Because the discriminant is positive, if you put it back in to the quadratic equation, you will get 2 real solutions.

If 1/2 gallon of paint covers 1/8 of a wall, then how many quarts of paint are needed for the entire wall?
Answers
Answer:
4
Step-by-step explanation:
What are some differences between linear equations and inequalities, specifically their solutions and the process of finding those solutions?
Answers
Answer:
difference between linear equations and inequalities is the solution set. A linear equation of two variables can have more than one solution. For instance, with x = 2_y_ + 3, (5, 1), then (3, 0) and (1, -1)
Step-by-step explanation:
Need help brainlest to whoever is right

Answers
Answer:
2
Step-by-step explanation:
Answer:
x is equal to 2
Step-by-step explanation:
Just because
Combine like terms:
12x+4x-7y+45b+78a-44b+1a+1z+0d+1.1a+1.1a+2+2+2
PLEASE PLEASE HELP
Answers
Answer:
16x -7y +b +81.2a +z +6
Step-by-step explanation:
Coefficients of terms with the same variable are added when like terms are combined.
12x+4x-7y+45b+78a-44b+1a+1z+0d+1.1a+1.1a+2+2+2
=(12 +4)x +(-7)y +(45 -44)b +(78 +1 +1.1 +1.1)a +(1)z +(2+2+2)
= 16x -7y +b +81.2a +z +6
Find the vale of x and explain.

Answers
Answer:
x=37
Step-by-step explanation:
You know all the angles in a triangle add up to 180.
Therefore, you add 72° and 71° which equals 143.
Then you subtract 180 and 143 which equals 36.
[72+71=143
180-143=37]
I hope this helps you!
-6 inches per second it takes her 4 minutes to reach the bottom what is the cliff's height in feet
Answers
The height of the cliff from which Oletha rappels is 1,440 feet. We simply used the speed, distance, and time formulas.
To find the height of the cliff, we can use the formula:
distance = rate x time
In this case, the rate is -6 inches per second (negative because Oletha is descending), and the time is 4 minutes or 240 seconds.
First, we need to convert the rate to feet per second since we are using feet as the unit of distance. There are 12 inches in a foot, so:
-6 inches per second = -0.5 feet per second
Now we can use the formula to find the distance:
distance = (-0.5 feet per second) x (240 seconds)
distance = -120 feet
The negative sign indicates that Oletha has descended 120 feet below the starting point. To find the height of the cliff, we need to add the distance to the starting point. Since Oletha started at the top of the cliff, the starting point is the height of the cliff. Therefore:
height of cliff = starting point + distance
height of cliff = 0 feet + 120 feet
height of cliff = 120 feet
Therefore, the height of the cliff is 1,440 feet since there are 12 inches in a foot and 1,440 = 12 x 120.
Learn more about Height:
https://brainly.com/question/28122539
#SPJ4
Complete Question:
Oletha rappels from the top of a cliff at a rate of -6 inches per second. It take Oletha 4 minutes to reach the bottom of the cliff. What is the height of the cliff in feet?
If the discriminant is 22, then the roots of the quadratic equation are ________________.
Answers
Since the discriminant given has a value that is greater than zero, hence the roots of the quadratic equation are real and distinct.
Discriminant of a quadratic equationQuadratic equation is an equation that has a leading degree of 2. The discriminant is used to determine the nature of the equation
If D > 0 , the roots of the quadratic equation are real and distinct.
If D < 0 , the roots of the quadratic equation are complex
Since the discriminant given has a value that is greater than zero, hence the roots of the quadratic equation are real and distinct.
Learn more on discriminant here: https://brainly.com/question/2507588
#SPJ1
choch Question 3 3.1 Construct the following triangles: 3.1.1 ADEF with EF = 7cm,

Answers
We have to construct a triangle DEF with EF = 7 cm. It is given that we have to make an angle of 50⁰ at E and at F.
Steps to make a triangle DEF:
Step 1: Draw a line segment EF equal to 7 cm, using a ruler.
Step 2: Put the center of the protractor on the one end of the line segment that is at E and make the given angle that is 50⁰.
Step 3: Make the angle of 50⁰ at F by using protractor.
Step 4: Extend the line from E and F in the direction of 50⁰. After extending the line segments, we get an intersection point, name that point D..
Step 5: The point D, is made from the intersection of the line segment from E and F.
Step 6: Hence, triangle DEF is constructed.
For similar question, visit:
https://brainly.com/question/929137
#SPJ9
if a=3 then what does 5(a+5) equal show all steps
Answers
Answer:
40
Step-by-step explanation:
5(a+5)
Let a =3
5(3+5)
Parentheses first
5(8)
Multiply
40
Answer:
40
Step-by-step explanation:
5(a+5)
5(3+5)
5(8)
40
Find the open interval(s) where the following function is increasing, decreasing, or constant. Express your answer in interval notation.
Answers
To find the open intervals where the function is increasing, decreasing, or constant, we need to analyze its first derivative. Follow these steps:
1. Find the first derivative of the function, f'(x).
2. Set f'(x) equal to 0 and solve for x. These are the critical points.
3. Test the intervals created by these critical points to determine if the function is increasing or decreasing.
Without knowing the specific function, I cannot provide a direct answer. However, once you have followed these steps, you can express your answer in interval notation. For example:
- If the function is increasing on the intervals (-∞, a) and (b, ∞), write it as "Increasing on (-∞, a) ∪ (b, ∞)".
- If the function is decreasing on the interval (a, b), write it as "Decreasing on (a, b)".
- If the function is constant on the interval (a, b), write it as "Constant on (a, b)".
Replace "a" and "b" with the actual values found when analyzing the first derivative of the function.
for such more questions on intervals
https://brainly.com/question/28490141
#SPJ11
solve the given differential equation by undetermined coefficients. y'' 2y' y = sin(x) 7 cos(2x)
Answers
The general solution is y = y_h + y_p = c1 \(e^{ (-x) }\) + c2 x e^(-x) - (1÷2) cos(x) - (1÷12) sin(2x) - (5÷24) cos(2x).
What is Differential Equation ?
A differential equation is a mathematical equation that relates a function or a set of functions with their derivatives or differentials. In other words, it is an equation that describes the behavior of a system in terms of the rates of change of one or more variables.
First, we find the homogeneous solution of the differential equation:
The characteristic equation is r*r + 2r + 1 = 0, which can be factored as (r+1)(r+1) = 0. Hence, the homogeneous solution is y_h = c1 \(e^{ (-x) }\) + c2 x\(e^{ (-x) }\)
Now, we look for a particular solution of the form y_p = A sin(x) + B cos(x) + C sin(2x) + D cos(2x), where A, B, C, and D are constants to be determined.
Taking derivatives, we get y_p' = A cos(x) - B sin(x) + 2C cos(2x) - 2D sin(2x) and y_p'' = -A sin(x) - B cos(x) - 4C sin(2x) - 4D cos(2x).
Substituting y_p, y_p', and y_p'' into the differential equation, we get:
(-A sin(x) - B cos(x) - 4C sin(2x) - 4D cos(2x)) + 2(A cos(x) - B sin(x) + 2C cos(2x) - 2D sin(2x)) + (A sin(x) + B cos(x) + C sin(2x) + D cos(2x)) = sin(x) + 7cos(2x)
Simplifying and collecting like terms, we get:
(-3A - 3C + 4D) sin(2x) + (3B + 4C - 3D) cos(2x) + 2A cos(x) - 2B sin(x) = sin(x) + 7cos(2x)
Equating coefficients of sin(2x), cos(2x), sin(x), and cos(x), we get the following system of equations:
-3A - 3C + 4D = 0
3B + 4C - 3D = 7
2A = 0
-2B = 1
Solving for A, B, C, and D, we get:
A = 0
B = -1÷2
C = -1÷12
D = -5÷24
Therefore, the particular solution is y_p = (-1÷2) cos(x) - (1÷12) sin(2x) - (5÷24) cos(2x).
The general solution is y = y_h + y_p = c1 \(e^{ (-x) }\) + c2 x \(e^{ (-x) }\) - (1÷2) cos(x) - (1÷12) sin(2x) - (5÷24) cos(2x).
To learn more about Differential Equation from given link.
https://brainly.com/question/14620493
#SPJ1
The Function F(X,Y)=Xy2−9x Has Only One Critical Point Select One: True FalseThe Gradient Vector Of The Function F(X,Y)=Ln(X
Answers
The function f(x,y) = xy^2 - 9x has only one critical point (0,0), but it is not a maximum or minimum point; it is a saddle point.
Regarding the second question about the gradient vector of the function f(x,y) = ln(x), the gradient of this function is:
∇f(x,y) = < 1/x, 0 >
The function f(x,y) = xy^2 - 9x has only one critical point. This is true.
To find the critical points of a function, we need to find the points where the gradient of the function is zero or undefined. In this case, the gradient of f(x,y) is:
∇f(x,y) = < y^2 - 9, 2xy >
Setting this equal to zero and solving for x and y, we get:
y^2 - 9 = 0 and 2xy = 0
The first equation gives us y = ±3, and the second equation gives us x = 0 or y = 0.
So there are four points that satisfy these equations: (0,3), (0,-3), (0,0), and (9/2,0). However, we also need to check if these points are maximum, minimum, or saddle points. To do this, we can use the second derivative test or examine the behavior of f in the neighborhoods of these points.
For example, at (0,3), the Hessian matrix of f is:
H(f)(0,3) = [0 6]
[6 0]
This matrix has determinant (-36), which is negative, so this point is a saddle point. Similarly, we can check that the other three points are also saddle points.
Therefore, the function f(x,y) = xy^2 - 9x has only one critical point (0,0), but it is not a maximum or minimum point; it is a saddle point.
Regarding the second question about the gradient vector of the function f(x,y) = ln(x), the gradient of this function is:
∇f(x,y) = < 1/x, 0 >
So the gradient vector only depends on x, not on y. This is because the function f(x,y) = ln(x) does not depend on y; it only depends on x. Therefore, the partial derivative with respect to y is zero everywhere.
Learn more about functions from
https://brainly.com/question/11624077
#SPJ11
in which of these rows of the truth table is the compound proposition (p ∧ r) → ¬(q ∨ p) true? The row where both p and q are true, but ris false. The row where both p and rare true, but q is false. The row where p q and rare all false. The row where p, q and r are all true.
Answers
By using the truth table The correct options are (a) The row where both p and q are true, but r is false and (c) The row where p, q and r are all false.
Given, (p ^ r) -> ~(q v p)
Creating truth table:
=> p ^ r will return true if both the values of p and r are true else will return false.
=> q v p will return true if at least one of the values of q or p is true else will return false.
=>~(q v p) will return true if the truth value of q v p is false else will return false.
=>(p ^ r) -> ~(q v p) will return false if truth value of p ^ r is true and truth value of ~(q v p) is false else will return true.
p q r (p ^ r) (q v p) ~(q v p) (p ^ r) -> ~(q v p)
F F F F F T T
F F T F F T T
F T F F T F T
F T T F T F T
T F F F T F T
T F T T T F F
T T F F T F T
T T T T T F F
=> Option (a) is correct because according to the row number 7 where p = q = T and r = F, the result = T
=> Option (b) is wrong because according to row number 6, where p = q = T and q = F, the result = F
=>Option (c) is correct because according to row number 1, where p = q = r = F the result = T
=>Option (d) is wrong because according to the row number 8 where p = q = r = T the result = F
To learn more about Truth table here:
brainly.com/question/29155453
#SPJ4
As an estimation, we are told £3 is €4.
Convert £10.70 to euros.
Give your answer rounded to 2 DP.
Answers
Answer:
Given:
As an estimation, we are told £3 is €4.
To find:
Convert £64.60 to euros. Give your answer rounded to 2 DP.
Solution:
To solve the above problem we will use the unitary method as follows:
As estimated → If £ 3 is equivalent to € 4
Then, £ 1 will be equivalent to = €
∴ £ 64.60 will be equivalent to = €
Step-by-step explanation:
Now, we have to round the answer up to 2 decimal points to get the final answer
i.e., € 86.1311 ≈ € 86.13
Thus, £ 64.60 is approximately equal to → € 86.13.
Which function can be used to represent the graphed geometric sequence? f(x) = 80(One-fourth) Superscript x minus 1 f(x) = 320(One-fourth) Superscript x minus 1 f(x) = 80(4)x – 1 f(x) = 320(4)x – 1.
Answers
You can use the formula for getting the xth term of a geometric sequence for the graphed points.
The function that represents the graphed geometric sequence is given by
Option A: \(f(x) = 80 \times (\dfrac{1}{4})^{x-1}\)
What is a geometric sequence and how to find its xth terms?There are three parameters which differentiate between which geometric sequence we're taking about.
The first parameter is the initial value of the sequence.The second parameter is the quantity by which we multiply previous term to get the next term.The third parameter is the length of the sequence. It can be finite or infinite.Suppose the initial term of a geometric sequence is \(a\) and the term by which we multiply the previous term to get the next term is \(r\)
Then the sequence would look like
\(a, ar, ar^2 , ar^3 ....\)
(till the terms to which it is defined)
Thus, the xth term of such sequence would be
\(f(x) = \text{xth term} = ar^{x-1}\)
How to find the function representing the graphed geometric sequence?The points plotted in the graph are
(1,80), (2, 20), (3, 5)
The x coordinate shows the position of the term and the y coordinate shows the value of that position's term in the sequence.
So we have the sequence as
\(80, 20, 5 ...\)
Thus, a = 80
and we have
\(r = \dfrac{ar}{a} = \dfrac{20}{80} = \dfrac{1}{4}\)
Thus, the multiplication factor for the given sequence is r = 1/4
Thus, by using the xth term formula, we have:
\(f(x) = \text{xth term} = ar^{x-1} = 80 \times (\dfrac{1}{4})^{x-1}\\\\f(x) = 80 \times (\dfrac{1}{4})^{x-1}\)
Thus,
The function that represents the graphed geometric sequence is given by
Option A: \(f(x) = 80 \times (\dfrac{1}{4})^{x-1}\)
Learn more about geometric sequence here:
https://brainly.com/question/11266123

Answer:
The answer is Option A
Step-by-step explanation:
I hope this helps with you situation
X/12 = -11
ugh so hard
help me pls to make my sad face happy
:(
Answers
Answer:
-132
Step-by-step explanation:
x/12 = -11
⋅ 12 = ⋅ 12
x = -132
Isolate the variable using inverse operations.
(multiple both sides by 12)
x= -11(12)
Simplify.
*x= -132*
Hope this helps :))
kay is making a drink that needs 3 gallons of of juice , how many cups can she make of juice?
Answers
PLEASE I WLL GIVE BRAINLIEST JUST HELPS MEEEEE OMG IM ON MY SECOND ATTEMPT PLS PLS PLS HELP
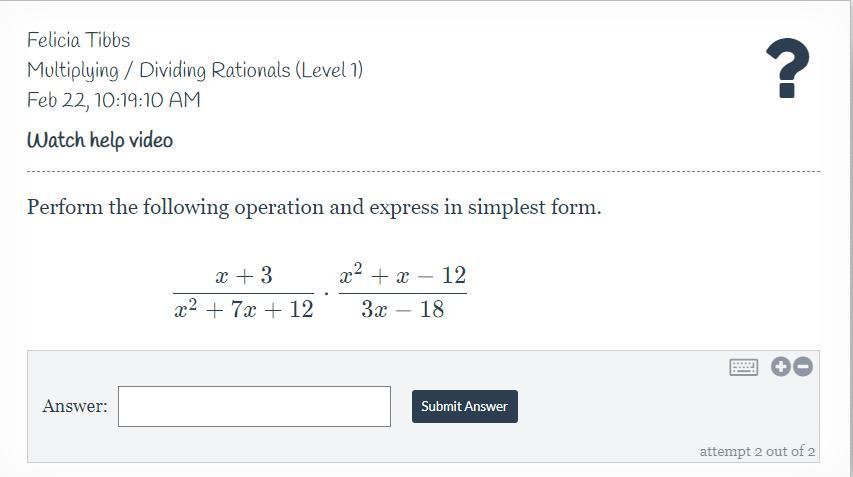
Answers
Answer:
\( \purple { \bold{ \frac{ (x - 3)}{3x - 18} }}\)
Step-by-step explanation:
\( \frac{x + 3}{ {x}^{2} + 7x + 12 } . \frac{ {x}^{2} + x - 12}{3x - 18} \\ \\ = \frac{x + 3}{ {x}^{2} + 4x + 3x + 12 } . \frac{ {x}^{2} + 4x - 3x - 12}{3(x - 6)} \\ \\ = \frac{x + 3}{ {x}(x + 4) + 3(x + 4) } . \frac{ {x}(x + 4) - 3(x + 4)}{3(x - 6)} \\ \\ = \frac{ \cancel{(x + 3)}}{ (x + 4) \cancel{(x + 3)} } . \frac{ (x + 4) (x - 3)}{3(x - 6)} \\ \\ = \frac{1}{ \cancel{ (x + 4)} } . \frac{ \cancel{ (x + 4)} (x - 3)}{3(x - 6)} \\ \\ \red{ \bold{= \frac{ (x - 3)}{3x - 18} }}\\ \\ \)
what is the initial value if the rate of change is -50 and after 4 hours your 200 meters away
Answers
The solution is, 400m is the initial value if the rate of change is -50 and after 4 hours your 200 meters away.
What is multiplication?
In mathematics, multiplication is a method of finding the product of two or more numbers. It is one of the basic arithmetic operations, that we use in everyday life.
here, we have,
the rate of change is -50
and after 4 hours your 200 meters away
so, in 4 hours change = -50*4
=-200
so, initial value = 200 -(-200)
= 400m
Hence, The solution is, 400m is the initial value if the rate of change is -50 and after 4 hours your 200 meters away.
To learn more on multiplication click:
brainly.com/question/5992872
#SPJ9
Find the most general real-valued solution to the linear system of differential equations x⃗ ′=[12−25]x⃗ .x→′=[1−225]x→.
Answers
The most general real-valued solution to the given linear system of differential equations is a combination of exponential terms involving eigenvalues and eigenvectors of the matrices A and B.
To find the most general real-valued solution to the linear system of differential equations:
x⃗ ′ = [12 − 25]x⃗
x→′ = [1 − 225]x→
Let's denote the matrix [12 − 25] as A and the matrix [1 − 225] as B. The system of differential equations can be written as:
x⃗ ′ = Ax⃗
x→′ = Bx→
To find the general solution, we need to solve the system of differential equations. Let's start with the first equation:
x⃗ ′ = Ax⃗
We can solve this equation by finding the eigenvalues and eigenvectors of matrix A. The eigenvalues, λ, are the solutions to the characteristic equation:
det(A - λI) = 0
where I is the identity matrix.
Solving this equation will give us the eigenvalues λ1 and λ2.
Once we have the eigenvalues, we can find the corresponding eigenvectors, v1 and v2.
The general solution for the first equation is then given by:
x⃗ = c1 * e^(λ1t) * v1 + c2 * e^(λ2t) * v2
where c1 and c2 are constants.
Now, let's move on to the second equation:
x→′ = Bx→
Similarly, we find the eigenvalues μ1 and μ2 of matrix B and the corresponding eigenvectors w1 and w2.
The general solution for the second equation is:
x→ = k1 * e^(μ1t) * w1 + k2 * e^(μ2t) * w2
where k1 and k2 are constants.
Combining the solutions for both equations, the most general real-valued solution to the given linear system of differential equations is:
x⃗ = c1 * e^(λ1t) * v1 + c2 * e^(λ2t) * v2
x→ = k1 * e^(μ1t) * w1 + k2 * e^(μ2t) * w2
where c1, c2, k1, and k2 are constants, and λ1, λ2, v1, v2, μ1, μ2, w1, and w2 are determined by the eigenvalue-eigenvector analysis of matrices A and B, respectively.
To know more about differential equations,
https://brainly.com/question/25731911
#SPJ11
Adam calculates his annual salary (base pay and commission), y, using the model y = 0.28x + 38,000, where x represents his total sales for the year. What is the meaning of the y-intercept in the model?
A. The y-intercept represents Adam's base pay
B. The y-intercept represents the highest salary Adam can earn
C. The y-intercept represents Adam's total sales when he earned no commission
D. The y-intercept represents Adam's commission pay when he had zero total sales
the y-intercept represents Adam's commission pay when he had zero total sales
Answers
Answer: A
Step-by-step explanation:
The Y intercept is his base pay, because no matter what he is getting 38,000. So, his total salary will always start out at 38,000 then continue up with commission sales.
NEED HELP NOWAngles not necessarily drawn to scale

Answers
Answer:
x = 45 degrees
Step-by-step explanation:
Opposite adjacent angles are congruent
160 - x = 115
x = 45
A test has a mean of 80 with a standard deviation of 4. Which of the following scores is within one standard deviation of the mean?A75B77СBOD90E09
Answers
The 89 scores are all within one standard deviation of the mean.
The range of data within one standard deviation of the mean is expressed as the mean plus or minus one standard deviation.
Since the mean in this situation is 80 and the standard deviation is 4, the range that falls within the mean's one standard deviation is as follows:
\(80±4 = (76, 84)\)
Therefore, scores A (75) and B (77) are both below this interval, score C (90) is above this interval, and score D (90) is much higher than this interval. Only score E (89) is within this interval
The 89 scores are all within one standard deviation of the mean.
so E) 89 is the right response.
To know more about mean visit
https://brainly.com/question/31101410
#SPJ4
It takes Boeing 29,454 hours to produce the fifth 787 jet. The learning factor is 80%. Time required for the production of the eleventh 787 : 11th unit time hours (round your response to the nearest whole number).
Answers
Boeing takes 29,454 hours to produce the fifth 787 jet. With an 80% learning factor, the time required for the production of the eleventh 787 is approximately 66,097 hours.
To calculate the time required for the production of the eleventh 787 jet, we can use the learning curve formula:
T₂ = T₁ × (N₂/N₁)^b
Where:
T₂ is the time required for the second unit (eleventh in this case)
T₁ is the time required for the first unit (fifth in this case)
N₂ is the quantity of the second unit (11 in this case)
N₁ is the quantity of the first unit (5 in this case)
b is the learning curve exponent (log(1/LF) / log(2))
Given that T₁ = 29,454 hours and LF (learning factor) = 80% = 0.8, we can calculate b:
b = log(1/LF) / log(2)
b = log(1/0.8) / log(2)
b ≈ -0.3219 / -0.3010
b ≈ 1.0696
Now, substituting the given values into the formula:
T₂ = 29,454 × (11/5)^1.0696
Calculating this expression, we find:
T₂ ≈ 29,454 × (2.2)^1.0696
T₂ ≈ 29,454 × 2.2422
T₂ ≈ 66,096.95
Rounding the result to the nearest whole number, the time required for the production of the eleventh 787 jet is approximately 66,097 hours.
Learn more about exponent here: https://brainly.com/question/29277932
#SPJ11
(close reading) Which letter is angrier?Find a quote to support your claim
Answers
I think that Jefferson's letter is angrier.
Why do I think so?I think this because you can kind of tell from the first sentences of both letters, Hamilton is kinder when he says, "Sir: --I have the pleasure of your private letter on the 26th of August."
However, Jefferson says something not really rude, but not as respectful than Hamilton to the President. He says, "DEAR SIR, I received your letter of August 23rd."
The two letters in question here is the letters written by both Alexander Hamilton and Thomas Jefferson to the President about the war between France and Britain.
Because he supported America throughout the Revolutionary War, Jefferson supported France. Hamilton supported the British because he believed it would facilitate trade.
Read more about letters here:
https://brainly.com/question/24623157
#SPJ1
Find the product (5) (8) (-7) (-4) (6)
Answers
Answer: 6720
Step-by-step explanation:
Assuming that is 5x8x-7x-4x6,
5(8) is 40.
-7(-4) is 28 because a negative number times a negative number is positive
40x28 is 1120 and 1120(6) is 6720
Helllllpppp me please I'm begging you please

Answers
Answer:
Step-by-step explanation:
1/5 = 0.2 ÷ 2 = 0.1 pounds
Option C is the correct answer