15(123)−72−2⋅7? i need help with this
Answers
Answer:
1770.3
Step-by-step explanation:
BODMAS
=15(123)-72-2.7
=1845-72-1.7
=1770.3.
Related Questions
Samantha has decided to donate $501,000 to a university. If the
endowment will earn a return of 9%, how much can be spent each year
while ensuring the funds last forever?
Answers
Samantha can spend approximately $45,090 each year from the endowment to ensure the funds last forever, assuming a return rate of 9%.
To determine how much can be spent each year while ensuring the funds last forever, we can use the concept of a perpetuity. A perpetuity is a series of equal payments that continues indefinitely.
The amount that can be spent each year from an endowment can be calculated using the following formula:
Annual Spending = Endowment Amount * Return Rate
In this case, Samantha has decided to donate $501,000 to the university, and the endowment is expected to earn a return of 9%.
Annual Spending = $501,000 * 0.09
Annual Spending = $45,090
Therefore, Samantha can spend approximately $45,090 each year from the endowment to ensure the funds last forever, assuming a return rate of 9%.
Learn more about perpetuity from the given link
https://brainly.com/question/30736885
#SPJ11
simplify
5cat + 3act - 6tac
Answers
Answer:
the correct answer is 2 act
Step-by-step explanation:
there is same base (act)
then 5+3=8
and 8-6 = 2act
Help please!! Math help!! asap please!!!

Answers
Answer:
4, 15/5 , 0.5, -1/5, -1.2 , -49
Step-by-step explanation:
4
15/5=3
0.5
-1/5=-0.2
-1.2
-49
We found that the estimated rate of increase in the cost when x = 60,000 is $318 per game system. We are asked to compare this estimate to the exact cost of producing the 60,001st game system. In order to find the exact cost of producing the 60,001st game system, we can find the exact cost of producing 60,000 game systems in an hour and the exact cost of producing 60,001 game systems in an hour and take the difference of the two numbers. Recall that the cost for the company to produce x game systems per hour is given by the cost function C(x) = 700,000 + 270x + 0.0004x². So the cost for the company to produce 60,000 game systems in an hour is given by the following. C(x) = 700,000 + 270x + 0.0004x² C(60,000) = 700,000 + 270(60,000) + 0.0004(60,000)² The cost for the company to produce 60,001 game systems in an hour is given by the following. C(x) = 700,000 + 270x + 0.0004x² C(60,001) = 700,000 + 270(60,001) + 0.0004(60,001)² = X Therefore, the exact cost for the company to produce the 60,001st game system is given by the following. C(60,001) C(60,000) - = Comparing this to the estimated value of $318 per game system, the actual value is [---Select--- the estimated value.
Answers
Once you have calculated the exact cost for the 60,001st game system, you can compare it to the estimated value of $318 per game system.
To compare the estimated rate of increase in cost ($318 per game system) to the exact cost of producing the 60,001st game system, we can calculate the difference between the exact cost of producing 60,001 game systems and the exact cost of producing 60,000 game systems.
Given the cost function: C(x) = 700,000 + 270x + 0.0004x², where x represents the number of game systems produced per hour.
The cost for the company to produce 60,000 game systems in an hour is:
C(60,000) = 700,000 + 270(60,000) + 0.0004(60,000)²
The cost for the company to produce 60,001 game systems in an hour is:
C(60,001) = 700,000 + 270(60,001) + 0.0004(60,001)²
To find the exact cost for the company to produce the 60,001st game system, we can subtract the cost of producing 60,000 game systems from the cost of producing 60,001 game systems:
C(60,001) - C(60,000)
Substituting the values, we have:
[700,000 + 270(60,001) + 0.0004(60,001)²] - [700,000 + 270(60,000) + 0.0004(60,000)²]
Simplifying the expression, we can calculate the exact cost for the 60,001st game system.
As the values for C(60,001) and C(60,000) are not provided in the question, you will need to substitute the corresponding expressions and compute the result using a calculator or mathematical software to find the exact cost.
Once you have calculated the exact cost for the 60,001st game system, you can compare it to the estimated value of $318 per game system. Based on the calculated result, you can determine whether the actual value is larger, smaller, or equal to the estimated value.
Learn more about value here:
https://brainly.com/question/30145972
#SPJ11
How to solve this please

Answers
The value of x, considering the proportional relationship in this problem, is given as follows:
\(x = 0.77 \times 10^{-46}\)
What is a proportional relationship?A proportional relationship is a relationship in which a constant ratio between the output variable and the input variable is present.
The proportional relationship for this problem is given as follows:
1u - \(6.02 \times 10^{23}\)
x u - \(4.65 \times 10^{-23}\)
Applying cross multiplication, the value of x is given as follows:
\(x = \frac{4.65 \times 10^{-23}}{6.02 \times 10^{23}}\)
\(x = 0.77 \times 10^{-46}\)
A similar problem, also featuring proportional relationships, is presented at https://brainly.com/question/7723640
#SPJ1
675 grams of sweets were distributed among 9 children. How much sweet was given to each child?
Answers
Answer:
75 Sweets
Step-by-step explanation:
To get the answer you have to divide the number of sweets by the number of children. If there are 675 grams and 9 children, then you divide 675/9 to get your answer of 75 sweets.
Each child was given 75 grams of sweets as per linear equation when distributed.
What is a linear equation?A linear equation is an equation where the highest degree of the variable is one.
Given, there are a total of 675 grams of sweets.
There are a total of 9 children among which the sweets is to be distributed equally.
Therefore, each children will get sweets of
(675 ÷ 9) grams
= 75 grams.
Therefore, each child will get 75 grams of sweets when it is distributed equally.
Learn more about a linear equation here: https://brainly.com/question/18467717
#SPJ2
What is 1/6
as a decimal?
Give your answer rounded to 3 decimal places.
Answers
0.167 I believe!
<3
I'm sure you'll do great!
Answer:
0.167(rounded 3dp)
Step-by-step explanation:
100/6=16.6666...
\(\frac{16.666}{100}\)= \(\frac{1}{6}\)
\(\frac{16.666}{100}=(into decimal)0.167\)
If gasoline costs $3.92 per gallon, and filling up a car’s tank costs $49.00, how many gallons does the tank hold?__________ gallons
HELP ASAPPPPPPP! THIS IS DUE AT 11:59 P.M. AND I HAVE BEEN DOING THIS TEST FOR SO LONG I CANT EVEN FUNCTION PROPERLY, PLEASE HELP :(
Answers
Answer:
12.5
Step-by-step explanation:
49.00/3.92=12.5
(PLEASE HELP ASAP)
Mr. Butler asked his 3rd period students if they had a part-time job or if they played a sport for the school. He created a VENN diagram to display the data.
What is the probability a student has a part-time job given they play a sport?
a) 17
b).40
c) .05
d) .44

Answers
Match each equation with its solution...I dont get this

Answers
Answer:
go to demos.com (calculator) trust me amd type the equation in as so ex: e+ 3/8 = 2 type it in as 2- 3/8 then you'll see the little fraction number by your answer sometimes youll have to press that
Can someone slove this equation system please????

Answers
Answer:
Step-by-step explanation:
Multiply the top equation by 3.
3*(x - y)/3 = 0.5 * 3
(x - y) = 1.5
There is no single solution that satisfies this system. There infinitely many values for x and y that will work. The only restriction is that x - y = 1,5
So if you make y = 3
x - 3 = 1.5 Add 3 to both results
x - 3 + 3 = 1.5 + 3 Combine
x = 4.5
Infinitely many solutions exist.
Solve problems 1 to 4 using the pigeonhole principle. For each problem, explain why you can apply the pigeonhole principle. Clearly indicate the pigeons, the pigeonholes, and a rule assigning each pigeon to a pigeonhole. 1. Consider a standard deck of 52 cards. A poker hand has 5 cards. In a poker hand, must there be at least two cards of the same suit?
Answers
To determine whether there must be at least two cards of the same suit in a poker hand, we can apply the pigeonhole principle.
The pigeonhole principle states that if you distribute more objects into fewer containers (pigeonholes), at least one container must contain more than one object.
In this case, the pigeons are the cards in the poker hand, and the pigeonholes are the four different suits (hearts, diamonds, clubs, and spades). The rule assigning each pigeon to a pigeonhole is that each card is assigned to its corresponding suit pigeonhole.
Now, let's consider the situation. We have a poker hand consisting of 5 cards. Since there are only four suits available, at least one of the suits must have more than one card assigned to it. This is because if each of the four suits had only one card, we would have a total of 4 cards, which is fewer than the 5 cards in a hand.
By the pigeonhole principle, if one suit has more than one card, there must be at least two cards of the same suit in the poker hand. Therefore, it is guaranteed that in any poker hand, there will be at least two cards of the same suit.
This conclusion holds true regardless of the specific arrangement of the cards in the hand. The pigeonhole principle provides a logical reasoning that ensures the existence of at least two cards of the same suit in a poker hand, based solely on the number of cards and suits involved.
Learn more about Pigeonhole Principle :
https://brainly.com/question/31876101
#SPJ11
Given m|n, find the value of x.
(8x+10)
mm
(6x+30)
>
Answer:

Answers
Answer:
x = 10
Step-by-step explanation:
When any 2 lines are parallel to each other and a transversal intersects both the lines , then THE INTERIOR ALTERNATIVE ANGLES ARE ALWAYS EQUAL.
According to the question , m || n & t is transversal. So, the interior alternative angles will be equal.
\( = > 8x + 10 = 6x + 30\)
\( = > 8x - 6x = 30 - 10\)
\( = > 2x = 20\)
\( = > x = \frac{20}{2} = 10\)
Answer:
The value of X is 10.
Step-by-step explanation:
solution
(8x+10)=(6x+30) (alternate angle)
8x-6x=30-10
2x=20
X=20/2
X=10
Jenny applies a force of 600 N to the floor. The
total area of her feet is 160 cm ². What is the
pressure, in N/m², between her and the floor if
she stands on both of her feet?
Answers
Pressure is the amount of force applied per unit area. It is a measure of how much force is distributed over a given surface area and is commonly measured in units such as Pascals (Pa) or pounds per square inch (psi). In physics, pressure can be described by the formula:
The pressure between Jenny and the floor can be calculated by dividing the force she applies by the total area of her feet. First, we need to convert the area of her feet from cm² to m², since pressure is measured in N/m². We can do this by dividing the area by 10,000 (since there are 10,000 cm² in 1 m²):
160 cm² ÷ 10,000 = 0.016 m²
Now we can calculate the pressure:
Pressure = Force ÷ Area
Pressure = 600 N ÷ 0.016 m²
Pressure = 37,500 N/m²
Therefore, the pressure between Jenny and the floor when she stands on both feet is 37,500 N/m². This means that for every square meter of floor that Jenny's feet are in contact with, there is a force of 37,500 N pushing against it. It's worth noting that this is quite a high pressure - for comparison, the average atmospheric pressure at sea level is around 101,325 N/m². This is because Jenny's weight is concentrated over a relatively small area (her feet), whereas the weight of the entire atmosphere is distributed over the entire surface of the Earth.
To know more about Pressure visit:
https://brainly.com/question/12971272
#SPJ11
please provide an answer

Answers
Answer:
\(<D=95\)
Step-by-step explanation:
The opposite angles in a quadrilateral theorem states that when a quadrilateral is inscribed in a circle, the angles that are opposite each other are supplementary, their degree measures add up to 180 degrees. One can apply this here by using the sum of (<C) and (<A) to find the measure of the parameter (z). Then one can substitute in the value of (z) to find the measure of (<B). Finally, one can use the opposite angles in a quadrilateral theorem to find the measure of angle (<D) by using the sum of (<B) and (D).
Use the opposite angles in an inscribed quadrialteral theorem,
<A + <C = 180
Substitute,
14x - 7 + 8z = 180
Simplify,
22z - 7 = 180
Inverse operations,
22z = 187
z = \(\frac{187}{22}\)
Simplify,
z = \(\frac{17}{2}\)
Now substitute the value of (z) into the expression given for the measure of angle (<B)
<B = 10z
<B = 10(\(\frac{17}{2}\))
Simplify,
<B = 85
Use the opposite angles in an inscribed quadrilateral theorem to find the measure of (<D)
<B + <D = 180
Substitute,
85 + <D = 180
Inverse operations,
<D = 95
10z=85 degree
8z=68 degree

1. In which quadrant does θ lie given that sinθ>0 and cosθ<0?
Quadrant I
Quadrant II
Quadrant III
Quadrant IV
Answers
Answer:
\(\theta\) is in quadrant II
Step-by-step explanation:
Given
\(\sin(\theta) > 0\) and \(\cos(\theta) < 0\)
Required
Where is \(\theta\)
\(\sin(\theta) > 0\) and \(\cos(\theta) < 0\) imply that
\(\theta\) is in quadrant II
Because that is only quadrant where \(\sin(\theta) > 0\) and \(\cos(\theta) < 0\) exist.
Answer:
Quadrant II
Step-by-step explanation:
just took the test :D
have a miraculous day!!! <3
Find a number between 2 and 1/8 which is as a mixed number 17/8 and 2.1251
Answers
Answer:
1?
Step-by-step explanation:
Just need the solution set, for some reason my calculator will not compute it

Answers
ANSWER:
\(x=\frac{6\ln5-5\ln7}{2\ln7-\ln5}\)The value of x is equal to -8.49
STEP-BY-STEP EXPLANATION:
We have the following equation:
\(7^{2x+5}=5^{x-6}\)We solve for x:
\(\begin{gathered} (2x+5)\cdot\ln (7)=(x-6)\ln (5) \\ \ln 7\cdot2x+5\ln 7=\ln 5\cdot x-6\ln 5 \\ \ln 7\cdot2x-\ln 5\cdot x=-6\ln 5-5\ln 7 \\ x(2\ln 7-\ln 5)=-6\ln 5-5\ln 7 \\ x=\frac{6\ln 5-5\ln 7}{2\ln 7-\ln 5} \\ x\cong-8.49 \end{gathered}\)The value of x is equal to -8.49
You accept a job that pays out $15,000 your first year. You are given the option of either earning an additional $500 each year you work or 2% of your current salary. Your plan is to stay with this job for 4 years. Which option do you choose and how much will you make? 2% or $500? $
Answers
To compare the two options, we need to calculate the total earnings for each option over the 4-year period.
Option 1: Earning an additional $500 each year
The total earnings for this option will be:
Year 1: $15,000
Year 2: $15,500 ($15,000 + $500)
Year 3: $16,000 ($15,500 + $500)
Year 4: $16,500 ($16,000 + $500)
Total earnings over 4 years = $15,000 + $15,500 + $16,000 + $16,500 = $63,000
Option 2: Earning 2% of current salary
To calculate the earnings for this option, we need to find the salary for each year using the formula:
salary = $15,000 + 2% × (previous year's salary)
Year 1: salary = $15,000
Year 2: salary = $15,300 ($15,000 + 2% × $15,000)
Year 3: salary = $15,606 ($15,300 + 2% × $15,300)
Year 4: salary = $15,924.12 ($15,606 + 2% × $15,606)
Total earnings over 4 years = $15,000 + $15,300 + $15,606 + $15,924.12 = $61,830.12
Therefore, the first option of earning an additional $500 each year results in higher total earnings of $63,000 over 4 years, compared to earning 2% of the current salary, which results in total earnings of $61,830.12. Hence, the first option of earning an additional $500 each year is the better choice.
Find the values of x and y
A. X=34, y=17 √3
B. X=17 √3, y=34
C. X=34 √3, y=17
D. X=17, y=34 √3

Answers
Step-by-step explanation:
B
\( \tan(30) = \frac{17}{x} \\ x = \frac{17}{ \tan(30) } \\ x = \frac{17}{0.577} = 29.46\)
\( \sin(30) = \frac{17}{y} \\ y = \frac{17}{ \sin(30) } = 34\)
What is the y-intercept of the line with the equation y = one-half x minus 3? a. -3 c. -6 b. 6 d. One-half
Answers
Select the equations in which
w
=
100
makes the equation true. Select all that apply.
A.
0
.
54
÷
w
=
0
.
0054
B.
4
,
086
÷
w
=
4
.
086
C.
362
.
7
÷
w
=
3
.
627
D.
5
.
3
÷
w
=
0
.
0053
E.
21
.
9
÷
w
=
0
.
219
Answers
Answer:
The answer is A, C, and E
this one confuses me
focuses on pythagorean triples :)

Answers
Answer:
EH is 9 cm
DE is 18 cm
x is
18^2=9^2+b^2
b≈15,588
Help!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!1

Answers
Answer:
"A ceiling fan"
Step-by-step explanation:
Cycles that may repeat or break.
What is the area of a square with side lengths of 3/5 units? Please explain how you know.
Answers
When trying to an area of a square, you multiply one side by another side to get the answer (length x width).
3/5 x 3/5 = 9/25
Numerator x numerator.
Denominator x denominator.
Please help me, explain
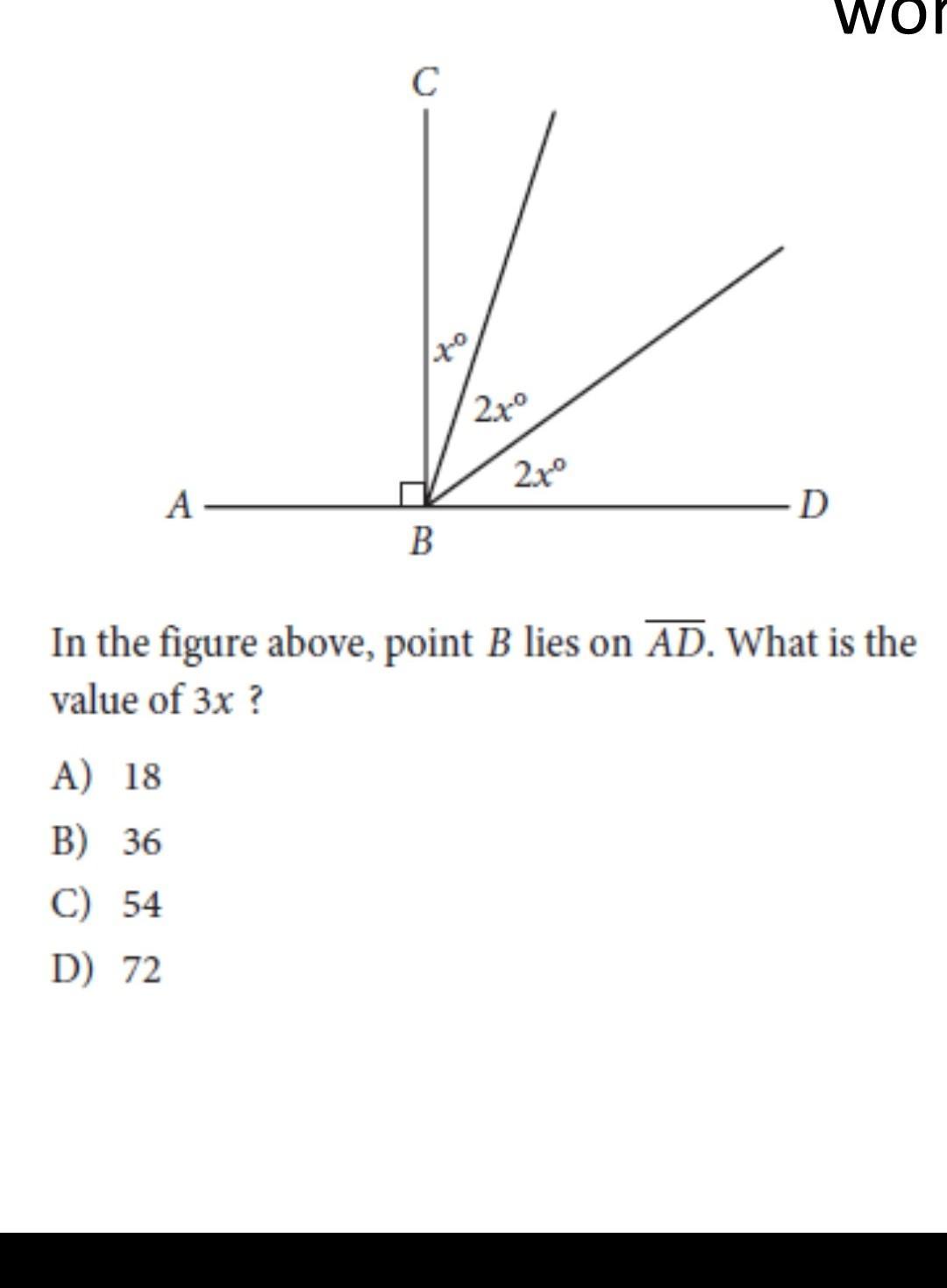
Answers
Answer:
C) 3x=54
Step-by-step explanation:
Angle ABD is a straight angle, which means it measures 180 degrees.
Angles ABC & CBD are linear pairs. (The two of them add up to 180°)
ABD is a right angle (90 degrees)
The straight angle (180) minus the right angle (90) equals 90 degrees.
x+2x+2x=90°
Combine like terms
5x=90°
divide by 5 on both sides
x=18
18×3=54
C
Hope this helps :-)
Solve the inequality and enter your solution as an inequality in the box below, using "" for if necessary. 8 >4-x>6

Answers
Answer:
-4 < x < -2
Step-by-step explanation:
8 > 4 - x > 6
4 > -x > 2
-4 < x < -2 (flip the sign when multiplying / dividing by a negative number)
2. find the perimeter of a rectangle with a length
of q and a width of (q-2).
Answers
Answer: 4q-4
Step-by-step explanation:
2(q+q-2) = 2(2q-2) = 4q-4
pls help! correct gets thanks and brainliest :)

Answers
Answer:
\( m\angle SYX=76\degree \)
Step-by-step explanation:
\( m\angle SYX = m\angle UYV\)
(Vertical angles)
\( \because m\angle UYV=76\degree \)
\( \therefore m\angle SYX=76\degree \)
Please help 60 points for a rapid answer-In the figure below which of the following is true in circle E?


Answers
Answer:
all 3 options are true : A, B, C
Step-by-step explanation:
warning : it has come to my attention that some testing systems have an incorrect answer stored as right answer for this problem.
they say that A and C are correct.
but I am going to show you that if A and C are correct, then also B must be correct.
therefore, my given answer above is the actual correct answer (no matter what the test systems say).
originally the information about the alignment of the point F in relation to point E was missing.
therefore, I considered both options :
1. F is on the same vertical line as E.
2. F is not on the same vertical line as E.
because of optical reasons (and the - incomplete - expected correct answers of A and C confirm that) I used the 1. assumption for the provided answer :
the vertical line of EF is like a mirror between the left and the right half of the picture.
A is mirrored across the vertical line resulting in B. and vice versa.
the same for C and D.
this leads to the effect that all 3 given congruence relationships are true.
if we consider assumption 2, none of the 3 answer options could be true.
but if the assumptions are true, then all 3 options have to be true.
now, for the "why" :
remember what congruence means :
both shapes, after turning and rotating, can be laid on top of each other, and nothing "sticks out", they are covering each other perfectly.
for that to be possible, both shapes must have the same basic structure (like number of sides and vertices), both shapes must have the same side lengths and also equally sized angles.
so, when EF is a mirror, then each side is an exact copy of the other, just left/right being turned.
therefore, yes absolutely, CAD is congruent with CBD. and ACB is congruent to ADB.
but do you notice something ?
both mentioned triangles on the left side contain the side AC, and both triangles in the right side contain the side BD.
now, if the triangles are congruent, that means that each of the 3 sides must have an equally long corresponding side in the other triangle.
therefore, AC must be equal to BD.
and that means that AC is congruent to BD.
because lines have no other congruent criteria - only the lengths must be identical.