15% of 500 is what number
Answers
Answer: 75
Step-by-step explanation: 15÷100×500 = 75
Answer:
75
Step-by-step explanation:
if you divide 500 by 10, you have 10%
500/10=50
and if you divide 500 by 5, you have 5%
500/5=25
25+50=75
Related Questions
Use the quadratic formula to find the exact solutions of x2 − 9x + 5 = 0.
x equals negative b plus or minus the square root of b squared minus 4 times a times c, all over 2 times a
Answers
Answer:
\(\frac{9\pm\sqrt{61}}{2}\)
Step-by-step explanation:
\(\displaystyle x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}=\frac{-(-9)\pm\sqrt{(-9)^2-4(1)(5)}}{2(1)}=\frac{9\pm\sqrt{81-20}}{2}\\\\=\frac{9\pm\sqrt{61}}{2}\)
9 = v + 6 solve for v
Answers
Answer: 3
Step-by-step explanation:
Basically all you have to do is subtract 6 from both sides so you will get:
3 = v
Help fast please geometry

Answers
Answer:
x = \(\frac{9\sqrt{6} }{2}\)
Explanation:
using sohcahtoa method:
middle line: 9 ÷ sin(45) = 9√2
x = sin(60) * 9√2 = \(\frac{9\sqrt{6} }{2}\)
Lucas writes a numerical expression that has a value of 37. Which of the following expressions did Lucas write?
Answers
Answer:
a) 2.5^2+19\times2.5-16.75
Step-by-step explanation:
(2.5)² +(19× 2.5) - 16.75
= 6.25 + 47.5 - 16.75 = 37
The length of the deck is 3 times it’s width. If the decks perimeter is 16 ft what is the decks area
Answers
Answer:
12 ft²Step-by-step explanation:
Givenl = 3wP = 16A = ?SolutionUse perimeter formula
P= 2(l + w) = 2(w + 3w) = 8wFind width:
8w = 16 ⇒ w = 2 ftFind length:
l = 3*2= 6 ftFind area:
A = lw = 6*2 = 12 ft²When a process is said to be at six sigma level, what does it
mean? (7 points)"
Answers
Answer:
Step-by-step explanation: It means a high level of quality, with 3.4 defects per million opportunities. It is this sigma level that leads to the term Six Sigma, which is a philosophy of delivering near perfect products or services by eliminating variabilities that lead to defects.
1. If f(x) = (3x-2)/(2x+3), then f'(x) =
Answers
Answer:
\(f'(x)= \frac{13}{(2x+3)^2}\\\)
Step-by-step explanation:
\(f(x)= \frac{3x-2}{2x+3} \\\)
\(f'(x)=\frac{dy}{dx} = \frac{d}{dx}(\frac{3x-2}{2x+3})\\ f'(x)= \frac{(2x+3)\frac{d}{dx}(3x-2)-(3x-2)\frac{d}{dx}(2x+3) }{(2x+3)^{2} } \\f'(x)= \frac{(2x+3)(3)-(3x-2)(2)}{(2x+3)^{2} } \\\)
\(f'(x)= \frac{6x+9-6x+4}{(2x+3)^{2} }\\ f'(x)= \frac{13}{(2x+3)^2}\\\)
Find the common ratio of the geometric sequence: 8, -2, 1/2, -1/8
Answers
Answer:
-1/4
Step-by-step explanation:
You just need to see what number is being divided from each term
Find the common ratio r for each geometric sequence and use r to find the next three terms. 8, 4, 2, 1, ... 200, -80, 32, -12.8, ...
Answers
Answer:
the sequence is the a geometric sequence because the terms has a common ratio. the common ratio = -80 / 200 = -2 / 5common ratio = 32 / -80 = -2 /5
so the next to term can be solve multiplying the last term with the common ratio
x = -12.8 ( -2 / 5)x = 5.12
y = 5.12 ( - 2 / 5)y = -2.048
Step-by-step explanation: HOPE THIS HELPS!!
a combination of three or more tones is called a(n) group of answer choices interval. scale. chord. octave.
Answers
A chord is a combination of three or more tones (notes) played together.
To determine the notes in a chord, we need to calculate the intervals between the notes. An interval is the distance between two notes. Intervals are measured in half steps (or semitones). A half step corresponds to the interval between two adjacent keys of a piano.
In a major chord, the formula for determining the intervals is 1-3-5. This means the root note (1st note) is a major third (4 half steps) away from the 3rd note and a perfect fifth (7 half steps) away from the 5th note.
For example, if the root note is C, the 3rd note is E and the 5th note is G. The intervals in this chord are C to E (4 half steps) and C to G (7 half steps). Therefore, the chord is a C major chord.
In a minor chord, the formula for determining the intervals is 1-b3-5. This means the root note is a minor third (3 half steps) away from the 3rd note and a perfect fifth (7 half steps) away from the 5th note.
For example, if the root note is F, the 3rd note is Ab and the 5th note is C. The intervals in this chord are F to Ab (3 half steps) and F to C (7 half steps). Therefore, the chord is a F minor chord.
Learn more about combination here:
https://brainly.com/question/14685054
#SPJ4
Hello! The question is posted below in the photo, willing to award brainliest.

Answers
Answer:
-1.5
Step-by-step explanation:
Hope this helps .
Pls mark brainliest.
Thank if correct :)

Find the range for the measure of the third side of a triangle when the measures of the other two sides are 6 ft and 19 ft.
Answers
The range of possibilities for the third side of the triangle, after using the triangle inequality theorem is 13 < n < 25.
According to the theorem of triangle inequality, the sum of any two of a triangle's sides must exceed the length of the third side. When we know the lengths of two sides, x and y, we can apply the triangle inequality theorem to estimate the length of the third side, which will be between x + y and x - y.
As a result,
x – y < n < x + y
19 – 6 < n < 19 + 6
13 ft < n < 25 ft
To learn more about triangle inequality theorem, click here:
https://brainly.com/question/30095626
#SPJ4
Please help.
Indices and Standard Form question

Answers
Answer:
A= 4.5×10^11
B= 3.5×10^3
Step-by-step explanation:
A
(5×10^3)= 5000
(9×10^7)=90000000
multiply both of them
=450000000000 or 4.5×10^11
B
(7×10^5)=700000
(2×10^2)=200
700000÷200
3500 or 3.5×10^3
Write each product or quotient in scientific notation. Round to the appropriate number of significant digits.
(7.2×10¹¹) (5×10⁶)
Answers
The product of (7.2×10¹¹) and (5×10⁶) in scientific notation, rounded to the appropriate number of significant digits, is 3.6 × 10¹⁸.
To write each product or quotient in scientific notation, we first need to multiply the numbers and then adjust the result to scientific notation. Let's start with the multiplication:
(7.2×10¹¹) (5×10⁶)
To multiply these numbers, we can simply multiply the coefficients (7.2 and 5) and add the exponents (10¹¹ and 10⁶):
(7.2 × 5) × (10¹¹ × 10⁶)
= 36 × 10¹⁷
Now, to express this result in scientific notation, we need to have a coefficient between 1 and 10. We can achieve this by moving the decimal point one place to the left:
3.6 × 10¹⁸
Therefore, the product of (7.2×10¹¹) and (5×10⁶) in scientific notation, rounded to the appropriate number of significant digits, is 3.6 × 10¹⁸.
To know more about scientific notation refer here:
https://brainly.com/question/16936662
#SPJ11
Which fraction comparison reasoning strategy can be used when both fractions have the same number of pieces?
Answers
The positon of a particle in the xy - plane at time t is r(t)=(cos2t)i + (3sin2t)j, t=0. Find an equation in x and y whose graph is the path of the particle. Then find the particle's acceleration vectors at t=0.
Answers
The equation in x and y representing the path of the particle is x² + 9y² = 1. This equation describes an ellipse centered at the origin. At t = 0, the particle's acceleration vector is -4i.
The given position vector of the particle in the xy-plane is r(t) = (cos(2t))i + (3sin(2t))j, where t represents time. We are also given t = 0. To find an equation in x and y that represents the path of the particle, we need to eliminate the parameter t.
We can express x and y in terms of t as follows:
x = cos(2t)
y = 3sin(2t)
To eliminate t, we can use the trigonometric identity cos²(θ) + sin²(θ) = 1. Rearranging this identity, we have:
sin²(θ) = 1 - cos²(θ)
Substituting x = cos(2t) and y = 3sin(2t) into the identity, we get:
sin²(2t) = 1 - cos²(2t)
(3sin(2t))² = 1 - (cos(2t))²
9y² = 1 - x²
Therefore, the equation in x and y representing the path of the particle is:
x² + 9y² = 1
Next, to find the particle's acceleration vector at t = 0, we need to differentiate the position vector twice with respect to time. Let's calculate it step by step:
r'(t) = (-2sin(2t))i + (6cos(2t))j
r''(t) = (-4cos(2t))i - (12sin(2t))j
Evaluating at t = 0, we get:
r'(0) = -2i + 6j
r''(0) = -4i
Therefore, the particle's acceleration vector at t = 0 is -4i.
To find an equation representing the path of the particle, we eliminated the parameter t by expressing x and y in terms of t and applying a trigonometric identity. This yielded the equation x² + 9y² = 1, which represents an ellipse centered at the origin with x and y as the variables.
Next, we found the particle's acceleration vector by differentiating the position vector twice with respect to time. Evaluating at t = 0, we obtained the acceleration vector as -4i. This indicates that the particle has constant acceleration along the x-axis, while its acceleration along the y-axis is zero.
These calculations provide insights into the motion of the particle. The equation of the path gives a geometric representation of the particle's trajectory, while the acceleration vector at t = 0 gives information about the particle's instantaneous acceleration at that specific time.
Learn more about trigonometric here:
https://brainly.com/question/29156330
#SPJ11
Draw a model of √12 using perfect squares.
Answers
Answer:
2√3
Step-by-step explanation:
√12 =
√4 * √3 =
√2^2 * √3 =
2 * √3 =
2√3
https://brainly.com/question/16800358
Answer:
We can write 12 as 4 + 4 + 4, or 4 ∙ 3. So, create three squares that each have two columns and two rows.
a series of 3 squares, each composed of 4 smaller squares
so √12 =√4 x 3 = √4 x √3 = 2√3
Step-by-step explanation:
I have a cockroach problem in my living room. Don't ask how, but I counted 125 cockroaches
today. And they are growing at a rate of 20% every day.
How many cockroaches do you think I'll have after three days?
Answers
Answer:
216 cockroaches
Step-by-step explanation:
Let's establish that 125 cockroahes is Day 0, the start. This means Day 1 is the next day.
Day 0 is 125 cockroaches.
They grow at 20% per day. That is a factor of 120% for the second day.
(125)*(1.20) = 150 cockroaches on Day 1.
The second day would be (150)*(1.20) = 180 cockroaches on Day 2.
The third day is (180)*(1.20) = 216 cockroaches on Day 3.
Let C(x) be the number of cockroaches on the xth day. The resulting formula for this would be:
The third day (x=3)
C(x) = 125*(1.20)^x
C(3) = (1.25)^(1.20)^3
correlation is measured on a scale from 0 to 1, where 0 indicates no linear relationship between two variables, and 1 indicates a perfect linear relationship. group of answer choices true false
Answers
The statement "correlation is measured on a scale from 0 to 1, where 0 indicates no linear relationship between two variables, and 1 indicates a perfect linear relationship" is true.
The correlation coefficient, typically denoted as r, is a statistical measure that quantifies the strength and direction of the linear relationship between two variables. It ranges from -1 to 1, where -1 represents a perfect negative linear relationship, 1 represents a perfect positive linear relationship, and 0 indicates no linear relationship or correlation between the variables.
A correlation coefficient of 0 means that there is no linear association between the variables being analyzed. As the correlation coefficient moves closer to 1 or -1, it indicates a stronger linear relationship between the variables. Therefore, the scale from 0 to 1 is commonly used to represent the extent of correlation, where 0 signifies no linear relationship and 1 signifies a perfect linear relationship.
learn more about "linear relationship":- https://brainly.com/question/13828699
#SPJ11
Given: f(x) = 0.25(2)*
is this exponential growth or decay?
what is the rate of growth or decay?
what was the initial amount?
Answers
Given the function f(x) = 0.25(2)x, where x represents time, we can determine the rate of growth or decay and the initial amount.
Rate of growth or decay: The general formula for exponential growth or decay is given by f(x) = a(b)x, where a is the initial amount, b is the growth or decay factor, and x is time. We can compare this with the given function f(x) = 0.25(2)x to determine the rate of growth or decay.
In the given function, b = 2, which is greater than 1. This indicates that the function represents exponential growth. Therefore, the rate of growth is 200% per unit of time.Initial amount:The initial amount, a, is the value of the function when x = 0. Substituting x = 0 in the given function f(x) = 0.25(2)x, we get:f(0) = 0.25(2)0= 0.25(1) = 0.25Therefore, the initial amount is 0.25.To summarize, the given function represents exponential growth with a rate of growth of 200% per unit of time and an initial amount of 0.25.
Know more about rate of growth here:
https://brainly.com/question/18485107
#SPJ11
suppose your class has 12 students, and the professor places students into 3 teams of 4 over the course of the semester, with 1 of the 4 students serving as the team lead. how many times does the professor need to form groups such that the 3 team compositions (and team leads) are guaranteed to repeat?
Answers
To guarantee that the 3 team compositions (and team leads) are repeated, the professor would need to form groups a total of 3 times over the course of the semester. Each time the professor forms groups, there would be 3 possible team compositions (since the team lead can change within each group) and after 3 formations, all 3 compositions would have been used and repeated.
To determine the number of possible unique team compositions and team leads that can be formed.
1. First, we'll find the number of ways to choose team leads for each group:
There are 12 students and 3 team leads needed, so there are 12 choose 3 ways to pick the team leads, which is calculated as C(12,3) = 12! / (3! * (12-3)!) = 220.
2. Now, we'll calculate the number of ways to divide the remaining 9 students into 3 groups of 3 students each. We can use the multinomial coefficient formula, which is:
C(9,3,3,3) = 9! / (3! * 3! * 3!) = 1680
3. To find the total number of unique team compositions (including team leads), we multiply the results from steps 1 and 2 Total unique team compositions = 220 * 1680 = 369,600
4. The professor needs to form the groups 369,601 times in order to guarantee that the 3 team compositions (and team leads) will repeat.
Learn more about compositions here: brainly.com/question/5217462
#SPJ11
I need help with this right now
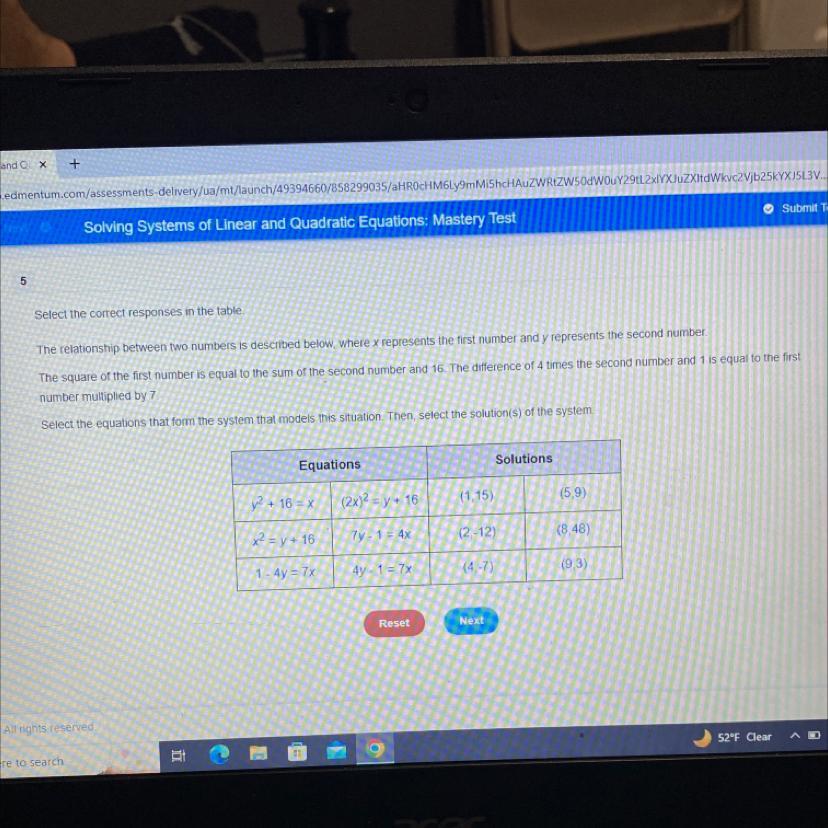
Answers
The equations are x² = y + 16 & 4y - 1 = 7x and the solution is (5, 9)
Selecting the numbers and the solutionsFrom the question, we have the following parameters that can be used in our computation:
x = first number
y = second number
Given that
The square of the first number is 16 more than the second number
This means that
x² = y + 16
Also, we have the difference expression to be
4y - 1 = 7x
When these equations are solved graphicaly, we have
(x, y) = (5, 9)
Hence, the equations are x² = y + 16 & 4y - 1 = 7x and the solution is (5, 9)
Read more about system of equations at
https://brainly.com/question/13729904
#SPJ1
If f(x)=6+5x−5x2, find the equation of the line tangent to the curve f(x) at x=3.
Answers
The equation of the tangent line to the curve f(x) = 6 + 5x − 5x² at x = 3 is y = -25x + 63.
The function given is f(x) = 6 + 5x − 5x². We need to find the equation of the tangent line to the curve at x = 3
.To find the equation of the tangent line at x = 3, we need to find the slope of the tangent at x = 3. The slope of the tangent at a point can be obtained by taking the derivative of the function at that point.
Thus, we first find the derivative of the given function at x = 3.f(x) = 6 + 5x − 5x²
Differentiating f(x) w.r.t x, we get:
f'(x) = 5 - 10x
At x = 3,f'(3) = 5 - 10(3) = -25
Thus, the slope of the tangent at x = 3 is -25.
We also know that the tangent line passes through the point (3, f(3)) = (3, 6 + 5(3) − 5(3)²) = (3, -12).
Using the point-slope form of the equation of a straight line, we can find the equation of the tangent line:
y - y₁ = m(x - x₁), where m is the slope of the line and (x₁, y₁) is a point on the line.
Substituting m = -25, x₁ = 3, and y₁ = -12 in the above equation, we get:y + 12 = -25(x - 3)
Simplifying the above equation, we get:
y = -25x + 63
Thus, the equation of the tangent line to the curve f(x) = 6 + 5x − 5x² at x = 3 is y = -25x + 63.
Learn more about the tangent line at
https://brainly.com/question/31586382
#SPJ11
1A) Quad ABCD is inscribed in the circle. Find x. *
1B) Using your answer from above, what is angle A? *

Answers
Step-by-step explanation:
2x + 9° + 3x + 1° =180° (opposite angles of a cyclic quadrilateral are supplementary)
5x + 10° = 180°
5x = 180° - 10°
5x = 170°
x = 34°
Now
Angle A
= 2 * 34° + 9°
= 77°
Hope it will help :)❤
x² = 64
= positive negative 64? Is that right?
Answers
Answer:
yes that is correct
Step-by-step explanation:
negative times negative always positive
A box of pasta contains 16 ounces of pasta. If on serving weighs 1/3 ounces, how many servings are in one box of pasta
Answers
Explanation: 3 servings for one ounce, so 3 x 16 ounces = 48
ryan drove 260 miles using 12 gallons of gas. at this rate, how many gallons of gas would he need to drive 286 miles?
Answers
The gallons of gas required by Ryan to travel 286 miles is 13.2 gallons.
Define the term inversely proportional?When two parameters are related, an inverse connection exists, where the value about one parameter usually falls as the value of other parameter rises. It's frequently referred to as a bad relationship. When one quantity increases or declines, the other quantity also rises or falls in direct proportion. On the other hand, in indirect and inverse proportion, if such quantity rises, the other one falls, and vice versa.As the stated question;
Total gallon of gas used to drive the 260 miles = 12 gallons
Let 'x' be the gallon of gas used to drive the 286 miles.
Then, bu using the proportion
12 / 260 = x / 286
Thus,
x = 286 x 12 / 260
x = 13.2 gallons
Thus, the amount of gas required by Ryan to travel 286 miles is 13.2 gallons.
To know more about the inversely proportional, here
https://brainly.com/question/1266676
#SPJ4
If you expand and simplify (2x+3)(x+4)
Answers
Answer:
The answer is 2x² + 11x + 12.
Step-by-step explanation:
You have apply Distributive Law,
\(a(m + n) = am + an\)
So for this question :
\((2x + 3)(x + 4)\)
\( = 2x(x) + 2x(4) + 3(x) + 3(4)\)
\( = 2 {x}^{2} + 8x + 3x + 12\)
\( = 2 {x}^{2} + 11x + 12\)
\((2x + 3)(x + 4) \\ = 2x(x + 4) + 3(x + 4) \\ = 2x \times x + 2x \times 4 + 3 \times x + 3 \times 4 \\ = {2x}^{2} + 8x + 3x + 12 \\ = {2x}^{2} + 11x + 12 \\ hope \: it \: helps\)
Henry predicted whether he got answers right or wrong in his 50 question exam.
He identified the 36 questions he thought he got right.
It turns out that Henry got 5 questions wrong that he thought he got correct and he only got 11 of the questions wrong he had predicted.
What is the percentage accuracy he had with predicting his scores?
Answers
Answer:
42/50 x2 =84%
Step-by-step explanation:
Camila wants to buy raspberries and pears to make a fruit tart. Raspberries cost $4 per pound and pears cost $3.25 per pound. How much does she spend if she buys 2.5 pounds of raspberries and 2 pounds of pears? How much does she spend if she buys x pounds of raspberries and y pounds of pears?
Answers
The total cost of raspberries and pears if she buys 2.5 pounds of raspberries and 2 pounds of pears is $16.5.
AlgebraCost of raspberries per pound =$4Cost of pears per pound = $3.25If she buys 2.5 pounds of raspberries and 2 pounds of pearsTotal cost = (Cost of raspberries × pounds of raspberries) + (Cost of pears × pounds of pears)
= (4 × 2.5) + (3.25 × 2)
= 10 + 6.5
= $16.5
if she buys x pounds of raspberries and y pounds of pears?Total cost = (Cost of raspberries × pounds of raspberries) + (Cost of pears × pounds of pears)
= (4 × x) + (3.25 × y)
= 4x + 2y
Therefore, the equation that can be used to represent the total cost of raspberries and pears given the cost per pound of raspberries and pears is 4x + 2y.
Learn more about algebra:
https://brainly.com/question/723406
#SPJ1